Skip to main content
SpringerLink
Log in

Classification of 4-qubit Entangled Graph States According to Bipartite Entanglement, Multipartite Entanglement and Non-local Properties

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

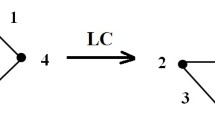
Abstract

We use concurrence to study bipartite entanglement, Meyer-Wallach measure and its generalizations to study multi-partite entanglement and MABK and SASA inequalities to study the non-local properties of the 4-qubit entangled graph states, quantitatively. Then, we present 3 classifications, each one in accordance with one of the aforementioned properties. We also observe that the classification according to multipartite entanglement does exactly coincide with that according to nonlocal properties, but does not match with that according to bipartite entanglement. This observation signifies the fact that non-locality and multipartite entanglement enjoy the same basic underlying principles, while bipartite entanglement may not reveal the non-locality issue in its entirety.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Hein, M., Dur, W., Eisert, J., Raussendorf, R., Van den Nest, M., Briegel, H.J.: Entanglement in graph states and its applications. Proc. Internat. School Phys. Enrico Fermi. Quantum Computers, Algorithms and Chaos. 162, 115 (2006)

    MathSciNet  Google Scholar 

  2. Severini, S.: Two-colorable graph states with maximal Schmidt measure. Phys. Lett. A 356, 99 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Hein, M., Eisert, J., Eisert, H., Briegel, J.: Multiparty entanglement in graph states. Phys. Rev. A 69, 062311 (2004)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Salimi, S., Karami, D., Salimi, E.: Investigation of preparation up to six and n-atom graph states. Int. J. Theor. Phys. 51, 2031 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  5. Schlingemann, D., Werner, R.F.: Quantum error-correcting codes associated with graphs. Phy. Rev. A 65, 012308 (2001)

    Article  ADS  Google Scholar 

  6. Gottesman, D.: A class of quantum error-correcting codes saturating the quantum Hamming bound. Phy. Rev. A 54, 1862 (1996)

    Article  ADS  MathSciNet  Google Scholar 

  7. Briegel, H.J., Raussendorf, R.: Persistent entanglement in arrays of interacting particles. Phys. Rev. Lett. 86, 910 (2001)

    Article  ADS  Google Scholar 

  8. Grassl, M., Klappenecker, A., Rotteler, M.: Graphs, quadratic forms, and quantum codes. arXiv:0703112v1 (2007)

  9. Schlingemann, D.: Quantum error correcting codes and one-way quantum computing towards a quantum memory. arXiv:quant-ph/0308022v1 (2003)

  10. Huang, W., Wei, Z.: Efficient one-way quantum computations for quantum error correction. J. Phys. A: Math. Theor. 42, 295301 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  11. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2010)

    Book  MATH  Google Scholar 

  12. Raussendorf, R., Briegel, H.J.: Quantum computing via measurements only. Phys. Rev. Lett. 86, 5188 (2000)

    Article  ADS  Google Scholar 

  13. Gottesman, D.: Stabilizer codes and quantum error correction. arXiv:9705052v1[quant-ph] (1997)

  14. Van den Nest, M., Dur, W., Miyake, A., Briegel, H.J.: Fundamentals of universality in one-way quantum computation. New J. Phys. 9, 204 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  15. Campbell, E.T., Fitzsimons, J.: An introduction to one-way quantum computing in distributed architectures. Int. J. Quantum Inf. 8, 219 (2010)

    Article  MATH  Google Scholar 

  16. Lee, S.M., Park, H.S., Cho, J., Kang, Y., Lee, J.Y., Kim, H., Lee, D.H., Choi, S.K.: Experimental realization of a four-photon seven-qubit graph state for one-way quantum computation. Opt. Express. 70, 6915 (2012)

    Article  ADS  Google Scholar 

  17. Browne, D., Briegel, H.: One-way quantum computation. arXiv:0603226v2[quant-ph] (2006)

  18. Nielsen, M.A.: Cluster-state quantum computation. Rep. Math. Phys. 57, 147 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Bell, B.A., Tame, M.S., Clark, A.S., Nock, R.W., Wadsworth, W.J., Rarity, J.G.: Experimental characterization of universal one-way quantum computing. New J. Phys. 15, 053030 (2013)

    Article  ADS  Google Scholar 

  20. Dur, W., Aschauer, H., Briegel, H.J.: Multiparticle entanglement purification for graph states. Phys. Rev. Lett. 91, 107903 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  21. Hajdusek, M., Murao, M.: Direct evaluation of pure graph state entanglement. New J. Phys. 15, 013039 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  22. Plesch, M., Buzek, V.: Entangled graphs: bipartite entanglement in multiqubit systems. Phys. Rev. A 67, 012322 (2003)

    Article  ADS  Google Scholar 

  23. Paul, N., Menon, J.V., Karumanchi, S., Muralidharan, S., Panigrahi, P.K.: Quantum tasks using six qubit cluster states. Quant. Inf. Process. 10, 619 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  24. Li, D. C. h., Cao, Z.L.: Teleportation of two-particle entangled state via cluster state. Theor. Phys. 47, 464 (2007)

    Article  ADS  Google Scholar 

  25. Guhne, O., Toth, G., Hyllus, P., Briegel, H.J.: Bell inequalities for graph states. Phys. Rev. Lett. 95, 120405 (2005)

    Article  ADS  MathSciNet  Google Scholar 

  26. Scarani, V., Acin, A., Schenck, E., Aspelmeyer, M.: Nonlocality of cluster states of qubits. Phys. Rev. A 71, 042325 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. Ceccarelli, R., Vallone, G., Martini, F.D., Mataloni, P., Cabello, A.: Experimental entanglement and nonlocality of a two-photon six-qubit cluster state. Phys. Rev. Lett. 103, 160401 (2009)

    Article  ADS  Google Scholar 

  28. Wootters, W.: Entangled chains. arXiv:0001114[quant-ph] (2000)

  29. Dur, W.: Multi-partite entanglement that is robust against disposal of particles. Phys. Rev. A 63, 020303 (2001)

    Article  ADS  Google Scholar 

  30. Koashi, M., Buzek, V., Imoto, N.: Entangled webs. Phys. Rev. A 62, 050302(R) (2000)

    Article  ADS  MathSciNet  Google Scholar 

  31. Akhtarshenas, S.J., GharahiGhahi, M.: Entangled graphs: a classification of four-qubit entanglement. arXiv:1003.2762v1[qunt-ph] (2010)

  32. Love, P.J., Van den Brink, A.M., Smirnov, A. Y., Amin, M.H.S., Grajcar, M., Ilichev, E., Izmalkov, A., Zagoskin, A.M.: A characterization of global entanglement. Quantum. Inf. Process. 6, 187 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  33. Meyer, D.A., Wallach, N.R.: Global entanglement in multiparticle systems. J. Math. Phys. 43, 4273 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. Scott, A.J.: Multi-partite entanglement quantum-error-correcting codes and entangling power of quantum evolutions. Phys. Rev. A 69, 052330 (2004)

    Article  ADS  Google Scholar 

  35. Belinskii, A.V., Klyshko, D.N.: Interference of light and Bell’s theorem. Phys. Ups 36, 653 (1993)

    Google Scholar 

  36. Zukowski, M.: Bell theorem involving all settings of measuring apparatus. Phys. Lett. A, 177 (1993)

  37. Mermin, N.D.: Extreme quantum entanglement in a superposition of macroscopically distinct states. Phys. Rev. Lett. 65, 1838 (1990)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. Ardehali, M.: Bell inequalities with a magnitude of violation that grows exponentially with the number of particles. Phys. Rev. A 46, 5375 (1992)

    Article  ADS  MathSciNet  Google Scholar 

  39. Horodecki, M., Horodecki, P., Horodecki, R.: Separability of mixed states: necessary and sufficient conditions. Phys. Lett. A, 223 (1996)

  40. Rai, S., Luthra, J.R.: Negativity and concurrence for two qutrits. arXiv:0507263v1[quant-ph] (2005)

  41. Wootters, W.: Entanglement of formation and concurrence. Quant. Inf. and Comp. 1(1), 27 (2001)

    MathSciNet  MATH  Google Scholar 

  42. Chunfeng, W. u., Ye, Yeo, Kwek, L.C., Oh, C.H: Quantum nonlocality of four-qubit entangled states. Phys. Rev. A 75, 032332 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  43. Gisin, N, Bechmann-Pasquinucci, H.: Bell inequality, bell states and maximally entangled states for n qubits. Phys. Lett. A, 246 (1998)

  44. Zukowski, M., Brukner, C.: Bell’s theorem for general N-qubit states. arXiv:0102039v3[quant-ph] (2002)

Download references

Author information

Authors and Affiliations

  1. Physics Department, Shahid Chamran University of Ahvaz, Ahvaz, Iran

    Leila Assadi & Mojtaba Jafarpour

Authors
  1. Leila Assadi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mojtaba Jafarpour
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mojtaba Jafarpour.

Appendices

Appendix: 1

Defining

$$\left( {\alpha^{2}+\frac{\gamma^{2}}{2}} \right)^{2}=A, \quad \left( {\beta^{2}+\frac{\gamma^{2}}{2}} \right)^{2}=B, \quad \left( {\alpha^{2}+\frac{\gamma^{2}}{6}} \right)^{2}=C, $$
$$\left( {\beta^{2}+\frac{\gamma^{2}}{6}} \right)^{2}=D, \quad \left( {\alpha^{2}+\frac{3\gamma^{2}}{5}} \right)^{2}=F, \quad \left( {\beta^{2}+\frac{2\gamma^{2}}{5}} \right)^{2}=G, $$
$$\left( {\alpha^{2}+\frac{2\gamma^{2}}{5}} \right)^{2}=H, \quad \left( {\beta^{2}+\frac{3\gamma^{2}}{5}} \right)^{2}=I, \quad \left( {\alpha^{2}+\frac{\gamma^{2}}{5}} \right)^{2}=J, $$
$$\left( {\beta^{2}+\frac{\gamma^{2}}{5}} \right)^{2}=K, \quad \left( {\alpha^{2}+\frac{3\gamma^{2}}{4}} \right)^{2}=L, \quad \left( {\beta^{2}+\frac{\gamma^{2}}{4}} \right)^{2}=M, $$
$$\left( {\alpha^{2}+\frac{\gamma^{2}}{4}} \right)^{2}=N, \quad \left( {\beta^{2}+\frac{3\gamma^{2}}{4}} \right)^{2}=O, \quad \alpha^{4}+\beta^{4}+\gamma^{4}=Z, $$
$$\left( {\alpha^{2}+\frac{\gamma^{2}}{3}} \right)^{2}=P, \quad \left( {\beta^{2}+\frac{2\gamma^{2}}{3}} \right)^{2}=Q, \quad \left( {\alpha^{2}+\frac{2\gamma^{2}}{3}} \right)^{2}=R, $$
$$\gamma^{2}\left( {\alpha +\beta } \right)^{2}=E, \quad \left( {\beta^{2}+\gamma^{2}} \right)^{2}=T, \quad \left( {\alpha^{2}+\gamma^{2}} \right)^{2}=Y, \quad \left( {\beta^{2}+\frac{\gamma^{2}}{3}} \right)^{2}=S, $$

We obtain

Table 5
Full size table

Appendix: 2

Table 5 MABK and SASA inequalities for the 4-qubit entangled graphs
Full size table

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Assadi, L., Jafarpour, M. Classification of 4-qubit Entangled Graph States According to Bipartite Entanglement, Multipartite Entanglement and Non-local Properties. Int J Theor Phys 55, 4809–4821 (2016). https://doi.org/10.1007/s10773-016-3104-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-016-3104-x

Keywords

Search

Navigation