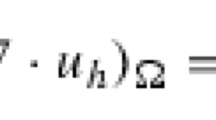Skip to main content
SpringerLink
Log in

Existence and Uniqueness of Very Weak Solutions to the Steady-State Navier–Stokes Problem in Lipschitz Domains

  • Published:
Journal of Mathematical Fluid Mechanics Aims and scope Submit manuscript

Abstract

We prove that in a bounded Lipschitz domain of \({\mathbb {R}}^3\) the steady-state Navier–Stokes equations with boundary data in \(L^2(\partial \Omega )\) have a very weak solution \(\varvec{u}\in L^3(\Omega )\), unique for large viscosity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Amrouche, C., Rodríguez-Bellido, M.A.: Stationary Stokes, Oseen and Navier–Stokes equations with singular data. Arch. Rational Mech. Anal. 199, 597–651 (2011)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  2. Farwig, R., Galdi, G.P., Sohr, H.: A New class of weak solutions of the Navier-Stokes equa- tions with nonhomogeneous data. J. Math. Fluid. Mech. 8, 423–444 (2006)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  3. Farwig, R., Sohr, H.: Existence, uniqueness and regularity of stationary solutions to inhomogeneous Navier-Stokes equations in \({\mathbb{R}}^n\). Czechoslovak Math. J. 59, 61–79 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  4. Galdi, G.P.: An introduction to the mathematical theory of the Navier–Stokes equations. Steady-state problems, 2nd edn. Springer, New York (2011)

  5. Galdi, G.P., Simader, C.G., Sohr, H.: A class of solutions to stationary Stokes and Navier–Stokes equations with boundary data in \(W^{-1/q, q}\). Math. Ann. 331, 41–74 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  6. Kapitanskii, L.V., Pileckas, K.: On spaces of solenoidal vector fields and boundary value problems for the Navier-Stokes equations in domains with noncompact boundaries, Trudy Mat. Inst. Steklov, 159 (1983), 5–36. English Transl.: Proc. Math. Inst. Steklov, 159 (1984)

  7. Kim, H.: Existence and regularity of very weak solutions of the stationary Navier–Stokes equations, Arch. Rational Mech. Anal. 193 (2009), 117–152. Erratum. Arch. Rational Mech. Anal. 196, 1079–1080 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  8. Korobkov, M.V., Pileckas, K., Russo, R.: On the flux problem in the theory of steady Navier–Stokes equations with nonhomogeneous boundary. Arch. Rational Mech. Anal. 207, 185–213 (2013)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  9. Korobkov, M.V., Pileckas, K., Russo, R.: Solution of Leray’s problem for stationary Navier–Stokes equations in plane and axially symmetric spatial domains. Ann. Math. 181, 769–807 (2015)

    Article  MATH  MathSciNet  Google Scholar 

  10. Korobkov, M.V., Pileckas, K., Russo, R.: The existence theorem for steady Navier–Stokes equations in the axially symmetric case. Ann. Scuola Norm. Sup. Pisa Cl. Sci (5) 14, 233–262 (2015)

    MATH  MathSciNet  Google Scholar 

  11. Ladyzhenskaia, O.A.: The Mathematical theory of viscous incompressible fluid. Gordon and Breach (1969)

  12. Leray, J.: Étude de diverses équations intégrales non linéaire et de quelques problèmes que pose l’hydrodynamique. J. Math. Pures Appl. 12, 1–82 (1933)

    MATH  Google Scholar 

  13. Marušić-Paloka, E.: Solvability of the Navier–Stokes system with \(L^2\) boundary data. Appl. Math. Optimization 41, 365–375 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  14. Mitrea, M., Taylor, M.: Navier-Stokes equations on Lipschitz domains in Riemannian manifolds. Math. Ann. 321, 955–987 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  15. Nečas, J.: Direct methods in the theory of elliptic equations. Springer, Berlin (2012)

    MATH  Google Scholar 

  16. Russo, R.: On the existence of solutions to the stationary Navier-Stokes equations. Ricerche Mat. 52, 285–348 (2003)

    MATH  MathSciNet  Google Scholar 

  17. Russo, R.: On Stokes’ problem. In: Rannacher, R., Sequeira, A. (eds.) Advances in mathematica fluid mechanics, pp. 473–511. Springer-Verlag, Berlin (2010)

    Chapter  Google Scholar 

  18. Russo, A., Starita, G.: On the existence of steady-state solutions to the Navier–Stokes equations for large fluxes. Ann. Scuola Norm. Sup. Pisa Cl. Sci (5) 7, 171–180 (2008)

    MATH  Google Scholar 

  19. Russo, A., Tartaglione, A.: On the Stokes problem with data in \(L^1\). ZAMP 64, 1327–1336 (2013)

    ADS  MATH  MathSciNet  Google Scholar 

  20. Schumacher, K.: Very weak solutions to the stationary Stokes and Stokes resolvent problem in weighted function spaces. Ann. Univ. Ferrara 54, 123–144 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  21. Serre, D.: Équations de Navier-Stokes stationnaires avec données peu régulières. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 10, 543–559 (1983)

    MATH  Google Scholar 

  22. Shen, Z.: A note on the Dirichlet problem for the Stokes system in Lipschitz domains. Proc. Am. Math. Soc. 123, 801–811 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  23. Sohr, H.: The Navier–Stokes equations. An elementary functional analytic approach. Birkhäuser Verlag, Switzerland (2001)

    MATH  Google Scholar 

  24. Stein, E.: Harmonic analysis: real-variable methods, orthogonality, and oscillatory integrals. Princeton University Press, Princeton (1993)

    MATH  Google Scholar 

  25. Tartaglione, A.: On the Stokes and Oseen problems with singular data. J. Math. Fluid Mech. 16, 407–417 (2014)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  26. Temam, R.: Navier-Stokes equations. North-Holland (1977)

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica e Informatica, Università di Ferrara, Via Machiavelli 35, 44121, Ferrara, Italy

    Vincenzo Coscia

Authors
  1. Vincenzo Coscia
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vincenzo Coscia.

Additional information

Communicated by K. Pileckas

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Coscia, V. Existence and Uniqueness of Very Weak Solutions to the Steady-State Navier–Stokes Problem in Lipschitz Domains. J. Math. Fluid Mech. 19, 819–829 (2017). https://doi.org/10.1007/s00021-016-0307-0

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00021-016-0307-0

Mathematics Subject Classification

Keywords

Search

Navigation