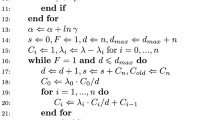Summary
Another bivariate generalisation (Type V) of the non-central negative binomial distribution is considered. This generalisation is constructed (i) as a latent structure model; (ii) as an extension of an accident proneness model investigated by Edwards/Gurland (1961); and (iii) as a reversible stochastic counter model. The third construction gives, as a result, an apparently new formulation of the Edwards/Gurland model. The probabilities, moments, recurrence formulas and some properties are given. An application to the data used by Holgate (1966) is considered.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Chihara TS (1978) An introduction to orthogonal polynomials. New York
Consael R (1952) Sur les processus composés de Poisson á deux variables aléatoires. Académie Royale de Belgique, Classe des Sciences, Mémoires 27:4–43
Edwars CB, Gurland J (1961) A class of distributions applicable to accidents. J Amer Statist Ass. 56:503–517
Erdélyi A (1953) Higher transcendental functions, vol II. McGraw Hill
Goodman LA (1974) Exploratory latent structure analysis using both identifiable and unidentifiable models. Biometrika 61:215–231
Holgate P (1964) Estimation for the bivariate Poisson distribution. Biometrika 51:241–245
Holgate P (1966) Bivariate generalizations of Neyman's typeA distribution. Biometrika 53:241–245
Johnson NL (1957) Uniqueness of a result in the theory of accident proneness. Biometrika 44:530–531
Kemp AW (1968) A wide class of discrete distributions and the associated differential equations. Sankhya A 30:401–410
Kemp CD, Kemp AW (1965) Some properties of the Hermite distribution. Biometrika 52:381–394
Laha RG (1954) On some properties of the Bessel function distributions. Bull Calcutta Math Soc 46:59–72
Lai CD (1978) A note on central limit theorem for the Wold's Markov process of gamma intervals. Tamkang J Math 9 (2):209–215
Lai CD (1981) On conditional correlation coefficients of a Wold Markov process of gamma intervals. Aust J Statist 23 (2):232–237
Lampard DG (1965) A stochastic process whose successive intervals between events form a first order Markov chain — I. Report No. MEE 65-1. Electrical Engineering Dept., Monash University, Australia
Lampard DG (1968) A stochastic process whose successive intervals between events form a first order Markov chain — I. J Appl Prob 5:648–668
Lee PA (1968) A study of some stochastic processes in Communication Engineering. PhD Thesis Monash Unviersity, Australia
Lee PA, Ong SH (1981) The bivariate non-central negative binomial distributions. Research Report 12/81, Dept. of Mathematics, University of Malaya, Malaysia (to appear in Metrika)
Mitchell CR, Paulson AS (1981) A new bivariate negative binomial distribution. Navals Res Logistics Quart 28:359–374
Ong SH, Lee PA (1979) The non-central negative binomial distribution. Biom J 21:611–627
Ong SH, Lee PA (1982) On the bivariate negative binomial distribution of Mitchell and Paulson. Research Report 5/82, Dept. of Mathematics, University of Malaya, Malaysia, (to appear in Navals Res Logistics Quart 1985)
Phatarfod RM (1971) Some approximate results in renewal and dam theory. J Aust Math Soc 12:425–432
Plunkett IG, Jain GC (1975) Three generalised negative binomial distributions. Biom J 17: 286–302
Sneddon IN (1961) Special functions of mathematical physics and chemistry. Edinburgh
Subrahmaniam Ko (1966) A test for “intrinsic correlation” in the theory of accident proneness. J R Statist Soc B 28:180–189
Subrahmaniam Ko, Subrahmaniam Ka (1973) On the estimation of the parameters in the bivariate negative binomial distribution. J R Statist Soc B 35:131–146
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Ong, S.H., Lee, P.A. Bivariate non-central negative binomial distribution: Another generalisation. Metrika 33, 29–46 (1986). https://doi.org/10.1007/BF01894724
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01894724