Abstract
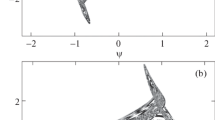
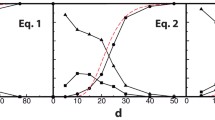
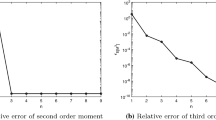
A delay-differential equationɛu(t)+u(t)=f(u(t−1)), 0⩽t < ∞, and its generalization are investigated in the limitɛ → 0, when the attractor's dimension increases infinitely. It is shown that a number of statistical characteristics are asymptotically independent ofɛ. As for the attractor, it can be regarded as a direct product ofO(1/ɛ) equivalent “subattractors,” their statistical characteristics being asymptotically independent of ɛ. The results enable one to predict some characteristics of the attractor with fractal dimensionD ≫ 1 for the caseɛ ≪ 1, when they are inaccessible numerically. The approach developed seems to be applicable for a wide class of spatiotemporal systems.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
J. D. Farmer, Chaotic attractors of infinite-dimensional systems,Physica 4D:366–392 (1982).
K. Ikeda and K. Matsumoto, High-dimensional chaotic behavior in systems with delayed feedback,Physica 29D:223–236 (1987).
K. Ikeda, K. Kondo, and O. Akimoto, Successive higher-harmonic bifurcations in systems with delayed feedback,Phys. Rev. Lett. 49:1467–1470 (1982).
K. Ikeda and K. Matsumoto, Study of a high dimensional chaotic attractor,J. Stat. Phys. 44:955–982 (1986).
F. A. Hopf, D. L. Kaplan,et al., Bifurcations to chaos in optical bistability,Phys. Rev. 25a:2172–2183 (1982).
B. Dorrizi, B. Grammaticos, M. Berre, Y. Pomeau, E. Ressayre, and A. Tallet, Statistics and dimension of chaos in differential delay systems,Phys. Rev. 35a:328–339 (1987).
A. N. Sharkovsky, Yu. L. Maistrenko, and E. Yu. Romanenko,Difference Equations and Their Applications (1986) [in Russian],
J. Mallet-Parret and R. Nussbaum, A bifurcation gap for a singularly perturbed delay equation, inChaotic Dynamics and Fractals (1986).
J.-P. Eckmann and D. Ruelle, Ergodic theory of chaos and strange attractors,Rev. Mod. Phys. 57:617–656 (1985).
R. Shaw, Strange attractors, chaotic behavior and information flow,Z. Naturforsch. 36a:80–112 (1981).
H.-G. Schuster,Deterministic Chaos (Physik Verlag, Weiheim, 1984).
S. V. Ershov and G. G. Malinetsky, On the solution of the direct and inverse problem for the Frobenius-Perron equation, IAM preprint # 166 (1988) [in Russian].
H. Haken and G. Mayer-Kress, Influence of noise on the logistic model,J. Stat. Phys. 26:149–171 (1981).
J. L. Doob,Stochastic Processes (1953).
J. I. Kifer, On small random perturbations of some smooth dynamical systems,Math. USSR Izv. 8:1083 (1974).
K. Kaneko, Pattern dynamics in spatio-temporal chaos,Physica 34D:1–41 (1989).
A. S. Pikovsky, Spatial development of chaos in nonlinear media,Phys. Lett. A 137:121–127 (1989).
R. J. Deissler, Noise-sustained structure, intermittency and the Ginzburg-Landau equation,J. Stat. Phys. 40:371–395 (1988).
R. J. Deissler, External noise and the origin and dynamics of structure in convectively unstable systems,J. Stat. Phys. 54:1458–1488 (1989).
H. Daido, Coupling sensitivity of chaos: Theory and further numerical evidence,Phys. Lett. A 121A:60–66 (1988).
J. P. Crutchfield and K. Kaneko, Are attractors relevant to turbulence?Phys. Rev. Lett. 60:2715–2718 (1988).
S. V. Ershov and A. B. Potapov, On the nature of nonchaotic turbulence, IAM preprint #7 (1991) [in Russian];Phys. Lett. A, submitted.
C. Grebogi, E. Ott, and J. A. Yorke, Crises, sudden changes in chaotic attractors and transient chaos,Physica 7D:181–200 (1983).
T. Schreiber, Spatio-temporal structure in coupled map lattices: Two-point correlations versus mutual information,J. Phys. A 23A:L393–398 (1990).
F. Hofbauer and G. Keller, Ergodic properties of invariant measures of piecewise monotonic transformations,Math. Z. 180:119–140 (1980).
V. L. Volevich, Kinetics of coupled map lattices,Nonlinearity 4:37–48 (1991).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Ershov, S.V. Asymptotic theory of multidimensional chaos. J Stat Phys 69, 781–812 (1992). https://doi.org/10.1007/BF01050434
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01050434