A Delayed Impulse Control Strategy for Spacecraft Formations

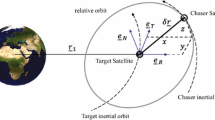
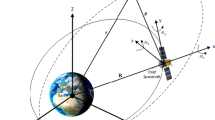
Abstract

An impulsive control strategy for spacecraft formation flying is suggested considering secular drift between satellites. The drift motion caused by orbital period difference affects impulsive correction. Preventing the drift has been treated as a natural effort in most of formation flying researches. However, this study proposes preserving the drift behavior by delaying the period-matching maneuver. The paper shows that the impulse delay could be effective under some conditions by reducing the required delta-v. Two impulsive control methods are designed by harnessing the drift in pure Keplerian orbits. By using a linear approximation, the proposed methods avoid iterative steps for obtaining the required impulse, so the new strategy can be implemented with less computational burden compared to numerical optimal solutions. Impulse magnitudes between an existing method and the proposed strategy are compared mathematically and the numerical simulation verifies that the impulse reduction could be achieved with the suggested methods.

Appendices

Appendix A: Tangential Impulse Difference in Case A

In Appendix A, the |Δv _θ |_diff between DIM and AIM in Case A are derived. For convenience, the tangential impulses of both methods in Eqs. 75 and 76 are rewritten as

$$\begin{array}{@{}rcl@{}} \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{DIM}} &=&\left| {\Delta \mathit{v}_{\theta_{\text{in}} } } \right|_{\text{DIM}} \\ &=&\frac{n_{0} a_{0} \eta }{4}\left( {\left| {\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }+\frac{\Delta e}{1+e}} \right|+\left| {\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }-\frac{\Delta e}{1-e}} \right|} \right) \end{array} $$

$$\begin{array}{@{}rcl@{}} \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{AIM}} &=&\left| {\Delta \mathit{v}_{\theta_{\text{add}} } } \right|_{\text{AIM}} +\left| {\Delta \mathit{v}_{\theta_{\text{in}} } } \right|_{\text{AIM}} \\ &=&\frac{n_{0} \eta }{2}\left( {a_{\text{add}} -a_{0} } \right)+\frac{n_{0} a_{0} \eta }{4}\left( {\left| {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\frac{\Delta e}{1+e}} \right|+\left| {\frac{\Delta a_{\text{add,d}} }{a_{0} }-\frac{\Delta e}{1-e}} \right|} \right) \\ \end{array} $$

The $\left | {\Delta \mathit {v}_{\theta _{\text {in}} } } \right |_{\text {DIM}}$ and $\left | {\Delta \mathit {v}_{\theta _{\text {in}} } } \right |_{\text {AIM}}$ depend on the four absolute terms consisting of the Δe. The Δe can be random in each mission, and for generality, the |Δv _θ |_DIM and |Δv _θ |_AIM for all possible Δe should be derived. The four Δe criteria, which make the absolute terms in Eqs. 75 and 76 be zero, are defined by

$$ {\Delta} e_{1} =-(1+e)\frac{\Delta a_{0,\mathrm{d}} }{a_{0} } $$

(99)

$$ {\Delta} e_{2} =(1-e)\frac{\Delta a_{0,\mathrm{d}} }{a_{0} } $$

(100)

$$ {\Delta} e_{3} =-(1+e)\frac{\Delta a_{\text{add,d}} }{a_{0} } $$

(101)

$$ {\Delta} e_{4} =(1-e)\frac{\Delta a_{\text{add,d}} }{a_{0} } $$

(102)

In Case A, the magnitude order of the Δe criteria can be determined by using the inequality a _add>a ₀>a _d in Eq. 74:

$$ \text{in}{\kern 1pt}\text{Case}{\kern 1pt}\mathrm{A}:{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\Delta} e_{4} <{\Delta} e_{2} <{\Delta} e_{1} <{\Delta} e_{3} $$

(103)

Then, the Δe region can be divided into five intervals as

$$ {\Delta} e_{\text{CaseA}} =\left\{ {{\begin{array}{c} {\Delta e<{\Delta} e_{4} } \\ {\Delta e_{4} \le {\Delta} e<{\Delta} e_{2} } \\ {\Delta e_{2} \le {\Delta} e<{\Delta} e_{1} } \\ {\Delta e_{1} \le {\Delta} e<{\Delta} e_{3} } \\ {\Delta e_{3} \le {\Delta} e} \\ \end{array} }} \right. $$

(104)

Now, the five |Δv _θ |_DIM and |Δv _θ |_AIM for each Δe interval are derived. Then, the smallest |Δv _θ |_diff is extracted. Because the |Δv _θ |_diff derivation procedures for the five intervals are the same, the |Δv _θ |_diff with the first Δe interval is only derived in detail. The derivations of the rest intervals are omitted and only the final results are shown. When the Δe is less than Δe ₄, the |Δv _θ | of DIM and AIM are obtained as

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{DIM},{\Delta} e<{\Delta} e_{4} } =\frac{n_{0} a_{0} \eta }{4}\left( {-\left( {\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }+\frac{\Delta e}{1+e}} \right)+\left( {\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }-\frac{\Delta e}{1-e}} \right)} \right) $$

(105)

$$\begin{array}{@{}rcl@{}} \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{AIM},{\Delta} e<{\Delta} e_{4} } &=&\frac{n_{0} \eta }{2}\left( {a_{\text{add}} -a_{0} } \right)+\frac{n_{0} a_{0} \eta }{4}\\&&\times\left( {-\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\frac{\Delta e}{1+e}} \right)+\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }-\frac{\Delta e}{1-e}} \right)} \right) \end{array} $$

(106)

The difference of |Δv _θ | between DIM and AIM become

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e<{\Delta} e_{4} } =-\frac{n_{0} \eta }{2}\left( {a_{\text{add},2} -a_{0} } \right) $$

(107)

The |Δv _θ |_diff for the other Δe intervals can be achieved by

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{4} \le {\Delta} e<{\Delta} e_{2} } =-\frac{n_{0} \eta }{2}\left( {a_{\text{add}} -a_{0} } \right)-\frac{n_{0} a_{0} \eta }{4}\left( {-2\frac{\Delta a_{\text{add,d}} }{a_{0} }+2\frac{\Delta e}{1-e}} \right) $$

(108)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{2} \le {\Delta} e<{\Delta} e_{1} } =-n_{0} \eta \left( {a_{\text{add}} -a_{0} } \right) $$

(109)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{1} \le {\Delta} e<{\Delta} e_{3} } =-\frac{n_{0} \eta }{2}\left( {a_{\text{add}} -a_{0} } \right)+\frac{n_{0} a_{0} \eta }{4}\left( {2\frac{\Delta a_{\text{add,d}} }{a_{0} }+2\frac{\Delta e}{1+e}} \right) $$

(110)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{3} \le {\Delta} e} =-\frac{n_{0} \eta }{2}\left( {a_{\text{add}} -a_{0} } \right) $$

(111)

Finally, the smallest |Δv _θ |_diff in Case A can be obtained from Eqs. 107–111 as

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff,A}} =-n_{0} \eta \left( {a_{\text{add}} -a_{0} } \right) $$

(112)

Appendix B: Tangential Impulse Difference in Case C

The |Δv _θ |_diff in Case C for the various Δe are presented. The four Δe criteria in |Δv _θ | of DIM and AIM are defined in Eqs. 99–102. However, unlike Eq. 103 in Case A, the magnitude order of the criteria is not strictly fixed and it depends on ${\Delta } M_{\min }^{\prime }$. There are three possible cases:

$$ \left\{ {{\begin{array}{c} {\text{in}{\kern 1pt}\text{Case}{\kern 1pt}\mathrm{C}_{{1}} :{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\Delta} e_{{3}} <{\Delta} e_{{2}} <{\Delta} e_{{1}} <{\Delta} e_{{4}} {\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}\text{if}{\kern 1pt}{\Delta} M_{\min }^{\prime} <{\Delta} M_{\mathrm{C}_{{1}} -\mathrm{C}_{{2}} }^{\prime} } \\ {\text{in}{\kern 1pt}\text{Case}{\kern 1pt}\mathrm{C}_{{2}} :{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\Delta} e_{{3}} <{\Delta} e_{{2}} <{\Delta} e_{{4}} \le {\Delta} e_{{1}} {\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}\text{if}{\kern 1pt}{\Delta} M_{\mathrm{C}_{{1}} -\mathrm{C}_{{2}} }^{\prime} \le {\Delta} M_{\min }^{\prime} <{\Delta} M_{\mathrm{C}_{{2}} -\mathrm{C}_{{3}} }^{\prime} } \\ {\text{in}{\kern 1pt}\text{Case}{\kern 1pt}\mathrm{C}_{{3}} :{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\Delta} e_{{2}} \le {\Delta} e_{{3}} <{\Delta} e_{{4}} <{\Delta} e_{{1}} {\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}{\kern 1pt}\text{if}{\kern 1pt}{\Delta} M_{\mathrm{C}_{{2}} -\mathrm{C}_{{3}} }^{\prime} \le {\Delta} M_{\min }^{\prime} } \\ \end{array} }} \right. $$

(113)

where

$$ {\Delta} M_{\mathrm{C}_{{1}} -\mathrm{C}_{{2}} }^{\prime} =\left( {\frac{-Ne-e-E-N+1}{1-e}} \right){\Delta} M_{\text{half}} $$

(114)

$$ {\Delta} M_{\mathrm{C}_{{2}} -\mathrm{C}_{{3}} }^{\prime} =\left( {\frac{Ne+e-E-N+1}{1+e}} \right){\Delta} M_{\text{half}} $$

(115)

The ${\Delta } M_{\mathrm {C}_{{1}} -\mathrm {C}_{{2}} }^{\prime }$ is the ${\Delta } M_{\min }^{\prime }$ boundary when Δe ₄ = Δe ₁, and the ${\Delta } M_{\mathrm {C}_{{2}} -\mathrm {C}_{{3}} }^{\prime }$ is the ${\Delta } M_{\min }^{\prime }$ boundary when Δe ₃ = Δe ₂. Now, the smallest |Δv _θ |_diff for the three cases in Eq. 113 are derived. Again, as Appendix A, the |Δv _θ |_diff derivation procedures of the three cases are the same. The first case, Case C₁, is only analyzed in detail, and the final results are only given for the other cases. In Case C₁, the Δe region can be divided into five intervals by

$$ {\Delta} e_{\text{CaseC}_{{1}} } =\left\{ {{\begin{array}{c} {\Delta e<{\Delta} e_{{3}} } \\ {\Delta e_{{3}} \le {\Delta} e<{\Delta} e_{{2}} } \\ {\Delta e_{{2}} \le {\Delta} e<{\Delta} e_{{1}} } \\ {\Delta e_{{1}} \le {\Delta} e<{\Delta} e_{{4}} } \\ {\Delta e_{{4}} \le {\Delta} e} \\ \end{array} }} \right. $$

(116)

Applying each Δe condition into the |Δv _θ | of DIM and AIM in Eqs. 99–102, the |Δv _θ |_diff for the five intervals can be derived by

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e<{\Delta} e_{3} } =-\frac{n_{0} \eta }{2}{\Delta} a_{0,\text{add}} $$

(117)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{3} \le {\Delta} e<{\Delta} e_{2} } =-\frac{n_{0} \eta }{2}{\Delta} a_{0,\text{add}} -\frac{n_{0} a_{0} \eta }{2}\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\frac{\Delta e}{1+e}} \right) $$

(118)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{2} \le {\Delta} e<{\Delta} e_{1} } =-\frac{n_{0} \eta }{2}{\Delta} a_{0,\text{add}} -\frac{n_{0} a_{0} \eta }{2}\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }+\left( {\frac{1}{1+e}-\frac{1}{1-e}} \right){\Delta} e} \right) $$

(119)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e_{1} \le {\Delta} e<{\Delta} e_{4} } =-\frac{n_{0} \eta }{2}{\Delta} a_{0,\text{add}} -\frac{n_{0} a_{0} \eta }{2}\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }-\frac{\Delta e}{1-e}} \right) $$

(120)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff},{\Delta} e\le {\Delta} e_{4} } =-\frac{n_{0} \eta }{2}{\Delta} a_{0,\text{add}} $$

(121)

where the Δa _0,add = Δa _add−Δa ₀. The minimum |Δv _θ |_diff in Case C₁ is obtained at Δe = Δe ₂ and the value is

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff,C}_{1} } =-\frac{n_{0} \eta }{2}\left( {a_{0} -a_{\text{add}} } \right)-\frac{n_{0} a_{0} \eta }{2}\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\left( {\frac{1-e}{1+e}} \right)\frac{\Delta a_{\text{0,d}} }{a_{0} }} \right) $$

(122)

The smallest |Δv _θ |_diff for Cases C₂ and C₃ could be obtained by the same approach of Case C₁.

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff,C}_{2} } =-\frac{n_{0} \eta }{2}\left( {a_{0} -a_{\text{add}} } \right)-\frac{n_{0} a_{0} \eta }{2}\left( {\frac{\Delta a_{\text{add,d}} }{a_{0} }+\left( {\frac{1-e}{1+e}} \right)\frac{\Delta a_{0,\mathrm{d}} }{a_{0} }} \right) $$

(123)

$$ \left| {\Delta \mathit{v}_{\theta } } \right|_{\text{diff,C}_{3} } =-\frac{n_{0} \eta }{2}\left( {a_{0} -a_{\text{add}} } \right) $$

(124)

where the $\left | {\Delta \mathit {v}_{\theta } } \right |_{\text {diff,C}_{2} }$ is equal to the $\left | {\Delta \mathit {v}_{\theta } } \right |_{\text {diff,C}_{1} }$, and there exists the two minimum |Δv _θ |_diff candidates of Eqs. 122 and 124 in Case C.