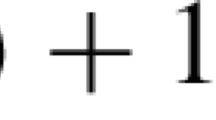Abstract
The existence of solution of the nonlinear Schrödinger equation
is stablished in \(\mathbb {R}^N\), where V changes sign and f is an asymptotically linear function at infinity, with V and f non periodic in x. Spectral theory, a classical linking theorem and interaction between translated solutions of the problem at infinity are employed.
Similar content being viewed by others
References
Ackermann, N., Clapp, M., Pacella, F.: Alternating sign multibump solutions of nonlinear elliptic equations in expanding tubular domains. Commun. Partial Differ. Equ. 38(5), 751–779 (2013)
Azzollini, A., Pomponio, A.: On the Schrödinger equation in \(\mathbb{R}^N\) under the effect of a general nonlinear term. Indiana Univ. Math. J. 58, 1361–1378 (2009)
Berestycki, H., Lions, P.L.: Nonlinear scalar field equations. I. Existence of a ground state. Arch. Ration. Mech. Anal. 82, 313–345 (1983)
Cerami, G.: Un criterio di ezistenza per i punti critici su varietà illimitate. Rend. Accad. Sci. Lett. Inst. Lombardo 112, 332–336 (1978)
Costa, D.G., Tehrani, H.: Existence and multiplicity results for a class of Schrödinger equations with indefinite nonlinearities. Adv. Differ. Equ. 8, 1319–1340 (2003)
Egorov, Y., Kondratiev, V.: On spectral theorey of elliptic operators. Birkhäuser, Basel (1996)
Jeanjean, L.: On the existence of bounded Palais-Smale sequences and application to a Landesman-Lazer-type problem set on \(\mathbb{R}^N\). Proc. R. Soc. Edinburgh Sect. A 129, 787–809 (1999)
Jeanjean, L., Tanaka, K.: A positive solution for an asymptotically linear elliptic problem on \(\mathbb{R}^N\) autonomous at infinity. ESAIM Control Optim. Calc. Var. 7, 597–614 (2002)
Kryszewski, W., Szulkin, A.: Generalized linking theorem with an application to semilinear Schrödinger equations. Adv. Differ. Equ. 3, 441–472 (1998)
Li, G., Szulkin, A.: An asymptotically periodic Schrödinger equation with indefinite linear part. Commun. Contemp. Math. 4, 763–776 (2002)
Li, G., Wang, C.: The existence of a nontrivial solution to a nonlinear elliptic problem of linking type without the Ambrosetti-Rabinowitz condition. Annales Academiae Scientiarum Fennicae 36, 461–480 (2011)
Lions, P.L.: The concentration-compactness principle in the calculus of variations. The locally compact case. Parts I and II, Ann. Inst. H. Poincaré Anal. Non Linéaire, 1, 109–145 and 223–283 (1984)
Maia, L.A, Oliveira Junior, J.C., Ruviaro, R.: A non periodic and asymptotically linear indefinite variational problem in \({\mathbb{R}^N}\), online Indiana University Mathematics Journal (2015)
Pankov, A.A.: Periodic nonlinear Schrödinger equation with application to photonic crystals. Milan J. Math. 73, 259–287 (2005)
Rabinowitz, P.H.: Some critical point theorems and applications to semilinear elliptic partial differential equations. Ann. Scuola Norm. Sup. Pisa Cl. Sci. 5, 215–223 (1978)
Stuart, C.A.: An introduction to elliptic equation in \({\mathbb{R}^N}\), Trieste Notes (1998)
Stuart, C.A., Zhou, H.S.: Applying the mountain pass theorem to an asymptotically linear elliptic equation on \(\mathbb{R}^N\). Commun. Partial Differ. Equ. 24, 1731–1758 (1999)
Szulkin, A., Weth, T.: Ground state solutions for some indefinite variational problems. J. Func. Anal. 257, 3802–3822 (2009)
Acknowledgments
The authors thank Professor Charles A. Stuart for elucidating many doubts in the spectral theory employed in the development of this work.
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Professor Paul H. Rabinowitz.
Research of the first and third authors are partially supported by CNPq/Brazil, PROEX/CAPES and FAPDF 193.000.939/2015.
Appendix
Appendix
Let \(\partial B_1\) be the boundary of \(B_1\), where \(B_1\) is the open ball of radius 1 in a finite dimensional space spanned by the functions \(u_0^+(\cdot -y),\phi _1,\ldots ,\phi _k\). We want to prove that
uniformly for \(u\in \partial B_1\). Indeed, for each \(R=n\in \mathbb {N}\), consider \(J_n:\partial B_1 \rightarrow \mathbb {R}\) the functional given by \(J_n(u) = \displaystyle \int _{\mathbb {R}^N}\left( \displaystyle \frac{a(x)}{2} - \displaystyle \frac{F(x,nu)}{(nu)^2} \right) u^2dx\). The continuity of the function F shows that \(J_n\) is a continuous functional for each fixed n. Hypothesis \((f_4)\) and equivalence of the norms \(\Vert \cdot \Vert \) and \(\Vert \cdot \Vert _E\) show that there exists a constant \(C>0\) such that
for all \(u\in \partial B_1\), where \(a_0=\sup _{\mathbb {R}^N} a(x)\). Hence the continuity of the functional \(J_n\) in the compact set \(\partial B_1\) ensures that, for each fixed n, the functional \(J_n\) assumes its maximum at \(u_n\in \partial B_1\). Consider \((u_n)\) the sequence of these maxima. Since \(\Vert u_n\Vert =1\) for each n and the space spanned by the functions \(u_0^+(\cdot -y),\phi _1,\ldots ,\phi _k\) is finite dimensional, there exists \(\overline{u}\in \partial B_1\) such that, up to a subsequence,
strongly in the norm \(\Vert \cdot \Vert \). For all \(u\in \partial B_1\) and for each n
that is,
for all u and for each n. Taking the limit \(n\rightarrow \infty \), firstly, note that
Thus, if \(\overline{u}(x)\ne 0\), it follows that \(|n\overline{u}(x)|\rightarrow \infty \) if \(n\rightarrow \infty \). Hence hypothesis \((f_4)\) yields
if \(n\rightarrow \infty \). If \(\overline{u}(x) = 0\), we also have (5.3). By the strongly convergence in (5.1), there exist a function \(\overline{h}\in L^1(\mathbb {R}^N)\) such that, up to a subsequence,
Finally, by (5.3) and (5.4), Lebesgue Dominated Convergence Theorem ensures that
Therefore taking \(n\rightarrow \infty \) in (5.2), we have
uniformly for \(u\in \partial B_1\). \(\square \)
Rights and permissions
About this article
Cite this article
Maia, L.A., Oliveira Junior, J.C. & Ruviaro, R. Nonautonomous and non periodic Schrödinger equation with indefinite linear part. J. Fixed Point Theory Appl. 19, 17–36 (2017). https://doi.org/10.1007/s11784-016-0346-4
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11784-016-0346-4