Skip to main content

Advertisement

SpringerLink
Log in

Heterogeneous Productivity and the Gains from Trade and FDI

  • Research Article
  • Published:
Open Economies Review Aims and scope Submit manuscript

Abstract

Nontraded goods account for a major share of GDP in most economies, but have not been incorporated in the welfare analysis of monopolistic-competition models with heterogeneous productivity. This paper extends Helpman, Melitz and Yeaple (American Economic Review 94(1):300–316, 2004) to explore welfare effects in the presence of a nontraded good. We derive new analytical results about how the gains from trade and FDI are determined and affected by key parameters in the case of symmetric countries. The model is calibrated to a country group that includes all major developed countries. The gains from openness (trade and FDI) are found to be substantial (between 3.24 and 6.27 per cent of income) even if nontraded goods represent a major part of the economy. Most of these gains are attributed to trade rather than FDI.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

Notes

  1. For other early contributions to this theory, see, for example, Dixit and Norman (1980, chapter 9), Lancaster (1980) and Helpman (1981). The term new trade theory is also used to include models with other forms of imperfect competition.

  2. Trade can also lead to gains from economies of scale. These gains, however, are not realized in the standard model with CES preference where the output of the firm (determined by the zero profit condition) is constant.

  3. See Eaton and Kortum (2002) for an alternative approach that allows for heterogeneous productivity across countries based on a Ricardian framework with perfect competition.

  4. Models with FDI also use this variant (Helpman et al. 2004; Chor 2009)

  5. This setup has been used in open-economy models with endogenous growth (Rivera-Batiz and Romer 1991a, b) as well as related models of FDI spillovers (Borensztein et al. 1998). Variants of this setup have also been used in the traditional trade models to examine the role of intermediate goods (Helpman and Krugman 1985, chapter 11) and producer services (van Marrewijk et al. 1997).

  6. A more refined definition of the nontraded sector would exclude some services with significant trade and include some products with little or no trade.

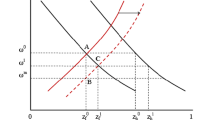
  7. Trade without FDI is possible in the model if (fixed) FDI costs are very large. Similarly, very large FDI and (fixed or variable) trade costs could result in autarky. We do not consider the possibility of FDI without trade because such a state would require very large trade costs. This case is precluded by a restriction on these costs (discussed in Section 2) motivated by the stylized fact that firms that engage in FDI are more productive than exporting firms.

  8. This result for the gains from trade is different from the implications of the basic (one differentiated-good) models with homogeneous or heterogeneous productivity. For example, an increase in the substitution elasticity reduces the gains from trade in the Krugman (1980) model, and has no effect on these gains in the Melitz (2003) model (e.g., see Feenstra 2009).

  9. Broda and Weinstein (2006) estimate gains from the availability of increased product varieties within the homogeneous-productivity framework. Additional gains from heterogeneous productivity are suggested by studies that find evidence of productivity improvement due to the selection effect of trade liberalization (Trefler 2004) and expansion of export varieties (Feenstra and Kee 2008).

  10. For example if instead of (1), we assume that C is produced according to the production function, C = L N 1−α Z α/[α α(1 − α 1−α], we would still get relations (5)–(7), and relations (2)–(4) would be unchanged.

  11. Note that in this case, π HE  < 0 for \( \theta = {\overline{\theta }_H} \), and π HI  < π HE for \( \theta = {\overline{\theta }_{{HE}}} \).

  12. Aggregating over the relevant productivity range, these indexes are defined as \( {\widetilde{\theta }_H} \equiv {\left[ {\int_{{{{\overline{\theta }}_H}}}^{\infty } {\frac{{{\theta^{{\sigma - 1}}}g(\theta )}}{{1 - G({{\overline{\theta }}_H})}}d\theta } } \right]^{{1/(\sigma - 1)}}},{\widetilde{\theta }_{{HE}}} \equiv {\left[ {\int_{{{{\overline{\theta }}_{{HE}}}}}^{{{{\overline{\theta }}_{{HI}}}}} {\frac{{{\theta^{{\sigma - 1}}}g(\theta )}}{{G({{\overline{\theta }}_{{HI}}}) - G({{\overline{\theta }}_{{HE}}})}}d\theta } } \right]^{{1/(\sigma - 1)}}} \), \( {\widetilde{\theta }_{{HI}}} \equiv {\left[ {\int_{{{{\overline{\theta }}_{{HI}}}}}^{\infty } {\frac{{{\theta^{{\sigma - 1}}}g(\theta )}}{{1 - G({{\overline{\theta }}_{{HI}}})}}d\theta } } \right]^{{1/(\sigma - 1)}}} \), where G(θ) is the cumulative distribution function.

  13. To derive the relation for p H , for example, use the definition of p H in (4) and note that in view of (8), \( \int_{{i \in {\Omega_H}}} {{p_H}{{(i)}^{{1 - \sigma }}}di} = {\int_{{{{\overline{\theta }}_H}}}^{\infty } {\left[ {\frac{{\sigma w}}{{(\sigma - 1)\theta }}} \right]}^{{1 - \sigma }}}\frac{{{n_H}g(\theta )}}{{1 - G({{\overline{\theta }}_H})}}d\theta . \)

  14. This is a standard simplification that has been used by Melitz (2003) among others. See Baldwin (2005) for further discussion of this simplifying assumption.

  15. To derive (19), note first that C = wL N  + pZ from (5) and (6). Next, use (3), (4), (12), (13) and their foreign counterparts, and (18) to obtain: \( C = w\left( {{L_N} + {L_H} + {L_{{HE}}} + {L_{{FI}}}} \right) + {n_H}{\widetilde{\pi }_H} + {n_{{HE}}}{\widetilde{\pi }_{{HE}}} + {n_{{HI}}}{\widetilde{\pi }_{{HI}}} \). Finally to show that the right hand side of this equation equals wL, note that \( wL - w\left( {{L_C} + {L_H} + {L_{{HE}}} + {L_{{FI}}}} \right) = w{n_H}\psi \delta /{\nu_H} = {n_H}\widetilde{\pi } \) from (15) and (17).

  16. Without FDI, Average productivity of exporters is defined as \( {\widetilde{\theta }_{{HE}}} \equiv {\left[ {\int_{{{{\overline{\theta }}_{{HI}}}}}^{\infty } {\frac{{{\theta^{{\sigma - 1}}}g\left( \theta \right)}}{{1 - G\left( {{{\overline{\theta }}_{{HE}}}} \right)}}d\theta } } \right]^{{1/\left( {\sigma - 1} \right)}}} \).

  17. In deriving (23), note that \( {\tau^{{1 - \sigma }}}{\left( {{{\widetilde{\theta }}_{{HE}}}/{{\widetilde{\theta }}_H}} \right)^{{\sigma - 1}}} = {\phi_E}/\phi \) according to (10) and (11), and ν HE (ϕ E /ϕ) = τ −k(ϕ E /ϕ)1−k/(σ−1) according to (10) and (20).

  18. In this derivation, note that \( {\nu_{{HE}}}{\left( {{{\widetilde{\theta }}_{{HE}}}/{{\widetilde{\theta }}_H}} \right)^{{\sigma - 1}}} = {\left( {{{\overline{\theta }}_H}/{{\overline{\theta }}_{{HE}}}} \right)^{{k - \sigma + 1}}} - {\left( {{{\overline{\theta }}_H}/{{\overline{\theta }}_{{HI}}}} \right)^{{k - \sigma + 1}}} \) from (11) and (14). Using this condition, and (10) and (14), it can be shown that the expressions in both the square brackets equal \( 1 + {\left( {{{\overline{\theta }}_H}/{{\overline{\theta }}_{{HE}}}} \right)^k}\frac{{{\phi_E}}}{\phi } + {\left( {{{\overline{\theta }}_H}/{{\overline{\theta }}_{{HI}}}} \right)^k}\frac{{\left( {{\phi_I} - {\phi_E}} \right)}}{\phi } = 1 + {\left( {{\tau^{{1 - \sigma }}}\phi /\phi } \right)^{{k/\left( {\sigma - 1} \right)}}}\frac{{{\phi_E}}}{\phi } + {\left[ {\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)/\left( {{\phi_I} - {\phi_E}} \right)} \right]^{{k/\left( {\sigma - 1} \right)}}} \frac{{\left( {{\phi_I} - {\phi_E}} \right)}}{\phi } \).

  19. The countries in the sample are France, Germany, Italy, Japan, Portugal, Sweden, United Kingdom and the United States (the selection criterion is discussed in Appendix 3)

  20. Estimation of macro models using aggregate data yields low values of this elasticity, which are often close to one (e.g., Bergin 2006) while studies based on micro trade data produces estimates of the average elasticity as high as 6 (e.g., Broda and Weinstein 2006).

  21. Anderson and van Wincoop (2004)) survey the measurement of trade costs. Their representative estimate of policy barriers (tariffs and nontariff barriers) is 8% for industrialized countries. The estimate of directly measured freight costs (based on US data) is 12%. Transportation costs would be higher if the cost of the time value of goods in transit is added. They also find that additional border costs are substantial (some of these costs may be included in fixed export costs in our model).

  22. Our range of values for the substitution elasticity is similar to values used in a number of calibrated models. For example, Obstfeld and Rogoff (2007) assume that the value of this elasticity equals 2 or 3. Our upper limit is close to the value of 3.8 used by Ghironi and Melitz (2005) based on estimates of Bernard et al. (2003).

  23. Trade flows for the service industries are not available from the STAN data base, but input–output data for EU indicates that a substantial portion of trade, transportation, communication, financial and business services is delivered to export demand.

References

  • Anderson JE, van Wincoop E (2004) “Trade costs,” NBER Working Paper No. 10480

  • Arkolakis C, Costinot A, Rodriguez-Clare A (2009) “New trade models, same old gains?” NBER Working Paper No. 15628

  • Baldwin R (2005) “Heterogeneous firms and trade: testable and untestable properties of the Melitz Model,” NBER Working Paper No.11471

  • Baldwin R, Forslid R (2006) “Trade liberalization with heterogeneous firms,” NBER Working Paper No. 12192

  • Bergin PR (2006) How well can the new open economy macroeconomics explain the exchange rate and current account? Mimeo, University of California at Davis

  • Bernard AB, Eaton J, Bradford Jensen J (2003) Plants and productivity in international trade. Am Econ Rev 93:1268–1290

    Article  Google Scholar 

  • Borensztein E, De Gregorio J, Lee J-W (1998) How does foreign direct investment affect economic growth? J Int Econ 45(1):115–135

    Article  Google Scholar 

  • Broda C, Weinstein DE (2006) Globalization and the gains from variety. Q J Econ 121(2):541–585

    Article  Google Scholar 

  • Chor D (2009) Subsidies for FDI: implications from a model with heterogeneous firms. J Int Econ 78(1):113–125

    Article  Google Scholar 

  • De Gregorio J, Giovannini A, Wolf H (1994) International evidence on tradables and nontradables inflation. Eur Econ Rev 38(6):1225–1244

    Article  Google Scholar 

  • Dixit A, Norman V (1980) Theory of international trade. Cambridge University Press

  • Eaton J, Kortum S (2002) Technology, geography and trade. Econometrica 70(5):1741–1779

    Article  Google Scholar 

  • Feenstra RC (2009) Measuring the gains from trade under monopolistic competition. Mimeo, University of California, Davis

  • Feenstra R, Kee HL (2008) Export variety and country productivity: estimating the monopolistic competition model with endogenous productivity. J Int Econ 74(2):500–518

    Article  Google Scholar 

  • Ghironi F, Melitz MJ (2005) International trade and macroeconomic dynamics with heterogeneous firms. Q J Econ 120:865–915

    Google Scholar 

  • Helpman E (1981) International trade in the presence of product differentiation, economies of scale and monopolistic competition: a Chamberlin-Heckscher-Ohlin approach. J Int Econ 11:305–340

    Article  Google Scholar 

  • Helpman E, Krugman P (1985) Market structure and foreign trade. MIT, Cambridge

    Google Scholar 

  • Helpman E, Melitz MJ, Yeaple SR (2004) Export versus FDI with heterogeneous firms. Am Econ Rev 94(1):300–316

    Article  Google Scholar 

  • Krugman P (1980) Scale economies, product differentiation, and the pattern of trade. Am Econ Rev 70:950–959

    Google Scholar 

  • Lancaster K (1980) Inter-industry trade under perfect monopolistic competition. J Int Econ 10:151–175

    Article  Google Scholar 

  • Melitz MJ (2003) The impact of trade on aggregate industry productivity and intra-industry reallocations. Econometrica 71(6):1695–1725

    Article  Google Scholar 

  • van Marrewijk C, Stibora J, de Vaal A, Viaene J-M (1997) Producer services, comparative advantage, and international trade patterns. J Int Econ 42:195–220

    Article  Google Scholar 

  • Obstfeld M, Rogoff KS (2007) The unsustainable U.S. current account position. In: Clarida RH (ed) G7 current account imbalances: sustainability and adjustment. University of Chicago Press

  • Ramondo N, Rodríguez-Clare A (2009) “Trade, multinational production, and the gains from openness,” NBER Working Paper No. 15604

  • Rivera-Batiz LA, Romer PM (1991a) Economic integration and endogenous growth. Q J Econ 106:531–555

    Article  Google Scholar 

  • Rivera-Batiz LA, Romer PM (1991b) International trade with endogenous technological change. Eur Econ Rev 35:715–721

    Article  Google Scholar 

  • Trefler D (2004) The long and short of the Canada-U.S. free trade agreement. Am Econ Rev 94(4):870–895

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Economics, Carleton University, 1125 Colonel By Drive, Ottawa, ON, K1S 5B6, Canada

    Ehsan U. Choudhri

  2. Department of Economics, Lahore University of Management Sciences, Lahore, Pakistan

    Antonio Marasco

Authors
  1. Ehsan U. Choudhri
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Antonio Marasco
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ehsan U. Choudhri.

Additional information

We are grateful to an anonymous referee for helpful comments and suggestions.

Appendices

Appendix 1. Proof of Proposition 2

First, differentiate Θ 1 and Θ 2 to obtain

$$ \matrix{{*{20}{c}} {\frac{{\partial {\Theta_1}}}{{\partial \tau }} = - k{\tau^{{ - k - 1}}}{{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}} < 0,\frac{{\partial {\Theta_1}}}{{\partial \left( {{\phi_E}/\phi } \right)}} = - {\tau^{{ - k}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{ - k/\left( {\sigma - 1} \right)}}} < 0,} \hfill \\ {\frac{{\partial {\Theta_2}}}{{\partial \tau }} = k{{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{ - 1 + k/\left( {\sigma - 1} \right)}}}{\tau^{{ - \sigma }}}{{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}} > 0,} \hfill \\ {\frac{{\partial {\Theta_2}}}{{\partial \left( {{\phi_E}/\phi } \right)}} = {{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{k/\left( {\sigma - 1} \right)}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{ - k/\left( {\sigma - 1} \right)}}} > 0,} \hfill \\ {\frac{{\partial {\Theta_2}}}{{\partial \left( {{\phi_I}/\phi } \right)}} = - {{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{k/\left( {\sigma - 1} \right)}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{ - k/\left( {\sigma - 1} \right)}}} < 0.} \hfill \\ } $$
(A1)

Also, noting that \( {\tau^{{\sigma - 1}}}{\phi_E} < {\phi_I} \) implies that \( {\left( {\frac{{{\tau^{{\sigma - 1}}} - 1}}{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}} \right)^J} < 1 \), for J > 0, determine the signs of the following derivatives as

$$ \matrix{{*{20}{c}} {\frac{{\partial \left( {{\Theta_1} + {\Theta_2}} \right)}}{{\partial \tau }} = - k{\tau^{{ - k - 1}}}{{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}} + k{{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{ - 1 + k/\left( {\sigma - 1} \right)}}}{\tau^{{ - \sigma }}}{{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}}} \hfill \\ {\quad \quad = k{\tau^{{ - k - 1}}}{{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}}\left[ { - 1 + {{\left( {\frac{{{\tau^{{\sigma - 1}}} - 1}}{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}} \right)}^{{\frac{k}{\sigma } - 1}}}} \right] < 0,} \hfill \\ } $$
(A2)
$$ \matrix{{*{20}{c}} {\frac{{\partial \left( {{\Theta_1} + {\Theta_2}} \right)}}{{\partial \left( {{\phi_E}/\phi } \right)}} = - {\tau^{{ - k}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{\frac{{ - k}}{{\sigma - 1}}}}} + {{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{k/\left( {\sigma - 1} \right)}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{\frac{{ - k}}{{\sigma - 1}}}}}} \hfill \\ {\quad \quad = {\tau^{{ - k}}}\left( {\frac{{k - \sigma + 1}}{{\sigma - 1}}} \right){{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{\frac{{ - k}}{{\sigma - 1}}}}}\left[ { - 1 + {{\left( {\frac{{{\tau^{{\sigma - 1}}} - 1}}{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}} \right)}^{{\frac{k}{{\sigma - 1}}}}}} \right] < 0.} \hfill \\ } $$
(A3)

Noting from (23) and (24) that Θ 1 and Θ 2 do not depend on α, the proof of part (a) of Proposition 2 follows immediately from (25). To prove parts (b) and (c) of the proposition, use (A1)–(A3) [and note that ∂ Θ 1/ ∂ (ϕ I /ϕ) = 0] to show that

$$ \begin{array}{*{20}{c}} {\frac{{\partial \ln \left[ {\left( {w(T)/w(A)} \right.} \right]}}{{\partial \xi }} = \frac{\alpha }{{k\left( {1 + {{\Theta }_{1}}} \right)}}\frac{{\partial {{\Theta }_{1}}}}{{\partial \xi }} < 0\,{\text{for}}\,\xi = \tau ,{{\phi }_{E}}/\phi ;} \\ {\frac{{\partial \ln \left[ {\left( {w(O)/w(T)} \right.} \right]}}{{\partial \xi }} = \frac{\alpha }{{k\left( {1 + {{\Theta }_{1}} + {{\Theta }_{2}}} \right)}}\left[ {\frac{{\partial {{\Theta }_{2}}}}{{\partial \xi }} - \frac{{{{\Theta }_{2}}}}{{1 + {{\Theta }_{1}}}}\left( {\frac{{\partial {{\Theta }_{1}}}}{{\partial \xi }}} \right)} \right]\left\{ {\begin{array}{*{20}{c}} { > 0\,{\text{for}}\,\xi = \tau ,{{\phi }_{E}}/\phi } \\ { < 0\,{\text{for}}\,\xi = {{\phi }_{I}}/\phi } \\ \end{array} } \right.;\,{\text{and}}} \\ {\frac{{\partial \ln \left[ {\left( {w(O)/w(A)} \right.} \right]}}{{\partial \xi }} = \frac{\alpha }{{k\left( {1 + {{\Theta }_{1}} + {{\Theta }_{2}}} \right)}}\left( {\frac{{\partial {{\Theta }_{1}}}}{{\partial \xi }} + \frac{{\partial {{\Theta }_{2}}}}{{\partial \xi }}} \right) < 0\,{\text{for}}\,\xi = \tau ,{{\phi }_{E}}/\phi ,{{\phi }_{I}}/\phi .} \\ \end{array} $$

Next, differentiate Θ 1 and Θ 2 with respect to k, simplify, and make use of the conditions that \( \frac{{{\tau^{{\sigma - 1}}}{\phi_E}}}{\phi } > 1 \) and \( \left( {\frac{{{\phi_I} - {\phi_E}}}{{\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)}}} \right) > 1 \), to determine the signs of these derivatives as follows:

$$ \matrix{{*{20}{c}} {\frac{{\partial {\Theta_1}}}{{\partial k}} = \left( {\frac{{ - 1}}{{\sigma - 1}}} \right){\tau^{{ - k}}}{{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}}\ln \left( {\frac{{{\tau^{{\sigma - 1}}}{\phi_E}}}{\phi }} \right) < 0,} \hfill \\ {\frac{{\partial {\Theta_2}}}{{\partial k}} = \left( {\frac{{ - 1}}{{\sigma - 1}}} \right){{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{k/\left( {\sigma - 1} \right)}}}{{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}}\ln \left( {\frac{{{\phi_I} - {\phi_E}}}{{\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)}}} \right) < 0.} \hfill \\ } $$
(A4)

Also, since \( \frac{{{\phi_I} - {\phi_E}}}{{\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)}} > \frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi } > {\left( {\frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi }} \right)^{{\frac{{{\Theta_1}}}{{1 + {\Theta_1}}}}}} \), it follows that

$$ \matrix{{*{20}{c}} {\frac{{\partial {\Theta_2}}}{{\partial k}} - \frac{{{\Theta_2}}}{{1 + {\Theta_1}}}\left( {\frac{{\partial {\Theta_1}}}{{\partial k}}} \right) = \left( {\frac{{ - 1}}{{\sigma - 1}}} \right){{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{\frac{k}{{\sigma - 1}}}}}{{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{1 - \frac{k}{{\sigma - 1}}}}}} \hfill \\ { \times \left[ {\ln \left( {\frac{{{\phi_I} - {\phi_E}}}{{\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)}}} \right) - \frac{{{\Theta_1}}}{{1 + {\Theta_1}}}\ln \left( {\frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi }} \right)} \right] < 0.} \hfill \\ } $$
(A5)

Make use of (A4) and (A5) to obtain

$$ \matrix{{*{20}{c}} {\frac{{\partial \ln \left[ {\left( {w(T)/w(A)} \right.} \right]}}{{\partial k}} = \frac{\alpha }{{k\left( {1 + {\Theta_1}} \right)}}\frac{{\partial {\Theta_1}}}{{\partial k}} - \ln \left( {1 + {\Theta_1}} \right)\frac{\alpha }{{{k^2}}} < 0,} \hfill \\ {\frac{{\partial \ln \left[ {\left( {w(O)/w(A)} \right.} \right]}}{{\partial k}} = \frac{\alpha }{{k\left( {1 + {\Theta_1} + {\Theta_2}} \right)}}\left( {\frac{{\partial {\Theta_1}}}{{\partial k}} + \frac{{\partial {\Theta_2}}}{{\partial k}}} \right) - \ln \left( {1 + {\Theta_1} + {\Theta_2}} \right)\frac{\alpha }{{{k^2}}} < 0,} \hfill \\ {\frac{{\partial \ln \left[ {\left( {w(O)/w(T)} \right.} \right]}}{{\partial k}} = \frac{\alpha }{{k\left( {1 + {\Theta_1} + {\Theta_2}} \right)}}\left[ {\frac{{\partial {\Theta_2}}}{{\partial k}} - \frac{{{\Theta_2}}}{{1 + {\Theta_1}}}\left( {\frac{{\partial {\Theta_1}}}{{\partial k}}} \right)} \right] - \ln \left( {\frac{{1 + {\Theta_1} + {\Theta_2}}}{{1 + {\Theta_1}}}} \right)\frac{\alpha }{{{k^2}}} < 0,} \hfill \\ } $$

which proves part (d) of the proposition.

Finally, to prove part (e) of the proposition, differentiate Θ 1 and Θ 2 with respect to σ, and simplify to get

$$ \frac{{\partial {\Theta_1}}}{{\partial \sigma }} = {\tau^{{ - k}}}{\left( {\frac{{{\phi_E}}}{\phi }} \right)^{{1 - k/\left( {\sigma - 1} \right)}}}\ln \left( {\frac{{{\phi_E}}}{\phi }} \right)\left( {\frac{k}{{{{\left( {\sigma - 1} \right)}^2}}}} \right)\;\matrix{{*{20}{c}} > \hfill \\ < \hfill \\ } \,0\,{\text{as}}\,{\phi_{\text{E}}}\,\matrix{{*{20}{c}} > \hfill \\ < \hfill \\ } \,\phi, $$
(A6)
$$ \frac{{\partial {\Theta_2}}}{{\partial \sigma }} = {\left( {1 - {\tau^{{1 - \sigma }}}} \right)^{{k/\left( {\sigma - 1} \right)}}}{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)^{{1 - k/\left( {\sigma - 1} \right)}}}\left( {\frac{k}{{{{\left( {\sigma - 1} \right)}^2}}}} \right)\left[ {\ln \left( {\frac{{{\phi_I} - {\phi_E}}}{{\phi \left( {1 - {\tau^{{1 - \sigma }}}} \right)}}} \right) + \frac{{{\tau^{{1 - \sigma }}}\ln \left( {{\tau^{{\sigma - 1}}}} \right)}}{{1 - {\tau^{{1 - \sigma }}}}}} \right]. $$
(A7)

Now use (A6) and (A7) to obtain

$$ \matrix{{*{20}{c}} {\frac{{\partial {\Theta_1}}}{{\partial \sigma }} + \frac{{\partial {\Theta_2}}}{{\partial \sigma }} = {\tau^{{ - k}}}{{\left( {\frac{{{\phi_E}}}{\phi }} \right)}^{{1 - k/\left( {\sigma - 1} \right)}}}\left( {\frac{k}{{{{\left( {\sigma - 1} \right)}^2}}}} \right)\left[ {\ln \left( {\frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi }} \right)} \right. + \left( {\frac{{{\tau^{{\sigma - 1}}} - 1}}{{\left( {{\phi_I}/{\phi_E}} \right) - 1}} - 1} \right)\ln \left( {{\tau^{{\sigma - 1}}}} \right)} \hfill \\ {\left. {\quad \quad + \left( {{\tau^{{\sigma - 1}}} - 1} \right){{\left( {\frac{{{\tau^{{\sigma - 1}}} - 1}}{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}} \right)}^{{\frac{k}{{\left( {\sigma - 1} \right)}} - 1}}}\left\{ {\ln \left( {\frac{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}{{{\tau^{{\sigma - 1}}} - 1}}} \right) + \ln \left( {\frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi }} \right)} \right\}} \right],} \hfill \\ } $$
(A8)
$$ \matrix{{*{20}{c}} {\frac{{\partial {\Theta_2}}}{{\partial \sigma }} - \frac{{{\Theta_2}}}{{1 + {\Theta_1}}}\left( {\frac{{\partial {\Theta_1}}}{{\partial \sigma }}} \right) = {{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{\frac{k}{{\sigma - 1}}}}}{{\left( {\frac{{{\phi_I} - {\phi_E}}}{\phi }} \right)}^{{1 - \frac{k}{{\sigma - 1}}}}}\left( {\frac{k}{{{{\left( {\sigma - 1} \right)}^2}}}} \right)} \hfill \\ {\quad \quad \times \left[ {\ln \left( {\frac{{\left( {{\phi_I}/{\phi_E}} \right) - 1}}{{{\tau^{{\sigma - 1}}} - 1}}} \right) + \frac{1}{{1 + {\Theta_1}}}\ln \left( {\frac{{{\phi_E}{\tau^{{\sigma - 1}}}}}{\phi }} \right) + \left( {\frac{1}{{{\tau^{{\sigma - 1}}} - 1}} + \frac{{{\Theta_1}}}{{1 + {\Theta_1}}}} \right)\ln \left( {{\tau^{{\sigma - 1}}}} \right)} \right] > 0.} \hfill \\ } $$
(A9)

From (A6), it follows that \( \frac{{\partial \ln \left[ {C(T)/C(A)} \right]}}{{\partial \sigma }} = \frac{\alpha }{{k\left( {1 + {\Theta_1}} \right)}}\frac{{\partial {\Theta_1}}}{{\partial \sigma }}\,\matrix{{*{20}{c}} > \hfill \\ < \hfill \\ } \,0\,{\text{as}}\,{\phi_{\text{E}}}\,\matrix{{*{20}{c}} > \hfill \\ < \hfill \\ } \,\phi \); \( \frac{{\partial \ln \left[ {w(O)/w(T)} \right]}}{{\partial \sigma }} = \frac{\alpha }{k}\left[ {\frac{1}{{1 + {\Theta_1} + {\Theta_2}}}\left( {\frac{{\partial {\Theta_1}}}{{\partial \sigma }} + \frac{{\partial {\Theta_2}}}{{\partial \sigma }}} \right) - \frac{1}{{1 + {\Theta_1}}}\left( {\frac{{\partial {\Theta_1}}}{{\partial \sigma }}} \right)} \right]\matrix{{*{20}{c}} > \hfill \\ < \hfill \\ } 0 \), as the expression in the square bracket in (A8) is positive or negative; and from (A9), \( \frac{{\partial \ln \left[ {w(O)/w(A)} \right]}}{{\partial \sigma }} = \frac{\alpha }{{k\left( {1 + {\Theta_1} + {\Theta_2}} \right)}}\left( {\frac{{\partial {\Theta_1}}}{{\partial \sigma }} + \frac{{\partial {\Theta_2}}}{{\partial \sigma }}} \right) > 0 \). Also note that ϕ E > ϕ implies that \( \frac{{\partial \ln \left[ {w(T)/w(A)} \right]}}{{\partial \sigma }} > 0 \), and \( \frac{{\partial \ln \left[ {w(O)/w(A)} \right]}}{{\partial \sigma }} > 0 \).

Appendix 2. Measures of Gains Conditional on Import and FDI Shares

Let S ET ( ≡ p FE Z FE /pZ) and S IT ( ≡ p FI Z FI /pZ) denote, respectively, the shares of imports and foreign subsidiaries in traded goods. Use (3), (6), (12), (16), and the symmetry assumption to express these shares as

$$ \matrix{{*{20}{c}} {{S_{{ET}}} = {{\left( {\frac{{{p_{{FE}}}}}{p}} \right)}^{{1 - \sigma }}} = \left[ {\frac{{{\nu_{{HE}}}{\tau^{{1 - \sigma }}}{{\left( {{{\widetilde{\theta }}_{{HE}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}}}}{{1 + {\nu_{{HE}}}{\tau^{{1 - \sigma }}}{{\left( {{{\widetilde{\theta }}_{{HE}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}} + {\nu_{{HI}}}{{\left( {{{\widetilde{\theta }}_{{HI}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}}}}} \right]} \hfill \\ {{S_{{IT}}} = {{\left( {\frac{{{p_{{FI}}}}}{p}} \right)}^{{1 - \sigma }}} = \left[ {\frac{{{\nu_{{HI}}}{{\left( {{{\widetilde{\theta }}_{{HI}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}}}}{{1 + {\nu_{{HE}}}{\tau^{{1 - \sigma }}}{{\left( {{{\widetilde{\theta }}_{{HE}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}} + {\nu_{{HI}}}{{\left( {{{\widetilde{\theta }}_{{HI}}}/{{\widetilde{\theta }}_H}} \right)}^{{\sigma - 1}}}}}} \right]} \hfill \\ } $$
(A10)

From (10), (11), and (14), we obtain \( {\nu_{{HE}}}\left( {\widetilde{\theta }_{{HE}}^{{\sigma - 1}}/\widetilde{\theta }_H^{{\sigma - 1}}} \right) = {\tau^{{ - k}}}{\left( {\frac{{{\phi_E}}}{\phi }} \right)^{{1 - \frac{k}{{\sigma - 1}}}}} - {\tau^{{1 - \sigma }}}{\left[ {\frac{{{\phi_I}/\phi - {\phi_E}/\phi }}{{1 - {\tau^{{1 - \sigma }}}}}} \right]^{{1 - \frac{k}{{\sigma - 1}}}}} \), and \( {\nu_{{HI}}}\left( {\widetilde{\theta }_{{HI}}^{{\sigma - 1}}/\widetilde{\theta }_H^{{\sigma - 1}}} \right) = 1 + {\tau^{{ - k}}}{\left( {\frac{{{\phi_E}}}{\phi }} \right)^{{1 - \frac{k}{{\sigma - 1}}}}} + {\left( {1 - {\tau^{{1 - \sigma }}}} \right)^{{\frac{k}{{\sigma - 1}}}}}{\left[ {\frac{{{\phi_I}}}{\phi } - \frac{{{\phi_E}}}{\phi }} \right]^{{1 - \frac{k}{{\sigma - 1}}}}} \). Using these expressions, we can restate (A10) as

$$ {S_{{ET}}} = \frac{{{\Theta_1} - {\tau^{{1 - \sigma }}}{{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{ - 1}}}{\Theta_2}}}{{1 + {\Theta_1} + {\Theta_2}}},\quad {S_{{IT}}} = \frac{{{{\left( {1 - {\tau^{{1 - \sigma }}}} \right)}^{{ - 1}}}{\Theta_2}}}{{1 + {\Theta_1} + {\Theta_2}}}, $$
(A11)

The relations in (A11) imply that

$$ {S_{{ET}}} + {S_{{IT}}} = \frac{{{\Theta_1} + {\Theta_2}}}{{1 + {\Theta_1} + {\Theta_2}}}, $$
(A12)

and

$$ {\Theta_2} = \left( {\frac{{{S_{{IT}}}}}{{1 - {S_{{ET}}} - {S_{{IT}}}}}} \right)\frac{{{\tau^{{\sigma - 1}}} - 1}}{{{\tau^{{\sigma - 1}}}}},\quad {\Theta_1} = \frac{{{S_{{ET}}} + {S_{{IT}}}{\tau^{{ - \left( {\sigma - 1} \right)}}}}}{{1 - {S_{{ET}}} - {S_{{IT}}}}}. $$
(A13)

In view of (A12), proportional gains from openness defined in (25) can be expressed as a function of S ET  + S IT , α and k, and these gains can be measured using estimates of shares, S ET , S IT and α, and the parameter k. According to (A13), proportional gains from trade and FDI defined in (25) also depend on τ σ−1, and thus an estimate of this variable would also be needed to separate the trade and FDI components of the openness gains.

Relation (A12) and (A13) also have to satisfy the constraint that \( 1 < {\tau^{{\sigma - 1}}}{\phi_E}/\phi < {\phi_I}/\phi \). As shown below, this constraint is met if S IT > 0 and the following condition is satisfied:

$$ 1 - {S_{{ET}}} - {S_{{IT}}} > {S_{{ET}}}{\tau^{{\left( {\sigma - 1} \right)}}} + {S_{{IT}}}. $$
(A14)

Given the estimates of S ET and S IT , condition (A14) constraints the value of τ σ−1.

Define \( {\chi_E} \equiv {\tau^{{\sigma - 1}}}\left( {{\phi_E}/\phi } \right),\;{\chi_I} \equiv \left( {{\phi_I}/{\phi_E}} \right)/{\tau^{{\sigma - 1}}} \), so that the constraint \( 1 < {\tau^{{\sigma - 1}}}{\phi_E}/\phi < {\phi_I}/\phi \) implies that χ E  > 1, χ I  > 1. Letting k = σ − 1 + ε, we have \( {\Theta_1} = {\tau^{{ - \left( {\sigma - 1} \right)}}}{\left( {{\chi_E}} \right)^{{\frac{{ - \varepsilon }}{{\sigma - 1}}}}} \). Now use (A13) to obtain

$$ {\chi_E} = {\left( {\frac{{1 - {S_{{ET}}} - {S_{{IT}}}}}{{{S_{{ET}}}{\tau^{{\left( {\sigma - 1} \right)}}} + {S_{{IT}}}}}} \right)^{{\frac{{\sigma - 1}}{\varepsilon }}}} $$
(A15)

If (A14) is satisfied, (A15) implies that χ E  > 1. Next, we can express \( {\Theta_2} = {\tau^{{ - \left( {\sigma - 1} \right)}}}{\left( {{\chi_E}} \right)^{{\frac{{ - \varepsilon }}{{\sigma - 1}}}}}{\left( {{\tau^{{\sigma - 1}}} - 1} \right)^{{1 + \frac{\varepsilon }{{\sigma - 1}}}}}{\left( {{\chi_I}{\tau^{{\sigma - 1}}} - 1} \right)^{{\frac{{ - \varepsilon }}{{\sigma - 1}}}}} \). Use (A13) to write this relation as \( \left( {\frac{{{S_{{IT}}}}}{{1 - {S_{{ET}}} - {S_{{IT}}}}}} \right) = {\left( {{\chi_E}} \right)^{{\frac{{ - \varepsilon }}{{\sigma - 1}}}}}{\left( {{\tau^{{\sigma - 1}}} - 1} \right)^{{\frac{\varepsilon }{{\sigma - 1}}}}}{\left( {{\chi_I}{\tau^{{\sigma - 1}}} - 1} \right)^{{\frac{{ - \varepsilon }}{{\sigma - 1}}}}} \). If S IT > 0, then this relation implies [using (A15) to substitute for χ E ] that \( \frac{{{\chi_I}{\tau^{{\sigma - 1}}} - 1}}{{{\tau^{{\sigma - 1}}} - 1}} = {\left( {\frac{{{S_{{ET}}}{\tau^{{\left( {\sigma - 1} \right)}}} + {S_{{IT}}}}}{{{S_{{IT}}}}}} \right)^{{\frac{{\sigma - 1}}{\varepsilon }}}} > 1 \) or χ I > 1.

Appendix 3. Calculation of Shares

3.1 Share of Services in GDP

The service shares in Table 1 represent average shares based on data from World Bank, World Development Indicators (2007). Developing countries include low income, lower-middle income, and upper-middle income (defined by the World Bank as the countries with 2010 GNI of less than $12276) while developed countries represent high-income countries (with 2010 GNI of greater than $12276). The country set includes all countries for which sufficient data were available (no more than 1 data points were missing for the period 1990–2005).

3.2 Share of Tradables in Consumption

For our country group (defined below), the share of tradables (α) is calculated from data in OECD, STAN database. The narrow concept of tradables is based on the conventional definition of the tradable sector as consisting of Agriculture (including Hunting, Forestry and Fishing), Mining (including Quarrying) and Manufacturing. The broader concept adds Electricity, Gas and Water Supply plus 25% of the following sectors: Wholesale and Retail Trade (including Restaurants and Hotels), Transport, Storage and Communications, and Finance, Insurance, Real Estate and Business Services. Parts of these sectors include service industries with significant trade.Footnote 23 For each definition, the share of tradables is calculated by dividing the sum of value added in the tradable industries by aggregate expenditure defined as GDP plus net exports.

3.3 Shares of Imports and FDI in Tradables

The import share (S IT ) is also calculated from data in STAN database. As import flows are measured in gross values, imports are divided by the gross value of the production of tradable sector to derive the share of imports in traded goods. Since import data are available for STAN database only for Agriculture, Mining and Manufacturing, the import share is calculated for the narrow concept of tradables. For the broader concept of the tradables, our calibration assumes that the share of imports in the additional sectors is the same as in the narrowly defined tradable sector.

To calculate the FDI share (S ET ), the data for sales of foreign affiliates of TNC’s in the host economy are obtained from UN, UNCTAD, Country Profiles (Table 44). As in the case of import share, the FDI share is calculated by dividing the FDI sales in Agriculture, Mining and Manufacturing by the gross production for these sectors. Again, the same share is assumed in the case of the broader definition of tradables.

3.4 The Sample

The sectoral data for FDI sales are available for a limited number of countries for a part or whole of the period from 1993 to 2001. We chose all countries, for which FDI sales data are available for at least three years in this period. Our country sample consists of eight countries and includes France, Germany, Italy, Japan, Portugal, Sweden, United Kingdom and the United States. For each country, the FDI share is calculated as the average share over the years in the 1993–2001 period for which data are available. For consistency, country shares of tradables and imports are also calculated over the same (1993–2001) period. The shares for the country group represent weighted averages of country shares.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Choudhri, E.U., Marasco, A. Heterogeneous Productivity and the Gains from Trade and FDI. Open Econ Rev 24, 339–360 (2013). https://doi.org/10.1007/s11079-012-9243-7

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11079-012-9243-7

Keywords

JEL codes

Search

Navigation