Skip to main content
SpringerLink
Log in

Partitioned and Implicit–Explicit General Linear Methods for Ordinary Differential Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

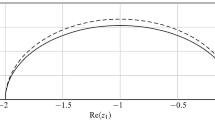
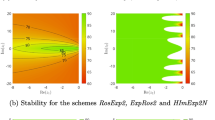
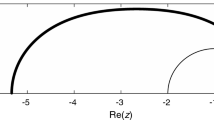
Abstract

Implicit–explicit (IMEX) time stepping methods can efficiently solve differential equations with both stiff and nonstiff components. IMEX Runge–Kutta methods and IMEX linear multistep methods have been studied in the literature. In this paper we study new implicit–explicit methods of general linear type. We develop an order conditions theory for high stage order partitioned general linear methods (GLMs) that share the same abscissae, and show that no additional coupling order conditions are needed. Consequently, GLMs offer an excellent framework for the construction of multi-method integration algorithms. Next, we propose a family of IMEX schemes based on diagonally-implicit multi-stage integration methods and construct practical schemes of order up to three. Numerical results confirm the theoretical findings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Notes

  1. http://www.mathworks.com/matlabcentral/fileexchange/18593-differential-evolution

References

  1. Ascher, U.M., Ruuth, S.J., Spiteri, R.J.: Implicit–explicit Runge–Kutta methods for time-dependent partial differential equations. Appl. Numer. Math 25, 151–167 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  2. Ascher, U.M., Ruuth, S.J., Wetton, B.T.R.: Implicit–explicit methods for time-dependent partial differential equations. SIAM. J. Numer. Anal. 32(3), 797–823 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  3. Blaise, S., St-Cyr, A.: A dynamic hp-adaptive discontinuous Galerkin method for shallow-water flows on the sphere with application to a global tsunami simulation. Mon. Weather Rev. 140, 978–996 (2012)

    Article  Google Scholar 

  4. Boscarino, S., Russo, G.: On a class of uniformly accurate IMEX Runge–Kutta schemes and applications to hyperbolic systems with relaxation. SIAM J. Sci. Comput. 31(3), 1926–1945 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  5. Butcher, J.: Diagonally-implicit multi-stage integration methods. Appl. Numer. Math. 11(5), 347–363 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  6. Butcher, J.: Numerical Methods for Ordinary Differential Equations. Wiley, New York (2008)

    Book  MATH  Google Scholar 

  7. Butcher, J., Jackiewicz, Z.: Construction of diagonally implicit general linear methods of type 1 and 2 for ordinary differential equations. Appl. Numer. Math. 21(4), 385–415 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  8. Butcher, J., Wright, W.: The construction of practical general linear methods. BIT Numer. Math. 43, 695–721 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  9. Butcher, J.C., Jackiewicz, Z.: Diagonally implicit general linear methods for ordinary differential equations. BIT Numer. Math. 33, 452–472 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  10. Butcher, J.C., Jackiewicz, Z.: Implementation of diagonally implicit multistage integration methods for ordinary differential equations. SIAM J. Numer. Anal. 34(6), 2119–2141 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  11. Cardone, A., Jackiewicz, Z., Sandu, A., Zhang, H.: Extrapolation-based implicit-explicit general linear methods. Numerical Algorithms, pp. 1–23 (2013)

  12. Comblen, R., Blaise, S., Legat, V., Remacle, J.F., Deleersnijder, E., Lambrechts, J.: A discontinuous finite element baroclinic marine model on unstructured prismatic meshes. Ocean Dyn. 60(6), 1395–1414 (2010)

    Article  Google Scholar 

  13. D’Ambrosio, R., Butcher, J.: Multivalue numerical methods for partitioned differential problems: from second order ODEs to separable Hamiltonians. Presentation given at Auckland Numerical Ordinary Differential Equations ANODE 2013 (celebration of the 80th birthday of John Butcher) (2013)

  14. Frank, J., Hundsdorfer, W., Verwer, J.: On the stability of implicit–explicit linear multistep methods. Appl. Numer. Math. 25(2–3), 193–205 (1997). (Special Issue on Time Integration)

    Article  MATH  MathSciNet  Google Scholar 

  15. Geuzaine, C., Remacle, J.F.: Gmsh: A 3-d finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Meth. Eng. 79(11), 1309–1331 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  16. Giraldo, F., Restelli, M., Läuter, M.: Semi-implicit formulations of the Navier–Stokes equations: application to nonhydrostatic atmospheric modeling. SIAM J. Sci. Comput. 32(6), 3394–3425 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  17. Giraldo, F.X., Restelli, M.: A study of spectral element and discontinuous Galerkin methods for the Navier–Stokes equations in nonhydrostatic mesoscale atmospheric modeling: equation sets and test cases. J. Comput. Phys. 227(8), 3849–3877 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  18. Hairer, E., Norsett, S., Wanner, G.: Solving Ordinary Differential Equations I. Nonstiff Problems. Springer, Berlin (1993)

    MATH  Google Scholar 

  19. Hundsdorfer, W., Ruuth, S.J.: IMEX extensions of linear multistep methods with general monotonicity and boundedness properties. J. Comput. Phys. 225(2), 2016–2042 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  20. Jackiewicz, Z.: General Linear Methods for ODE. Wiley, New York (2009)

    Google Scholar 

  21. Kameni, A., Lambrechts, J., Remacle, J., Mezani, S., Bouillault, F., Geuzaine, C.: Discontinuous Galerkin Method for computing induced fields in superconducting materials. IEEE Trans. Magn. 48(2), 591–594 (2012)

    Article  Google Scholar 

  22. Karna, T., Legat, V., Deleersnijder, E.: A baroclinic discontinuous Galerkin finite element model for coastal flows. Ocean Model. 61, 1–20 (2013)

    Article  Google Scholar 

  23. Kennedy, C.A., Carpenter, M.H.: Additive Runge–Kutta schemes for convection–diffusion–reaction equations. Appl. Numer. Math. 44(1–2), 139–181 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  24. Nair, R., Thomas, S., Loft, R.: A discontinuous Galerkin global shallow water model. Mon. Weather Rev. 133(4), 876–888 (2003)

    Article  Google Scholar 

  25. Pareschi, L., Russo, G.: Implicit–explicit Runge–Kutta schemes and applications to hyperbolic systems with relaxation. J. Sci. Comput. 3, 269–287 (2000)

    MathSciNet  Google Scholar 

  26. Prothero, A., Robinson, A.: On the stability and accuracy of one-step methods for solving stiff systems of ordinary differential equations. Math. Comput. 28(125), 145–162 (1974)

    Article  MathSciNet  Google Scholar 

  27. Seny, B., Lambrechts, J., Comblen, R., Legat, V., Remacle, J.F.: Multirate time stepping for accelerating explicit discontinuous Galerkin computations with application to geophysical flows. Int. J. Numer. Meth. Fluids 71(1), 41–64 (2013)

    Article  MathSciNet  Google Scholar 

  28. Seny, B., Lambrechts, J., Toulorge, T., Legat, V., Remacle, J.F.: An efficient parallel implementation of explicit multirate Runge–Kutta schemes for discontinuous Galerkin computations. J. Comput. Phys. 256, 135–160 (2014)

    Article  MathSciNet  Google Scholar 

  29. Skamarock, W.C., Klemp, J.B.: Efficiency and accuracy of the Klemp–Wilhelmson time-splitting technique. Mon. Weather Rev. 122(11), 2623–2630 (1994)

    Article  Google Scholar 

  30. St-Cyr, A., Neckels, D.: A fully implicit jacobian-free high-order discontinuous Galerkin mesoscale flow solver. In: Allen, G., Nabrzyski, J., Seidel, E., Albada, G., Dongarra, J., Sloot, P. (eds.) Computational Science ICCS 2009. Lecture Notes in Computer Science, vol. 5545, pp. 243–252. Springer, Berlin (2009)

    Chapter  Google Scholar 

  31. Verwer, J., Sommeijer, B., Hundsdorfer, W.: RKC time-stepping for advection–diffusion–reaction problems. J. Comput. Phys. 201(1), 61–79 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  32. Wright, W.M.: General linear methods with inherent Runge–Kutta stability. Ph.D. thesis, The University of Auckland (2002)

  33. Zhang, H., Sandu, A.: Implicit–explicit DIMSIM time stepping algorithms. arXiv:1302.2689

  34. Zhang, H., Sandu, A.: A second-order diagonally-implicit–explicit multi-stage integration method. Procedia CS 9, 1039–1046 (2012)

    Google Scholar 

Download references

Acknowledgments

This work has been supported in part by NSF through awards NSF OCI-8670904397, NSF CCF-0916493, NSF DMS-0915047, NSF CMMI-1130667, NSF CCF-1218454 AFOSR FA9550-12-1-0293-DEF, FOSR 12-2640-06, DoD G&C 23035, and by the Computational Science Laboratory at Virginia Tech. Sébastien Blaise is a Postdoctoral Researcher with the Belgian Fund for Research (FNRS).

Author information

Authors and Affiliations

  1. Department of Computer Science, Virginia Polytechnic Institute and State University, Blacksburg, VA , 24061, USA

    Hong Zhang & Adrian Sandu

  2. Institute of Mechanics, Materials and Civil Engineering, Université catholique de Louvain, Louvain-la-Neuve, Belgium

    Sebastien Blaise

Authors
  1. Hong Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Adrian Sandu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sebastien Blaise
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Adrian Sandu.

Additional information

This paper is dedicated to Prof. J.C. Butcher’s 80-th birthday.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zhang, H., Sandu, A. & Blaise, S. Partitioned and Implicit–Explicit General Linear Methods for Ordinary Differential Equations. J Sci Comput 61, 119–144 (2014). https://doi.org/10.1007/s10915-014-9819-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-014-9819-z

Keywords

Search

Navigation