Capillary pumps with constant flow rate

Abstract

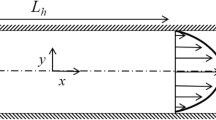
This paper unambiguously derives, ab initio starting from the Navier–Stokes and Laplace equations, the geometric parameters defining capillary pumps (CPs) with rectangular cross section with constant volumetric flow rate and steady velocity profile. The parametric formulation of the channel shape is derived using Taylor series approximations of the capillary pressure and the hydrodynamic flow resistance with negligible error. First, the design parameters are derived for a CP consisting of a single channel and illustrated with an example. Thereafter, the design parameters for multichannel and for micropillar array CPs are derived. Finally, the design of CPs for multistep constant flow rates derived and those for arbitrarily varying flow rates are discussed.

Appendix

This appendix derives an expression for the capillary pressure in a channel with rectangular cross section. We will first derive such expression for a zero-th order Taylor series approximation for w(s) around s = x, and thereafter for first order Taylor series approximation. The latter will allow estimating the maximum error induced by the zero-th order approximation, and hence the interval of s where such approximation is valid. The below is an expansion of the work by Man et al. (1998). We start from

$$P\left( s \right) = \gamma \cdot \left[ {\cos \left( \theta \right)\frac{{{\text{d}}A_{\text{sl}} }}{{{\text{d}}V_{\text{l}} }} - \frac{{{\text{d}}A_{ \lg } }}{{{\text{d}}V_{\text{l}} }}} \right],$$

(46)

where U _S, V _l, A _sl, A _la and γ denote the total surface energy of the channel geometry, the liquid volume in the channel, the total wetted area of the channel, the total liquid–air interfacial surface area and the liquid–gas surface energy, respectively.

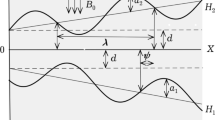
For a zero-th order approximation w(s) = w(x), the capillary pressure equals that of a liquid in a rectangular channel with constant cross section, see Fig. 7.

Fig. 7

Perspective illustration of the liquid in a capillary channel with constant rectangular cross section

The shape and area A _lg of the liquid–gas interface remains constant as the liquid front advances, and hence

$$\frac{{{\text{d}}A_{\lg } }}{{{\text{d}}V_{\text{l}} }} = 0.$$

(47)

$$\frac{{{\text{d}}A_{\text{sl}} }}{{{\text{d}}V_{\text{l}} }} = \frac{{\frac{{{\text{d}}A_{\text{sl}} }}{{{\text{d}}s}}}}{{\frac{{{\text{d}}V_{\text{l}} }}{s}}} = \frac{{\frac{{\left( {2 \cdot w + 2 \cdot h} \right) \cdot {\text{d}}s}}{{{\text{d}}s}}}}{{\frac{{w \cdot h \cdot {\text{d}}s}}{{{\text{d}}s}}}}.$$

(48)

Inserting Eqs. 47 and 48 in 46 results in

$$P_{0} \left( s \right) = 2 \cdot \gamma \cdot \cos \left( \theta \right) \cdot \left[ {\frac{1}{h} + \frac{1}{w}} \right].$$

(49)

For a first order approximation w(s) around x, the capillary pressure equals that of a liquid in a tapered channel with a rectangular cross section of which the width w decreases linearly with increasing s, as shown in top view in Fig. 8.

Fig. 8

Top view illustration of the capillary filling of a tapered channel, here illustrated as narrow slit channel with cylindrical liquid–gas surface

As in the above case, the liquid–gas interface constitutes a complicated 3D surface that cannot be generally described parametrically. If $w \ll h$, the shape of the interface surface is close to that of a parallel plate slit, i.e. cylindrical. We will here solve the capillary pressure drop for such cylindrical surface shape, as depicted in Fig. 8, and assume that the results can be extended to general liquid surface geometries for w ≤ h.

Defining

$$\beta = \frac{1}{2}{\text{atan}}\left( {\frac{{{\text{d}}w(x)}}{{{\text{d}}s}}} \right),$$

(50)

$$\alpha = \pi /2 - \theta - \beta ,$$

(51)

and

$$R = \frac{w}{2 \cdot \sin \alpha },$$

(52)

we can calculate

$${\text{d}}V = - h \cdot \left[ {\frac{1}{tg\beta } - \frac{1}{tg\alpha } + \frac{\alpha }{{\sin^{2} \alpha }}} \right] \cdot \frac{w}{2}.\frac{{{\text{d}}w}}{{{\text{d}}s}} \cdot {\text{d}}s,$$

(53)

$${\text{d}}A_{\lg } = 2\alpha h{\text{d}}R = \frac{\alpha h}{\sin \alpha } \cdot \frac{{{\text{d}}w}}{{{\text{d}}s}} \cdot {\text{d}}s,\,{\text{and}}$$

(54)

$${\text{d}}A_{\text{sl}} = - \left[ {\frac{2 \cdot h}{\cos \beta } + 2 \cdot \left[ {\frac{1}{tg\beta } - \frac{1}{tg\alpha } + \frac{\alpha }{{\sin^{2} \alpha }}} \right] \cdot \frac{w}{2} \cdot \frac{dw}{ds} \cdot } \right] \cdot {\text{d}}s.$$

(55)

Substituting 50, 52, 53, and 54 in 46 results in

$$P_{1} \left( s \right) = \gamma \cdot \left[ {\cos \theta \cdot \frac{2}{h} + \frac{{\frac{2 \cdot h}{\cos \beta } \cdot \cos \theta }}{{h \cdot \left[ {\frac{1}{tg\beta } - \frac{1}{tg\alpha } + \frac{\alpha }{{2 \cdot \sin^{2} \alpha }}} \right] \cdot w \cdot \frac{{{\text{d}}w}}{{{\text{d}}s}}}} + \frac{{\frac{\alpha h}{\sin \alpha } \cdot \frac{{{\text{d}}w}}{{{\text{d}}s}}}}{{h \cdot \left[ {\frac{1}{tg\beta } - \frac{1}{tg\alpha } + \frac{\alpha }{{2 \cdot \sin^{2} \alpha }}} \right] \cdot \frac{{{\text{d}}w}}{{{\text{d}}s}} \cdot w}}} \right],$$

(56)

which can be rewritten as

$$P_{1} (s) = \gamma \cdot \left[ {\frac{2 \cdot \cos \theta }{h} + \frac{2 \cdot \cos \theta }{w} \cdot \frac{2}{{\cos \beta \cdot \left[ {\frac{1}{tg\beta } - \frac{1}{tg\alpha } + \frac{\alpha }{{\sin^{2} \alpha }}} \right] \cdot tg(2\beta )}} + \frac{2 \cdot \alpha }{{\left[ {\frac{\sin \alpha }{tg\beta } - \cos \alpha + \frac{\alpha }{\sin \alpha }} \right] \cdot w}}} \right].$$

(57)