Interaction of surface water waves with a finite dock over two-stepped bottom profile

Abstract

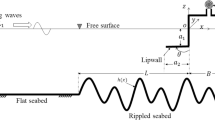
Based on linear water wave theory, scattering of surface waves by a finite dock over two step-type bottom topography is examined. A matched eigenfunction expansion method is employed where both propagating as well as non-propagating modes are considered. The expansion method is applied to the evaluation of the physical quantities, namely, the reflection and transmission coefficients of monochromatic waves caused by the finite dock and the abrupt depth change. These coefficients are validated with the results available in literature for a particular case where a good agreement is achieved. The force and moment on the finite dock are obtained numerically. The effect of various parameters on the reflection coefficient, transmission coefficient, force and moment is studied through different graphs. The energy identity relation, an important factor of the study, is derived and verified. This problem is further generalized to M-steps and the comparison is made between the flat bottom, 2-step bottom and M-steps bottom. The present results are compared with the results available in the literature. In the present study, it is highlighted that the reflection is increasing with increasing the wave number, dock length and width of the step-1 whereas the transmission coefficient is decreasing for the same. Hence, the rigid dock and the two step bottom topography help to create the calm zone in the lee side of the floating rigid dock. This information will be helpful for the marine scientists and engineers while making the breakwaters.

Appendix

1.1 Derivation of energy identity relation

For derivation of the energy identity for Case-I of the present problem, we use the Green’s integral theorem:

$$\begin{aligned} \int _C \left( \phi \frac{\partial \phi ^*}{\partial n}-\phi ^* \frac{\partial \phi }{\partial n}\right) ds=0 \end{aligned}$$

(A1)

where $\phi ^*$ is the complex conjugate of $\phi$, ${\partial }/{\partial n}$ represents the outward normal derivative to the boundary denoted by C of the fluid region

$$\begin{aligned}&y=0 \, (a<x< X); y=0 \, (-a<x< a); y=0 \, (-X<x< -a);\\&x=-X \, (0< y < h_1); \end{aligned}$$

$$\begin{aligned}&y=h_1 \, (-X<x< 0); x=0 \, (h_1< y< h_2); y=h_2 \, (0<x< b);\\&x=b \, (h_2< y < h_3); \end{aligned}$$

$$\begin{aligned}&y=h_3 \, (b<x< X); x=X \, (0<y< h_3). \end{aligned}$$

Then, we take limit as $X \rightarrow \infty$.

The contributions from the lines $y=0 \, ( a<x< X); \, y=0 \, (-a<x< a)$ and $y=0 \, (-X<x< -a)$ are zero.

There are no contributions from the lines $y=h_1 \,(-X<x< 0); \, x=0 \, (h_1< y< h_2); \, y=h_2\, (0<x< b); \, x=b \, (h_2< y< h_3)$ and $y=h_3\, (b<x< X)$.

The contribution from the line $x=-X \,(0< y < h_1)$ is

$$\begin{aligned} i(1-|R|^2)\frac{2k_0h_1+\sinh 2k_0h_1}{2\cosh ^2 k_0h_1}, \end{aligned}$$

(A2)

and from the line $x=X\, (0< y < h_3)$ is

$$\begin{aligned} -i|T|^2\frac{2p_0h_3+\sinh 2p_0h_3}{2\cosh ^2 p_0h_3}. \end{aligned}$$

(A3)

Hence, on combining all the contributions shown above, the relation (A1) produces the energy balance relation as given by

$$\begin{aligned} |R|^2+J_1|T|^2=1, \end{aligned}$$

(A4)

where $\displaystyle J_1=\left( \frac{2p_0h_3+\sinh 2p_0h_3}{2k_0h_1+\sinh 2k_0h_1}\right) \left( \frac{2\cosh ^2 k_0h_1}{2\cosh ^2 p_0h_3}\right)$.

Similarly, the energy balance relation can be derived for the Case-II, when the wave is propagating from positive infinity towards the dock and is given by

$$\begin{aligned} |\hat{R}|^2+J_2|\hat{T}|^2=1, \end{aligned}$$

(A5)

where $\displaystyle J_2=\left( \frac{2k_0h_1+\sinh 2k_0h_1}{2p_0h_3+\sinh 2p_0h_3}\right) \left( \frac{2\cosh ^2 p_0h_3}{2\cosh ^2 k_0h_1}\right)$.