A dynamical study on the adverse effects of industrial activities in the forest and wildlife region through modelling

Abstract

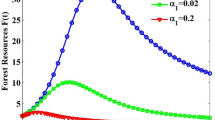
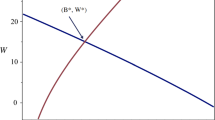
A nonlinear mathematical model is designed and analysed to investigate the impact of forest, non-forest-based industries and their pollutants on forest assets and wildlife population in the forest territory. The pollution from forest and non-forest-based industries is major cause for the depletion of forest resources and wildlife population. In the modelling process, it is assumed that pollutants emitted by both types of industries abate the growth rate of forest resources and wildlife population. However, the forest assets are being depleted by forest-based industries directly. Whether excessive expansion of non-forest-based industries and their pollutants are also major responsible for the loss of ecology between forest resources and wildlife population. The model is expressed in the form of nonlinear dynamical systems considering as different variables and equations. The model is analysed by both ways of method like qualitative and quantitative analysis. Qualitative analysis deduces important results and properties of the model like stability region, finding of equilibriums and their stabilities. However, the model is also analysed by quantitatively to obtained numerical results. It is also deduced that the pollutants which are emitted by non-forest-based industries are more vulnerable to ecology.

Appendices

Appendix A

Following are the transformations that can be made:

$$F = F^{ * } + x_{1} ,\,I_{W} = I_{W}^{ * } + x{}_{2},\,I_{N} = I_{N}^{ * } + x_{3} ,\,P = P^{ * } + x_{4} ,\,W = W^{ * } + x_{5} ,$$

with $x_{1} ,\,x_{2} ,\,x_{3} ,\,x_{4} \,{\text{and}}\,x_{5}$ as minor perturbations around $E_{3}$, the following positive definite function is considered:

$$V = \frac{1}{2}\left( {\frac{{x_{1}^{2} }}{{F^{ * } }} + l_{1} x_{2}^{2} + l_{2} x_{3}^{2} + l_{3} x_{4}^{2} + l_{4} x_{5}^{2} } \right),$$

(51)

where $l_{1} ,\,l_{2} ,\,l_{3} \,{\text{and}}\,l_{4}$ are positive constants.

In Eq. (51), we get the derivative of $V$ with respect to '$t$'.

$$\frac{dV}{{dt}} = \frac{{x_{1} }}{{F^{ * } }}\frac{{dx_{1} }}{dt} + l_{1} x_{2} \frac{{dx_{2} }}{dt} + l_{2} x_{3} \frac{{dx_{3} }}{dt} + l_{3} x_{4} \frac{{dx_{4} }}{dt} + l_{4} x_{5} \frac{{dx_{5} }}{dt}$$

(52)

$$\begin{aligned} \frac{dV}{{dt}} & = - (\frac{r}{L} + \beta_{2} I_{N}^{ * } )x_{1}^{2} - l_{1} (c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )x_{2}^{2} - l_{2} (c_{2} I_{W}^{ * } + \varphi_{2} )x_{3}^{2} - l_{3} (\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )x_{4}^{2} \hfill \\ & \quad - l_{4} (\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } )x_{5}^{2} + ( - \varphi + l_{4} \theta \varphi W^{ * } )x_{1} x_{5} + ( - \beta_{1} + l_{1} \pi_{1} \beta_{1} W^{ * } + l_{1} \pi_{2} I_{N}^{ * } F^{ * } )x_{1} x_{2} \hfill \\ &\quad - \beta_{2} F^{ * } x_{1} x_{3} - (\beta_{3} + l_{3} \theta_{3} P^{ * } )x_{1} x_{4} + (l_{1} \pi_{2} {F^{ * }}^{2} - l_{1} c_{1} I_{W}^{ * } - l_{2} c_{2} I_{N}^{ * } )x_{2} x_{3} + l_{3} \theta_{1} x_{2} x_{4} + l_{3} \theta_{2} x_{3} x_{4} \hfill \\ & \quad + (l_{3} \pi \alpha_{1} P^{ * } - l_{4} \alpha_{1} W^{ * } )x_{4} x_{5} \hfill \\ \end{aligned}$$

(53)

If we choose

$( - \varphi + l_{4} \theta \varphi W^{ * } )$ = 0, this gives $l_{4} = \frac{1}{{\theta W^{ * } }}$.

$( - \beta_{1} + l_{1} \pi_{1} \beta_{1} W^{ * } + l_{1} \pi_{2} I_{N}^{ * } F^{ * } )$ = 0, this gives $l_{1} = \frac{{\beta_{1} }}{{\pi \beta_{1} W^{ * } + \pi_{2} I_{N}^{ * } F^{ * } }}$.

$(l_{1} \pi_{2} {F^{ * }}^{2} - l_{1} c_{1} I_{W}^{ * } - l_{2} c_{2} I_{N}^{ * } )$ = 0, this gives $l_{2} = \frac{{\beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )}}{{c_{2} I_{N}^{ * } (\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )}}$.

With these, Eq. (53) can be written as

$$\begin{aligned} \frac{dV}{{dt}} & = - (\frac{r}{L} + \beta_{2} I_{N}^{ * } )x_{1}^{2} - l_{1} (c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )x_{2}^{2} - l_{2} (c_{2} I_{W}^{ * } + \varphi_{2} )x_{3}^{2} - l_{3} (\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )x_{4}^{2} \hfill \\ & \quad - l_{4} (\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } )x_{5}^{2} - \beta_{2} F^{ * } x_{1} x_{3} - (\beta_{3} + l_{3} \theta_{3} P^{ * } )x_{1} x_{4} + l_{3} \theta_{1} x_{2} x_{4} \hfill \\ & \quad + l_{3} \theta_{2} x_{3} x_{4} + (l_{3} \pi \alpha_{1} P^{ * } - l_{4} \alpha_{1} W^{ * } )x_{4} x_{5} \hfill \\ \end{aligned}$$

(54)

If we choose $l_{3} = 1$, we have.

Now,$\frac{dV}{{dt}}$ will be negative if.

• (i) $( - \beta_{2} F^{ * } )^{2} < \frac{{l_{2} }}{4}(\frac{r}{L} + \beta_{2} I_{N}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )$which can be written as

  $$4c_{2} I_{N}^{ * } (\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )(\beta_{2} F^{ * } )^{2} < (\frac{r}{L} + \beta_{2} I_{N}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )\beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )$$

• (ii) $(\beta_{3} + l_{3} \theta_{3} P^{ * } )^{2} < \frac{{l_{3} }}{8}(\frac{r}{L} + \beta_{2} I_{N}^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$8(\beta_{3} + \theta_{3} P^{ * } )^{2} < (\frac{r}{L} + \beta_{2} I_{N}^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (iii) $(l_{3} \theta_{1} )^{2} < \frac{{l_{1} l_{3} }}{4}(c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$4(\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )\theta_{1}^{2} < \beta_{1} (c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (iv) $(l_{3} \theta_{2} )^{2} < \frac{{l_{2} l_{3} }}{8}(c_{2} I_{W}^{ * } + \varphi_{2} )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$8(\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )c_{2} I_{N}^{ * } \theta_{2}^{2} < \beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (v) $(l_{3} \pi \alpha_{1} P^{ * } - l_{4} \alpha_{1} W^{ * } ) < \frac{{l_{3} l_{4} }}{4}(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )(\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } )$

  which can be written as

  $$4\theta W^{ * } (\pi \alpha_{1} P^{ * } - \frac{{\alpha_{1} }}{\theta })^{2} < (\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )(\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } ).$$

Appendix B

$$U = \left( {F - F^{ * } - F^{ * } \ln \frac{F}{{F^{ * } }}} \right) + \frac{1}{2}m_{1} (I_{W} - I_{W}^{ * } )^{2} + \frac{1}{2}m_{2} (I_{N} - I_{N}^{ * } )^{2} + \frac{1}{2}m_{3} (P - P^{ * } )^{2} + \frac{1}{2}m_{4} (W - W^{ * } )^{2} ,$$

(55)

where $m_{1} ,\,m_{2} ,\,m_{3} \,{\text{and}}\,m_{4}$ are positive constants.

In Eq. (55), we get the derivative of $U$ with respect to '$t$'.

$$\begin{aligned} \frac{dU}{{dt}} & = \frac{{(F - F^{ * } )}}{F}\frac{dF}{{dt}} + m_{1} (I_{W} - I_{W}^{ * } )\frac{{dI_{W} }}{dt} + m_{2} (I_{N} - I_{N}^{ * } )\frac{{dI_{N} }}{dt} + m_{3} (P - P^{ * } )\frac{dP}{{dt}} + m_{4} (W - W^{ * } )\frac{dW}{{dt}} \hfill \\ \frac{dU}{{dt}} & = - \frac{r}{L}(F - F^{ * } )^{2} - m_{1} (c_{1} I_{N} + \varphi_{1} - \pi_{1} \beta_{1} F)(I_{W} - I_{W}^{ * } )^{2} - m_{2} (c_{2} I_{W} + \varphi_{2} )(I_{N} - I_{N}^{ * } )^{2} \hfill \\ & \quad - m_{3} (\theta_{3} F + \mu_{0} - \pi \alpha_{1} W)(P - P^{ * } )^{2} - m_{4} (\delta_{0} W + \theta_{0} + \alpha_{1} P - \theta \varphi F)(W - W^{ * } )^{2} \hfill \\ & \quad+ ( - \varphi + m_{4} \theta \varphi W^{ * } )(F - F^{ * } )(W - W^{ * } ) + ( - \beta_{3} - m{}_{3}\theta_{3} P^{ * } )(F - F^{ * } )(P - P^{ * } ) \hfill \\ & \quad + ( - \beta_{1} + m_{1} \pi_{1} \beta_{1} I_{W}^{ * } + m_{1} \pi_{2} I_{N}^{ * } F^{ * } )(F - F^{ * } )(I_{W} - I_{W}^{ * } ) - \beta_{2} F^{ * } (F - F^{ * } )(I_{N} - I_{N}^{ * } ) \hfill \\ & + (m_{1} \pi_{2} {F^{ * }}^{2} - c_{1} m_{1} I_{W}^{ * } - m_{2} c_{2} I_{N}^{ * } )(I_{W} - I_{W}^{ * } )(I_{N} - I_{N}^{ * } ) + m_{3} \theta_{1} (P - P^{ * } )(I_{W} - I_{W}^{ * } ) \hfill \\ & \quad+ m_{3} \theta_{2} (P - P^{ * } )(I_{N} - I_{N}^{ * } ) + (m_{3} \pi \alpha_{1} P^{ * } - m_{4} \alpha_{1} W^{ * } )(W - W^{ * } )(P - P^{ * } ) \hfill \\ \end{aligned}$$

(56)

If we choose

$( - \varphi + m_{4} \theta \varphi W^{ * } )$ = 0, this gives $m_{4} = \frac{1}{{\theta W^{ * } }}$.

$( - \beta_{1} + m_{1} \pi_{1} \beta_{1} W^{ * } + m_{1} \pi_{2} I_{N}^{ * } F^{ * } )$ = 0, this gives $m_{1} = \frac{{\beta_{1} }}{{\pi \beta_{1} W^{ * } + \pi_{2} I_{N}^{ * } F^{ * } }}$.

$(m_{1} \pi_{2} {F^{ * }}^{2} - m_{1} c_{1} I_{W}^{ * } - m_{2} c_{2} I_{N}^{ * } )$ = 0, this gives $m_{2} = \frac{{\beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )}}{{c_{2} I_{N}^{ * } (\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )}}$.

If we choose $m_{3} = 1$, we have

Now,$\frac{dU}{{dt}}$ will be negative if

• (i) $( - \beta_{2} F^{ * } )^{2} < \frac{{m_{2} }}{4}(\frac{r}{L} + \beta_{2} I_{N}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )$which can be written as

  $$4c_{2} I_{N}^{ * } (\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )(\beta_{2} F^{ * } )^{2} < (\frac{r}{L} + \beta_{2} I_{N}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )\beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )$$

• (ii) $(\beta_{3} + m_{3} \theta_{3} P^{ * } )^{2} < \frac{{m_{3} }}{8}(\frac{r}{L} + \beta_{2} I_{N}^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$8(\beta_{3} + \theta_{3} P^{ * } )^{2} < (\frac{r}{L} + \beta_{2} I_{N}^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (iii) $(m_{3} \theta_{1} )^{2} < \frac{{m_{1} m_{3} }}{4}(c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$4(\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )\theta_{1}^{2} < \beta_{1} (c_{1} I_{N}^{ * } + \varphi_{1} - \pi_{1} \beta_{1} F^{ * } )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (iv) $(m_{3} \theta_{2} )^{2} < \frac{{m_{2} m_{3} }}{8}(c_{2} I_{W}^{ * } + \varphi_{2} )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$which can be written as

  $$8(\pi_{1} \beta_{1} I_{W}^{ * } + \pi_{2} F^{ * } I_{N}^{ * } )c_{2} I_{N}^{ * } \theta_{2}^{2} < \beta_{1} (\pi_{2} {F^{ * }}^{2} - c_{1} I_{W}^{ * } )(c_{2} I_{W}^{ * } + \varphi_{2} )(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )$$

• (v) $(m_{3} \pi \alpha_{1} P^{ * } - m_{4} \alpha_{1} W^{ * } ) < \frac{{m_{3} m_{4} }}{4}(\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )(\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } )$

  which can be written as

  $$4\theta W^{ * } (\pi \alpha_{1} P^{ * } - \frac{{\alpha_{1} }}{\theta })^{2} < (\theta_{3} F^{ * } + \mu_{0} - \pi \alpha_{1} W^{ * } )(\delta_{0} W^{ * } + \theta_{0} + \alpha_{1} P^{ * } - \theta \varphi F^{ * } ).$$