Skip to main content
SpringerLink
Log in

Some extensions of Hilbert–Kunz multiplicity

  • Published:
Collectanea Mathematica Aims and scope Submit manuscript

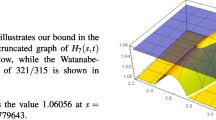
Abstract

Let R be an excellent Noetherian ring of prime characteristic. Consider an arbitrary nested pair of ideals (or more generally, a nested pair of submodules of a fixed finite module). We do not assume that their quotient has finite length. In this paper, we develop various sufficient numerical criteria for when the tight closures of these ideals (or submodules) match. For some of the criteria we only prove sufficiency, while some are shown to be equivalent to the tight closures matching. We compare the various numerical measures (in some cases demonstrating that the different measures give truly different numerical results) and explore special cases where equivalence with matching tight closure can be shown. All of our measures derive ultimately from Hilbert–Kunz multiplicity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Notes

  1. We remark here that by the methods used in proving our Theorem 2.4, the assumption that \(\hat{R}\) is reduced is unnecessary.

  2. Note that rings with FFRT need not be F-regular (or even F-rational). Shibuta [29] proved that if R is any 1-dimensional complete local domain of prime characteristic whose residue field is either finite or algebraically closed, then R has finite F-representation type.

  3. Here too, the reducedness assumption appears to be unnecessary, in light of methods used in the proof of Theorem 2.4.

  4. Recent work of Polstra [24] indicates that this limit exists in some generality, as well as the other limits defined in this section and the next.

References

  1. Aberbach, I.M.: The existence of the F-signature for rings with large \(\mathbb{Q}\)-Gorenstein locus. J. Algebra 319(7), 2994–3005 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  2. Achilles, R., Manaresi, M.: Multiplicity for ideals of maximal analytic spread and intersection theory. J. Math. Kyoto Univ. 33(4), 1029–1046 (1993)

    MathSciNet  MATH  Google Scholar 

  3. Achilles, R., Manaresi, M.: Multiplicities of a bigraded ring and intersection theory. Math. Ann. 309(4), 573–591 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  4. Brenner, H.: Irrational Hilbert-Kunz multiplicities. arXiv:1305.5873 (2013)

  5. Brenner, H., Monsky, P.: Tight closure does not commute with localization. Ann. of Math. (2) 171(1), 571–588 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  6. Buchsbaum, D.A., Eisenbud, D.: What makes a complex exact? J. Algebra 25, 259–268 (1973)

    Article  MathSciNet  MATH  Google Scholar 

  7. Dao, H., Smirnov, I.: On generalized Hilbert–Kunz function and multiplicity. arXiv:1305.1833 (2013)

  8. Dao, H., Watanabe, K.: Some computations of generalized Hilbert–Kunz function and multiplicity. arXiv:1503.00894 (2015)

  9. Epstein, N.: Phantom depth and stable phantom exactness. Trans. Am. Math. Soc. 359(10), 4829–4864 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  10. Epstein, N., Yao, Y.: Criteria for flatness and injectivity. Math. Z. 271(3–4), 1193–1210 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  11. Epstein, N., Yao, Y.: A computation concerning relative Hilbert–Kunz multiplicities. arXiv:1605.01807 (2016)

  12. Flenner, H., Manaresi, M.: A numerical characterization of reduction ideals. Math. Z. 238(1), 205–214 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  13. Hochster, M., Huneke, C.: Tight closure, invariant theory, and the Briançon-Skoda theorem. J. Am. Math. Soc. 3(1), 31–116 (1990)

    MathSciNet  MATH  Google Scholar 

  14. Hochster, M., Huneke, C.: \(F\)-regularity, test elements, and smooth base change. Trans. Am. Math. Soc. 346(1), 1–62 (1994)

    MathSciNet  MATH  Google Scholar 

  15. Hochster, M., Huneke, C.: Localization and test exponents for tight closure. Michigan Math. J. 48, 305–329 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  16. Huneke, C.: Tight Closure and its Applications. In: CBMS Reg. Conf. Ser. in Math., vol. 88, Amer. Math. Soc., Providence (1996)

  17. Huneke, C., Leuschke, G.J.: Two theorems about maximal Cohen–Macaulay modules. Math. Ann. 324(2), 391–404 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  18. Katzman, M., Sharp, R.Y.: Uniform behaviour of the Frobenius closures of ideals generated by regular sequences. J. Algebra 295(1), 231–246 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  19. Kurano, K.: The singular Riemann–Roch theorem and Hilbert–Kunz functions. J. Algebra 304(1), 487–499 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  20. Lam, T.Y.: A First Course in Noncommutative Rings. Graduate Texts in Mathematics. Springer, New York (1991)

    Book  Google Scholar 

  21. Lyubeznik, G.: \(F\)-modules: applications to local cohomology and \(D\)-modules in characteristic \(p>0\). J. Reine Angew. Math. 491, 65–130 (1997)

    MathSciNet  MATH  Google Scholar 

  22. Monsky, P.: The Hilbert–Kunz function. Math. Ann. 263(1), 43–49 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  23. Peskine, C., Szpiro, L.: Dimension projective finie et cohomologie locale. applications à la démonstration de conjectures de M. Auslander, H. Bass et A. Grothendieck, Inst. Hautes Études Sci. Publ. Math. 42, 47–119 (1973)

    Article  MathSciNet  MATH  Google Scholar 

  24. Polstra, T.: Uniform bounds in F-finite rings and lower semi-continuity of the F-signature. arXiv:1506.01073, (2015)

  25. Seibert, G.: Complexes with homology of finite length and Frobenius functors. J. Algebra 125(2), 278–287 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  26. Sharp, R.Y.: Graded annihilators of modules over the Frobenius skew polynomial ring, and tight closure. Trans. Am. Math. Soc 359(9), 4237–4258 (2007) (electronic)

  27. Sharp, R.Y.: On the Hartshorne-Speiser-Lyubeznik theorem about Artinian modules with a Frobenius action. Proc. Am. Math. Soc. 135(3), 665–670 (2007) (electronic)

  28. Sharp, R.Y., Nossem, N.: Ideals in a perfect closure, linear growth of primary decompositions, and tight closure. Trans. Am. Math. Soc. 356(9), 3687–3720 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  29. Shibuta, T.: One-dimensional rings of finite \(F\)-representation type. J. Algebra 332(1), 434–441 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  30. Smith, K.E., van der Bergh, M.: Simplicity of rings of differential operators in prime characteristic. Proc. Lond. Math. Soc. 75(1), 32–62 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  31. Ulrich, B., Validashti, J.: Numerical criteria for integral dependence. Math. Proc. Camb. Philos. Soc. 151(1), 95–102 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  32. Vraciu, A.: An observation on generalized Hilbert-Kunz functions. arXiv:1510.00668, (2015)

  33. Yao, Y.: Modules with finite \(F\)-representation type. J. Lond. Math. Soc. 2(72), 53–72 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  34. Yoshino, Y.: Skew-polynomial rings of Frobenius type and the theory of tight closure. Comm. Algebra 22(7), 2473–2502 (1994)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, George Mason University, Fairfax, VA, 22030, USA

    Neil Epstein

  2. Department of Math and Statistics, Georgia State University, 30 Pryor St., Atlanta, GA, 30303, USA

    Yongwei Yao

Authors
  1. Neil Epstein
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yongwei Yao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Neil Epstein.

Additional information

The second author was partially supported by the National Science Foundation DMS-0700554.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Epstein, N., Yao, Y. Some extensions of Hilbert–Kunz multiplicity. Collect. Math. 68, 69–85 (2017). https://doi.org/10.1007/s13348-016-0174-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s13348-016-0174-2

Keywords

Mathematics Subject Classification

Search

Navigation