An Approach for Increasing Sensitivity of a Tunable Micro Electro Mechanical Sensor Using Electrostatic Hopping Voltage

Abstract

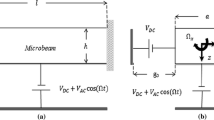
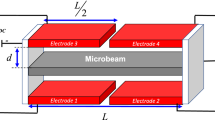
The impetus of the study is to present a novel micro electro mechanical system based tunable gyroscope with the possibility of sensitivity enhancement using appropriate electrostatic hopping voltages. The proposed model is a silicon-based clamped–clamped micro beam sandwiched with two piezoelectric layers throughout the entire length. The nonlinear electrostatic forces are applied to the micro beam along its sense and drive mode directions (either lateral sides). The drive mode actuation is a combination of a direct current (DC) and an alternating current voltage; whereas the sense mode actuation is a pure DC voltage. The micro beam oscillates along the drive mode due to the harmonic drive mode excitation; as the micro beam undergoes base rotation, the Coriolis force induces another motion in the direction of the sense mode which is perpendicular to the drive mode direction. The more is the amplitude of the base rotation, the more is the sense mode amplitude. The sense mode amplitude is directly attributed to the magnitude of base rotation. The piezoelectric layers are actuated by a DC voltage which leads to an axial force proportional to the applied DC voltage. Exciting the piezoelectric layers changes the overall stiffness of the micro beam and as a result the operating frequency of the gyroscope becomes tunable. The partial differential equation of the motion is derived using Hamiltonian principle and discretized into two nonlinear ordinary differential equations along the drive and sense mode directions. The shooting method is used to capture the periodic motion orbits and accordingly the frequency response curves. By using Floquet theory the stability of the periodic orbits is determined. Due to the nonlinearity of the governing equations in the vicinity of the primary resonance, the gyroscope exhibits multi-response solution; Applying appropriate hopping voltages, the micro beam is pushed into the attraction basin of the response with higher amplitude and accordingly the sensitivity of the gyroscope is enhanced. The proposed gyroscope not only has the capability of having improved sensitivity but also its operating frequency can be tuned both in forward and backward directions by means of applying appropriate piezoelectric voltage with an appropriate polarity.

Appendix

The governing motion equations reduces to:

$$ \begin{aligned} & \omega_{n}^{2} \eta_{n} + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\omega_{i}^{2} \eta_{i} \eta_{j} \eta_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\omega_{i}^{2} \eta_{i} \eta_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } \\ & \quad + \frac{{h^{2} }}{{a^{2} }}\left( {\ddot{\eta }_{n} + \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\ddot{\eta }_{i} \eta_{j} \eta_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \ddot{\eta }_{j} } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } \right) + \alpha_{3} \dot{\eta }_{n} \\ & \quad + \alpha_{3} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \dot{\eta }_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\alpha_{3} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \dot{\eta }_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } \\ & \quad - \alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \eta_{k} \varGamma (\varphi_{i} ,\varphi_{j} )} } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{k}^{\prime \prime } dx} - \alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \eta_{k} \eta_{l} \eta_{m} \varGamma (\varphi_{i} ,\varphi_{j} )} } } } } \\ & \quad \int\limits_{0}^{1} {\varphi_{n} \varphi_{m} \varphi_{l} \varphi_{k}^{\prime \prime } dx} + 2\alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\sum\limits_{l = 1}^{M} {\eta_{i} \eta_{j} \eta_{k} \eta_{l} \varGamma (\varphi_{i} ,\varphi_{j} )\int\limits_{0}^{1} {\varphi_{n} \varphi_{l} \varphi_{k}^{\prime \prime } dx} } } } } \\ & \quad - \alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \eta_{k} \varGamma (\varphi_{i} ,\varphi_{j} )} } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{k}^{\prime \prime } dx} - \alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \zeta_{k} \zeta_{l} \eta_{m} \varGamma (\varphi_{i} ,\varphi_{j} )} } } } } \\ & \quad \int\limits_{0}^{1} {\varphi_{n} \varphi_{m} \varphi_{l} \varphi_{k}^{\prime \prime } dx} + 2\alpha_{2} \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\sum\limits_{l = 1}^{M} {\zeta_{i} \zeta_{j} \eta_{k} \eta_{l} \varGamma (\varphi_{i} ,\varphi_{j} )\int\limits_{0}^{1} {\varphi_{n} \varphi_{l} \varphi^{\prime\prime}_{k} dx} } } } } + 2\varOmega_{x} \frac{{h^{2} }}{{a^{2} }} \\ & \quad \left( {\dot{\zeta }_{i} + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \dot{\zeta }_{k} } } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \dot{\zeta }_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } } \right) + \varOmega_{x}^{2} \frac{{h^{2} }}{{a^{2} }}\left( {\eta_{i} } \right) \\ & \quad + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \eta_{k} } } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } = \alpha_{4} (v_{DC} )^{2} \int\limits_{0}^{1} {\varphi_{n} dx} \\ \end{aligned} $$

(A.1)

and

$$ \begin{aligned} & \omega_{n}^{2} \zeta_{n} + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\omega_{i}^{2} \zeta_{i} \zeta_{j} \zeta_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\omega_{i}^{2} \zeta_{i} \zeta_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } \\ & \quad + \ddot{\zeta }_{n} + \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\ddot{\zeta }_{i} \zeta_{j} \zeta_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \ddot{\zeta }_{j} } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } + \alpha_{7} \dot{\zeta }_{n} \\ & \quad + \alpha_{7} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \dot{\zeta }_{k} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} } } } - 2\alpha_{7} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \dot{\zeta }_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } \\ & \quad - \alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \zeta_{k} \varGamma (\varphi_{i} ,\varphi_{j} )} } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{k}^{{\prime \prime }} dx} - \alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \zeta_{k} \zeta_{l} \zeta_{m} \varGamma (\varphi_{i} ,\varphi_{j} )} } } } } \\ & \quad \int\limits_{0}^{1} {\varphi_{n} \varphi_{m} \varphi_{l} \varphi_{k}^{{\prime \prime }} dx} + 2\alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\sum\limits_{l = 1}^{M} {\zeta_{i} \zeta_{j} \zeta_{k} \zeta_{l} \varGamma (\varphi_{i} ,\varphi_{j} )\int\limits_{0}^{1} {\varphi_{n} \varphi_{l} \varphi_{k}^{{\prime \prime }} dx} } } } } \\ & \quad - \alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\eta_{i} \eta_{j} \zeta_{k} \varGamma (\varphi_{i} ,\varphi_{j} )} } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{k}^{{\prime \prime }} dx} - \alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \eta_{k} \eta_{l} \zeta_{m} \varGamma (\varphi_{i} ,\varphi_{j} )} } } } } \\ & \quad \int\limits_{0}^{1} {\varphi_{n} \varphi_{m} \varphi_{l} \varphi_{k}^{{\prime \prime }} dx} + 2\alpha_{6} \sum\limits_{i = 1}^{M} {\sum\limits_{j = 1}^{M} {\sum\limits_{k = 1}^{M} {\sum\limits_{l = 1}^{M} {\eta_{i} \eta_{j} \zeta_{k} \zeta_{l} \varGamma (\varphi_{i} ,\varphi_{j} )\int\limits_{0}^{1} {\varphi_{n} \varphi_{l} \varphi_{k}^{{\prime \prime }} dx} } } } } \\ & \quad - 2\varOmega_{x} \left( {\dot{\eta }_{i} + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \dot{\eta }_{k} } } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \dot{\eta }_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } } \right) + \varOmega_{x}^{2} (\zeta_{i} \\ & \quad + \sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \zeta_{k} } } } \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} \varphi_{k} dx} - 2\sum\limits_{i = 1}^{M} {\sum\limits_{i = 1}^{M} {\zeta_{i} \zeta_{j} \int\limits_{0}^{1} {\varphi_{n} \varphi_{i} \varphi_{j} dx} } } = \alpha_{8} (v_{DC} + v_{AC} \cos (\varOmega t))^{2} \int\limits_{0}^{1} {\varphi_{n} dx} \\ \end{aligned} $$

(A.2)

For n = 1, 2 … M.