Analysis Technique for Exceptional Points in Open Quantum Systems and QPT Analogy for the Appearance of Irreversibility

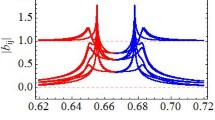
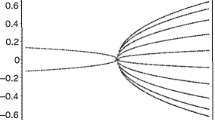
Abstract

We propose an analysis technique for the exceptional points (EPs) occurring in the discrete spectrum of open quantum systems (OQS), using a semi-infinite chain coupled to an endpoint impurity as a prototype. We outline our method to locate the EPs in OQS, further obtaining an eigenvalue expansion in the vicinity of the EPs that gives rise to characteristic exponents. We also report the precise number of EPs occurring in an OQS with a continuum described by a quadratic dispersion curve. In particular, the number of EPs occurring in a bare discrete Hamiltonian of dimension n _D is given by n _D(n _D−1); if this discrete Hamiltonian is then coupled to continuum (or continua) to form an OQS, the interaction with the continuum generally produces an enlarged discrete solution space that includes a greater number of EPs, specifically $2^{n_{\mathrm{C}}} (n_{\mathrm{C}} + n_{\mathrm{D}} ) [ 2^{n_{\mathrm{C}}} (n_{\mathrm{C}} + n_{\mathrm{D}} ) - 1]$, in which n _C is the number of (non-degenerate) continua to which the discrete sector is attached. Finally, we offer a heuristic quantum phase transition analogy for the emergence of the resonance (giving rise to irreversibility via exponential decay) in which the decay width plays the role of the order parameter; the associated critical exponent is then determined by the above eigenvalue expansion.

Appendices

Appendix A: Derivation of the Effective Hamiltonian H _eff for the Prototype Model

In order to keep our presentation reasonably self-contained, we briefly outline the method from Ref. [25] to obtain the effective Hamiltonian reported in Eq. (13) from the full Hamiltonian for our prototype model given in Eq. (8). We start by writing the wavefunction along the chain in generic form as

$$ \psi(x) = C e^{i k x} \quad \mbox{for $x \ge2$} $$

(26)

with C an undetermined normalization constant. To solve the model from this point we find it useful to close the Schrödinger equation on the right with an arbitrary bra 〈x| to obtain

$$ \langle x \vert H \vert \psi \rangle = \epsilon\langle x | \psi\rangle $$

(27)

in which H is the full Hamiltonian from Eq. (8), while x might represent the impurity site or any site along the chain x∈{d,1,2,…}. If we specify any site x>2 and plug Eq. (8) into Eq. (27) then, after applying the usual commutation relations, we find

$$ - \frac{1}{2} \bigl( \langle x-1 | \psi\rangle+ \langle x+1 | \psi \rangle \bigr) = \epsilon_k \langle x | \psi\rangle . $$

(28)

Then plugging in Eq. (26) for the wave function we immediately find ϵ _k =−cosk for the dispersion along the chain. Next we solve for the normalization constant C by choosing x=2 in Eq. (27), which yields

$$ - \frac{1}{2} \bigl( \langle1 | \psi\rangle+ C e^{3ik} \bigr) = \epsilon_k C e^{2ik} . $$

(29)

After applying ϵ _k =−cosk here we re-arrange to find C=〈1|ψ〉e ^−ik.

Finally, evaluating Eq. (27) for the cases x=1 and x=d we obtain the matrix equation

$$ \left ( \begin{array}{c@{\quad}c} \epsilon_\mathrm{d} & - \frac{g}{\sqrt{2}} \\ - \frac{g}{\sqrt{2}} & - \frac{1}{2} e^{i k} \end{array} \right ) \left ( \begin{array}{c} \langle d | \psi\rangle\\ \langle1 | \psi\rangle \end{array} \right ) = z \left ( \begin{array}{c} \langle d | \psi\rangle\\ \langle1 | \psi\rangle \end{array} \right ) , $$

(30)

in which ϵ=z is the discrete eigenvalue associated with the impurity sector. Applying the transformation k=cos⁻¹(−z) in the 2×2 matrix on the LHS of this equation we find $- e^{i k} = z - \sqrt{z^{2} - 1} = \varSigma(z)$, hence we obtain the effective Hamiltonian H _eff reported in Eq. (13) in the main text. We now obtain the characteristic equation by solving det(z−H _eff(z))=0, as reported in Eq. (10).

Appendix B: Comment on the Number of Discrete Solutions Resulting from a General Effective Hamiltonian H _eff

In this Appendix we briefly comment on the number of solutions emerging from the characteristic equation under the effective Hamiltonian treatment (the reader should also consult Appendices D and H of [24] for similar discussions). Let us consider a slightly more general form for H _eff of dimension two as

$$ H_\mathrm{eff} (z) = \left ( \begin{array}{c@{\quad}c} \epsilon_\mathrm{d} & - \frac{g}{\sqrt{2}} \\ - \frac{g}{\sqrt{2}} & - F e^{i k} \end{array} \right ). $$

(31)

(compare this with H _eff from Eq. (30) for the semi-infinite chain model with an endpoint impurity). Note that we may just as well solve the characteristic equation in terms of k rather than z by writing det(z(k)−H _eff(k))=0, which gives

$$ \bigl( e^{ik} + e^{-ik} + 2 \epsilon_{\mathrm{d}} \bigr) \bigl( (1 - 2 F) e^{ik} + e^{-ik} \bigr) - 2 g^2 = 0 $$

(32)

after applying the transformation z(k)=−cosk=(e ^ik+e ^−ik)/2 for a tight-binding chain. Making the replacement w=e ^ik here and multiplying through by w ² immediately yields a fourth order polynomial equation for w, hence a general effective Hamiltonian of dimension two should be expected to yield four discrete eigenvalues. From this point it is not difficult to convince oneself that adding a single discrete impurity site increases the dimension of H _eff by one and simultaneously introduces two new solutions to the characteristic equation (it is in this sense we say that ϵ _k =−cosk represents a quadratic dispersion in the leads). Meanwhile, adding a new channel also increases the dimension of H _eff by one but also requires us to solve a second polynomial equation, hence doubling the total number of solutions after the increase in the dimension of the effective Hamiltonian has already been taken into account.

However, our semi-infinite chain model with an endpoint impurity happens to have the special value F=1/2, which we note is the only value for F under which the order of the polynomial Eq. (32) is reduced from four to two. Hence our present model represents a special case with regard to the number of discrete solutions (and therefore the number of EPs as well).