Skip to main content
SpringerLink
Log in

Multigrid methods for convergent mixed finite difference scheme for Monge–Ampère equation

  • Special Issue IMG 2016
  • Published:
Computing and Visualization in Science

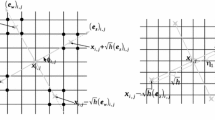
Abstract

We propose multigrid methods for convergent mixed finite difference discretization for the two dimensional Monge–Ampère equation. We apply mixed standard 7-point stencil and semi-Lagrangian wide stencil discretization, such that the numerical solution is guaranteed to converge to the viscosity solution of the Monge–Ampère equation. We investigate multigrid methods for two scenarios. The first scenario considers applying standard 7-point stencil discretization on the entire computational domain. We use full approximation scheme with four-directional alternating line smoothers. The second scenario considers the more general mixed stencil discretization and is used for the linearized problem. We propose a coarsening strategy where wide stencil points are set as coarse grid points. Linear interpolation is applied on the entire computational domain. At wide stencil points, injection as the restriction yields a good coarse grid correction. Numerical experiments show that the convergence rates of the proposed multigrid methods are mesh-independent.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Akian, M., Séquier, P., Sulem, A.: A finite horizon multidimensional portfolio selection problem with singular transactions. In: Decision and Control, 1995., Proceedings of the 34th IEEE Conference on, vol. 3, pp. 2193–2198. IEEE (1995)

  2. Akian, M., Sulem, A., Taksar, M.I.: Dynamic optimization of long-term growth rate for a portfolio with transaction costs and logarithmic utility. Math. Finance 11(2), 153–188 (2001). doi:10.1111/1467-9965.00111

    Article  MathSciNet  MATH  Google Scholar 

  3. Azimzadeh, P., Forsyth, P.A.: Weakly Chained Matrices, Policy Iteration, and Impulse Control. SIAM J. Numer. Anal. 54(3), 1341–1364 (2016). doi:10.1137/15M1043431

    Article  MathSciNet  MATH  Google Scholar 

  4. Bank, R.E., Wan, J.W.L., Qu, Z.: Kernel preserving multigrid methods for convection–diffusion equations. SIAM J. Matrix Anal. Appl. 27(4), 1150–1171 (2006). doi:10.1137/040619533

    Article  MathSciNet  MATH  Google Scholar 

  5. Barles, G., Souganidis, P.E.: Convergence of approximation schemes for fully nonlinear second order equations. Asymptot. Anal. 4(3), 271–283 (1991)

    MathSciNet  MATH  Google Scholar 

  6. Benamou, J.D., Collino, F., Mirebeau, J.M.: Monotone and consistent discretization of the Monge–Ampère operator. Math. Comput. 85(302), 2743–2775 (2016). doi:10.1090/mcom/3080

    Article  MATH  Google Scholar 

  7. Benamou, J.D., Froese, B.D., Oberman, A.M.: Two numerical methods for the elliptic Monge–Ampère equation. M2AN. Math. Model. Numer. Anal. 44(4), 737–758 (2010). doi:10.1051/m2an/2010017

    Article  MathSciNet  MATH  Google Scholar 

  8. Bey, J., Wittum, G.: Downwind numbering: Robust multigrid for convection–diffusion problems. Appl. Numer. Math. 23(1), 177–192 (1997). doi:10.1016/S0168-9274(96)00067-0

    Article  MathSciNet  MATH  Google Scholar 

  9. Bloss, M., Hoppe, R.H.W.: Numerical computation of the value function of optimally controlled stochastic switching processes by multi-grid techniques. Numer. Funct. Anal. Optim. 10(3–4), 275–304 (1989). doi:10.1080/01630568908816304

    Article  MathSciNet  MATH  Google Scholar 

  10. Bokanowski, O., Maroso, S., Zidani, H.: Some convergence results for Howard’s algorithm. SIAM J. Numer. Anal. 47(4), 3001–3026 (2009). doi:10.1137/08073041X

    Article  MathSciNet  MATH  Google Scholar 

  11. Brandt, A.: Multi-level adaptive solutions to boundary-value problems. Math. Comput. 31(138), 333–390 (1977)

    Article  MathSciNet  Google Scholar 

  12. Brandt, A., Yavneh, I.: On multigrid solution of high-Reynolds incompressible entering flows. J. Comput. Phys. 101(1), 151–164 (1992). doi:10.1016/0021-9991(92)90049-5

    Article  MathSciNet  MATH  Google Scholar 

  13. Caffarelli, L.A., Milman M. (eds): Monge–Ampère equation: applications to geometry and optimization. In: Contemporary Mathematics, vol. 226. American Mathematical Society, Providence, RI (1999). doi:10.1090/conm/226

  14. Chen, Y., Wan, J.W.: Monotone mixed narrow/wide stencil finite difference scheme for Monge–Ampère equation. ArXiv preprint arXiv:1608.00644 (2016)

  15. Crandall, M.G., Ishii, H., Lions, P.L.: User’s guide to viscosity solutions of second order partial differential equations. Bull. Am. Math. Soc. (N.S.) 27(1), 1–67 (1992). doi:10.1090/S0273-0979-1992-00266-5

    Article  MathSciNet  MATH  Google Scholar 

  16. Crandall, M.G., Lions, P.L.: Viscosity solutions of Hamilton–Jacobi equations. Trans. Am. Math. Soc. 277(1), 1–42 (1983). doi:10.2307/1999343

    Article  MathSciNet  MATH  Google Scholar 

  17. Debrabant, K., Jakobsen, E.R.: Semi-Lagrangian schemes for linear and fully non-linear diffusion equations. Math. Comput. 82(283), 1433–1462 (2013). doi:10.1090/S0025-5718-2012-02632-9

    Article  MathSciNet  MATH  Google Scholar 

  18. Feng, X., Jensen, M.: Convergent semi-Lagrangian methods for the Monge–Ampère equation on unstructured grids. ArXiv preprint arXiv:1602.04758 (2016)

  19. Forsyth, P.A., Labahn, G.: Numerical methods for controlled Hamilton–Jacobi–Bellman PDEs in finance. J. Comput. Finance 11(2), 1 (2007)

    Article  Google Scholar 

  20. Froese, B.D., Oberman, A.M.: Convergent finite difference solvers for viscosity solutions of the elliptic Monge–Ampère equation in dimensions two and higher. SIAM J. Numer. Anal. 49(4), 1692–1714 (2011). doi:10.1137/100803092

    Article  MathSciNet  MATH  Google Scholar 

  21. Froese, B.D., Oberman, A.M.: Fast finite difference solvers for singular solutions of the elliptic Monge–Ampère equation. J. Comput. Phys. 230(3), 818–834 (2011). doi:10.1016/j.jcp.2010.10.020

    Article  MathSciNet  MATH  Google Scholar 

  22. Froese, B.D., Oberman, A.M.: Convergent filtered schemes for the Monge–Ampère partial differential equation. SIAM J. Numer. Anal. 51(1), 423–444 (2013). doi:10.1137/120875065

    Article  MathSciNet  MATH  Google Scholar 

  23. Han, D., Wan, J.W.L.: Multigrid methods for second order Hamilton–Jacobi–Bellman and Hamilton–Jacob–Bellman–Isaacs equations. SIAM J. Sci. Comput. 35(5), S323–S344 (2013). doi:10.1137/120881476

  24. Hoppe, R.H.W.: Multigrid methods for Hamilton–Jacobi–Bellman equations. Numer. Math. 49(2–3), 239–254 (1986). doi:10.1007/BF01389627

    Article  MathSciNet  MATH  Google Scholar 

  25. Howard, R.A.: Dynamic Programming and Markov Processes. The Technology Press of M.I.T., Cambridge, Mass.; John Wiley & Sons, Inc., New York, London (1960)

    MATH  Google Scholar 

  26. Jameson, A.: Solution of the Euler equations for two-dimensional transonic flow by a multigrid method. Appl. Math. Comput. 13(3–4), 327–355 (1983). doi:10.1016/0096-3003(83)90019-X

    Article  MathSciNet  MATH  Google Scholar 

  27. Krylov, N.V.: The control of the solution of a stochastic integral equation. Teor. Verojatnost. i Primenen. 17, 111–128 (1972)

    MathSciNet  Google Scholar 

  28. Lin, J.: Wide Stencil for the Monge–Ampère Equation. Technical Report, University of Waterloo Master Essay, Supervised by Justin WL Wan, Available on https://uwaterloo.ca/computational-mathematics/sites/ca.computational-mathematics/files/uploads/files/cmmain1.pdf (2014)

  29. Lions, P.L.: Hamilton–Jacobi–Bellman equations and the optimal control of stochastic systems. In: Proceedings of the International Congress of Mathematicians, Vol. 1, 2 (Warsaw, 1983), pp. 1403–1417. PWN, Warsaw (1984)

  30. Ma, K., Forsyth, P.A.: An unconditionally monotone numerical scheme for the two-factor uncertain volatility model. IMA J. Numer. Anal. 37(2), 905–944 (2016)

  31. Napov, A., Notay, Y.: An algebraic multigrid method with guaranteed convergence rate. SIAM J. Sci. Comput. 34(2), A1079–A1109 (2012)

    Article  MathSciNet  Google Scholar 

  32. Notay, Y.: An aggregation-based algebraic multigrid method. Electron. Trans. Numer. Anal. 37, 123–146 (2010)

    MathSciNet  MATH  Google Scholar 

  33. Oberman, A.M.: Wide stencil finite difference schemes for the elliptic Monge–Ampère equation and functions of the eigenvalues of the Hessian. Discrete Contin. Dyn. Syst. Ser. B 10(1), 221–238 (2008). doi:10.3934/dcdsb.2008.10.221

    Article  MathSciNet  MATH  Google Scholar 

  34. Reisinger, C., Arto, J.R.: Boundary treatment and multigrid preconditioning for semi-Lagrangian schemes applied to Hamilton–Jacobi–Bellman equations. ArXiv preprint arXiv:1605.04821 (2016)

  35. Ruge, J.W., Stüben, K.: Algebraic multigrid. In: Multigrid Methods, Frontiers in Applied Mathematics, vol. 3, pp. 73–130. SIAM, Philadelphia, PA (1987)

  36. Seibold, B.: Performance of algebraic multigrid methods for non-symmetric matrices arising in particle methods. Numer. Linear Algebra Appl. 17(2–3), 433–451 (2010). doi:10.1002/nla.710

    Article  MathSciNet  MATH  Google Scholar 

  37. Smears, I.: Hamilton–Jacobi–Bellman Equations Analysis and Numerical Analysis. Technical Report, Research Report Available on www.math.dur.ac.uk/Ug/projects/highlights/PR4/Smears_HJB_report.pdf

  38. Stüben, K.: An introduction to algebraic multigrid. In: Trottenberg, U., Oosterlee, C.W., Schüller, A. (eds.) Multigrid, pp. 413–532. Academic Press Inc, San Diego, CA (2001)

  39. Stüben, K.: A review of algebraic multigrid. J. Comput. Appl. Math. 128(1–2), 281–309 (2001). doi:10.1016/S0377-0427(00)00516-1. Numerical analysis 2000, Vol. VII, Partial differential equations

    Article  MathSciNet  MATH  Google Scholar 

  40. Trottenberg, U., Oosterlee, C.W., Schüller, A.: Multigrid. Academic Press Inc, San Diego, CA (2001)

    MATH  Google Scholar 

  41. Wang, J., Forsyth, P.A.: Maximal use of central differencing for Hamilton–Jacobi–Bellman PDEs in finance. SIAM J. Numer. Anal. 46(3), 1580–1601 (2008). doi:10.1137/060675186

    Article  MathSciNet  MATH  Google Scholar 

  42. Xu, J., Zikatanov, L.T.: Algebraic multigrid methods. ArXiv preprint arXiv:1611.01917 (2016)

Download references

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, University of Waterloo, 200 University Avenue West, Waterloo, ON, N2L 3G1, Canada

    Yangang Chen

  2. David R. Cheriton School of Computer Science, University of Waterloo, 200 University Avenue West, Waterloo, ON, N2L 3G1, Canada

    Justin W. L. Wan

Authors
  1. Yangang Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Justin W. L. Wan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yangang Chen.

Additional information

Communicated by Gabriel Wittum.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, Y., Wan, J.W.L. Multigrid methods for convergent mixed finite difference scheme for Monge–Ampère equation. Comput. Visual Sci. 22, 27–41 (2019). https://doi.org/10.1007/s00791-017-0284-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00791-017-0284-8

Search

Navigation