Skip to main content
SpringerLink
Log in

Vibration analysis of functionally graded thermoelastic nonlocal sphere with dual-phase-lag effect

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

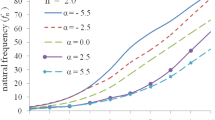
The vibration of a functionally graded axisymmetric nonlocal thermoelastic hollow sphere with dual-phase-lag effect is addressed in this paper. Surfaces of the sphere are assumed to be thermally insulated or isothermal and stress free. According to a simple power law, the material is assumed to be graded in the radial direction. The linear theory of modified thermoelasticity with a dual phase lag based on Eringen’s nonlocal elasticity is employed to model this problem. The Matrix Frobenius method of continued power series is introduced to derive the analytical solutions. The phase velocity relations for the existence of various modes of vibrations in the designed hollow sphere are derived in compact forms. In order to explore the attributes of vibrations, the fixed-point numerical iteration technique is used to solve the secular equations. The numerical computations for the material crust in respect of the natural frequencies, thermoelastic damping and the frequency shifting are presented graphically using MATLAB software tools.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Abbreviations

\(\sigma _{ij}\), \(e_{ij}\) and \(T_{0} \) :

Stress, strain components and reference temperature

u(r, t) and T(r, t):

Displacement and temperature change

\(\lambda \), \(\mu \), \(\rho \), K :

Lame’s, mass density, thermal conductivity parameters

\(\alpha _{\mathrm{T}}\), \(C_{\mathrm{e}} \) :

Coefficient of linear thermal expansion, specific heat at constant strain

\(t_{0}\), \(t_{1}\) :

Phase lag of the heat flux, phase lag of temperature gradient

\(\beta =(3\lambda +2\mu )\alpha _{\mathrm{T}} \) :

Thermoelastic coupling constant

\(\omega \), \(\omega ^{*} \), \(\Omega ^{*}\) :

Circular frequency of vibrations, thermoelastic characteristic frequency, characteristic frequency of vibrations

\(\xi =ea\) :

Nonlocal elastic parameter, where a is internal characteristic length and e is a material constant.

\(R_{\mathrm{I}}\) and \(R_{\mathrm{O}} =\hbar R_{\mathrm{I}} \) :

Inner radius and outer radius (\(\hbar \) is thickness of disk)

\(\hbar =\frac{R_{\mathrm{O}} }{R_{\mathrm{I}} }\) :

Ratio of outer radius to inner radius

\(\varepsilon _{\mathrm{T}} \) :

Thermoelastic coupling parameter

\(c_{1}\), \(c_{2} \) :

Velocity of longitudinal wave, velocity of shear wave

References

  1. Nowacki, W.: Dynamic Problems of Thermoelasticity. Noordhof, Leyden (1975)

    MATH  Google Scholar 

  2. Lord, H.W., Shulman, Y.: Generalized dynamical theory of thermoelasticity. J. Mech. Phys. Solids 15, 299–309 (1967)

    MATH  Google Scholar 

  3. Green, A.E., Lindsay, K.A.: Thermoelasticity. J. Elasticity 2, 1–7 (1972)

    MATH  Google Scholar 

  4. Green, A.E., Naghdi, P.M.: On undamped heat waves in an elastic solid. J. Therm. Stress. 15, 253–264 (1992)

    MathSciNet  Google Scholar 

  5. Green, A.E., Naghdi, P.M.: On thermoelasticity without energy dissipation. J. Elasticity 31, 189–208 (1993)

    MathSciNet  MATH  Google Scholar 

  6. Tzou, D.Y.: A unified field approach for heat conduction from macro to micro-scales. ASME J. Heat. Transf. 117, 8–16 (1995)

    Google Scholar 

  7. Chandrasekharaiah, D.S.: Hyperbolic thermoelasticity: a review of recent literature. Appl. Mech. Rev. 51, 705–729 (1998)

    Google Scholar 

  8. Chou, Y., Yang, R.-J.: Two-dimensional dual-phase-lag thermal behavior in single-/multilayer structures using CESE method. Int. J. Heat Mass Transf. 52, 239–249 (2009)

    MATH  Google Scholar 

  9. Quintanilla, R.: Some solutions for a family of exact phase-lag heat conduction problems. Mech. Res. Commun. 38, 355–360 (2011)

    MATH  Google Scholar 

  10. Chirita, S., Ciarletta, M., Tibullo, V.: On the wave propagation in the time differential dual-phase-lag thermoelastic model. Proc. R. Soc. Lond. Ser. A 471, 20150400 (2015)

    MathSciNet  MATH  Google Scholar 

  11. Marin, M., Baleanu, D., Vlase, S.: Effect of microtemperatures for micropolar thermoelastic bodies. Struct. Eng. Mech. 61, 381–387 (2017)

    Google Scholar 

  12. Marin, M., Craciun, E.M.: Uniqueness results for a boundary value problem in dipolar thermoelasticity to model composite materials. Compos. Part B Eng. 126, 27–37 (2017)

    Google Scholar 

  13. Mondal, S., Sarkar, N., Sarkar, N.: Waves in dual-phase-lag thermoelastic materials with voids based on Eringen’s nonlocal elasticity. J. Therm. Stress. 42, 1035–1050 (2019)

    Google Scholar 

  14. Sarkar, N., Mondal, S.: Thermoelastic interactions in a slim strip due to a moving heat source under dual-phase-lag heat transfer. ASME J. Heat. Transf. 141, 124501 (2019)

    Google Scholar 

  15. Eringen, A.C.: Nonlocal Continuum Field Theories. Springer, New York (2002)

    MATH  Google Scholar 

  16. Eringen, A.C.: On Rayleigh surface waves with small wave lengths. Lett. Appl. Eng. Sci. 1, 11–17 (1973)

    Google Scholar 

  17. Eringen, A.C.: Plane waves in nonlocal micropolar elasticity. Int. J. Eng. Sci. 22, 1113–1121 (1984)

    MATH  Google Scholar 

  18. Roy, I., Acharya, D.P., Acharya, S.: Rayleigh wave in a rotating nonlocal magneto-elastic half-plane. J. Theor. Appl. Mech. 45, 61–78 (2015)

    MATH  Google Scholar 

  19. Narendra, S.: Spectral finite element and nonlocal continuum mechanics based formulation for torsional wave propagation in nano-rods. Finite Elem. Anal. Des. 62, 65–75 (2012)

    MathSciNet  Google Scholar 

  20. Yu, Y.J., Tian, X.G., Liu, X.R.: Size-dependent generalized thermoelasticity using Eringen’s nonlocal model. Eur. J. Mech. A Solids 51, 96–106 (2015)

    MathSciNet  MATH  Google Scholar 

  21. Khurana, A., Tomar, S.K.: Wave propagation in nonlocal microstretch solid. Appl. Math. Model. 40, 5858–5875 (2016)

    MathSciNet  MATH  Google Scholar 

  22. Kaur, G., Singh, D., Tomar, S.K.: Rayleigh-type wave in a nonlocal elastic solid with voids. Eur. J. Mech. A Solids 71, 134–150 (2018)

    MathSciNet  MATH  Google Scholar 

  23. Singh, D., Kaur, G., Tomar, S.K.: Waves in nonlocal elastic solid with voids. J. Elasticity 128, 85–114 (2017)

    MathSciNet  MATH  Google Scholar 

  24. Bachher, M., Sarkar, N.: Nonlocal theory of thermoelastic materials with voids and fractional derivative heat transfer. Waves Random Complex Media 29, 595–613 (2019)

    MathSciNet  Google Scholar 

  25. Biswas, S., Sarkar, N.: Fundamental solution of the steady oscillations equations in porous thermoelastic medium with dual-phase-lag model. Mech. Mater. 126, 140–147 (2018)

    Google Scholar 

  26. Sarkar, N., Tomar, S.K.: Plane waves in nonlocal thermoelastic solid with voids. J. Therm. Stress. 42, 580–606 (2019)

    Google Scholar 

  27. Das, N., Sarkar, N., Lahiri, A.: Reflection of plane waves from the stress-free isothermal and insulated boundaries of a nonlocal thermoelastic solid. Appl. Math. Model. 73, 526–544 (2019)

    MathSciNet  Google Scholar 

  28. Sarkar, N., De, S., Sarkar, N.: Waves in nonlocal thermoelastic solids of type II. J. Therm. Stress. 42, 1153–1170 (2019)

    Google Scholar 

  29. Heyliger, P.R., Pan, E.: Free vibration of layered magnetoelectroelastic spheres. J. Acoust. Soc. Am. 140, 988–999 (2016)

    Google Scholar 

  30. Lamb, H.: On the vibrations of an elastic sphere. Proc. Lond. Math. Soc. 13, 189–212 (1881)

    MathSciNet  MATH  Google Scholar 

  31. Love, A.E.H.: A Treatise on the Mathematical Theory of Elasticity. Dover, New York (1944)

    MATH  Google Scholar 

  32. Sato, Y., Usami, T.: Basic study on the oscillation of homogeneous elastic sphere; part I, frequency of the free oscillations. Geophys. Mag. 31, 15–24 (1962)

    Google Scholar 

  33. Sato, Y., Usami, T.: Basic study on the oscillation of a homogeneous elastic sphere; part II, distribution of displacement. Geophys. Mag. 31, 25–47 (1962)

    Google Scholar 

  34. Erbay, H.A., Erbay, S., Dost, S.: Thermally induced vibrations in a generalized thermoelastic solid with a cavity. J. Therm. Stress. 14, 161–172 (1991)

    MATH  Google Scholar 

  35. Sharma, J.N., Sharma, N.: Three-dimensional free vibration analysis of a homogeneous transradially isotropic thermoelastic sphere. J. Appl. Mech. 77, 021004 (2010)

    Google Scholar 

  36. Sharma, J.N., Sharma, N.: Vibration analysis of homogeneous transradially isotropic generalized thermoelastic spheres. ASME J. Vib. Acoust. 133, 041001 (2011)

    Google Scholar 

  37. Sharma, J.N., Sharma, D.K., Dhaliwal, S.S.: Three-dimensional free vibration analysis of a viscothermoelastic hollow sphere. Open J. Acoust. 2, 12–24 (2012)

    Google Scholar 

  38. Sharma, J.N., Sharma, D.K., Dhaliwal, S.S.: Free vibration analysis of a rigidly fixed viscothermoelastic hollow sphere. Indian J. Pure Appl. Math. 44, 559–586 (2013)

    MathSciNet  MATH  Google Scholar 

  39. Sharma, D.K., Sharma, J.N., Dhaliwal, S.S., Walia, V.: Vibration analysis of axisymmetric functionally graded viscothermoelastic spheres. Acta. Mech. Sin. 30, 100–111 (2014)

    MathSciNet  MATH  Google Scholar 

  40. Keles, I., Tutuncu, N.: Exact analysis of axisymmetric dynamic response of functionally graded cylinders (or disks) and spheres. J. Appl. Mech. 78, 061014 (2011)

    Google Scholar 

  41. Sharma, P.K., Mishra, K.C.: Analysis of thermoelastic response in functionally graded hollow sphere without load. J. Therm. Stress. 40, 185–197 (2017)

    Google Scholar 

  42. Abbas, I.A.: Analytical solution for a free vibration of a thermoelastic hollow sphere. Mech. Based Des. Struct. 43, 265–276 (2015)

    Google Scholar 

  43. Sharma, J.N., Singh, H., Sharma, Y.D.: Modeling of thermoelastic damping and frequency shift of vibrations in a transversely isotropic solid cylinder. Multidiscip. Model Mater. Struct. 7, 245–265 (2011)

    Google Scholar 

  44. Pierce, A.D.: Acoustics: An introduction to Its Physical Principles and Applications. American Institute of Physics, New York (1991)

    Google Scholar 

  45. Tomantschger, K.W.: Series solutions of coupled differential equations with one regular singular point. J. Comput. Appl. Math. 140, 773–783 (2002)

    MathSciNet  MATH  Google Scholar 

  46. Cullen, C.G.: Matrices and Linear Transformation, 2nd edn. Addison-Wesley, Reading (1972)

    MATH  Google Scholar 

  47. Moosapour, M., Hajabasi, M.A., Ehteshami, H.: Thermoelastic damping effect analysis in micro flexural resonator of atomic force microscopy. Appl. Math. Model. 38, 2716–2733 (2014)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the Editor and the anonymous referee for their suggestions and comments to improve the quality of the manuscript.

Author information

Authors and Affiliations

  1. Department of Mathematics, School of Basic and Applied Science, Maharaja Agrasen University, Baddi, Solan, H.P., India

    Dinesh Kumar Sharma

  2. Department of Mathematics, Dinabandhu Mahavidyalaya, Bongaon, West Bengal, 743235, India

    Mitali Bachher

  3. Discipline of Mathematics, Indian Institute of Technology Indore, Indore, 453552, M.P., India

    Santanu Manna

  4. Department of Applied Mathematics, University of Calcutta, Kolkata, 700009, India

    Nantu Sarkar

Authors
  1. Dinesh Kumar Sharma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mitali Bachher
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Santanu Manna
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Nantu Sarkar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nantu Sarkar.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned}&G_{11}^{k} (p_{j} )=\frac{\Omega ^{2}}{\left\{ {(p_{j} +k+2)^{2}\,-n^{2}} \right\} },\end{aligned}$$
(38)
$$\begin{aligned}&G_{12}^{k} (p_{j} )=\frac{\tilde{{\varepsilon }}(p_{j} +k+1+b^{*})}{(p_{j} +k+2)^{2}\,-n^{2}}, \end{aligned}$$
(39)
$$\begin{aligned}&G_{21}^{k} (p_{j} )=\frac{-m_{4} \Omega ^{2}\tilde{{\uptau }}_{0} \,(p_{j} +k+2+b^{*})}{(p_{j} +k+2)^{2}-(a^{*})^{2}}, \end{aligned}$$
(40)
$$\begin{aligned}&G_{22}^{k} (p_{j} )=\frac{\Omega ^{*}\Omega ^{2}\tilde{\uptau }_{0} }{(p_{j} +k+2)^{2}-(a^{*})^{2}} \end{aligned}$$
(41)
$$\begin{aligned}&\left. {\begin{array}{l} d_{11}^{2k} (p_{j} )=G_{12}^{2k-2} (p_{j} )d_{21}^{2k-1} (p_{j} )-G_{11}^{2k-2} (p_{j} )d_{11}^{2k-2} (p_{j} ) \\ d_{22}^{2k} (p_{j} )=G_{21}^{2k-2} (p_{j} )d_{12}^{2k-1} (p_{j} )-G_{22}^{2k-2} (p_{j} )d_{22}^{2k-2} (p_{j} ) \\ \end{array}} \right\} \end{aligned}$$
(42)
$$\begin{aligned}&\left. {\begin{array}{l} d_{12}^{2k+1} (p_{j} )=-\,G_{12}^{2k-1} (p_{j} )d_{22}^{2k} (p_{j} )+G_{11}^{2k-1} (p_{j} )d_{12}^{2k-1} (p_{j} ) \\ d_{21}^{2k+1} (p_{j} )=-\,G_{21}^{2k-1} (p_{j} )d_{11}^{2k} (p_{j} )+G_{22}^{2k-1} (p_{j} )d_{21}^{2k-1} (p_{j} ) \\ \end{array}} \right\} \end{aligned}$$
(43)
$$\begin{aligned}&\left( {{\begin{array}{*{20}c} {d_{11}^{0} (p_{j} )} &{} \quad 0 \\ 0 &{}\quad {d_{22}^{0} (p_{j} )} \\ \end{array} }} \right) \,\,=\,\,\,\left( {{\begin{array}{*{20}c} 1 &{}\quad 0 \\ 0 &{} \quad 1 \\ \end{array} }} \right) ,\,\,\,\,\,\,j=1,2,3,4. \end{aligned}$$
(44)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sharma, D.K., Bachher, M., Manna, S. et al. Vibration analysis of functionally graded thermoelastic nonlocal sphere with dual-phase-lag effect. Acta Mech 231, 1765–1781 (2020). https://doi.org/10.1007/s00707-020-02612-y

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-020-02612-y

Search

Navigation