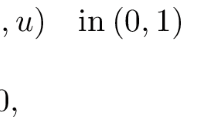Abstract
In this article, we study the fourth-order problem with the first and second derivatives in nonlinearity under nonlocal boundary value conditions
where \(f: [0,1]\times\mathbb{R}_{+}\times\mathbb{R}\times\mathbb{R}_{-}\to \mathbb{R}_{+}\) is continuous, \(h\in L^{1}(0,1)\) and \(\beta_{i}[u]\) is Stieltjes integral (\(i=1,2,3\)). This equation describes the deflection of an elastic beam. Some inequality conditions on nonlinearity f are presented that guarantee the existence of positive solutions to the problem by the theory of fixed point index on a special cone in \(C^{2}[0,1]\). Two examples are provided to support the main results under mixed boundary conditions involving multi-point with sign-changing coefficients and integral with sign-changing kernel.
Similar content being viewed by others
1 Introduction
In this article, we study the existence of positive solutions for fourth-order boundary value problem (BVP) with dependence on the first and second derivatives in nonlinearity subject to boundary conditions of Stieltjes integral type
where \(\beta_{i}[u]=\int_{0}^{1}u(t)\,d\mathcal{B}_{i}(t)\) is Stieltjes integral with \(\mathcal{B}_{i}\) of bounded variation (\(i=1,2,3\)). This equation describes the deflection of an elastic beam.
Alves et al. [1] established the existence of positive solutions for the beam equation
under boundary conditions
where g is a continuous function. Using of the monotonically iterative technique, Yao [2] investigated the positive solution for fourth-order two-point boundary value problem
Li [3] and Ma [4] dealt with the existence of positive solutions for the fourth-order boundary value problem
Their methods are respectively based on fixed point index theory on cones and global bifurcation techniques. Bai [5] and Guo et al. [6] explored the existence of positive solutions respectively for the nonlocal fourth-order problems
and
subject to the same boundary conditions
where \(p,q\in L[0,1]\) are nonnegative. Li [7] discussed the existence of positive solutions for a local fully nonlinear problem
where \(f: [0,1]\times\mathbb{R}_{+}^{3}\times\mathbb{R}_{-}\to\mathbb {R}_{+}\) is continuous. Under the conditions that the nonlinearity \(f(t,x_{1},x_{2},x_{3},x_{4})\) may have superlinear or sublinear growth in \(x_{1}\), \(x_{2}\), \(x_{3}\), \(x_{4}\), the existence of positive solutions is obtained. We also refer to some previous studies, for instance, [8,9,10,11,12]. Recently the existence of positive solutions was proved in [13] to the following problems:
and
where \(\beta_{i}[u]\) and \(\alpha_{i}[u]\) (\(i=1,2,3\)) are Stieltjes integrals of signed measures. All the signs of the derivatives from the first to the third with respect to t of the Green’s functions corresponding to (1.2) and (1.3) do not change, which plays an essential role in [13] when estimating the norms. The readers are referred to [14, 15] for more information and techniques about the issue considered.
Note that the boundary conditions in (1.1) are different from those in (1.2) and (1.3), and both the first and third derivatives with respect to t of the Green’s function corresponding to (1.1) may be sign-changing. We reformulate BVP (1.1) as an integral equation by the method due to Webb and Infante [16], see also [17, 18]. If \(u(0)=u(1)\), the existence of positive solutions to the resulting integral equation is tackled by the theory of fixed point index on a special cone in \(C^{2}[0,1]\) under the inequality conditions posed on the nonlinearity. In particular, the fixed point indexes are computed via the cone expansion and compression conditions of functional type. Two examples are provided to support the main results under mixed boundary conditions involving multi-point with sign-changing coefficients and integral with sign-changing kernel.
2 Preliminaries
In order to prove the main theorems, we need the notion of a fixed point index; see, for example, [19, 20]. Let X be a Banach space, a nonempty subset K is called a cone in X if it is a closed convex set and satisfies the properties that \(\lambda x\in K\) for any \(\lambda>0\), \(x\in K\), and that \(\pm x\in K\) implies \(x=0\) (the zero element in X). We say that \(\alpha: K\to[0,+\infty)\) is a sublinear functional if \(\alpha(tx)\le t\alpha(x)\) for all \(x\in K\), \(t\in[0,1]\). The following lemmas come from [21].
Lemma 2.1
LetKbe a cone in Banach spaceXandΩbe a bounded open subset relative toKwith \(0\in\varOmega\), \(S: \overline{\varOmega}\to K\)is a completely continuous operator. Suppose that \(\alpha: K\to[0,+\infty)\)is a continuous and sublinear functional with \(\alpha(0)=0\), \(\alpha(x)\ne0\)for \(x\ne0\). If \(Sx\ne x\)and \(\alpha(Sx)\le\alpha(x)\)for all \(x\in\partial\varOmega\), then the fixed point index \(i(S,\varOmega,K)=1\).
Lemma 2.2
LetKbe a cone in Banach spaceXandΩbe a bounded open subset relative toKwith \(0\in\varOmega\), \(S: \overline{\varOmega}\to K\)is a completely continuous operator. Suppose that \(\alpha: K\to[0,+\infty)\)is a continuous and sublinear functional with \(\alpha(0)=0\), \(\alpha(x)\ne0\)for \(x\ne0\), and \(\inf_{x\in\partial\varOmega}\alpha(x)>0\). If \(Sx\ne x\), \(\alpha(Sx)\ge\alpha (x)\)for all \(x\in\partial\varOmega\), then the fixed point index \({i(S,\varOmega,K)=0}\).
Let \(X=C^{2}[0,1]\) be the Banach space consisting of all twice continuously differentiable functions on \([0,1]\) with the norm
where \(\|u\|_{C}=\max\{|u(t)|: t\in[0,1]\}\) for \(u\in C[0,1]\). Define an operator in \(C^{2}[0,1]\) as
where \(\gamma_{1}(t)=1\), \(\gamma_{2}(t)=\frac{1}{6}t(1-t)(5-t)\), \(\gamma _{3}(t)=\frac{1}{6}t(1-t)(1+t)\),
in which \(\beta_{i}[u]=\int_{0}^{1}u(t)\,d\mathcal{B}_{i}(t)\) (\(i=1,2,3\)). We set
so \((Tu)(t)=(Bu)(t)+(Fu)(t)\).
We assume throughout this paper that
- \((C_{1})\):
\(f: [0,1]\times\mathbb{R}_{+}\times\mathbb{R}\times\mathbb {R}_{-}\to\mathbb{R}_{+}\) is continuous; here \(\mathbb{R}_{+}=[0,\infty)\) and \(\mathbb{R}_{-}=(-\infty,0]\), \(h\in L^{1}(0,1)\) with \(h(t)\ge0\) and \(\int_{0}^{1}h(t)\,dt>0\).
- \((C_{2})\):
For each \(i\in\{1,2,3\}\), \(\mathcal{B}_{i}\) is of bounded variation and
$$\mathcal{K}_{i}(s):= \int_{0}^{1}k_{0}(t,s)\,d \mathcal{B}_{i}(t)\ge0,\quad \forall s\in[0,1]. $$- \((C_{3})\):
\(\beta_{i}[\gamma_{j}]\ge0\) (\(i,j=1,2,3\)) and for the \(3\times3\) matrix
$$[B] = \left ( \textstyle\begin{array}{c@{\quad}c@{\quad}c} \beta_{1}[\gamma_{1}] & \beta_{1}[\gamma_{2}] & \beta_{1}[\gamma_{3}] \\ \beta_{2}[\gamma_{1}] & \beta_{2}[\gamma_{2}] & \beta_{2}[\gamma_{3}] \\ \beta_{3}[\gamma_{1}] & \beta_{3}[\gamma_{2}] & \beta_{3}[\gamma_{3}] \end{array}\displaystyle \right ), $$its spectral radius \(r([B])<1\).
Writing \(\langle\beta,\gamma\rangle=\sum_{i=1}^{3}\beta_{i}\gamma_{i}\) for the inner product in \(\mathbb{R}^{3}\), we define the operator S in \(C^{2}[0,1]\) as
where \(\beta[Fu]=(\beta_{1}[Fu],\beta_{2}[Fu],\beta_{3}[Fu])^{T}\) is the transposed vector. Similar to [16] we have the following lemmas.
Lemma 2.3
Suppose that \((C_{1})\)holds. Then BVP (1.1) has a solution if and only if there exists a fixed point ofTin \(C^{2}[0,1]\).
Lemma 2.4
Suppose that \((C_{1})\)–\((C_{3})\)hold. ThenScan be written as
where \(\mathcal{K}(s)=(\mathcal{K}_{1}(s),\mathcal{K}_{2}(s),\mathcal {K}_{2}(s))^{T}\), i.e.,
and \(\kappa_{i}(s)\)is theith component of \((I-[B])^{-1}\mathcal{K}(s)\).
Lemma 2.5
If \((C_{2})\)and \((C_{3})\)hold, then \(\kappa _{i}(s)\ge0\) (\(i=1,2\)),
and for \(t, s\in[0,1]\),
where
and
where \(\varPhi_{1}(s)=2\kappa_{2}(s)+\kappa_{3}(s)+s(1-s)\), \(c_{1}(t)=\min\{ t,(1-t)/2\}\).
Proof
Inequality \(\kappa_{i}(s)\ge0\) is due to [16] and we can find in [18] the inequalities
As for (2.7), it can be checked easily. □
Define a cone K in \(C^{2}[0,1]\) as follows:
Lemma 2.6
If \((C_{1})\)–\((C_{3})\)hold, then \(S:P\to K\)is a completely continuous operator.
Proof
For \(u\in P\) and \(t\in[0,1]\), it is easy to see that \(Su\in C^{2}[0,1]\), \((Su)(t)\ge0\) and \((Su)''(t)\le0\). By Lemma 2.5,
Also by Lemma 2.5,
and
hence we have
and
therefore \((Su)(t)\ge c_{0}(t)\|Su\|_{C}\) and \(-(Su)''(t)\ge c_{1}(t)\| (Su)''\|_{C}\) for \(t\in[0,1]\). Moreover, it follows from \((C_{2})\) that
that is, \(Su\in K\). The complete continuity of S is obvious. □
Lemma 2.7
If \((C_{1})\)–\((C_{3})\)hold, thenSandThave the same fixed points inK. As a result, BVP (1.1) has a positive solution if and only ifShas a fixed point inK.
3 Positive solutions of BVP
Take \(\tau\in(0,1/3)\) such that \(\int_{\tau}^{1-\tau}h(t)\,dt>0\) and denote
Define a functional \(\alpha: K\to[0,+\infty)\) as
Clearly, α is a continuous and sublinear functional with \(\alpha (0)=0\). Moreover, since
it is easy to see that \(\alpha(u)\ne0\) for \(u\ne0\).
Theorem 3.1
Suppose that \((C_{1})\)–\((C_{3})\)are satisfied. If there exist constantsaandbwith \(0< b< a\)satisfying \(3b<\tau a\),
for \((t,x_{1},x_{2},x_{3})\in D_{1}=[0,1]\times[0,3b]\times[-3b,3b]\times [-3b,0]\)and
for \((t,x_{1},x_{2},x_{3})\in D_{2}\cup D_{3}\), where
then BVP (1.1) has at least one positive solution.
Proof
Obviously, \(D_{1}\cap(D_{2}\cup D_{3})=\emptyset\) since \(3b<\tau a\). Let
then it is clear that \(\varOmega_{1}\) and \(\varOmega_{2}\) are open sets in K with \(0\in\varOmega_{1}\) and \(\overline{\varOmega}_{1}\subset\varOmega_{2}\).
If \(u\in\varOmega_{2}\), by Lemma 2.5, we have
and
Since \(u(0)=u(1)\), there exists \(\xi\in(0,1)\) such that \(u'(\xi)=0\) and thus
Therefore, \(\varOmega_{2}\) is bounded and \(\|u\|_{C^{2}}<3a\), \(\forall u\in \varOmega_{2}\). Similarly, \(\varOmega_{1}\) is bounded and \(\|u\|_{C^{2}}<3b\), \(\forall u\in\varOmega_{1}\).
If \(u\in\partial\varOmega_{1}\), then \(\alpha(u)=b\) and \(\|u\|_{C^{2}}\le3b\). From Lemma 2.5 and (3.1) it follows that
and hence \(\alpha(Su)\le\alpha(u)\). So by Lemma 2.1 the fixed point index
provided \(Su\ne u\) for \(u\in\partial\varOmega_{1}\).
If \(u\in\partial\varOmega_{2}\), then \(\alpha(u)=a\) and, by Lemma 2.5 for \(t\in[\tau,1-\tau]\),
and
When \(\alpha(u)=a=\max_{\tau\le t\le1-\tau}|u(t)|\), it follows from Lemma 2.5, together with (3.2) and (3.4), that
and hence \(\alpha(Su)\ge\alpha(u)\); when \(\alpha(u)=a=\max_{\tau\le t\le 1-\tau}|u''(t)|\), it similarly follows from Lemma 2.5, together with (3.2) and (3.5), that \(\alpha(Su)\ge\alpha(u)\). So by Lemma 2.2 and since \(\inf_{x\in\partial\varOmega_{2}}\alpha (u)=a>0\), the fixed point index
provided \(Su\ne u\) for \(u\in\partial\varOmega_{2}\).
From (3.3) and (3.6) it follows that the fixed point index
hence S has at least one fixed solution and BVP (1.1) has at least one positive solution. □
Theorem 3.2
Suppose that \((C_{1})\)–\((C_{3})\)are satisfied. If there exist constantsaandbwith \(0< b< a\)satisfying \(a>3h_{0}h_{\tau}^{-1}b\),
for \((t,x_{1},x_{2},x_{3})\in D_{1}\cup D_{2}\), where
and
for \((t,x_{1},x_{2},x_{3})\in D_{3}=[0,1]\times[0,3a]\times[-3a,3a]\times[-3a,0]\), then BVP (1.1) has at least one positive solution.
Proof
Obviously, \(D_{1}\cup D_{2}\subset D_{3}\); however, (3.7) and (3.8) are well-posed since \(a>3h_{0}h_{\tau}^{-1}b\). Letting
we know form the proof of Theorem 3.1 that \(\varOmega_{1}\) and \(\varOmega_{2}\) are bounded open sets in K with \(0\in\varOmega_{1}\) and \(\overline{\varOmega}_{1}\subset\varOmega_{2}\); moreover, \(\|u\|_{C^{2}}<3b\) for \(u\in\varOmega_{1}\) and \(\|u\|_{C^{2}}<3a\) for \(u\in\varOmega_{2}\).
If \(u\in\partial\varOmega_{1}\), then \(\alpha(u)=b\) and, by Lemma 2.5 for \(t\in[\tau,1-\tau]\),
and
When \(\alpha(u)=b=\max_{\tau\le t\le1-\tau}|u(t)|\), it follows from Lemma 2.5, as well as (3.7) and (3.9), that
and hence \(\alpha(Su)\ge\alpha(u)\); when \(\alpha(u)=b=\max_{\tau\le t\le 1-\tau}|u''(t)|\), it similarly follows from Lemma 2.5, together with (3.7) and (3.10), that \(\alpha(Su)\ge\alpha(u)\). So by Lemma 2.2 and since \(\inf_{x\in\partial\varOmega_{1}}\alpha (u)=b>0\), the fixed point index
provided \(Su\ne u\) for \(u\in\partial\varOmega_{1}\).
If \(u\in\partial\varOmega_{2}\), then \(\alpha(u)=a\) and \(\|u\|_{C^{2}}\le3a\). From Lemma 2.5 and (3.8) it follows that
and hence \(\alpha(Su)\le\alpha(u)\). So by Lemma 2.1 the fixed point index
provided \(Su\ne u\) for \(u\in\partial\varOmega_{2}\).
From (3.11) and (3.12) it follows that the fixed point index
hence S has at least one fixed solution and BVP (1.1) has at least one positive solution. □
4 Examples
We consider fourth-order problems under mixed boundary conditions involving multi-point with sign-changing coefficients and integral with sign-changing kernel
that is, \(\beta_{1}[u]=\frac{1}{4}u(\frac{1}{4})-\frac{1}{12}u(\frac {3}{4})\), \(\beta_{2}[u]=\int_{0}^{1}u(t)(2t-\frac{1}{2})\,dt\), \(\beta_{3}[u]=\frac {1}{2}u(\frac{1}{2})-\frac{1}{4}u(\frac{3}{4})\). Hence for \(s\in[0,1]\),
and the \(3\times3\) matrix
Its spectral radius is \(r([B])\thickapprox0.1832<1\). This means that \((C_{2})\) and \((C_{3})\) are satisfied. Moreover,
Take \(\tau=1/4\) and then
Example 4.1
If \(f(t,x_{1},x_{2},x_{3})=x_{1}^{2}+\frac {1+t}{2}x_{2}^{2}+x_{3}^{2}\), then BVP (4.1) has a positive solution.
Proof
For \(a=1600\), \(b=0.01\), it is clear that \(3b< a/4\). Moreover,
for \((t,x_{1},x_{2},x_{3})\in D_{1}=[0,1]\times[0,0.03]\times[-0.03,0.03]\times [-0.03,0]\), and
for \((t,x_{1},x_{2},x_{3})\in([0,1]\times[400,1600]\times[-4800,4800]\times [-4800,0])\cup([0,1]\times[0,4800]\times[-4800,4800]\times[-1600,-400])\). Then BVP (4.1) has a positive solution by Theorem 3.1. □
Example 4.2
If \(f(t,x_{1},x_{2},x_{3})=2000 (1- \frac {1}{1+x_{1}^{2}+(1+t)x_{2}^{4}+x_{3}^{2}} )\), then BVP (4.1) has a positive solution.
Proof
For \(a=1000\), \(b=1\), it is clear that \(a>3h_{0}h_{\tau}^{-1}b\). Moreover,
for \((t,x_{1},x_{2},x_{3})\in[0,1]\times[0,3000]\times[-3000,3000]\times [-3000,0]\), and
for \((t,x_{1},x_{2},x_{3})\in([0,1]\times[1/4,1]\times[-3,3]\times[-3,0])\cup ([0,1]\times[0,3]\times[-3,3]\times[-1,-1/4])\). Then BVP (4.1) has a positive solution by Theorem 3.2. □
References
Alves, E., Ma, T.F., Pelicer, M.L.: Monotone positive solutions for a fourth order equation with nonlinear boundary conditions. Nonlinear Anal. 71, 3834–3841 (2009)
Yao, Q.: Monotonically iterative method of nonlinear cantilever beam equations. Appl. Math. Comput. 205, 432–437 (2008)
Li, Y.: On the existence of positive solutions for the bending elastic beam equations. Appl. Math. Comput. 189, 821–827 (2007)
Ma, R.: Existence of positive solutions of a fourth-order boundary value problem. Appl. Math. Comput. 168, 1219–1231 (2005)
Bai, Z.: Positive solutions of some nonlocal fourth-order boundary value problem. Appl. Math. Comput. 215, 4191–4197 (2010)
Guo, Y., Yang, F., Liang, Y.: Positive solutions for nonlocal fourth-order boundary value problems with all order derivatives. Bound. Value Probl. (2012). https://doi.org/10.1186/1687-2770-2012-29
Li, Y.: Existence of positive solutions for the cantilever beam equations with fully nonlinear terms. Nonlinear Anal., Real World Appl. 27, 221–237 (2016)
Kaufmann, E.R., Kosmatov, N.: Elastic beam problem with higher order derivatives. Nonlinear Anal., Real World Appl. 8, 811–821 (2007)
Li, Y.: Positive solutions of fourth-order boundary value problems with two parameters. J. Math. Anal. Appl. 281, 477–484 (2003)
Minhós, F., Gyulov, T., Santos, A.I.: Lower and upper solutions for a fully nonlinear beam equation. Nonlinear Anal. 71, 281–292 (2009)
Yao, Q.: Local existence of multiple positive solutions to a singular cantilever beam equation. J. Math. Anal. Appl. 363, 138–154 (2010)
Zhang, J., Zhang, G., Li, H.: Positive solutions of second-order problem with dependence on derivative in nonlinearity under Stieltjes integral boundary condition. Electron. J. Qual. Theory Differ. Equ. (2018). https://doi.org/10.14232/ejqtde.2018.1.4
Ma, Y., Yin, C., Zhang, G.: Positive solutions of fourth-order problems with dependence on all derivatives in nonlinearity under Stieltjes integral boundary conditions. Bound. Value Probl. (2019). https://doi.org/10.1186/s13661-019-1155-7
Bai, Z., Du, Z., Zhang, S.: Iterative method for a class of fourth-order p-Laplacian beam equation. J. Appl. Anal. Comput. 9, 1443–1453 (2019)
Wei, Y., Song, Q., Bai, Z.: Existence and iterative method for some fourth order nonlinear boundary value problems. Appl. Math. Lett. 87, 101–107 (2019)
Webb, J.R.L., Infante, G.: Non-local boundary value problems of arbitrary order. J. Lond. Math. Soc. 79, 238–259 (2009)
Infante, G., Pietramala, P.: A cantilever equation with nonlinear boundary conditions. Electron. J. Qual. Theory Differ. Equ. (2009). https://doi.org/10.14232/ejqtde.2009.4.15
Webb, J.R.L., Infante, G., Franco, D.: Positive solutions of nonlinear fourth-order boundary-value problems with local and non-local boundary conditions. Proc. R. Soc. Edinb. A 138, 427–446 (2008)
Deimling, K.: Nonlinear Functional Analysis. Springer, Berlin (1985)
Guo, D., Lakshmikantham, V.: Nonlinear Problems in Abstract Cones. Academic Press, San Diego (1988)
Avery, R., Henderson, J., O’Regan, D.: Functional compression–expansion fixed point theorem. Electron. J. Differ. Equ. 2008, Article ID 22 (2008)
Acknowledgements
The authors express their sincere gratitude to the editors and anonymous referee for the careful reading of the original manuscript and thoughtful comments.
Funding
The project is supported by the Natural Science Foundation of China (61473065) and the National Training Program of Innovation and Entrepreneurship for Undergraduates (191034).
Author information
Authors and Affiliations
Contributions
GZ provided the idea of this article, all authors completed the paper, read and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing interests.
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Wang, S., Chai, J. & Zhang, G. Positive solutions of beam equations under nonlocal boundary value conditions. Adv Differ Equ 2019, 470 (2019). https://doi.org/10.1186/s13662-019-2404-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13662-019-2404-x