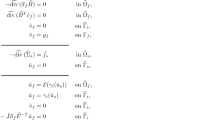Abstract
A priori bounds were derived for the flow in a bounded domain for the viscous-porous interfacing fluids. We assumed that the viscous fluid was slow in \(\Omega _{1}\), which was governed by the Boussinesq equations. For a porous medium in \(\Omega _{2}\), we supposed that the flow satisfied the Darcy equations. With the aid of these a priori bounds we were able to demonstrate the result of the continuous dependence type for the Boussinesq coefficient λ. Following the method of a first-order differential inequality, we can further obtain the result that the solution depends continuously on the interface boundary coefficient α. These results showed that the structural stability is valid for the interfacing problem.
Similar content being viewed by others
1 Introduction
Recently, people have become interested in obtaining stability results of solutions for physical problems of partial differential equations with changes in coefficients. Sometimes the equations themselves are changed. This stability was called the structural stability in order to distinguish it from the traditional stability on initial data and boundary data. These problems were widely studied in many papers by many authors. For the problems of continuum mechanics, it is important for the authors to establish the structural stability of the model. This importance is discussed by Hirsch and Smale [1] in the form of a differential equation. This stability estimation is basic. We want to know whether a slight change in the coefficients in the equations or boundary data, or even the equation itself, will lead to drastic changes in the solution. For a review of the nature of the structural stability, refer to the books written by Ames and Straughan [2] and Straughan [3].
There are many papers studying the structural stability on the coefficients in fluids equations in porous media. Representative is the work of Ames et al. [4, 5], Franchi and Straughan [6], Hoang and Ibragimov [7], Lin and Payne [8–10], Liu [11, 12], Liu et al. [13–15], Scott [16], Scott and Straughan [17], Payne et al. [18–21] and some related papers [22–24]. The previous publications of structural stability usually study one fluid in a bounded domain. Usually, there exists more than one fluid in a domain. These fluids have some interactions. It is desirable to see what effect they can have on each other. So the study of two interfacing fluids may be interesting and meaningful. In [19], the authors studied the structural stability for a flow interfacing with a porous solid. They proved that the solution depends continuously on the coefficient of the interface boundary condition.
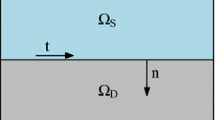
In this paper, we want to study the continuous dependence type results on the interface boundary coefficient and the Boussinesq coefficient for the solution of the Boussinesq–Darcy problem in \(R^{3}\). The Boussinesq equations interface with the Darcy equations through the mutual boundary. Thus, we suggest an appropriate part of the plane \(z=x_{1}=0\) is the mutual boundary for a porous fluid in a bounded region \(\Omega _{2}\) in and a nonlinear viscous fluid in \(\Omega _{1}\) in \(R^{3}\). We denote the interface by L. The remaining part of \(\partial \Omega _{1}\) is denoted by \(\Gamma _{1}\), and the remaining part of \(\partial \Omega _{2}\) is denoted by \(\Gamma _{2}\). We also denote \(\partial \Omega _{1}=\Gamma _{1}\cup L\) and \(\partial \Omega _{2}=\Gamma _{2}\cup L\).
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) denote the velocity, temperature and pressure in \(\Omega _{1}\) and \(\Omega _{2}\), respectively. Then the Boussinesq flow equations are (see [25–27])
where \(g_{i}\) is the gravity force function; λ is the Boussinesq coefficient. The coefficients μ and \(k_{1}\) are kinematic viscosity and thermal conductivity, respectively. From [28, 29]), we can see that the Boussinesq equations are useful in studying fluid and geophysical fluid dynamics.
The Darcy equations can be written as (see Nield and Bejan [30])
where \(\Omega _{1}\) and \(\Omega _{2}\) are all bounded domains. They are all simply connected and star-shaped. The boundaries \(\partial \Omega _{1}\) and \(\partial \Omega _{2}\) are their boundaries, respectively. τ is a positive constant which satisfies \(0<\tau <\infty \). The following boundary conditions are satisfied:
for prescribed functions \(G(x,t)\) and \(\widetilde{G}(x,t)\) and \(n_{i}^{(1)}\), \(n_{i}^{(2)}\) denote the unit outward normals of \(\Omega _{1}\), \(\Omega _{2}\), respectively. Obviously, \(n_{3}^{(1)}=-n_{3}^{(2)}=-1\). The initial conditions are written as
for prescribed functions \(f_{i}\), \(T_{0}\) and \(\theta _{0}\). The interface L conditions are
where α is a positive coefficient and the value of α can be defined by experiment. It is determined by the given fluid and porous solid. The boundary conditions (5) were given by Nield and Bejan in [30]. In [31], Jones deduced the last condition in (5).
In this paper, we want to obtain the continuous dependence on the Boussinesq coefficient λ and the interface boundary coefficient α for the Boussinesq–Darcy interfacing problems in a bounded domain. However, there are only a few papers studying this interfacing problem in a bounded domain (see Payne and Straughan [19] and Liu et al. [13]). For the unbounded domain, refer to Liu et al. [32]. However, compared with the above literature, in this paper, there is a nonlinear term \(u_{i}u_{i,j}\). In particular, the bound of \(\int _{\Omega }u_{i,j}u_{i,j}\,dx\) is needed in this paper. But the methods proposed in [13, 19, 32] cannot be used directly. Second, some well-known Sobolev inequalities cannot be held for the interfacing problem. Our biggest innovation is to overcome these difficulties. We are sure that we can obtain some new and interesting results. We will derive some useful a priori bounds by using different inequalities. With the aid of these a priori bounds, we derive the continuous dependence on the Boussinesq coefficient and the interface boundary coefficient.
In the following discussions, we use the comma to denote partial differentiation. We also use \(u_{i,k}\) to denote the partial differentiation with respect to the direction \(x_{k}\). This is to say \(u_{i,k}=\frac{\partial u_{i}}{\partial x_{k}}\). We also use the usual summation convection with repeated Latin subscripts summed from 1 to 3, and the Greek subscripts summed from 1 to 2. Therefore, \(u_{i,i}=\sum_{i=1}^{3} (\frac{\partial u_{i}}{\partial x_{i}} )^{2}\), \(u_{\beta ,\beta }=\sum_{\beta =1}^{2} ( \frac{\partial u_{\beta }}{\partial x_{\beta }} )^{2}\).
2 A priori bounds
In this section, we want to drive bounds for various norms of \(u_{i}\) in terms of known data which will be used in the next sections.
Lemma 2.1
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\). Then the temperatures satisfy
where \(N_{M}=\max \{\|T_{0}\|_{\infty }, \sup_{[0,\tau ]}\|G\|_{\infty }, \| \theta _{0}\|_{\infty }, \sup_{[0,\tau ]}\|\widetilde{G}\|_{\infty }\}\).
Proof
First, we let \(T_{LM}\) denotes the maximum of the temperature on the interface L. Payne, Rodrigues and Straughan [33] have derived
and
However, in the area \(\Omega _{1}\cup \Omega _{2}\times [0, \tau ]\), the maximum of the temperature cannot be reached on the interface L. Therefore, we have the result (6). □
Lemma 2.2
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\) and \(\Omega _{1}\), \(\Omega _{2}\) are bounded regions. Then
Proof
Multiplying (1)1 by \(u_{i}\), integrating over \(\Omega _{1}\) and using (6), we obtain
By the divergence theorem and (2) and the conditions in the interface, we have
or
From (8) it follows that
Upon integration, we can arrive at Lemma 2.2. □
Now we define
Lemma 2.3
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\) and \(\Omega _{1}\), \(\Omega _{2}\) are bounded regions, then
where \(a_{1}=\frac{1}{\mu }A_{1}+\frac{1}{2\mu }g^{2}N_{M}^{2}|\Omega _{1}|+ \frac{1}{4\mu }g^{2}N_{M}^{2}|\Omega _{2}|\).
Proof
Using the divergence theorem, we have
In the light of the condition on L, we compute
Combining (11) and (12), we have Lemma 2.3. □
Lemma 2.4
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\) and \(\Omega _{1}\), \(\Omega _{2}\) are bounded regions. Then
where \(A_{4}(t)\) is a positive function which will be defined later.
Proof
We firstly compute
We find that the result given in Appendix B of Lin and Payne [10] for \(\|\boldsymbol{{u}}\|_{4}^{2}\)
So, we have for an arbitrary constant \(\varepsilon _{1}>0\)
We notice that one has obtained the following results [13]:
and
where γ and C are positive constants which have been defined in [13]. Following the methods in [13], we can derive a similar result,
Combining (14), (16)–(19) and using (7), we obtain
where we have chosen \(\varepsilon _{1}=\frac{4\mu }{3k_{5}}\).
We now use (1)2 and (2)2 with the boundary conditions (3) and (5) and the divergence theorem to obtain
By integration of (24) we thus obtain
Inserting (25) into (20) and setting
we have
Then, using Lemma 2.3 and the Hölder inequality in (27), we get
for some computable positive constants \(b_{i}\) (\(i=1,2,3,4,5\)). Now, we define
We have from (32)
where \(b_{6}=\frac{1}{b_{4}+M_{0}}\max \{1, \frac{a_{7}}{b_{4}+M_{0}}, \frac{a_{6}}{(b_{4}+M_{0})M_{0}}\}\). Obviously, we have from (34)
where
Therefore, we can get the result
where
In view of (9), Lemma 3 and (18), we also have
and
where
Combining (13), (15) and (34), we may get the following lemma. □
Lemma 2.5
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\) and \(\Omega _{1}\), \(\Omega _{2}\) are bounded regions. Then
3 Continuous dependence on λ
In this section, we want to establish the continuous dependence on \(g_{i}\). Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be solutions of (1)–(5) with \(\lambda =\lambda ^{(1)}\), and \((u^{*}_{i}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) be solutions of (1)–(5) with \(\lambda =\lambda ^{(2)}\), respectively.
We define
and
Then \((w_{i}, T, \pi )\) satisfy the following equations:
and \((w^{m}_{i}, S^{m}, \pi ^{m})\) satisfy the equations
The boundary conditions are
The initial conditions can be written as
The interface L conditions are
We first give some useful lemmas.
Lemma 3.1
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be the classical solutions to the initial-boundary value problem (1)–(5) corresponding to \(\lambda ^{(1)}\), and \((u_{i}^{*}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) also be the classical solutions to the initial-boundary value problem (1)–(5) but corresponding to \(\lambda ^{(2)}\). Then for any \(t>0\) the differences of velocities satisfy
where \(c_{1}(t)\) is a positive function which depends on t.
Proof
We begin with the identity
From (44) it follows that
We now deal with \(I_{1}\) and \(I_{5}\). Using the divergence theorem, we have
Using the Hölder inequality, (15), Lemma 2.4, Lemma 2.5 and the Young inequality with \(\delta _{1}>0\), we have
Following a similar procedure to deriving \(I_{3}\), we obtain
where \(\delta _{2}\), \(\delta _{3}\) are positive constants to be determined later. Similarly, we have
We note that \(I_{6}\) can be bounded,
Similar to (19), we also have
We define the functions \(F_{1}(t)\) and \(F_{2}(t)\) by
Inserting (45)–(51) into (44) and using (52), we have
We choose \(\delta _{1}\), \(\delta _{2}\), \(\delta _{3}\) and \(\delta _{4}\) small enough such that
Letting
we can get Lemma 3.1. □
Lemma 3.2
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be the classical solutions to the initial-boundary value problem (1)–(5) corresponding to \(\lambda ^{(1)}\), and \((u_{i}^{*}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) also be the classical solutions to the initial-boundary value problem (1)–(5) but corresponding to \(\lambda ^{(2)}\). Then for any \(t>0\) we have
Proof
We multiply (39)2 and (40)2 by S and \(S^{m}\), respectively, and integrate by parts to find
Integrating (54) from 0 to t one may deduce
Combining (52) and (55), we may obtain Lemma 3.2. □
Now, we use Lemmas 3.1 and 3.2 to obtain
Setting
we obtain
where
Thus after integration we may derive from (58) the estimate
Combining (57), Lemma 3.2 and (60), we have the following theorem.
Theorem 3.1
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be the classical solutions to the initial-boundary value problem (1)–(5) corresponding to \(\lambda ^{(1)}\), and \((u_{i}^{*}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) also be the classical solutions to the initial-boundary value problem (1)–(5) but corresponding to \(\lambda ^{(2)}\). Then for any \(t>0\) we have
as \(\lambda ^{(1)}\rightarrow \lambda ^{(2)}\). The differences of velocities satisfy
where w, \(\boldsymbol{{w}}^{m}\), S, \(S^{m}\), g̃ have been defined in (37) and (38).
Furthermore, there are two positive \(c_{2}\), \(c_{3}(t)\), such that
Inequalities (62) and (63) demonstrate the continuous dependence on λ in the indicated measure.
4 Continuous dependence on the interface coefficient
In this section, we want to establish the continuous dependence on the interface coefficient α. Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be solutions of (1)–(5) with \(\alpha =\alpha _{1}\), and \((u^{*}_{i}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) be solutions of (1)–(5) with \(\alpha =\alpha _{2}\), respectively.
We define
and
Then \((w_{i}, S, \pi )\) satisfy the following equation:
and \((w^{m}_{i}, S^{m}, \pi ^{m})\) satisfy equations
The boundary conditions are
The initial conditions can be written as
The interface L conditions are
We give some useful lemmas.
Lemma 4.1
If \(T_{0}, \theta _{0}, G, \widetilde{G}\in L^{\infty }\) and \(\Omega _{1}\), \(\Omega _{2}\) are bounded regions, then
where \(A_{6}(t)\) is a positive function which depends on t.
Proof
We use Eqs. (1), (2) to derive
Using the interface conditions, we obtain from (71)
By using the Hölder inequality, the AG mean inequality, (31), (34), Lemma 2.2 and Lemma 2.5, we have from (72)
Therefore
where
□
Lemma 4.2
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be the classical solutions to the initial-boundary value problem (1)–(5) corresponding to \(\lambda ^{(1)}\), and \((u_{i}^{*}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) also be the classical solutions to the initial-boundary value problem (1)–(5) but corresponding to \(\lambda ^{(2)}\). Then for any \(t>0\) we have
Proof
We begin with the identity
From (77) it follows that
Integrating by parts as in Sect. 3 now leads to
Inserting (79), (48), (49), and (50) into (78) and using (51) and (52), we have
Choosing \(\delta _{2}\), \(\delta _{3}\), \(\delta _{4}\) such that
and using Lemma 7 in (80), we have
where
In view of (52), we have from (81)
Defining \(F_{3}(t)\) as in (59), we have from (83)
where
Thus after integration we may derive Lemma 4.2. □
Combining Lemma 8 and Lemma 9, we have the following theorem.
Theorem 4.1
Let \((u_{i}, T, p)\) and \((v_{i}, \theta , q)\) be the classical solutions to the initial-boundary value problem (1)–(5) corresponding to \(\alpha =\alpha _{1}\), and \((u_{i}^{*}, T^{*}, p^{*})\) and \((v^{*}_{i}, \theta ^{*}, q^{*})\) also be the classical solutions to the initial-boundary value problem (1)–(5) but corresponding to \(\alpha =\alpha _{2}\). Then for any \(t>0\) we have
as \(\alpha _{1}\rightarrow \alpha _{2}\). The differences of velocities satisfy
where w, \(\boldsymbol{{w}}^{m}\), S, \(S^{m}\), σ have been defined in (64) and (65), and \(c_{5}\), \(c_{6}\) are positive constants which will be defined later.
Moreover, the differences of the temperatures satisfy
Inequalities (87) and (88) are a priori bounds demonstrating the continuous dependence of the solution on the interface coefficient α.
Availability of data and materials
Not applicable.
References
Hirsch, M.W., Smale, S.: Differential Equations, Dynamical Systems, and Linear Algebra. Academic Press, NewYork (1974)
Ames, K.A., Straughan, B.: Nonstandard and Improperly Posed Problems. Math. Sci. Eng., vol. 194. Academic Press, NewYork (1997)
Straughan, B.: The Energy Method, Stability and Nonlinear Convection, 2nd edn. Appl. Math. Sci., vol. 91. Springer, Berlin (2004)
Ames, K.A., Straughan, B.: Stability and Newton’s law of cooling in double diffusive flow. J. Math. Anal. Appl. 230, 57–69 (1999)
Ames, K.A., Payne, L.E.: Continuous dependence results for solutions of the Navier–Stokes equations backward in time. Nonlinear Anal., Theory Methods Appl. 23(1), 103–113 (1994)
Franchi, F., Straughan, B.: Continuous dependence and decay for the Forchheimer equations. Proc. R. Soc. Lond. A 459, 3195–3202 (2003)
Hoang, L., Ibragimov, A.: Structural stability of generalized Forchheimer equations for compressible fluids in porous media. Nonlinearity 24, 1–41 (2011)
Lin, C., Payne, L.E.: Structural stability for a Brinkman fluid. Math. Methods Appl. Sci. 30, 567–578 (2007)
Lin, C., Payne, L.E.: Structural stability for the Brinkman equations of flow in double diffusive convection. J. Math. Anal. Appl. 325, 1479–1490 (2007)
Lin, C., Payne, L.E.: Continuous dependence on the Soret coefficient for double diffusive convection in Darcy flow. J. Math. Anal. Appl. 342, 311–325 (2008)
Liu, Y.: Convergence and continuous dependence for the Brinkman–Forchheimer equations. Math. Comput. Model. 49, 1401–1415 (2009)
Liu, Y.: Continuous dependence for a thermal convection model with temperature-dependent solubility. Appl. Math. Comput. 308, 18–30 (2017)
Liu, Y., Xiao, S., Lin, Y.W.: Continuous dependence for the Brinkman–Forchheimer fluid interfacing with a Darcy fluid in a bounded domain. Math. Comput. Simul. 150, 66–88 (2018)
Liu, Y., Du, Y., Lin, C.: Convergence results for Forchheimer’s equations for fluid flow in porous media. J. Math. Fluid Mech. 12, 576–593 (2010)
Liu, Y., Du, Y., Lin, C.H.: Convergence and continuous dependence results for the Brinkman equations. Appl. Math. Comput. 215, 4443–4455 (2010)
Scott, N.L.: Continuous dependence on boundary reaction terms in a porous mediu of Darcy type. J. Math. Anal. Appl. 399, 667–675 (2013)
Scott, N.L., Straughan, B.: Continuous dependence on the reaction terms in porous convection with surface reactions. Q. Appl. Math. 71, 501–508 (2013)
Payne, L.E., Song, J.C., Straughan, B.: Continuous dependence and convergence results for Brinkman and Forchheimer models with variable viscosity. Proc. R. Soc. Lond. A 455, 2173–2190 (1999)
Payne, L.E., Straughan, B.: Analysis of the boundary condition at the interface between a viscous fluid and a porous medium and related modelling questions. J. Math. Pures Appl. 77, 317–354 (1998)
Payne, L.E., Straughan, B.: Convergence and continuous dependence for the Brinkman–Forchheimer equations. Stud. Appl. Math. 102, 419–439 (1999)
Payne, L.E., Straughan, B.: Structural stability for the Darcy equations of flow in porous media. Proc. R. Soc. Lond. A 454, 1691–1698 (1998)
Esquivel-Avila, J.A.: Blow-up in damped abstract nonlinear equations. Electron. Res. Arch. 28(1), 347–367 (2020)
Chu, J., Escher, J.: Variational formulations of steady rotational equatorial waves. Adv. Nonlinear Anal. 10(1), 534–547 (2020)
Yang, F., Ning, Z.H., Chen, L.: Exponential stability of the nonlinear Schrödinger equation with locally distributed damping on compact Riemannian manifold. Adv. Nonlinear Anal. 10(1), 569–583 (2020)
Diaz, J.I., Galiano, G.: On the Boussinesq system with nonlinear thermal diffusion. Nonlinear Anal. 30, 3255–3263 (1997)
Gunzburger, M., Saka, Y., Wang, X.M.: Well-posedness of the infinite Prandtl number model for convection with temperature-dependent viscosity. Anal. Appl. 7, 297–308 (2009)
Turcotte, D.L., Schubert, G.: Geodynamics Applications of Continuum Physics to Geological Problems. Wiley, New York (1982)
Majda, A.J.: Introduction to PDEs and Waves for the Atmosphere and Ocean. Courant Lecture Notes in Mathematics, vol. 9. AMS/CIMS (2003)
Pedlosky, J.: Geophysical Fluid Dynamics. Springer, New York (1987)
Nield, D.A., Bejan, A.: Convection in Porous Media. Springer, New York (1992)
Jones, I.P.: Lower Reynolds number flow past a porous spherical shell. Proc. Camb. Philos. Soc. 73, 231–238 (1973)
Liu, Y., Xiao, S.: Structural stability for the Brinkman fluid interfacing with a Darcy fluid in an unbounded domain. Nonlinear Anal., Real World Appl. 42, 308–333 (2018)
Payne, L.E., Rodrigues, J.F., Straughan, B.: Effect of anisotropic permeability on Darcy’s law. Math. Methods Appl. Sci. 24, 427–438 (2001)
Acknowledgements
The authors would like to thank the referees for their helpful comments and suggestions.
Funding
This research was supported by the Foundation for natural Science in Higher Education of Guangdong (Grant No. 2019KZDXM042).
Author information
Authors and Affiliations
Contributions
YL proposed the main idea of this paper and wrote the whole paper. ZS prepared the manuscript initially. LC performed all the steps of the proofs in this research. All authors read and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing interests.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Li, Y., Zhang, S. & Lin, C. Structural stability for the Boussinesq equations interfacing with Darcy equations in a bounded domain. Bound Value Probl 2021, 27 (2021). https://doi.org/10.1186/s13661-021-01501-0
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13661-021-01501-0