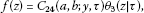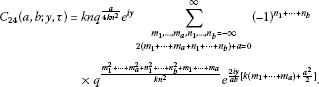Some circular summation formulas for theta functions

Abstract

In this paper, we obtain some circular summation formulas of theta functions using the theory of elliptic functions and show some interesting identities of theta functions and applications.

MSC:11F27, 33E05, 11F20.

1 Introduction

Throughout this paper we take $q=e^{i\pi \tau }$, where $\mathrm{\Im }(\tau )>0$. The classical Jacobi theta functions ${\theta }_i(z|\tau )$, $i=1,2,3,4$, are defined as follows:

(1.1)

(1.2)

(1.3)

(1.4)

Recently, Chan, Liu and Ng [1] proved Ramanujan’s circular summation formulas and derived identities similar to Ramanujan’s summation formula and connected these identities to Jacobi’s elliptic functions.

Subsequently, Zeng [2] gave a generalized circular summation of the theta function ${\theta }_3(z|\tau )$ as follows:

(1.5)

where

$${\mathcal{C}}_{33}(a,b;y,\tau )=kn\underset{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}{\overset{\mathrm{\infty }}{\sum _{m_1+\cdots +m_a+n_1+\cdots +n_b=0}}}{q}^{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2}{e}^{2k(m_1+\cdots +m_a)iy}.$$

A special case of formula (1.5) yields the following result (see [[1], Theorem 3.1]):

$$\sum _{s=0}^{kn-1}{\theta }_3^k(\frac{z}{kn}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2})={\mathcal{C}}_{33}(k,0;0,\frac{\tau }{k{n}^2}){\theta }_3(z|\tau ),$$

(1.6)

where

$${\mathcal{C}}_{33}(k,0;0,\tau )=kn\underset{m_1,\dots ,m_k=-\mathrm{\infty }}{\overset{\mathrm{\infty }}{\sum _{m_1+\cdots +m_k=0}}}{q}^{m_1^2+\cdots +m_k^2}.$$

(1.7)

Upon a, b, n and k are any positive integer with $k=a+b$.

More recently, Liu further obtained the general formulas for theta functions (see [3]), but from one main result, Theorem 1 of Liu, we do not deduce our results in the present paper. Many people research the circular summation formulas of theta functions and find more interesting formulas (see, for details, [4–15]).

In the present paper, we obtain analogues and uniform formulas for theta functions ${\theta }_1(z|\tau )$, $a{\theta }_2(z|\tau )$, ${\theta }_3(z|\tau )$ and ${\theta }_4(z|\tau )$. We now state our result as follows.

Theorem 1 For any positive integer k, n, a and b with $k=a+b$, $\alpha =1,2$, $\beta =3,4$.

• For a, b even, we have

  

  (1.8)

• For a even, n and b odd, we have

  

  (1.9)
  

  (1.10)

where

$$\begin{array}{rcl}{\mathcal{C}}_{\alpha \beta }(a,b;y,\tau )& =& kn{i}^{a\alpha }{q}^{\frac{a}{4}}{e}^{abiy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{(-1)}^{\alpha (m_1+\cdots +m_a)+(\beta +1)(n_1+\cdots +n_b)}\\ \times q^{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{e}^{(2k(m_1+\cdots +m_a)+a^2)iy}.\end{array}$$

(1.11)

2 Proof of Theorem 1

From Jacobi’s theta functions (1.1)-(1.4), we have the following properties respectively:

(2.1)

(2.2)

(2.3)

(2.4)

From (2.1)-(2.4), by using the induction, we easily obtain

(2.5)

(2.6)

(2.7)

(2.8)

Let

$$f(z)=\sum _{s=0}^{kn-1}{\theta }_{\alpha }^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_{\beta }^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).$$

(2.9)

Case 1. When $\alpha =1$, $\beta =3$.

The function $f(z)$ becomes of the following form:

$$f(z)=\sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).$$

(2.10)

From (2.10) we easily obtain

(2.11)

(2.12)

Comparing (2.11) and (2.12), when a is even, we get

$$f(z+\pi )=f(z).$$

(2.13)

By (2.5) and (2.7), and noting that $a+b=k$, we obtain

$$\begin{array}{rcl}f(z+\pi \tau )& =& \sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2})\\ =& {(-1)}^{na}{q}^{-1}{e}^{-2iz}\sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).\end{array}$$

(2.14)

Obviously, when a is even, we have

$$f(z+\pi \tau )=q^{-1}{e}^{-2iz}f(z).$$

(2.15)

We construct the function $\frac{f(z)}{{\theta }_3(z|\tau )}$. By (2.13) and (2.15), we find that the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is an elliptic function with double periods π and πτ and only has a simple pole at $z=\frac{\pi }{2}+\frac{\pi \tau }{2}$ in the period parallelogram. Hence the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is a constant, say, this constant is denoted by $C_{13}(a,b;y,\tau )$, i.e.,

$$\frac{f(z)}{{\theta }_3(z|\tau )}=C_{13}(a,b;y,\tau ),$$

we have

$$f(z)=C_{13}(a,b;y,\tau ){\theta }_3(z|\tau ).$$

(2.16)

By (1.3), (2.10) and (2.16), we have

(2.17)

By (1.1) and (1.3), we obtain

(2.18)

By equating the constant term of both sides of (2.18), we obtain

(2.19)

Clearly,

$$C_{13}(a,b;y,\tau )={\mathcal{C}}_{13}(a,b;\frac{y}{ab},\frac{\tau }{k{n}^2}),$$

(2.20)

where

(2.21)

In the same manner as in Case 1, we can obtain Case 2 below.

Case 2. When $\alpha =2$, $\beta =3$.

The function $f(z)$ becomes of the following form:

$$f(z)=\sum _{s=0}^{kn-1}{\theta }_2^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).$$

(2.22)

From (2.22) we easily obtain

(2.23)

(2.24)

Comparing (2.23) and (2.24), when a is even, we get

$$f(z+\pi )=f(z).$$

(2.25)

By (2.6) and (2.7), and noting that $a+b=k$, we obtain

$$\begin{array}{rcl}f(z+\pi \tau )& =& \sum _{s=0}^{kn-1}{\theta }_2^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2})\\ =& q^{-1}{e}^{-2iz}\sum _{s=0}^{kn-1}{\theta }_2^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_3^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).\end{array}$$

(2.26)

Obviously, we have

$$f(z+\pi \tau )=q^{-1}{e}^{-2iz}f(z).$$

(2.27)

We construct the function $\frac{f(z)}{{\theta }_3(z|\tau )}$. By (2.25) and (2.27), we find that the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is an elliptic function with double periods π and πτ and only has a simple pole at $z=\frac{\pi }{2}+\frac{\pi \tau }{2}$ in the period parallelogram. Hence the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is a constant, say, this constant is denoted by $C_{23}(a,b;y,\tau )$, i.e.,

$$\frac{f(z)}{{\theta }_3(z|\tau )}=C_{23}(a,b;y,\tau ),$$

we have

$$f(z)=C_{23}(a,b;y,\tau ){\theta }_3(z|\tau ).$$

(2.28)

By (1.3), (2.22) and (2.28), we have

(2.29)

By (1.2) and (1.3), we obtain

(2.30)

By equating the constant term of both sides of (2.30), we obtain

$$\begin{array}{rcl}{C}_{23}(a,b;y,\tau )& =& kn{q}^{\frac{a}{4k{n}^2}}{e}^{iy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{q}^{\frac{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{k{n}^2}}\\ \times e^{\frac{2iy}{ab}[k(m_1+\cdots +m_a)+\frac{a^2}{2}]}.\end{array}$$

(2.31)

Clearly,

$$C_{23}(a,b;y,\tau )={\mathcal{C}}_{23}(a,b;\frac{y}{ab},\frac{\tau }{k{n}^2}),$$

(2.32)

where

$$\begin{array}{rcl}{\mathcal{C}}_{23}(a,b;y,\tau )& =& kn{q}^{\frac{a}{4}}{e}^{abiy}\sum _{\begin{array}{c}{m}_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }\\ 2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0\end{array}}^{\mathrm{\infty }}{q}^{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}\\ \times e^{(2k(m_1+\cdots +m_a)+a^2)iy}.\end{array}$$

(2.33)

Case 3. When $\alpha =1$, $\beta =4$.

The function $f(z)$ becomes of the following form:

$$f(z)=\sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_4^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).$$

(2.34)

From (2.34) we easily obtain

(2.35)

(2.36)

Comparing (2.35) and (2.36), when a is even, we have

$$f(z+\pi )=f(z).$$

(2.37)

By (2.5) and (2.8), and noting that $a+b=k$, we obtain

$$\begin{array}{rcl}f(z+\pi \tau )& =& \sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2}){\theta }_4^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}+n\pi \frac{\tau }{k{n}^2}|\frac{\tau }{k{n}^2})\\ =& {(-1)}^{kn}{q}^{-1}{e}^{-2iz}\sum _{s=0}^{kn-1}{\theta }_1^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_4^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).\end{array}$$

(2.38)

• When a and b are even, then kn is also even, we have

  $$f(z+\pi \tau )=q^{-1}{e}^{-2iz}f(z).$$

  (2.39)

We construct the function $\frac{f(z)}{{\theta }_3(z|\tau )}$, by (2.37) and (2.39), we find that the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is an elliptic function with double periods π and πτ and only has a simple pole at $z=\frac{\pi }{2}+\frac{\pi \tau }{2}$ in the period parallelogram. Hence the function $\frac{f(z)}{{\theta }_3(z|\tau )}$ is a constant, say, this constant is denoted by $C_{14}(a,b;y,\tau )$, i.e.,

$$\frac{f(z)}{{\theta }_3(z|\tau )}=C_{14}(a,b;y,\tau ),$$

we have

$$f(z)=C_{14}(a,b;y,\tau ){\theta }_3(z|\tau ).$$

(2.40)

By (1.3), (2.34) and (2.40), we have

(2.41)

By (1.1) and (1.4), we obtain

(2.42)

By equating the constant term of both sides of (2.42), we obtain

$$\begin{array}{rcl}{C}_{14}(a,b;y,\tau )& =& kn{i}^a{q}^{\frac{a}{4k{n}^2}}{e}^{iy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{(-1)}^{m_1+\cdots +n_b}\\ \times q^{\frac{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{k{n}^2}}{e}^{\frac{2iy}{ab}[k(m_1+\cdots +m_a)+\frac{a^2}{2}]}.\end{array}$$

(2.43)

• When a is even, n and b are odd, then kn is also odd, we have

  $$f(z+\pi \tau )=-q^{-1}{e}^{-2iz}f(z).$$

  (2.44)

We construct the function $\frac{f(z)}{{\theta }_4(z|\tau )}$. By (2.37) and (2.44), we find that the function $\frac{f(z)}{{\theta }_4(z|\tau )}$ is an elliptic function with double periods π and πτ and only has a simple pole at $z=\frac{\pi }{2}+\frac{\pi \tau }{2}$ in the period parallelogram. Hence the function $\frac{f(z)}{{\theta }_4(z|\tau )}$ is a constant, say, this constant is denoted by $C_{14}(a,b;y,\tau )$, i.e.,

$$\frac{f(z)}{{\theta }_4(z|\tau )}=C_{14}(a,b;y,\tau ),$$

we have

$$f(z)=C_{14}(a,b;y,\tau ){\theta }_4(z|\tau ).$$

(2.45)

By (1.4), (2.34) and (2.45), we have

(2.46)

By (1.1) and (1.4), we obtain

(2.47)

By equating the constant term of both sides of (2.47), we obtain

$$\begin{array}{rcl}{C}_{14}(a,b;y,\tau )& =& kn{i}^a{q}^{\frac{a}{4k{n}^2}}{e}^{iy}\sum _{\begin{array}{c}{m}_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }\\ 2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0\end{array}}^{\mathrm{\infty }}{(-1)}^{m_1+\cdots +n_b}\\ \times q^{\frac{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{k{n}^2}}{e}^{\frac{2iy}{ab}[k(m_1+\cdots +m_a)+\frac{a^2}{2}]}.\end{array}$$

(2.48)

Clearly, in (2.43) and (2.48), we have

$$C_{14}(a,b;y,\tau )={\mathcal{C}}_{14}(a,b;\frac{y}{ab},\frac{\tau }{k{n}^2}),$$

where

$$\begin{array}{rcl}{\mathcal{C}}_{14}(a,b;y,\tau )& =& kn{i}^a{q}^{\frac{a}{4}}{e}^{abiy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{(-1)}^{m_1+\cdots +n_b}\\ \times q^{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{e}^{(2k(m_1+\cdots +m_a)+a^2)iy}.\end{array}$$

(2.49)

In the same manner as in Case 3, we can obtain Case 4 below.

Case 4. When $\alpha =2$, $\beta =4$.

The function $f(z)$ becomes of the following form:

$$f(z)=\sum _{s=0}^{kn-1}{\theta }_2^a(\frac{z}{kn}+\frac{y}{a}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}){\theta }_4^b(\frac{z}{kn}-\frac{y}{b}+\frac{\pi s}{kn}|\frac{\tau }{k{n}^2}).$$

(2.50)

• When a and b are even, we have

  

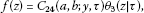  (2.51)
  

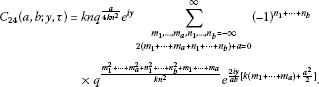  (2.52)

• When a is even, n and b are odd, we have

  $$f(z)=C_{24}(a,b;y,\tau ){\theta }_4(z|\tau ),$$

  (2.53)

$$\begin{array}{rcl}{C}_{24}(a,b;y,\tau )& =& kn{q}^{\frac{a}{4k{n}^2}}{e}^{iy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{(-1)}^{n_1+\cdots +n_b}\\ \times q^{\frac{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}{k{n}^2}}{e}^{\frac{2iy}{ab}[k(m_1+\cdots +m_a)+\frac{a^2}{2}]}.\end{array}$$

(2.54)

Clearly, in (2.52) and (2.54), we have

$$C_{24}(a,b;y,\tau )={\mathcal{C}}_{24}(a,b;\frac{y}{ab},\frac{\tau }{k{n}^2}),$$

where

$$\begin{array}{rcl}{\mathcal{C}}_{24}(a,b;y,\tau )& =& kn{q}^{\frac{a}{4}}{e}^{abiy}\underset{2(m_1+\cdots +m_a+n_1+\cdots +n_b)+a=0}{\overset{\mathrm{\infty }}{\sum _{m_1,\dots ,m_a,n_1,\dots ,n_b=-\mathrm{\infty }}}}{(-1)}^{n_1+\cdots +n_b}{q}^{m_1^2+\cdots +m_a^2+n_1^2+\cdots +n_b^2+m_1+\cdots +m_a}\\ \times e^{(2k(m_1+\cdots +m_a)+a^2)iy}.\end{array}$$

(2.55)

Therefore we complete the proof of Theorem 1.

3 Some special cases of Theorem 1

In this section we give some special cases of Theorem 1 and obtain some interesting identities of theta functions.

Corollary 1 For any positive integer n, we have

(3.1)

(3.2)

(3.3)

(3.4)

where ${\mathcal{C}}_{13}(2,2;y,\tau )$ and ${\mathcal{C}}_{24}(2,2;y,\tau )$ are defined by (2.21) and (2.55), respectively.

Proof Taking $a=b=2$ and $\alpha =1$, $\beta =3$ in (1.11), we have

(3.5)

Taking $a=b=2$ and $\alpha =2$, $\beta =4$ in (1.11), we have

$$\begin{array}{rcl}{\mathcal{C}}_{24}(2,2;y,\tau )& =& 4n{q}^{\frac{1}{2}}\underset{m_1+m_2+n_1+n_2+1=0}{\overset{\mathrm{\infty }}{\sum _{m_1,m_2,n_1,n_2=-\mathrm{\infty }}}}{(-1)}^{n_1+n_2}{q}^{m_1^2+m_2^2+n_1^2+n_2^2+m_1+m_2}{e}^{8iy(m_1+m_2)}{e}^{5iy}\\ =& 4n{e}^{-3iy}{\theta }_1^2(4y|\tau ){\theta }_3^2(0|\tau ).\end{array}$$

(3.6)

Obviously, we find that ${\mathcal{C}}_{13}(2,2;y,\tau )={\mathcal{C}}_{24}(2,2;y,\tau )=4n{e}^{-3iy}{\theta }_1^2(4y|\tau ){\theta }_3^2(0|\tau )$. □

Taking $n=1$ and letting $z\mapsto 4z$, $y\mapsto 2y$, $\tau \mapsto 4\tau$ in Corollary 1, we get the following identities for theta functions:

(3.7)

(3.8)

(3.9)

Further, taking $y=0$ in the above identities, we obtain the following additive formulas of the theta function ${\theta }_3(z|\tau )$:

$$\begin{array}{rcl}{\theta }_3(4z|4\tau )& =& \frac{1}{4{\theta }_1^2{\theta }_3^2}[{\theta }_1^2(z|\tau ){\theta }_3^2(z|\tau )+{\theta }_1^2(z+\frac{\pi }{4}|\tau ){\theta }_3^2(z+\frac{\pi }{4}|\tau )\\ +{\theta }_1^2(z+\frac{\pi }{2}|\tau ){\theta }_3^2(z+\frac{\pi }{2}|\tau )+{\theta }_1^2(z+\frac{3\pi }{4}|\tau ){\theta }_3^2(z+\frac{3\pi }{4}|\tau )]\end{array}$$

(3.10)

$$\begin{array}{rc}=& \frac{1}{4{\theta }_1^2{\theta }_3^2}[{\theta }_2^2(z|\tau ){\theta }_4^2(z|\tau )+{\theta }_2^2(z+\frac{\pi }{4}|\tau ){\theta }_4^2(z+\frac{\pi }{4}|\tau )\\ +{\theta }_2^2(z+\frac{\pi }{2}|\tau ){\theta }_4^2(z+\frac{\pi }{2}|\tau )+{\theta }_2^2(z+\frac{3\pi }{4}|\tau ){\theta }_4^2(z+\frac{3\pi }{4}|\tau )],\end{array}$$

(3.11)

where ${\theta }_1={\theta }_1(0|\tau )$, ${\theta }_3={\theta }_3(0|\tau )$.

Similarly, we have the following.

Corollary 2 For any positive integer n, we have

(3.12)

(3.13)

(3.14)

(3.15)

where ${\mathcal{C}}_{14}(2,2;y,\tau )={\mathcal{C}}_{23}(2,2;y,\tau )=4n{e}^{-3iy}{\theta }_2^2(4y|\tau ){\theta }_3^2(0|\tau )$ are defined by (2.49) and (2.33), respectively.

Taking $n=1$ and letting $z\mapsto 4z$, $y\mapsto 2y$, $\tau \mapsto 4\tau$ in Corollary 2, we get the following identities of theta functions:

(3.16)

(3.17)

(3.18)

Further, taking $y=0$ in the above identities, we obtain other additive formulas for the theta function ${\theta }_3(z|\tau )$ as follows:

$$\begin{array}{rcl}{\theta }_3(4z|4\tau )& =& \frac{1}{4{\theta }_2^2{\theta }_3^2}[{\theta }_1^2(z|\tau ){\theta }_4^2(z|\tau )+{\theta }_1^2(z+\frac{\pi }{4}|\tau ){\theta }_4^2(z+\frac{\pi }{4}|\tau )\\ +{\theta }_1^2(z+\frac{\pi }{2}|\tau ){\theta }_4^2(z+\frac{\pi }{2}|\tau )+{\theta }_1^2(z+\frac{3\pi }{4}|\tau ){\theta }_4^2(z+\frac{3\pi }{4}|\tau )]\end{array}$$

(3.19)

$$\begin{array}{rc}=& \frac{1}{4{\theta }_2^2{\theta }_3^2}[{\theta }_2^2(z|\tau ){\theta }_3^2(z|\tau )+{\theta }_2^2(z+\frac{\pi }{4}|\tau ){\theta }_3^2(z+\frac{\pi }{4}|\tau )\\ +{\theta }_2^2(z+\frac{\pi }{2}|\tau ){\theta }_3^2(z+\frac{\pi }{2}|\tau )+{\theta }_2^2(z+\frac{3\pi }{4}|\tau ){\theta }_3^2(z+\frac{3\pi }{4}|\tau )],\end{array}$$

(3.20)

where ${\theta }_2={\theta }_2(0|\tau )$, ${\theta }_3={\theta }_3(0|\tau )$.

Taking $a=2$, $b=1$ in (1.9), (1.10) and (1.11), we have the following.

Corollary 3 For n odd, we have

(3.21)

(3.22)

(3.23)

(3.24)

where ${\mathcal{C}}_{14}(2,2;y,\tau )$ and ${\mathcal{C}}_{24}(2,2;y,\tau )$ are defined by (2.49) and (2.55), respectively.

Proof Taking $a=2$, $b=1$ and $\alpha =(1,2)$, $\beta =4$ in (1.11), we have

(3.25)

(3.26)

 □

Taking $n=1$ and letting $z\mapsto 3z$, $y\mapsto 2y$, $\tau \mapsto 3\tau$ in Corollary 3, we get the following identities for theta functions:

(3.27)

and

(3.28)

Further taking $y=0$ in the above identities, we obtain the following additive formulas for the theta function ${\theta }_4(z|\tau )$ as follows:

$$\begin{array}{rcl}{\theta }_4(3z|3\tau )& =& \frac{1}{3{\theta }_3{\theta }_2^2}[{\theta }_1^2(z|\tau ){\theta }_4(z|\tau )+{\theta }_1^2(z+\frac{\pi }{3}|\tau ){\theta }_4(z+\frac{\pi }{3}|\tau )\\ +{\theta }_1^2(z+\frac{2\pi }{3}|\tau ){\theta }_4(z+\frac{2\pi }{3}|\tau )]\end{array}$$

(3.29)

and

$$\begin{array}{rcl}{\theta }_4(3z|3\tau )& =& \frac{1}{3{\theta }_3{\theta }_1^2}[{\theta }_2^2(z|\tau ){\theta }_4(z|\tau )+{\theta }_2^2(z+\frac{\pi }{3}|\tau ){\theta }_4(z+\frac{\pi }{3}|\tau )\\ +{\theta }_2^2(z+\frac{2\pi }{3}|\tau ){\theta }_4(z+\frac{2\pi }{3}|\tau )],\end{array}$$

(3.30)

where ${\theta }_1={\theta }_1(0|\tau )$, ${\theta }_2={\theta }_2(0|\tau )$, ${\theta }_3={\theta }_3(0|\tau )$.

Corollary 4 When n is odd, we have

$$\sum _{s=0}^{kn-1}{(-1)}^s{\theta }_2^k(z+\frac{\pi s}{kn}|\tau )={\mathcal{C}}_{33}(k,0;0,\tau ){\theta }_2(knz|k{n}^2\tau ),$$

(3.31)

where ${\mathcal{C}}_{33}(k,0;0,\tau )$ is defined by (1.7).

Proof Replacing z by $knz+kn\pi +\frac{k{n}^2\pi \tau }{2}$ and τ by $k{n}^2\tau$ in (1.6), we obtain

(3.32)

Substituting n by $2n+1$ in the left-hand side of (3.32), we get (3.31). □