Abstract
In this paper, we study some coupled fixed point results in a quasi-partial metric space. Also, we introduce some examples to support the useability of our results.
MSC:47H10, 54H25.
Similar content being viewed by others
1 Introduction and preliminaries
In 1994, Matthews [1] introduced the notion of partial metric spaces and extended the Banach contraction principle from metric spaces to partial metric spaces. After that, many fixed point theorems in partial metric spaces have been given by several authors (for example, see [2–29]). Very recently, Haghi et al. [30, 31] showed in their interesting paper that some of fixed point theorems in partial metric spaces can be obtained from metric spaces.
Following Matthews [1], the notion of partial metric space is given as follows.
Definition 1.1 [1]
A partial metric on a nonempty set X is a function such that for all :
() ,
() ,
() ,
() .
A partial metric space is a pair such that X is a nonempty set and p is a partial metric on X.
Karapinar et al. [32] introduced the concept of quasi-partial metric spaces and studied some fixed point theorems on quasi-partial metric spaces.
Definition 1.2 [32]
A quasi-partial metric on a nonempty set X is a function which satisfies:
() If , then ,
() ,
() , and
()
for all .
A quasi-partial metric space is a pair such that X is a nonempty set and q is a quasi-partial metric on X.
Let q be a quasi-partial metric space on the set X. Then
is a metric on X.
Definition 1.3 [32]
Let be a quasi-partial metric space. Then:
-
(1)
A sequence converges to a point if and only if
-
(2)
A sequence is called a Cauchy sequence if and exist (and are finite).
-
(3)
The quasi-partial metric space is said to be complete if every Cauchy sequence in X converges, with respect to , to a point such that
The following lemma is crucial in our work.
Lemma 1.1 [32]
Let be a quasi-partial metric space. Then the following statements hold true:
-
(A)
If , then .
-
(B)
If , then and .
Bhaskar and Lakshmikantham [33] introduced the concept of coupled fixed point and studied some nice coupled fixed point theorems. Later, Lakshmikantham and Ćirić [34] introduced the notion of a coupled coincidence point of mappings. For some works on a coupled fixed point, we refer the reader to [35–46].
Definition 1.4 [33]
Let X be a nonempty set. We call an element a coupled fixed point of the mapping if
Definition 1.5 [34]
An element is called a coupled coincidence point of the mappings and if
Abbas et al. [47] introduced the concept of w-compatible mappings as follows.
Definition 1.6 [47]
Let X be a nonempty set. We say that the mappings and are w-compatible if whenever and .
In this paper, we study some coupled fixed point theorems in the setting of quasi-partial metric spaces. We introduce some examples to support our results.
2 The main results
We start this section with the following coupled fixed point theorem.
Theorem 2.1 Let be a quasi-partial metric space, and be two mappings. Suppose that there exist , and in with such that the condition
holds for all . Also, suppose the following hypotheses:
-
(1)
.
-
(2)
is a complete subspace of X with respect to the quasi-partial metric q.
Then the mappings F and g have a coupled coincidence point satisfying .
Moreover, if F and g are w-compatible, then F and g have a unique common fixed point of the form .
Proof Let . Since , we put and . Again, since , we put and . Continuing this process, we can construct two sequences and in X such that
and
-
Let . Then by inequality (2.1), we obtain
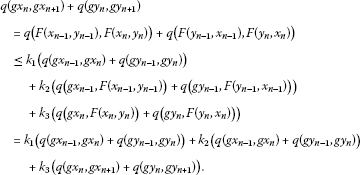 (2.2)
(2.2)
From (2.2), we have
Put . Then . Repeating (2.3) n-times, we get
Let m and n be natural numbers with . Then
Letting , we get
-
By similar arguments as above, we can show that
(2.6)
Thus the sequences and are Cauchy in . Since is complete, there are u and v in X such that and with respect to , that is,
and
From (2.5) and (2.6), we have
and
For n in ℕ, we obtain
On letting in the above inequalities and using (2.7) and (2.8), we have
Similarly, we have
-
We show that and .
For , we have
Letting in above inequalities and using (2.9)-(2.10), we get
Since , we get . By Lemma 1.1, we get and . Next, we will show that . Now, from (2.1) we have
Using (2.7) and (2.8), we obtain
Since , we have By Lemma 1.1, we get that . Finally, assume that g and F are w-compatible. Let and . Then
and
From (2.11) and (2.12), we can show that
-
We claim that and .
From (2.1), we have
Since , we conclude that . By Lemma 1.1, we get and . Therefore and . Again, since , we get . Hence F and g have a unique common coupled fixed point of the form . □
Corollary 2.1 Let be a quasi-partial metric space, and be two mappings. Suppose that there exist a, b, c, d, e, f in with such that
holds for all . Also, suppose the following hypotheses:
-
(1)
.
-
(2)
is a complete subspace of X with respect to the quasi-partial metric q.
Then F and g have a coupled coincidence point satisfying .
Moreover, if F and g are w-compatible, then F and g have a unique common fixed point of the form .
Proof Given . From (2.13), we have
and
Adding inequality (2.14) to inequality (2.15), we get
Thus, the result follows from Theorem 2.1. □
Corollary 2.2 Let be a quasi-partial metric space, let and be two mappings. Suppose that there exists with such that
holds for all . Also, suppose the following hypotheses:
-
(1)
.
-
(2)
is a complete subspace of X with respect to the quasi-partial metric q.
Then F and g have a coupled coincidence point satisfying .
Moreover, if F and g are w-compatible, then F and g have a unique common fixed point of the form .
Corollary 2.3 Let be a quasi-partial metric space, and be two mappings. Suppose that there exists with such that
holds for all . Also, suppose the following hypotheses:
-
(1)
.
-
(2)
is a complete subspace of X with respect to the quasi-partial metric q.
Then F and g have a coupled coincidence point satisfying .
Moreover, if F and g are w-compatible, then F and g have a unique common fixed point of the form .
Corollary 2.4 Let be a quasi-partial metric space, and be two mappings. Suppose that there exists with such that
holds for all . Also, suppose the following hypotheses:
-
(1)
.
-
(2)
is a complete subspace of X with respect to the quasi-partial metric q.
Then F and g have a coupled coincidence point satisfying .
Moreover, if F and g are w-compatible, then F and g have a unique common fixed point of the form .
Let (the identity mapping) in Theorem 2.2 and Corollaries 2.1-2.4. Then we have the following results.
Corollary 2.5 Let be a quasi-partial metric space and let be a mapping. Suppose that there exist with such that
holds for all .
Then F has a unique coupled fixed point of the form .
Corollary 2.6 Let be a quasi-partial metric space and let be a mapping. Suppose that there exist with such that
holds for all .
Then F has a unique coupled fixed point of the form .
Corollary 2.7 Let be a complete quasi-partial metric space and let be a mapping. Suppose that there exists such that
holds for all .
Then F has a unique coupled fixed point of the form .
Corollary 2.8 Let be a complete quasi-partial metric space and let be a mapping. Suppose that there exists with such that
holds for all .
Then F has a unique coupled fixed point of the form .
Corollary 2.9 Let be a complete quasi-partial metric space and let be a mapping. Suppose that there exists with such that
holds for all .
Then F has a unique coupled fixed point of the form .
Theorem 2.2 Let be a complete quasi-partial metric space and let , be two mappings. Suppose that there exists a function such that
holds for all . Also, assume that the following hypotheses are satisfied:
-
(a)
;
-
(b)
if , , then for each sequence , we have for some .
Then F and g have a coupled coincidence point . In addition, and .
Proof Consider . As , there are and from X such that and . By repeating this process, we construct two sequences, and with and .
The fourth property of the quasi-partial metric space gives us
Based on the above inequality, for , we obtain
Consider . Inequality (2.17) implies that
hence the nondecreasing sequence is bounded, so it is convergent. Taking the limit as in (2.16), we conclude that
Using similar arguments, it can be proved that
As and are Cauchy sequences in the complete quasi-partial metric space , there are u, v in X such that and . Having in mind hypothesis (b), the following relations hold true:
We get , and by Lemma 1.1, it follows that .
Analogously, it can be proved that .
As a conclusion, we have obtained that is a coupled coincidence point of the mappings F and g, and , . □
Corollary 2.10 Let be a complete quasi-partial metric space and let be a mapping. Suppose that there exists a function such that
holds for all . Also, assume that the following hypotheses are satisfied:
-
(a)
;
-
(b)
if , , then for each sequence , we have for some .
Then F has a coupled coincidence point . In addition, and .
Proof Follows from Theorem 2.2 by taking (the identity mapping). □
3 Examples
Now, we introduce some examples to support our results.
Example 3.1 On the set , define
Also, define
and by . Then
-
(1)
is a complete quasi-partial metric space.
-
(2)
.
-
(3)
For any , we have
Proof The proofs of (1) and (2) are clear. To prove (3), we consider the following cases.
Case 1: and . Here we have
Therefore
Case 2: and . Here we have
and . Therefore
Case 3: and . Using similar arguments to those given in Case (2), we can show that
Case 4: and . Using similar arguments to those given in Case (1), we can show that
Thus F and g satisfy all the hypotheses of Corollary 2.7. So, F and g have a unique common fixed point. Here is the unique common fixed point of F and g. □
We end with an example related to Theorem 2.2.
Example 3.2 Let . Define
Also, define
Then:
-
(1)
is a complete quasi-partial metric space.
-
(2)
.
-
(3)
For any , we have
-
(4)
Let be defined by . If and are two sequences in X with , then .
Proof The proofs of (1) and (2) are clear. To prove (3) given , , , , , and . Thus
To prove (4), let and be two sequences in X such that for some . Then and . Thus
and
Therefore
and
Therefore
Hence in . Now
So, F and g satisfy all the hypotheses of Theorem 2.2. Hence F and g have a coupled coincidence point. Here is the coupled coincidence point of F and g. □
References
Matthews SG: Partial metric topology. Ann. N. Y. Acad. Sci. 728. General Topology and Its Applications 1994, 183–197., Proc. 8th Summer Conf., Queen’s College, 1992
Abdeljawad T, Karapinar E, Taş K: Existence and uniqueness of a common fixed point on partial metric spaces. Appl. Math. Lett. 2011, 24(11):1900–1904. 10.1016/j.aml.2011.05.014
Abdeljawad T, Karapinar E, Taş K: A generalized contraction principle with control functions on partial metric spaces. Comput. Math. Appl. 2012, 63(3):716–719.
Abdeljawad T: Fixed points for generalized weakly contractive mappings in partial metric spaces. Math. Comput. Model. 2011, 54(11–12):2923–2927. 10.1016/j.mcm.2011.07.013
Altun I, Erduran A: Fixed point theorems for monotone mappings on partial metric spaces. Fixed Point Theory Appl. 2011., 2011: Article ID 508730
Altun I, Simsek H: Some fixed point theorems on dualistic partial metric spaces. J. Adv. Math. Stud. 2008, 1(1–2):1–8.
Altun I, Simsek H: Some fixed point theorems on ordered metric spaces and application. Fixed Point Theory Appl. 2010., 2010: Article ID 6214469
Altun I, Sola F, Simsek H: Generalized contractions on partial metric spaces. Topol. Appl. 2010, 157(18):2778–2785. 10.1016/j.topol.2010.08.017
Altun I, Sadarangani K: Corrigendum to “Generalized contractions on partial metric spaces” [Topology Appl. 157 (2010) 2778–2785]. Topol. Appl. 2011, 158(13):1738–1740. 10.1016/j.topol.2011.05.023
Aydi H: Some fixed point results in ordered partial metric spaces. J. Nonlinear Sci. Appl. 2011, 4(2):1–12.
Aydi H: Some coupled fixed point results on partial metric spaces. Int. J. Math. Math. Sci. 2011., 2011: Article ID 647091
Aydi H: Fixed point theorems for generalized weakly contractive condition in ordered partial metric spaces. J. Nonlinear Anal. Optim. Theory Appl. 2011, 2(2):33–48.
Aydi H, Karapinar E, Shatanawi W:Coupled fixed point results for -weakly contractive condition in ordered partial metric spaces. Comput. Math. Appl. 2011, 62: 4449–4460.
Ćirić L, Samet B, Aydi H, Vetro C: Common fixed points of generalized contractions on partial metric spaces and an application. Appl. Math. Comput. 2011, 218: 2398–2406. 10.1016/j.amc.2011.07.005
Golubović Z, Kadelburg Z, Radenović S: Coupled coincidence points of mappings in ordered partial metric spaces. Abstr. Appl. Anal. 2012., 2012: Article ID 192581
Karapınar E, Erhan I: Fixed point theorems for operators on partial metric spaces. Appl. Math. Lett. 2011, 24: 1894–1899. 10.1016/j.aml.2011.05.013
Nashine HK, Kadelburg Z, Radenović S: Common fixed point theorems for weakly isotone increasing mappings in ordered partial metric spaces. Math. Comput. Model. 2013, 57: 2355–2365. 10.1016/j.mcm.2011.12.019
Oltra S, Valero O: Banach’s fixed point theorem for partial metric spaces. Rend. Ist. Mat. Univ. Trieste 2004, 36(1–2):17–26.
Romaguera S: A Kirk type characterization of completeness for partial metric spaces. Fixed Point Theory Appl. 2010., 2010: Article ID 493298
Romaguera S: Fixed point theorems for generalized contractions on partial metric spaces. Topol. Appl. 2012, 159: 194–199. 10.1016/j.topol.2011.08.026
Samet B, Rajović M, Lazović R, Stoiljković R: Common fixed point results for nonlinear contractions in ordered partial metric spaces. Fixed Point Theory Appl. 2011., 2011: Article ID 71
Shatanawi W, Nashine HK: A generalization of Banach’s contraction principle for nonlinear contraction in a partial metric space. J. Nonlinear Sci. Appl. 2012, 5: 37–43.
Shatanawi W, Nashine HK, Tahat N: Generalization of some coupled fixed point results on partial metric spaces. Int. J. Math. Math. Sci. 2012., 2012: Article ID 686801
Shatanawi W, Samet B, Abbas M: Coupled fixed point theorems for mixed monotone mappings in ordered partial metric spaces. Math. Comput. Model. 2012, 55: 680–687. 10.1016/j.mcm.2011.08.042
Shatanawi W, Postolache M: Coincidence and fixed point results for generalized weak contractions in the sense of Berinde on partial metric spaces. Fixed Point Theory Appl. 2013., 2013: Article ID 54
Radenović S: Remarks on some coupled fixed point results in partial metric spaces. Nonlinear Funct. Anal. Appl. 2013, 18(1):39–50.
Nashine HK, Kadelburg Z, Radenović S: Fixed point theorems via various cyclic contractive conditions in partial metric spaces. Publ. Inst. Math. (Belgr.) 2013, 93(107):69–93. 10.2298/PIM1307069N
Valero O: On Banach fixed point theorems for partial metric spaces. Appl. Gen. Topol. 2005, 6(2):229–240.
Altun I, Acar Ö: Fixed point theorems for weak contractions in the sense of Berinde on partial metric spaces. Topol. Appl. 2012, 159: 2642–2648. 10.1016/j.topol.2012.04.004
Haghi RH, Rezapour Sh, Shahzad N: Be careful on partial metric fixed point results. Topol. Appl. 2013, 160: 450–454. 10.1016/j.topol.2012.11.004
Haghi RH, Rezapour S, Shahzad N: Some fixed point generalizations are not real generalizations. Nonlinear Anal. 2011, 74: 1799–1803. 10.1016/j.na.2010.10.052
Karapinar E, Erhan İ, Öztürk A: Fixed point theorems on quasi-partial metric spaces. Math. Comput. Model. 2012. doi:10.1016/j.mcm.2012.06.036
Bhaskar TG, Lakshmikantham V: Fixed point theorems in partially ordered metric spaces and applications. Nonlinear Anal. 2006, 65: 1379–1393. 10.1016/j.na.2005.10.017
Lakshmikantham V, Ćirić L: Coupled fixed point theorems for nonlinear contractions in partially ordered metric spaces. Nonlinear Anal. 2009, 70: 4341–4349. 10.1016/j.na.2008.09.020
Cho YJ, Rhoades BE, Saadati R, Samet B, Shatanawi W: Nonlinear coupled fixed point theorems in ordered generalized metric spaces with integral type. Fixed Point Theory Appl. 2012., 2012: Article ID 8
Choudhury BS, Maity P: Coupled fixed point results in generalized metric spaces. Math. Comput. Model. 2011, 54: 73–79. 10.1016/j.mcm.2011.01.036
Choudhury, BS, Metiya, N, Postolache, M: A generalized weak contraction principle with applications to coupled coincidence point problems. Fixed Point Theory Appl. (submitted)
Karapinar E: Couple fixed point theorems for nonlinear contractions in cone metric spaces. Comput. Math. Appl. 2010, 59: 3656–3668.
Samet B: Coupled fixed point theorems for a generalized Meir-Keeler contraction in partially ordered metric spaces. Nonlinear Anal. 2010, 72: 4508–4517. 10.1016/j.na.2010.02.026
Sedghi S, Altun I, Shobe N: Coupled fixed point theorems for contractions in fuzzy metric spaces. Nonlinear Anal. 2010, 72: 1298–1304. 10.1016/j.na.2009.08.018
Shatanawi W, Samet B, Abbas M: Coupled fixed point theorems for mixed monotone mappings in ordered partial metric spaces. Math. Comput. Model. 2012, 55: 680–687. 10.1016/j.mcm.2011.08.042
Shatanawi W: On w -compatible mappings and common coupled coincidence point in cone metric spaces. Appl. Math. Lett. 2012, 25: 925–931. 10.1016/j.aml.2011.10.037
Aydi H, Postolache M, Shatanawi W: Coupled fixed point results for -weakly contractive mappings in ordered G -metric spaces. Comput. Math. Appl. 2012, 63(1):298–309.
Radenović S: Remarks on some recent coupled coincidence point results in symmetric G -metric spaces. J. Operators 2013., 2013: Article ID 290525. doi:10.1155/2013/290525
Radenović S: Remarks on some coupled coincidence point result in partially ordered metric spaces. Arab J. Math. Sci. 2013. doi:10.1016/j.ajmsc.2013.02.003
Shatanawi W: Fixed point theorems for nonlinear weakly C -contractive mappings in metric spaces. Math. Comput. Model. 2011. doi:10.1016/j.mcm.2011.06.069
Abbas M, Khan MA, Radenović S: Common coupled fixed point theorems in cone metric spaces for w -compatible mapping. Appl. Math. Comput. 2010, 217(1):195–202. 10.1016/j.amc.2010.05.042
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
Both authors contributed equally and significantly in writing this article. Both authors read and approved the final manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Shatanawi, W., Pitea, A. Some coupled fixed point theorems in quasi-partial metric spaces. Fixed Point Theory Appl 2013, 153 (2013). https://doi.org/10.1186/1687-1812-2013-153
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1687-1812-2013-153