Bernstein-Jackson-type inequalities and Besov spaces associated with unbounded operators

Abstract

Besov-type interpolation spaces and appropriate Bernstein-Jackson inequalities, generated by unbounded linear operators in a Banach space, are considered. In the case of the operator of differentiation these spaces and inequalities exactly coincide with the classical ones. Inequalities are applied to a best approximation problem in a Banach space, particularly, to spectral approximations of regular elliptic operators.

MSC:47A58, 41A17.

1 Introduction and preliminaries

The classical Jackson and Bernstein inequalities express a relation between smoothness modules of functions and properties of their best approximations by polynomials or entire functions of exponential type that can be characterized with the help of Besov norms [[1], Sections 1.5, 7.2]. These results are extended to approximations of smooth functions by wavelets (see e.g. [2–4]), and to approximations of linear operators in Banach spaces by operators with finite ranks [5], and other similar approximations.

The motivation of our work is to extend the Bernstein-Jackson inequalities to cases of best spectral approximations in a Banach space. An analog of Bernstein-Jackson inequalities in the case of approximations in the space $L_p(G)$ on a Lie group G by spectral subspaces ${\mathcal{M}}_p(h)=\{f\in L_p(G):E(\lambda )f=f\text{ if }\lambda \ge h>0\}$ of the group sublaplacian ${\mathrm{\Delta }}_G$, where ${\mathrm{\Delta }}_G={\int }_0^{\mathrm{\infty }}\lambda dE(\lambda )$ is its spectral resolution, is established in [6, 7]. Spectral subspaces are analogous subspaces of entire functions of exponential type. The appropriate Besov space is characterized by the functional of best approximation $E_p(h,f)={inf}_{g\in {\mathcal{M}}_p(h)}{\parallel f-g\parallel }_{L_p(G)}$.

This approach is a prototype of our generalizations. We consider a closed operator A in a Banach space 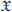instead of ${\mathrm{\Delta }}_G$ and replace the spectral subspaces ${\mathcal{M}}_p(h)$ by invariant subspaces of exponential type entire vectors of A. Note that similar subspaces of exponential type entire vectors have appeared in [8–11].

Our goal is to investigate a best approximation problem by invariant subspaces of exponential type entire vectors of an arbitrary unbounded closed linear operator A in a Banach space 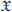. As a basic tool, we use an analog of approximate Bernstein and Jackson inequalities and an abstract quasi-normed Besov-type interpolation space ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$, associated with exponential type entire vectors of A, which sharply characterizes the behavior of the best spectral approximation.

Using the quasi-norm of ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$, the main result is formulated in Theorem 5 as two inequalities, estimating the minimal distance from a given element to a subspace of exponential type vectors with fixed indices. In the case of the operator of differentiation in $L_p(\mathbb{R})$, the spaces ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ coincide with the classical Besov-type spaces (Theorem 7) and the estimations reduce to the known Bernstein and Jackson inequalities (Theorem 8). A new application to spectral approximations of elliptic operators is shown in Section 6 (see also Theorem 6).

In a Banach complex space $(\mathfrak{X},\parallel \cdot \parallel )$ we consider a closed unbounded linear operator with the norm dense domain ${\mathcal{C}}^1(A)$,

$$A:{\mathcal{C}}^1(A)\subset \mathfrak{X}⟶\mathfrak{X}.$$

Let ${\mathcal{C}}^{k+1}(A)=\{x\in {\mathcal{C}}^k(A):A^{k}x\in {\mathcal{C}}^k(A)\}$ and ${\mathcal{C}}^{\mathrm{\infty }}(A)=\bigcap \{{\mathcal{C}}^k(A):k\in \mathbb{N}\}$. We call the elements

$$\mathcal{E}(A)=\bigcup _{t>0}\bigcap _{k\in {\mathbb{Z}}_{+}}\{x\in {\mathcal{C}}^{\mathrm{\infty }}(A):\parallel A^{k}x\parallel \le c{t}^k\}$$

exponential type entire vectors of A, where the constant $c=c(x,A)$ is independent on $k\in {\mathbb{Z}}_{+}$ and $A^0$ is the unit operator on 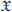. Clearly, every exponential type entire vector also is an analytic vector of A in the well-known Nelson sense.

Throughout this article we assume that the norm density condition $\overline{\mathcal{E}(A)}=\mathfrak{X}$ holds and that the operators $A^k$ ($k\in \mathbb{N}$) are closed in 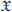. In many important cases for applications these assumptions hold. Particularly, we have the cases:

• (i) if A has a real spectrum and $\mathrm{\forall }\epsilon >0$ the integral ${\int }_0^{\epsilon }lnlnM(r)dr$ with $M(r)={sup}_{|\mathrm{\Im }\lambda |\ge r}\parallel {(\lambda -A)}^{-1}\parallel$ is convergent (see [8]);

• (ii) if A generates we have an one-parameter group $e^{tA}$ with the convergent integral ${\int }_{\mathbb{R}}\parallel e^{tA}\parallel {(1+{|t|}^2)}^{-1}dt$ [8];

• (iii) if A generates we have a bounded $C_0$-group 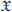$e^{tA}$ on (see [10]).

If $\mathfrak{X}=L_p(\mathbb{R})$ ($1\le p\le \mathrm{\infty }$) and $A=D$ is the differentiation operator on ℝ then $\mathcal{E}(A)$ is the space of entire functions of exponential type, belonging to $L_p(\mathbb{R})$. In this case the inequality $\parallel A^{k}x\parallel \le c{t}^k$ reduces to the Bernstein inequality. If the spectrum $\sigma (A)$ of an operator A is discrete then the subspace $\mathcal{E}(A)$ exactly coincides with the linear span of all its spectral subspaces in 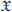(see [12]).

Recall the real interpolation method (for more details see [1, 13]). Let $(X,{|\cdot |}_X)$ and $(Y,{|\cdot |}_Y)$ be a quasi-normed complex spaces. Given the pair positive numbers $\{0<\vartheta <1,1\le p\le \mathrm{\infty }\}$ or $\{0<\vartheta \le 1,p=\mathrm{\infty }\}$ the interpolation vector space can be defined as the set ${(X,Y)}_{\vartheta ,p}=\{a\in X+Y:{|a|}_{{(X,Y)}_{\vartheta ,p}}<\mathrm{\infty }\}$ endowed with the quasi-norm

$${|a|}_{{(X,Y)}_{\vartheta ,p}}=\{\begin{array}{ll}{({\int }_0^{\mathrm{\infty }}{[{\tau }^{-\vartheta }K(\tau ,a;X,Y)]}^p\frac{d\tau }{\tau })}^{1/p},& p<\mathrm{\infty },\\ {sup}_{0<\tau <\mathrm{\infty }}{\tau }^{-\vartheta }K(\tau ,a;X,Y),& p=\mathrm{\infty },\end{array}$$

(1)

where $K(\tau ,a;X,Y):={inf}_{a=x+y}({|x|}_X+\tau {|y|}_Y)$ is called a K-functional [[1], Section 3.11]. Clearly, $X\cap Y\subset {(X,Y)}_{\vartheta ,p}\subset X+Y$.

2 Scales of invariant subspaces

Let $0<t<\mathrm{\infty }$ and $1\le p\le \mathrm{\infty }$. Consider the mapping

$${\mathcal{C}}^{\mathrm{\infty }}(A)\ni x⟶\{{(A/t)}^{k}x:k\in {\mathbb{Z}}_{+}\}$$

which image is formed by sequences of elements of a Banach space 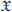. For any pair of indices t, p we define the normed spaces ${\mathcal{E}}_p^t(A)=\{x\in {\mathcal{C}}^{\mathrm{\infty }}(A):{\parallel x\parallel }_{{\mathcal{E}}_p^t}<\mathrm{\infty }\}$, where

$${\parallel x\parallel }_{{\mathcal{E}}_p^t}=\{\begin{array}{ll}{({\sum }_{k\in {\mathbb{Z}}_{+}}{\parallel {(A/t)}^{k}x\parallel }^p)}^{1/p},& 1\le p<\mathrm{\infty },\\ {sup}_{k\in {\mathbb{Z}}_{+}}\parallel {(A/t)}^{k}x\parallel ,& p=\mathrm{\infty }.\end{array}$$

Theorem 1

• (i) The contractive inclusions ${\mathcal{E}}_p^t(A)\looparrowright {\mathcal{E}}_p^{\tau }(A)\looparrowright {\mathcal{E}}_{\mathrm{\infty }}^{\tau }(A)\looparrowright \mathfrak{X}$ with $\tau >t$ hold.

• (ii) Every space ${\mathcal{E}}_p^t(A)$ is A-invariant and the restriction $A{|}_{{\mathcal{E}}_p^t}$ is a bounded operator over ${\mathcal{E}}_p^t(A)$ with the norm ${\parallel A{|}_{{\mathcal{E}}_p^t}\parallel }_{{\mathcal{E}}_p^t}\le t$.

• (iii) The spectrum of A has the property $\sigma (A{|}_{{\mathcal{E}}_p^t})\subset \sigma (A)$.

• (iv) Every space ${\mathcal{E}}_p^t(A)$ is complete.

Proof

• (i) The inequalities $\parallel x\parallel \le {\parallel x\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}$ and ${\parallel x\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}^p\le {\parallel x\parallel }_{{\mathcal{E}}_p^t}^p$ yield the contractive inclusions ${\mathcal{E}}_{\mathrm{\infty }}^t(A)\looparrowright \mathfrak{X}$ and ${\mathcal{E}}_p^t(A)\looparrowright {\mathcal{E}}_{\mathrm{\infty }}^t(A)$, respectively. If $x\in {\mathcal{E}}_p^t(A)$ then ${\parallel A^{k}x\parallel }^p\le t^{pk}{\parallel x\parallel }_{{\mathcal{E}}_p^t}^p$ and ${\parallel A^{k}x\parallel }^{p/k}\le t^p{\parallel x\parallel }_{{\mathcal{E}}_p^t}^{p/k}$ for all $k\in {\mathbb{Z}}_{+}$. It follows that ${lim\hspace{0.17em}sup}_{k\to \mathrm{\infty }}{\parallel A^{k}x\parallel }^{p/k}\le t^p$. Therefore, for any $\tau >t$ the series ${\parallel x\parallel }_{{\mathcal{E}}_p^{\tau }}^p={\sum }_k{\parallel {(A/\tau )}^{k}x\parallel }^p$ is convergent. As a result, $x\in {\mathcal{E}}_p^{\tau }(A)$. Moreover, ${\parallel x\parallel }_{{\mathcal{E}}_p^{\tau }}^p\le {\parallel x\parallel }_{{\mathcal{E}}_p^t}^p$ for all $x\in {\mathcal{E}}_p^t(A)$ and $\tau >t$.

• (ii) Using $A{(A/t)}^{k}x=t{(A/t)}^{k+1}x$, we obtain ${\parallel Ax\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}\le t{\parallel x\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}$ and ${\parallel Ax\parallel }_{{\mathcal{E}}_p^t}^p\le t^p{\parallel x\parallel }_{{\mathcal{E}}_p^t}^p$ when $1\le p<\mathrm{\infty }$.

• (iii) For any $\lambda \in \rho (A)$ and $x\in {\mathcal{E}}_p^t(A)$ the equality ${(A/t)}^k{(\lambda -A)}^{-1}x={(\lambda -A)}^{-1}{(A/t)}^{k}x$ holds. It follows that ${\parallel {(\lambda -A)}^{-1}x\parallel }_{{\mathcal{E}}_p^t}\le \parallel {(\lambda -A)}^{-1}\parallel {\parallel x\parallel }_{{\mathcal{E}}_p^t}$ for all $x\in {\mathcal{E}}_p^t$. Hence, λ belongs to the resolvent set $\rho (A{|}_{{\mathcal{E}}_p^t})$.

• (iv) Let us use the inequality ${\parallel x\parallel }_{{\mathcal{E}}_p^t}\ge \parallel {(A/t)}^{k}x\parallel$ with $x\in {\mathcal{E}}_p^t(A)$, $k\in {\mathbb{Z}}_{+}$. It follows that if ${(x_n)}_{n\in \mathbb{N}}$ is a Cauchy sequence in the space ${\mathcal{E}}_p^t(A)$ then ${(x_n)}_{n\in \mathbb{N}}$ and $\{{(A/t)}^k{x}_n:n\in \mathbb{N}\}$ are Cauchy sequences in the space 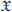for all $k\in {\mathbb{Z}}_{+}$. The completeness of 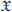implies that there exist $x,y\in \mathfrak{X}$ such that $x_n\to x$ and ${(A/t)}^k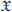{x}_n\to y$ by norm of . The graph of $A^k$ is closed in $\mathfrak{X}\times \mathfrak{X}$, therefore $y={(A/t)}^{k}x$ and $x\in {\mathcal{C}}^k(A)$. It is true for all $k\in {\mathbb{Z}}_{+}$, so $x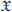\in {\mathcal{C}}^{\mathrm{\infty }}(A)$ and ${(A/t)}^k{x}_n\to {(A/t)}^{k}x$ by norm of for all $k\in {\mathbb{Z}}_{+}$.

We reason standardly: $\mathrm{\forall }\epsilon >0$ $\mathrm{\exists }{n}_{\epsilon }\in \mathbb{N}:{\parallel x_n-x_m\parallel }_{{\mathcal{E}}_p^t}<\epsilon$, $\mathrm{\forall }n,m\ge n_{\epsilon }$. It follows that $\parallel {(A/t)}^k(x_n-x_m)\parallel <\epsilon$, $\mathrm{\forall }n,m\ge n_{\epsilon }$, $k\in {\mathbb{Z}}_{+}$. So, $\mathrm{\forall }k\in {\mathbb{Z}}_{+}$ $\mathrm{\exists }{m}_{\epsilon ,k}\ge n_{\epsilon }:\parallel {(A/t)}^k(x_m-x_n)\parallel <\epsilon /2^k$ and $\parallel {(A/t)}^k(x_m-x)\parallel <\epsilon /2^k$ for $m\ge m_{\epsilon ,k}$. Hence, from $\parallel {(A/t)}^{k}x\parallel \le \parallel {(A/t)}^k{x}_{n_{\epsilon }}\parallel +\parallel {(A/t)}^k(x_m-x_{n_{\epsilon }})\parallel +\parallel {(A/t)}^k(x_m-x)\parallel$ it follows that $\parallel {(A/t)}^{k}x\parallel \le \parallel {(A/t)}^k{x}_{n_{\epsilon }}\parallel +2\epsilon /2^k$ for all $k\in {\mathbb{Z}}_{+}$. We may use the fact that the scalar sequences $a={(a_k)}_{k\in \mathbb{N}}$ with $a_k=\parallel {(A/t)}^k{x}_{n_{\epsilon }}\parallel$ and $b={(2^{-k})}_{k\in \mathbb{N}}$ belong to the Banach space ${\ell }_p$. Calculating ${\ell }_p$-norms of these elements and applying the previous inequality, we obtain

$${\parallel x\parallel }_{{\mathcal{E}}_p^t}\le {\parallel a+b\parallel }_{{\ell }_p}\le {\parallel a\parallel }_{{\ell }_p}+{\parallel b\parallel }_{{\ell }_p}={\parallel x_{n_{\epsilon }}\parallel }_{{\mathcal{E}}_p^t}+4\epsilon .$$

Hence, $x\in {\mathcal{E}}_p^t(A)$. Moreover, $\parallel {(A/t)}^k(x_n-x)\parallel \le \parallel {(A/t)}^k(x_{m_{\epsilon ,k}}-x)\parallel +\parallel {(A/t)}^k(x_n-x_{m_{\epsilon ,k}})\parallel$, where in this inequality all sequences by k belong to ${\ell }_p$. We obtain ${\parallel x_n-x\parallel }_{{\mathcal{E}}_p^t}\le 4\epsilon$, $n\ge n_{\epsilon }$. So, ${\mathcal{E}}_p^t(A)$ is complete. □

On the subspace ${\mathcal{E}}_p(A):={\bigcup }_{t>0}{\mathcal{E}}_p^t(A)$ we define the function

$${|x|}_p:=\parallel x\parallel +inf\{t>0:x\in {\mathcal{E}}_p^t(A)\}.$$

(2)

Theorem 2

• (i) For every p ($1\le p\le \mathrm{\infty }$) the embedding ${\mathcal{E}}_{\mathrm{\infty }}^t(A)\subset {\mathcal{E}}_p^{\tau }(A)$ with $\tau >t$ and the equality $\mathcal{E}(A)={\mathcal{E}}_p(A)$ hold.

• (ii) The function (2) is a quasi-norm satisfying the inequality ${|x+y|}_p\le {|x|}_p+{|y|}_p$ for all $x,y\in \mathcal{E}(A)$. Moreover, the contractive embedding ${\mathcal{E}}_p(A)\looparrowright \mathfrak{X}$ is true.

Proof (i) Let $x\in {\mathcal{E}}_{\mathrm{\infty }}^t(A)$. We reason similarly to the above. For every $k\in {\mathbb{Z}}_{+}$ we have ${\parallel A^{k}x\parallel }^p\le t^{pk}{\parallel x\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}^p$. So ${\parallel A^{k}x\parallel }^{p/k}\le t^p{\parallel x\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}^{p/k}$. It follows that ${lim\hspace{0.17em}sup}_{k\to \mathrm{\infty }}{\parallel A^{k}x\parallel }^{p/k}\le t^p$. Therefore, for every $\tau >t$ the series ${\parallel x\parallel }_{{\mathcal{E}}_p^{\tau }}^p={\sum }_k{\parallel {(A/\tau )}^{k}x\parallel }^p$ is convergent, i.e. $x\in {\mathcal{E}}_p^{\tau }(A)$. Hence, ${\mathcal{E}}_{\mathrm{\infty }}^t(A)\subset {\mathcal{E}}_p^{\tau }(A)$.

The constant c in the definition $\mathcal{E}(A)$ is independent on the index $k\in {\mathbb{Z}}_{+}$. It yields the equality ${\mathcal{E}}_{\mathrm{\infty }}^t(A)={\bigcap }_{k\in {\mathbb{Z}}_{+}}\{x\in {\mathcal{C}}^{\mathrm{\infty }}(A):\parallel A^{k}x\parallel \le c{t}^k\}$. Hence, $\mathcal{E}(A)={\bigcup }_{t>0}{\mathcal{E}}_{\mathrm{\infty }}^t(A)$. Therefore, the embedding ${\mathcal{E}}_{\mathrm{\infty }}^t(A)\subset {\mathcal{E}}_p^{\tau }(A)$ from Theorem 2(i) yields the embedding $\mathcal{E}(A)\subset {\mathcal{E}}_p(A)$ for any index p. The inverse embedding ${\mathcal{E}}_p(A)\subset \mathcal{E}(A)$ follows from Theorem 1(i).

(ii) Use that $\mathcal{E}(A)={\mathcal{E}}_p(A)$ and set $r(x)=inf\{t>0:x\in {\mathcal{E}}_p^t(A)\}$. For each $x,y\in \mathcal{E}(A)$ and $\epsilon >0$ the values ${\parallel x\parallel }_{{\mathcal{E}}_p^{r(x)+\epsilon }}$, ${\parallel y\parallel }_{{\mathcal{E}}_p^{r(y)+\epsilon }}$ are finite and the inequalities

$${\parallel x+y\parallel }_{{\mathcal{E}}_p^{r+\epsilon }}\le {\parallel x\parallel }_{{\mathcal{E}}_p^{r+\epsilon }}+{\parallel y\parallel }_{{\mathcal{E}}_p^{r+\epsilon }}\le {\parallel x\parallel }_{{\mathcal{E}}_p^{r(x)+\epsilon }}+{\parallel y\parallel }_{{\mathcal{E}}_p^{r(y)+\epsilon }}$$

with $r=max\{r(x),r(y)\}$ hold. It follows that $r(x+y)\le r+\epsilon \le r(x)+r(y)+\epsilon$. Since ε is arbitrary, $r(x+y)\le r(x)+r(y)$ for all $x,y\in \mathcal{E}(A)$. Evidently, $r(x)=r(-x)$ for all $x\in \mathcal{E}(A)$. So ${|\cdot |}_p$ is a quasi-norm. The contractility of ${\mathcal{E}}_p(A)\subset \mathfrak{X}$ is a direct consequence of (2). □

3 Besov-type scales of approximation spaces

Let $1\le p\le \mathrm{\infty }$. In what follows we denote by ${\mathcal{E}}_p(A)$ the subspace $\mathcal{E}(A)$ endowed with the quasi-norm ${|\cdot |}_p$. Consider the auxiliary functional

$$E_p(t,x)=inf\{\parallel x-x^0\parallel :x^0\in {\mathcal{E}}_p(A),{\left|x^0\right|}_p<t\},x\in \mathfrak{X}.$$

Given a pair of numbers $\{0<\alpha <\mathrm{\infty },0<\tau \le \mathrm{\infty }\}$ and $\{0\le \alpha <\mathrm{\infty },\tau =\mathrm{\infty }\}$ we consider the scale of spaces ${\mathcal{B}}_{p,\tau }^{\alpha }(A)=\{x\in \mathfrak{X}:{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}<\mathrm{\infty }\}$,

$${|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}=\{\begin{array}{ll}{({\int }_0^{\mathrm{\infty }}{[t^{\alpha }{E}_p(t,x)]}^{\tau }\frac{dt}{t})}^{1/\tau },& 0<\tau <\mathrm{\infty },\\ {sup}_{t>0}{t}^{\alpha }{E}_p(t,x),& \tau =\mathrm{\infty },\end{array}$$

where by [[1], Lemma 7.1.6] the function ${|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}$ is a quasi-norm on ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$.

We can call the space ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ endowed with the quasi-norm ${|\cdot |}_{{\mathcal{B}}_{p,\tau }^{\alpha }}$ an abstract Besov-type space, determined by an operator A. The following properties of ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ are deduced from well-known interpolation theorems.

Theorem 3 (i) If ${[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }$ is the space ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ endowed with the quasi-norm ${|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}^{\vartheta }$ with $x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A)$ then the equality

$${[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }={({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g},\vartheta =1/(\alpha +1),\tau =g\vartheta$$

(3)

(up to a quasi-norm equivalence) holds.

• (ii) The spaces ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ are complete.

• (iii) If $0<\tau <\mathrm{\infty }$, $0<\vartheta <1$, $\alpha =(1-\vartheta ){\alpha }_0+\vartheta {\alpha }_1$ with ${\alpha }_0\ne {\alpha }_1$ then

  $${({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A),{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A))}_{\vartheta ,\tau }={\mathcal{B}}_{p,\tau }^{\alpha }(A)$$

  (4)

and there exist constants $c_1$, $c_2$ such that

$${|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}\le c_1{|x|}_{{\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}}^{1-\vartheta }{|x|}_{{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}}^{\vartheta },x\in {\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A)\cap {\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A),$$

(5)

$$K(t,x;{\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0},{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1})\le c_2{t}^{\vartheta }{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }},x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A),t>0.$$

(6)

• (iv) If $0<\tau \le \varrho <\mathrm{\infty }$ then the following continuous embedding holds:

  $${\mathcal{B}}_{p,\tau }^{\alpha }(A)\looparrowright {\mathcal{B}}_{p,\varrho }^{\alpha }(A).$$

  (7)

Proof (i) The equality (3) is a direct consequence of the definition and [[1], Theorem 7.1.7].

• (ii) To prove the completeness of ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$, we equip the sum ${\mathcal{E}}_p+\mathfrak{X}$ (which is equal to 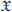, because ${\mathcal{E}}_p\subset \mathfrak{X}$) with the norm ${\parallel x\parallel }_{{\mathcal{E}}_p+\mathfrak{X}}={inf}_{x=x^0+x^1}({|x^0|}_p+\parallel x^1\parallel )$ with $x^0\in {\mathcal{E}}_p$ and $x^1\in \mathfrak{X}$. Since ${|x|}_p\ge \parallel x\parallel$, we have ${\parallel x\parallel }_{{\mathcal{E}}_p+\mathfrak{X}}=\parallel x\parallel$. Hence, the space 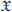with the norm ${\parallel \cdot \parallel }_{{\mathcal{E}}_p+\mathfrak{X}}$ is complete. Consequently, every series ${\sum }_{n\in \mathbb{N}}{x}_n$ with $x_n\in {({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}$ such that ${\sum }_{n\in \mathbb{N}}{\parallel x_n\parallel }_{{({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}}<\mathrm{\infty }$ is convergent to an element $x\in {\mathcal{E}}_p+\mathfrak{X}=\mathfrak{X}$. Using the inequality ${\parallel {\sum }_{n\in \mathbb{N}}{x}_n\parallel }_{{({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}}\le {\sum }_{n\in \mathbb{N}}{\parallel x_n\parallel }_{{({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}}$, we obtain $x\in {({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}$. So ${({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}$ is complete. The isomorphism (3) implies that the space ${[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }$ is complete. Thus, ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ is complete as well.

• (iii) Applying the reiteration property of the real interpolation [[1], Theorem 3.11.5] for the indices $\vartheta =(1-\eta ){\vartheta }_0+\eta {\vartheta }_1$ with ${\vartheta }_i=1/({\alpha }_i+1)$ ($i=0,1$), $\vartheta =1/(\alpha +1)$, $\tau =g\vartheta$ and $0<\eta <1$, we obtain

  $${({[{\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A)]}^{{\vartheta }_0},{[{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A)]}^{{\vartheta }_1})}_{\eta ,g}={[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }.$$

  (8)

Applying the interpolation degree property [[1], Theorem 3.11.6], we obtain

$${({[{\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A)]}^{{\vartheta }_0},{[{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A)]}^{{\vartheta }_1})}_{\eta ,g}={({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A),{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A))}_{\varrho ,\tau }^{\vartheta },\varrho =\eta {\vartheta }_1/\vartheta .$$

(9)

The equalities (8) and (9) for $\alpha =(1-\varrho ){\alpha }_0+\varrho {\alpha }_1$ yield (4) with $\varrho =\vartheta$. The inequalities (5), (6) are a consequence of (4) and the well-known interpolation properties [[1], Theorem 3.11.2].

• (iv) For every $x\in {({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A),{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A))}_{\vartheta ,\tau }$ there exists $c>0$ such that

  $$\begin{array}{rcl}{|x|}_{{({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0},{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1})}_{\vartheta ,\varrho }}& \le & {(\underset{t>0}{sup}{t}^{-\vartheta }K(t,x;\cdot ))}^{1-\tau /\varrho }{\left({\int }_0^{\mathrm{\infty }}{[t^{-\vartheta }K(t,x;\cdot )]}^{\tau }\frac{dt}{t}\right)}^{1/\varrho }\\ \le & c{|x|}_{{({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0},{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1})}_{\vartheta ,\tau }}.\end{array}$$

Hence, the embedding ${({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A),{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A))}_{\vartheta ,\tau }\looparrowright {({\mathcal{B}}_{p,{\tau }_0}^{{\alpha }_0}(A),{\mathcal{B}}_{p,{\tau }_1}^{{\alpha }_1}(A))}_{\vartheta ,\varrho }$ is continuous. Finally using (4), we obtain (7). □

Corollary 4 If $\mathcal{E}(A)$ is norm dense in 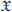then ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ is as well.

4 Bernstein-Jackson-type inequalities

Let $1\le p\le \mathrm{\infty }$ and let the space ${\mathcal{E}}_p(A)$ be endowed with the quasi-norm ${|\cdot |}_p$. Consider the problem of the approximation of a given element in a Banach space 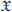by elements of an A-invariant subspace ${\mathcal{E}}_p^t(A)$ with a fixed index p. The distance between $x\in \mathfrak{X}$ and ${\mathcal{E}}_p^t(A)$ we denote by

$$d_p(t,x)=inf\{\parallel x-x^0\parallel :x^0\in {\mathcal{E}}_p^t(A)\},t>0.$$

To investigate this problem, we will use spaces ${\mathcal{B}}_{p,\tau }^{\alpha }(A)$ defined for pair indices $\{0<\alpha <\mathrm{\infty },0<\tau \le \mathrm{\infty }\}$ or $\{0\le \alpha <\mathrm{\infty },\tau =\mathrm{\infty }\}$.

Theorem 5 There are constants $c_1$ and $c_2$ such that the following inequalities hold:

$${|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}\le c_1{|x|}_p^{\alpha }\parallel x\parallel ,x\in {\mathcal{E}}_p(A),$$

(10)

$$d_p(t,x)\le c_2{t}^{-\alpha }{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }},x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A).$$

(11)

Proof Via Theorem 3(i) the space ${[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }$ is interpolating between ${\mat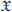hcal{E}}_p(A)$ and for any $\vartheta =1/(\alpha +1)$ and $\tau =g\vartheta$. As a consequence, ${\mathcal{E}}_p(A)\subset {[{\mathcal{B}}_{p,\tau }^{\alpha }(A)]}^{\vartheta }={({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}\subset \mathfrak{X}$. Hence, by [[1], Theorem 3.11.4(b)] for some constant $c(\vartheta ,g)$ we obtain

$${|x|}_{{({\mathcal{E}}_p,\mathfrak{X})}_{\vartheta ,g}}\le c{|x|}_p^{1-\vartheta }{\parallel x\parallel }^{\vartheta },x\in {\mathcal{E}}_p(A).$$

This inequality and the isomorphism (3) imply that there is a constant $c_1(\alpha ,\tau )$ such that the inequality (10) is true. By [[1], Theorem 3.11.4(a)] for some constant $c^{\prime }(\vartheta ,g)$ we have

$$K(t,x;{\mathcal{E}}_p(A),\mathfrak{X})\le c^{\prime }{t}^{\vartheta }{|x|}_{{({\mathcal{E}}_p,\mathfrak{X})}_{\vartheta ,g}},x\in {({\mathcal{E}}_p(A),\mathfrak{X})}_{\vartheta ,g}.$$

Hence, by virtue of the isomorphism (3) there is a constant $c_0(\alpha ,\tau )$ such that

$$K(t,x;{\mathcal{E}}_p(A),\mathfrak{X})\le c_0{t}^{\vartheta }{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}^{\vartheta },x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A).$$

Following [[1], Section 7.1], we introduce the function

$$K_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X})=\underset{x=x^0+x^1}{inf}max\{{\left|x^0\right|}_p,t\parallel x^1\parallel \},x^0\in {\mathcal{E}}_p(A),x^1\in \mathfrak{X}.$$

From the inequality $K_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X})\le K(t,x;{\mathcal{E}}_p(A),\mathfrak{X})$ it follows that

$$t^{-\vartheta }{K}_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X})\le c_0{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}^{\vartheta },x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A).$$

(12)

By [[1], Lemma 7.1.2] for every $t>0$ there exists $s>0$ such that

$$K_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X})=s,\underset{v\downarrow s}{lim}{E}_p(v,x)=E_p(s+0,x)\le s/t.$$

So, for every $s_1>0$ there is $t>0$ such that $s_1\le K_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X})=s$. For any fixed x the function $E_p(s,x)$ is decreasing, so $E_p(s,x)\le E_p(s_1+0,x)\le s_1/t$. Hence, we have ${[E_p(s,x)]}^{\vartheta }\le t^{-\vartheta }{s_1}^{\vartheta }\le t^{-\vartheta }{s}^{\vartheta -1}s$. As a result,

$$s^{1-\vartheta }{[E_p(s,x)]}^{\vartheta }\le t^{-\vartheta }{K}_{\mathrm{\infty }}(t,x;{\mathcal{E}}_p(A),\mathfrak{X}).$$

Using (12), we have $s^{1-\vartheta }{[E_p(s,x)]}^{\vartheta }\le c_0{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }}^{\vartheta }$. Putting $\alpha =(1-\vartheta )/\vartheta$, we obtain

$$s^{\alpha }{E}_p(s,x)\le c_0^{1/\vartheta }{|x|}_{{\mathcal{B}}_{p,\tau }^{\alpha }},x\in {\mathcal{B}}_{p,\tau }^{\alpha }(A).$$

(13)

If ${|x^0|}_p=r(x^0)+\parallel x^0\parallel <s$, then $r(x^0)<s-\parallel x^0\parallel$, where $r(x^0)=inf\{t>0:x^0\in {\mathcal{E}}_p^t(A)\}$. Therefore, $x^0\in {\mathcal{E}}_p^t(A)$ for all numbers $t>0$ such that $r(x^0)<t<s-\parallel x^0\parallel$. By Theorem 1(i), we have ${\mathcal{E}}_p^t(A)\subset {\mathcal{E}}_p^s(A)$. Therefore, $x^0\in {\mathcal{E}}_p^s(A)$. Hence, the inequality

$$d_p(s,x)\le E_p(s,x),x\in \mathfrak{X},s>0$$

(14)

holds. Taking $c_2=c_0^{1/\vartheta }$ in (13) and using (14), we obtain (11). □

Theorem 6 Let A be an operator with the discrete spectrum $\sigma (A)=\{{\lambda }_n\in \mathbb{C}\}$, $n\in \mathbb{N}$ and let ${\mathcal{R}}^t$ be the complex linear span of all $\{\mathcal{R}({\lambda }_n):|{\lambda }_n|<t\}$, where $\mathcal{R}({\lambda }_n)$ is the root subspace of A corresponding to ${\lambda }_n$. Then for every α, τ there is a constant c such that

$$inf\{\parallel x-x^0\parallel :x^0\in {\mathcal{R}}^t\}\le c{t}^{-\alpha }{|x|}_{{\mathcal{B}}_{1,\tau }^{\alpha }},x\in {\mathcal{B}}_{1,\tau }^{\alpha }(A).$$

(15)

Proof In [12] it is proven that for operators A, having discrete spectra, the equality ${\mathcal{E}}_1^t(A)={\mathcal{R}}^t$ holds. Hence, the inequality (11) directly implies the estimation (15) for the distance from an element $x\in {\mathcal{B}}_{1,\tau }^{\alpha }(A)$ to the spectral subspace ${\mathcal{R}}^t$. □

5 Connections with classical results

Let us put $A=D_q$, where $D_q$ is the closure in $\mathfrak{X}=L_q(\mathbb{R})$ ($1<q\le \mathrm{\infty }$) of the operator of differentiation. In the considered case we have ${\mathcal{E}}_{\mathrm{\infty }}^t(D_q)=\{u\in {\mathcal{C}}^{\mathrm{\infty }}(D_q):{\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}<\mathrm{\infty }\}$, where ${\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}={sup}_{k\in {\mathbb{Z}}_{+}}{\parallel {(D_q/t)}^{k}u\parallel }_{L_q}$ ($t>0$). Thus, ${\mathcal{E}}_{\mathrm{\infty }}(D_q)={\bigcup }_{t>0}{\mathcal{E}}_{\mathrm{\infty }}^t(D_q)$.

Consider the space ${\mathcal{M}}_q^t$ of entire complex functions $U:\mathbb{C}\ni \xi +i\eta ⟶U(\xi +i\eta )$ of exponential type $t>0$, belonging to $L_q(\mathbb{R})$ for $\eta =0$. Denote ${\mathcal{M}}_q={\bigcup }_{t>0}{\mathcal{M}}_q^t$. Following [[1], Section 7.2], we can define on ${\mathcal{M}}_q$ the quasi-norm

$${|u|}_{{\mathcal{M}}_q}={\parallel u\parallel }_{L_q}+sup\{|\zeta |:\zeta \in suppFu\},u\in {\mathcal{M}}_q,$$

where $suppFu$ is a support of the Fourier-image Fu of a function $u\in {\mathcal{M}}_q$.

For any pair $\{0<\alpha <\mathrm{\infty },0<\tau \le \mathrm{\infty }\}$ or $\{0\le \alpha <\mathrm{\infty },\tau =\mathrm{\infty }\}$ and $1<q\le \mathrm{\infty }$ we define the classical Besov space $B_{q,\tau }^{\alpha }(\mathbb{R})$ with the norm ${\parallel \cdot \parallel }_{B_{q,\tau }^{\alpha }}$ (see e.g. [[1], Section 6.2]). Let us show a relationship between the spaces ${\mathcal{B}}_{p,\tau }^{\alpha }(D_q)$ and $B_{q,\tau }^{\alpha }(\mathbb{R})$.

Theorem 7 The following isomorphism holds:

$${\mathcal{B}}_{\mathrm{\infty },\tau }^{\alpha }(D_q)=B_{q,\tau }^{\alpha }(\mathbb{R}).$$

Proof Let us denote $u(\xi )=U(\xi +i0)$ for any $U\in {\mathcal{M}}_q^t$, where $\xi \in \mathbb{R}$, $t>0$. For every such entire function u the Bernstein inequality ${\parallel D_q^{k}u\parallel }_{L_q}\le t^k{\parallel u\parallel }_{L_q}$ for all $k\in {\mathbb{Z}}_{+}$ holds (see [[1], Section 7.2]). It follows that

$${\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}=\underset{k\in {\mathbb{Z}}_{+}}{sup}{\parallel {(D_q/t)}^{k}u\parallel }_{L_q}\le {\parallel u\parallel }_{L_q}.$$

Hence, if $U\in {\mathcal{M}}_q^t$ then $u\in {\mathcal{E}}_{\mathrm{\infty }}^t(D_q)$.

Vice versa, let $u\in {\mathcal{E}}_{\mathrm{\infty }}^t(D_q)$ with a fixed $t>0$. The norm definition in ${\mathcal{E}}_{\mathrm{\infty }}^t(D_q)$ implies that ${\parallel D_q^{k}u\parallel }_{L_q}\le t^k{\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}$ for all $k\in {\mathbb{Z}}_{+}$. It follows that

$${\parallel U(\cdot +i\eta )\parallel }_{L_q}\le \sum _{k\in {\mathbb{Z}}_{+}}{\parallel D_q^{k}u\parallel }_{L_q}\frac{{|\eta |}^k}{k!}\le {\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}exp(t|\eta |),\eta \in \mathbb{R}$$

for any function $U:\mathbb{C}\ni \xi +i\eta ⟶U(\xi +i\eta )$ such that $u(\xi )=U(\xi +i0)$ for all $\xi \in \mathbb{R}$. Hence, $U(\cdot +i\eta )\in L_q(\mathbb{R})$ for all $\eta \in \mathbb{R}$. The above inequality implies that if $q=\mathrm{\infty }$ than $U(\cdot +i\eta )\in {\mathcal{M}}_{\mathrm{\infty }}^t$.

Show that $U(\cdot +i\eta )\in {\mathcal{M}}_q^t$ for $1<q<\mathrm{\infty }$. From Sobolev’s embedding theorem (see [[14], Chapter I, Section 8, Theorem 1] or [[13], Theorem 2.8.1]) we have

$${\parallel u\parallel }_{L_{\mathrm{\infty }}}\le c{\parallel u\parallel }_{W_q^1},u\in W_q^1(\mathbb{R}),1<q\le \mathrm{\infty }.$$

Consequently, ${\parallel D_q^{k}u\parallel }_{L_{\mathrm{\infty }}}\le c{\parallel D_q^{k}u\parallel }_{W_q^1}$ for all $k\in {\mathbb{Z}}_{+}$. Now using the inequality ${\parallel D_q^{k}u\parallel }_{W_q^1}^q={\parallel D_q^{k}u\parallel }_{L_q}^q+{\parallel D_q^{k+1}u\parallel }_{L_q}^q\le (1+t^q)t^{kq}{\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}^q$, we have

$${\parallel U(\cdot +i\eta )\parallel }_{L_{\mathrm{\infty }}}\le \sum _{k\in {\mathbb{Z}}_{+}}{\parallel D_q^{k}u\parallel }_{L_{\mathrm{\infty }}}\frac{{|\eta |}^k}{k!}\le c{(1+t^q)}^{1/q}exp(t|\eta |){\parallel u\parallel }_{{\mathcal{E}}_{\mathrm{\infty }}^t}$$

for all $\eta \in \mathbb{R}$. Hence, $U\in {\mathcal{M}}_q^t$ for all $1<q\le \mathrm{\infty }$. So, up to the restriction ${\mathcal{M}}_q^t\ni U⟶u\in {\mathcal{E}}_{\mathrm{\infty }}^t(D_q)$, we have

$${\mathcal{M}}_q^t={\mathcal{E}}_{\mathrm{\infty }}^t(D_q),{\mathcal{M}}_q={\mathcal{E}}_{\mathrm{\infty }}(D_q).$$

(16)

Now applying (3), (16) and the well-known interpolation properties of Besov spaces (see [[1], Theorem 7.2.4]), we obtain the required equality:

$${\mathcal{B}}_{\mathrm{\infty },\tau }^{\alpha }(D_q)={({\mathcal{E}}_{\mathrm{\infty }}(D_q),L_q(\mathbb{R}))}_{\vartheta ,r}^{1/\vartheta }={({\mathcal{M}}_q,L_q(\mathbb{R}))}_{\vartheta ,r}^{1/\vartheta }=B_{q,\tau }^{\alpha }(\mathbb{R})$$

with $\vartheta =1/(\alpha +1)$ and $\tau =r\vartheta$. □

Theorem 8 There exist constants $c_1$ and $c_2$ such that

$${\parallel u\parallel }_{B_{q,\tau }^{\alpha }}\le c_1{|u|}_{{\mathcal{M}}_q}^{\alpha }{\parallel u\parallel }_{L_q},u\in {\mathcal{M}}_q,$$

(17)

$$d_{\mathrm{\infty }}(t,u)\le c_2{t}^{-\alpha }{\parallel u\parallel }_{B_{q,\tau }^{\alpha }},u\in B_{q,\tau }^{\alpha }(\mathbb{R}),$$

(18)

where $d_{\mathrm{\infty }}(t,u)=inf\{{\parallel u-v\parallel }_{L_q}:v\in {\mathcal{E}}_{\mathrm{\infty }}^t(D_q)\}=inf\{{\parallel u-v\parallel }_{L_q}:v\in {\mathcal{M}}_q^t\}$.

Proof Using the first equality (16) and the Paley-Wiener theorem, we obtain

$$sup\{|\zeta |:\zeta \in suppFu\}=inf\{t>0:u\in {\mathcal{E}}_{\mathrm{\infty }}^t(D_q)\},u\in {\mathcal{M}}_q.$$

Hence, the quasi-norms ${|u|}_{\mathrm{\infty }}$ and ${|u|}_{{\mathcal{M}}_q}$ are equal on ${\mathcal{M}}_q$. Now the above claims is a consequence of Theorems 5, 7. □

Note that the equalities (17) and (18) exactly coincide with the well-known Bernstein and Jackson inequalities in the form given in [[1], Section 7.2].

6 An application to regular elliptic operators

Let $\mathrm{\Omega }\subset {\mathbb{R}}^n$ be an open bounded domain with the infinitely smooth boundary ∂ Ω and the system of operators

$$\begin{array}{c}(Lu)(\xi )=\sum _{|s|\le 2m}{a}_s{D}^{s}u(\xi ),a_s\in \mathbb{C},\hfill \\ (B_{j}u)(\xi )=\sum _{|s|\le m_j}{b}_{j,s}(\xi )D^{s}u(\xi ),b_{j,s}(\xi )\in C^{\mathrm{\infty }}(\partial \mathrm{\Omega }),j=1,\dots ,m\hfill \end{array}$$

is regular elliptic (see e.g. [[13], Section 5.2.1]). Denote $D^{s}u=\frac{{\partial }^{|s|}u}{\partial {\xi }_1^{s_1}\dots \partial {\xi }_n^{s_n}}$, where $\xi =({\xi }_1,\dots ,{\xi }_n)\in \mathrm{\Omega }$ and $|s|=s_1+\cdots +s_n$ for all $s=(s_1,\dots ,s_n)\in {\mathbb{Z}}_{+}^n$, $\overline{\mathrm{\Omega }}=\mathrm{\Omega }\cup \partial \mathrm{\Omega }$.

In the complex space $L_q(\mathrm{\Omega })$ ($1<q<\mathrm{\infty }$) we consider the closed linear operator

$$Au=Lu\text{ with the domain }{\mathcal{C}}^1(A)=W_{q,\{B_j\}}^{2m}(\mathrm{\Omega }),$$

(19)

where $W_{q,\{B_j\}}^{2m}(\mathrm{\Omega }):=\{u\in W_q^{2m}(\mathrm{\Omega }):B_{j}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m\}$ and $W_q^{2m}(\mathrm{\Omega })$ is the classical Sobolev space. As is well known [[13], Section 5.4.4], A has a discrete spectrum $\sigma (A)$ and the corresponding root subspaces are independent of q. The subspaces of the root vectors belong to the closed subspaces in $C^{\mathrm{\infty }}(\overline{\mathrm{\Omega }})$,

$$C_{A,\{B_j\}}^{\mathrm{\infty }}(\overline{\mathrm{\Omega }})=\{u\in C^{\mathrm{\infty }}(\overline{\mathrm{\Omega }}):B_j{A}^{k}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m,k\in {\mathbb{Z}}_{+}\},$$

endowed with the seminorms ${sup}_{\xi \in \mathrm{\Omega }}|D^{s}u(\xi )|$, $0\le |s|<\mathrm{\infty }$.

Theorem 9 The following topological isomorphism holds:

$${\mathcal{B}}_{q,\tau }^{\alpha }(A)=B_{q,\tau ,\{B_j\}}^{\alpha }(\mathrm{\Omega }),$$

(20)

where $B_{q,\tau ,\{B_j\}}^{\alpha }(\mathrm{\Omega })=\{u\in B_{q,\tau }^{\alpha }(\mathrm{\Omega }):B_j{A}^{k}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m,k\in {\mathbb{Z}}_{+}\}$ and $B_{q,\tau }^{\alpha }(\mathrm{\Omega })$ is the Besov space.

Proof Consider the space ${\mathcal{E}}_q^t(D)=\{u\in C^{\mathrm{\infty }}(\overline{\mathrm{\Omega }}):D^{s}u\in L_q(\mathrm{\Omega }),|s|=k\in {\mathbb{Z}}_{+}\}$ endowed with the norm

$${\parallel u\parallel }_{{\mathcal{E}}_q^t(D)}={\left(\sum _{k\in {\mathbb{Z}}_{+}}\sum _{|s|=k}{t}^{-qk}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}^q\right)}^{1/q},t>0.$$

Check that the union ${\mathcal{E}}_q(D)={\bigcup }_{t>0}{\mathcal{E}}_q^t(D)$ coincides with the space of all entire analytic functions of exponential type, which restrictions to Ω belong to $L_q(\mathrm{\Omega })$. The space ${\mathcal{E}}_q(D)$ we endow with the quasi-norm

$${|u|}_{{\mathcal{E}}_q(D)}:={\parallel u\parallel }_{L_q(\mathrm{\Omega })}+inf\{t>0:u\in {\mathcal{E}}_q^t(D)\}.$$

For simplicity we put $0\in \mathrm{\Omega }$. If $l>n/q$ and $u\in {\mathcal{E}}_q^t(D)$ then the Sobolev embedding theorem yields

$$\underset{\xi \in \mathrm{\Omega }}{sup}|D^{s}u(\xi )|\le cmax\{1,t,\dots ,t^l\}{t}^k{\parallel u\parallel }_{{\mathcal{E}}_q^t(D)}\le c_0{t}^k,|s|=k\in {\mathbb{Z}}_{+},$$

(21)

where the constants c, $c_0$ are independent of k. It follows that (see [10])

$$|u(\xi +i\eta )|\le \sum _{k\in {\mathbb{Z}}_{+}}\sum _{|s|=k}|D^{s}u(\xi )|\frac{{|\eta |}^k}{k!}\le c_1{e}^{t|\eta |}$$

(22)

for all $\xi \in \mathrm{\Omega }$ and $\eta \in {\mathbb{R}}^n$, where the constant $c_1$ is independent of $k\in {\mathbb{Z}}_{+}$. Hence, u has an entire analytic extension onto ${\mathbb{C}}^n$ of exponential type.

Conversely, let an entire function u satisfy (22). Then the inequality $|D^{s}u(\xi )|\le c_2{(2nt)}^k{e}^{t|\xi |}$ for all $\xi \in {\mathbb{R}}^n$ and $|s|=k\in {\mathbb{Z}}_{+}$ holds. Here the constant $c_2$ is independent of k. By boundedness of Ω we have

$$\underset{\xi \in \mathrm{\Omega }}{sup}|D^{s}u(\xi )|\le c_3{(2nt)}^k\text{and}\sum _{|s|=k}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}\le c_2{\left(2n^{2}t\right)}^k.$$

It follows that $u\in {\mathcal{E}}_q^{4n^{2}t}(D)$ and consequently $u\in {\mathcal{E}}_q(D)$, because

$$\sum _{k\in {\mathbb{Z}}_{+}}\sum _{|s|=k}{\left(4n^{2}t\right)}^{-qk}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}^q\le \frac{2^q}{2^q-1}\underset{k\in {\mathbb{Z}}_{+}}{sup}\frac{{\sum }_{|s|=k}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}^q}{{(2n^{2}t)}^{qk}}.$$

(23)

Using the inequality (21), (23), and the Paley-Wiener theorem, we obtain the quasi-norm equivalence

$${|u|}_{{\mathcal{E}}_q(D)}\sim \underset{v{|}_{\mathrm{\Omega }}=u,v\in L_q({\mathbb{R}}^n)}{inf}\{{\parallel v\parallel }_{L_q({\mathbb{R}}^n)}+\underset{\zeta \in suppFv}{sup}|\zeta |\},$$

where $suppFv$ denotes the support of the Fourier-image Fv of a function $v\in L_q({\mathbb{R}}^n)$.

Applying [[13], Theorem 4.2.2], [[1], Theorem 7.1.7] and the Bernstein-Jackson inequalities from [[1], Section 7.2], we find that for any $l\in \mathbb{N}$ there exists a constant $c_l$ such that

$$\begin{array}{r}{\parallel u\parallel }_{W_q^l(\mathrm{\Omega })}^{1/(l+1)}\le c_l{|u|}_{{\mathcal{E}}_q(D)}^{1-1/(l+1)}{\parallel u\parallel }_{L_q(\mathrm{\Omega })}^{1/(l+1)},u\in {\mathcal{E}}_q(D),\\ K(t,u;{\mathcal{E}}_q(D),L_q(\mathrm{\Omega }))\le c_l{t}^{1/(l+1)}{\parallel u\parallel }_{W_q^l(\mathrm{\Omega })}^{1/(l+1)},u\in W_q^l(\mathrm{\Omega }).\end{array}$$

(24)

Following Section 3, we define the space

$${\mathcal{B}}_{q,\tau }^{\alpha }(D):=\{u\in L_q(\mathrm{\Omega }):{|u|}_{{\mathcal{B}}_{q,\tau }^{\alpha }(D)}:={\left({\int }_0^{\mathrm{\infty }}{(t^{\alpha }{E}_q(t,u))}^{\tau }\frac{dt}{t}\right)}^{1/\tau }<\mathrm{\infty }\},$$

where $E_q(t,u)=inf\{{\parallel u-u^0\parallel }_{L_q(\mathrm{\Omega })}:u^0\in {\mathcal{E}}_q(D),{|u^0|}_{{\mathcal{E}}_q(D)}<t\}$. Using the inequality (24) and well-known theorems [[1], Theorems 3.11.5, 3.11.6, 7.1.7], [[13], Theorem 2.4.2/2], we obtain

$$\begin{array}{rcl}{\mathcal{B}}_{q,\tau }^{\alpha }(D)& =& {\left({({\mathcal{E}}_q(D),L_q(\mathrm{\Omega }))}_{1/(\alpha +1),\tau (\alpha +1)}\right)}^{\alpha +1}\\ =& {(L_q(\mathrm{\Omega }),W_q^l(\mathrm{\Omega }))}_{\alpha /l,\tau }=B_{q,\tau }^{\alpha }(\mathrm{\Omega }).\end{array}$$

(25)

Now let us prove the equality

$${\mathcal{E}}_q(A)=\{u\in {\mathcal{E}}_q(D):B_j{A}^{k}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m,k\in {\mathbb{Z}}_{+}\}.$$

(26)

By [[13], Theorem 5.4.3] for any $k\in \mathbb{N}$ there exist positive numbers c and C such that

$$c^k{\parallel u\parallel }_{W_q^{2mk}(\mathrm{\Omega })}\le {\parallel A^{k}u\parallel }_{L_q(\mathrm{\Omega })}\le C^k{\parallel u\parallel }_{W_q^{2mk}(\mathrm{\Omega })},u\in {\mathcal{C}}^k(A).$$

It follows that we have the inequalities

$$\begin{array}{rcl}\sum _{k\in {\mathbb{Z}}_{+}}{\left(C{(nt)}^{2m}\right)}^{-kq}{\parallel A^{k}u\parallel }_{L_q(\mathrm{\Omega })}^q& \le & C_1\sum _{k\in {\mathbb{Z}}_{+}}\sum _{|s|=2mk}{t}^{-2mkq}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}^q\\ \le & C_1{\parallel u\parallel }_{{\mathcal{E}}_q^t(D)}^q\end{array}$$

(27)

with a constant $C_1$. Thus, the embedding $\{u\in {\mathcal{E}}_q^t(D):B_j{A}^{k}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m,k\in {\mathbb{Z}}_{+}\}\subset {\mathcal{E}}_q^{\tau }(A)$ with $\tau =C{(nt)}^{2m}$ holds. Conversely, let $u\in {\mathcal{E}}_q^t(A)$. Then

$${\parallel u\parallel }_{{\mathcal{E}}_q^t(A)}^q=\sum _{k\in {\mathbb{Z}}_{+}}{t}^{-kq}{\parallel A^{k}u\parallel }_{L_q(\mathrm{\Omega })}^q\ge \sum _{k\in {\mathbb{Z}}_{+}}\sum _{|s|=k}{\left(c^{-1}t\right)}^{-kq}{\parallel D^{s}u\parallel }_{L_q(\mathrm{\Omega })}^q.$$

(28)

It follows that ${\mathcal{E}}_q^t(A)\subset \{u\in {\mathcal{E}}_q^{c^{-1}t}(D):B_j{A}^{k}u{|}_{\partial \mathrm{\Omega }}=0,j=1,\dots ,m,k\in {\mathbb{Z}}_{+}\}$. Using (25) and (26), we obtain the required equality (20). □

Corollary 10 There exist constants $c_1$ and $c_2$ such that

$$\begin{array}{c}{\parallel u\parallel }_{B_{q,\tau }^{\alpha }(\mathrm{\Omega })}\le c_1{|u|}_{{\mathcal{E}}_q(D)}^{\alpha }{\parallel u\parallel }_{L_q(\mathrm{\Omega })},u\in {\mathcal{E}}_q(A),\hfill \\ d_q(t,u)\le c_2{t}^{-\alpha }{\parallel u\parallel }_{B_{q,\tau }^{\alpha }(\mathrm{\Omega })},u\in B_{q,\tau ,\{B_j\}}^{\alpha }(\mathrm{\Omega }),\hfill \end{array}$$

where $d_q(t,u)=inf\{{\parallel u-v\parallel }_{L_q(\mathrm{\Omega })}:v\in {\mathcal{E}}_q^t(A)\}$. In particular, for every α, τ there is a constant c such that

$$inf\{{\parallel u-u^0\parallel }_{L_q(\mathrm{\Omega })}:u^0\in {\mathcal{R}}^t\}\le c{t}^{-\alpha }{\parallel u\parallel }_{B_{1,\tau }^{\alpha }(\mathrm{\Omega })},u\in B_{1,\tau ,\{B_j\}}^{\alpha }(\mathrm{\Omega }),$$

where ${\mathcal{R}}^t$ is the complex linear span of root subspaces $\{\mathcal{R}({\lambda }_n):|{\lambda }_n|<t\}$ of the operator (19).

Proof From the inequality (27)-(28) and the Paley-Wiener theorem it follows that we have the quasi-norm equivalence ${|u|}_{{\mathcal{E}}_q(D)}\sim {|u|}_{q_{}}$ on ${\mathcal{E}}_q(A)$. It remains to apply Theorems 5, 6, and 9. □