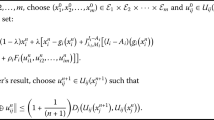Abstract
The main purpose of this paper is to introduce and study a new class of generalized nonlinear mixed ordered variational inequalities systems with ordered Lipschitz continuous mappings in ordered Banach spaces. Then, applying the matrix analysis and the vector-valued mapping fixed point analysis method, an existence theorem of solutions for this kind of the system is established. Furthermore, based on the existence theorem and the new ordered B-restricted-accretive mappings, a general algorithm for solving the systems is introduced and applied to the approximation solvability of the systems on hand. The obtained results seem to be general in nature.
MSC:49J40, 47H06.
Similar content being viewed by others
1 Introduction
Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. Let be single-valued nonlinear ordered compression mappings, and let be a Lipschitz continuous mapping (), and for any ,
we consider the following problem:
For , find such that
which is called a generalized nonlinear mixed ordered variational inequality system (GNM ordered variational inequality system) with ordered Lipschitz continuous mappings in an ordered Banach space. Obviously, system (1.1) belongs to a new class of generalized nonlinear mixed ordered variational inequality systems with the ⊕ calculation.
For a suitable choice of the mappings u, v, f, g, () and the space X, a number of known classes of ordered variational inequalities, which have been studied by the authors as special cases of system (1.1) in the Banach space (see [1, 2]).
Remark 1.1 Some special cases of system (1.1):
-
(1)
Let be zero operators, and for any , then system (1.1) becomes the following problem: Find such that
(1.2)which is called a generalized nonlinear ordered variational inequality (a generalized nonlinear ordered equation, as changed ≥ to =) in an ordered Banach space (see [1]).
-
(2)
Let be zero operators, , and for any , then system (1.1) becomes the following problem: Find such that
(1.3)which is called a new class of general nonlinear ordered variational inequality (a general nonlinear ordered equation, as changed ≥ to =) in an ordered Banach space (see [2]).
-
(3)
Let be zero operators and , then system (1.1) becomes the following problem: Find such that
(1.4)which is studied by many authors in a Banach space (see [3]et al.).
In recent years, though we have succeeded in the area of studies of variational inequality (inclusion) systems, yet, the studies of ordered variational inequality (inclusion) systems are beginning in very recent research works on an ordered Banach space (see [1, 2, 4–9]). From 1999 till present, some new and interesting problems for systems of variational inequalities (inclusions) have been introduced and studied in this field (see [1–32]).
Very recently, the approximation solution for general nonlinear ordered variational inequalities and ordered equations [1, 2] and a nonlinear ordered inclusion problem [8, 9] have been studied by Li in an ordered Banach space. For details, we refer the reader to [1–32] and the references therein.
2 Preliminaries
We need to recall the following concepts and results for solving system (1.1).
Definition 2.1 [21]
Let X be a real ordered Banach space with a norm , a normal cone P and a partial ordered relation ≤ defined by the cone P, for , if (or ) holds, then x and y are said to be a comparison between each other (denoted by for and ).
Lemma 2.2 [1]
Let X be a real ordered Banach space with a norm , a normal cone P and a partial ordered relation ≤ defined by the cone P, for arbitrary , and express the least upper bound of the set and the greatest lower bound of the set on the partial ordered relation ≤, respectively. Suppose that and exist, some binary operators can be defined as follows:
-
(1)
;
-
(2)
;
-
(3)
.
∨, ∧, and ⊕ are called OR, AND, and XOR operations, respectively. For arbitrary , the following relations hold:
-
(1)
;
-
(2)
;
-
(3)
;
-
(4)
let λ be real, then ;
-
(5)
if x, y, and w can be compared with each other, then
-
(6)
let exist, and if and , then
-
(7)
if x, y, z, w can be compared with each other, then
-
(8)
, if .
Lemma 2.3 [5]
If , then , and exist, , and .
Lemma 2.4 [5]
If for any natural number n, , and (), then .
Lemma 2.5 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. is comparative, then for , if [1], then
-
(1)
,
-
(2)
,
-
(3)
if and only if .
Proof Result (1) is obvious; (2) follows from (1), Definition 2.2 in [1], Lemma 2.7 in [2]; (3) follows from (1) and (2). This completes the proof. □
Definition 2.6 Let X be a real ordered Banach space, and let be a mapping. The operator is said to be an ordered Lipschitz continuous with constants if , , then , and there exist constants such that
Definition 2.7 [2]
Let X be a real ordered Banach space, let be a mapping, and let I be an identity mapping on X. A mapping is said to be a B-restricted-accretive mapping if A, B and all are comparisons, and they are comparisons with each other, and there exist two constants such that for arbitrary ,
holds, where I is an identity mapping on X.
Definition 2.8 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. If is a product Banach space with the normal and an ordered relation ≤, and the following conditions are satisfied:
-
(1)
for any ;
-
(2)
if and only if , , and if and only if , in X;
-
(3)
Then is called an ordered product Banach space.
Definition 2.9 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. Let be an ordered product Banach space. For a vector-valued mapping in , if there exists a point such that
then is called a fixed point of vector-valued mapping in ordered product Banach space.
The following results are obvious.
Lemma 2.10 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. Let be an ordered product Banach space. For sequences and in X, in ,
Lemma 2.11 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P. Let be an ordered product Banach space. Let be a vector-valued mapping in if for any , , and there exist a constance such that
then has a fixed point in .
Proof This directly follows from Lemma 2.2, Lemma 2.5(2) and the contraction mapping principle. □
3 Approximation solution for GNM system (1.1)
In this section, we will change from the solution of system (1.1) to finding a fixed point for a vector-valued mapping, and by using the vector-valued mapping fixed point analysis method, show the convergence of the approximation sequences of the solution for system (1.1) in an ordered product Banach space.
Lemma 3.1 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P, and let be an ordered product Banach space. If are ordered compressions, is order -Lipschitz continuous, and let g, f, , and be comparison mappings with each other (where ). Then system (1.1) has a solution if and only if there exist two ordered compressions and such that the vector-valued mapping ,
has the fixed point in an ordered Banach space .
Proof Let be a fixed point of the vector-valued mapping (3.1), then, obviously, is a solution of system (1.1).
On the other hand, choosing
and
where , if is a solution of system (1.1), then by using [1, 2],
hold. Therefore, is a fixed point of the vector-valued mapping (3.1), where the mappings and are ordered compressions [2]. This completes the proof. □
Theorem 3.2 Let X be a real ordered Banach space with a norm , a zero θ, a normal cone P, a normal constance N of P and a partial ordered relation ≤ defined by the cone P, and let be an ordered product Banach space. Let g and f be ordered compressions with respect to and , respectively; let be an ordered compression mapping with , let be an ordered -Lipschitz continuous (), and let g, f, , and () be comparison mappings with each other. If is a -restricted-accretive mapping with , and is a -restricted-accretive mapping with , and
holds, then for the general nonlinear mixed ordered variational inequality system (1.1), there exists a solution .
Proof Let X be a real ordered Banach space, and let be an ordered product Banach space. Setting
Since is a -restricted-accretive mapping with , is a -restricted-accretive mapping with , and and are ordered -Lipschitz continuous and -Lipschitz continuous, respectively, then for any given and (), by Lemma 2.2(6), (7), Definition 2.7 and [1, 2], we can obtain the following inequalities:
and
and
where
Further, by [27] and Definition 2.2 in [1], we have
where
and N is a normal constant of P.
It follows from (3.8) and the assumption condition (3.2) that , and hence the vector-valued mapping
has a fixed point for Lemma 2.11, in an ordered Banach space , which is a solution for system (1.1) by Lemma 3.1. This completes the proof. □
Theorem 3.3 Let the assumption conditions in Theorem 3.2 and (3.2) hold, that is,
Then the iterative sequence generated by the following algorithm:
for any , , and , converges strongly to , which is a solution of system (1.1).
Proof Let the assumption conditions in Theorem 3.2 hold. For any given and , , setting
then for any , by algorithm (3.10), (3.4) and (3.5), we have
and
combining (3.12) and (3.13), and by Definition 2.8, we have
where
Since (3.2) holds, that is,
the inequality is true. It follows that strongly from Lemma 2.11.
Since g, f, , and () are ordered compressions, and they are comparisons of each other, so that
holds. Therefore, is a fixed point of the vector-valued mapping
By using Lemma 3.1, is a solution of system (1.1). This completes the proof. □
Remark 3.4 For a suitable choice of the mappings g, f, , and (), we can obtain several known results [1] and [2] as special cases of Theorem 3.2, 3.3.
References
Li HG: Approximation solution for general nonlinear ordered variational inequalities and ordered equations in ordered Banach space. Nonlinear Anal. Forum 2008, 13(2):205–214.
Li HG: Approximation solution for a new class of general nonlinear ordered variational inequalities and ordered equations in ordered Banach space. Nonlinear Anal. Forum 2009, 14: 1–9.
Verma RU: Projection methods, algorithms and a new system of nonlinear variational inequalities. Comput. Math. Appl. 2001, 41: 1025–1031. 10.1016/S0898-1221(00)00336-9
Amann H: On the number of solutions of nonlinear equations in ordered Banach space. J. Funct. Anal. 1972, 11: 346–384. 10.1016/0022-1236(72)90074-2
Du YH: Fixed points of increasing operators in ordered Banach spaces and applications. Appl. Anal. 1990, 38: 1–20. 10.1080/00036819008839957
Ge DJ, Lakshmikantham V: Couple fixed points of nonlinear operators with applications. Nonlinear Anal. TMA 1987, 11: 623–632. 10.1016/0362-546X(87)90077-0
Ge DJ: Fixed points of mixed monotone operators with applications. Appl. Anal. 1988, 31: 215–224. 10.1080/00036818808839825
Li HG: Nonlinear inclusion problems for ordered RME set-valued mappings in ordered Hilbert spaces. Nonlinear Funct. Anal. Appl. 2011, 16(1):1–8.
Li HG:Nonlinear inclusion problem involving - set-valued mappings in ordered Hilbert space. Appl. Math. Lett. 2012, 25: 1384–1388. 10.1016/j.aml.2011.12.007
Ansari QH, Yao JC: A fixed point theorem and its applications to a system of variational inequalities. Bull. Aust. Math. Soc. 1999, 59: 433–442. 10.1017/S0004972700033116
Ansari QH, Schaible S, Yao JC: Systems of vector equilibrium problems and its applications. J. Optim. Theory Appl. 2000, 107: 547–557. 10.1023/A:1026495115191
Cho YJ, Fang YP, Huang NJ: Algorithms for systems of nonlinear variational inequalities. J. Korean Math. Soc. 2004, 41: 489–499.
Fang YP, Huang NJ: H -Monotone operators and system of variational inclusions. Commun. Appl. Nonlinear Anal. 2004, 11(1):93–101.
Yan WY, Fang YP, Huang NJ: A new system of set-valued variational inclusions with H -monotone operators. Math. Inequal. Appl. 2005, 8(3):537–546.
Fang YP, Huang NJ, Thompson HB:A new system of variational inclusions with -monotone operators in Hilbert spaces. Comput. Math. Appl. 2005, 49: 365–374. 10.1016/j.camwa.2004.04.037
Lan HY, Kang JI, Cho YJ:Nonlinear -monotone operator inclusion systems involving non-monotone set-valued mappings. Taiwan. J. Math. 2007, 11: 683–701.
Peng JW, Zhu DL:Three-step iterative algorithm for a system of set-valued variational inclusions with -monotone operators. Nonlinear Anal. 2008, 68: 139–153. 10.1016/j.na.2006.10.037
Li HG, Xu AJ, Jin MM:A hybrid proximal point three-step algorithm for nonlinear set-valued quasi-variational inclusions system involving -accretive mappings. Fixed Point Theory Appl. 2010., 2010: Article ID 635382 10.1155/2010/635382
Lan HY, Cho YJ, Verma RU:On nonlinear relaxed cocoercive inclusions involving -accretive mappings in Banach spaces. Comput. Math. Appl. 2006, 51: 1529–1538. 10.1016/j.camwa.2005.11.036
Li HG:Approximation solutions for generalized multi-valued variational-like inclusions with -monotone mappings. J. Jishou Univ., Nat. Sci. Ed. 2009, 30(4):7–12.
Schaefer HH: Banach Lattices and Positive Operators. Springer, Berlin; 1974.
Li HG, Xu AJ, Jin MM:An Ishikawa-hybrid proximal point algorithm for nonlinear set-valued inclusions problem based on -accretive framework. Fixed Point Theory Appl. 2010., 2010: Article ID 501293 10.1155/2010/501293
Pan XB, Li HG, Xu AJ: The over-relaxed A -proximal point algorithm for general nonlinear mixed set-valued inclusion framework. Fixed Point Theory Appl. 2011., 2011: Article ID 840978 10.1155/2011/840978
Li H-G, Xu A-J: A new class of generalized nonlinear random set-valued quasi-variational inclusion system with random nonlinear -accretive mappings in q -uniformly smooth Banach spaces. Nonlinear Anal. Forum 2010, 15: 1–20.
Shim SH, Kang SM, Huang NJ, Yao JC: Perturbed iterative algorithms with errors for completely generalized strongly nonlinear implicit variational-like inclusions. J. Inequal. Appl. 2000, 5(4):381–395.
Verma RU: A -Monotonicity and applications to nonlinear variational inclusions. J. Appl. Math. Stoch. Anal. 2004, 17(2):193–195.
Horn RA, Johnson CR: Matrix Analysis. Cambridge University Press, Cambridge; 1986.
Li HG:Approximation solutions for generalized multi-valued variational-like inclusions with -monotone mappings. J. Jishou Univ., Nat. Sci. Ed. 2009, 30(4):7–12.
Alimohammady M, Balooee J, Cho YJ, Roohi M: Iterative algorithms for a new class of extended general nonconvex set-valued variational inequalities. Nonlinear Anal. 2010, 73: 3907–3923. 10.1016/j.na.2010.08.022
Alimohammady M, Balooee J, Cho YJ, Roohi M: New perturbed finite step iterative algorithms for a system of extended generalized nonlinear mixed-quasi variational inclusions. Comput. Math. Appl. 2010, 60: 2953–2970. 10.1016/j.camwa.2010.09.055
Yao Y, Cho YJ, Liou Y: Iterative algorithms for variational inclusions, mixed equilibrium problems and fixed point problems approach to optimization problems. Cent. Eur. J. Math. 2011, 9: 640–656. 10.2478/s11533-011-0021-3
Yao Y, Cho YJ, Liou Y: Algorithms of common solutions for variational inclusions, mixed equilibrium problems and fixed point problems. Eur. J. Oper. Res. 2011, 212: 242–250. 10.1016/j.ejor.2011.01.042
Acknowledgements
This work was supported by the National Natural Science Foundation of China (Grant no. 11201512) and the Natural Science Foundation Project of CQ CSTC (cstc2012jjA00001).
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
All authors read and approved the final manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License (https://creativecommons.org/licenses/by/2.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Li, HG., Qiu, D. & Jin, M. GNM ordered variational inequality system with ordered Lipschitz continuous mappings in an ordered Banach space. J Inequal Appl 2013, 514 (2013). https://doi.org/10.1186/1029-242X-2013-514
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1029-242X-2013-514