Application of a thermo-elastoplastic constitutive model for numerical modeling of thermal triaxial tests on saturated clays

Abstract

In the present study, the behavior of saturated clays while they are mechanically loaded under constant temperatures has been investigated by simulating thermal triaxial test. A critical state-based thermo-mechanical constitutive model has been added to the ABAQUS finite element (FE) software through a user subroutine to define the stress–strain, volume change and pore pressure behavior of saturated clays. In order to have better predictions by the numerical implemented model, it has been changed by defining a new plastic potential function similar to its yield surface. The model has been integrated via an explicit integration scheme. Verification of the discretized axisymmetric numerical model has been carried out by three different series of triaxial tests for both drained and undrained conditions, and the experimental results were compared with numerical simulations. According to these comparisons, the adopted numerical model is able to predict the results of thermal triaxial tests on saturated normally consolidated and overconsolidated clays in a good manner. The presented methodology is general and can be used for application of any other critical state-based thermal constitutive model in a FE program. Based on the results, it can be suggested to be implemented in similar thermal boundary value problems of geotechnical engineering.

Appendix

Constitutive model parameters

As it was pointed out in the text, additional parameters of the model are discussed here. The slopes of NCL and URL are represented by λ and κ, respectively. Also, C shows how κ is affected by temperature variations and is calculated through Eq. (18).

$$\left( {\frac{{\kappa_{T} }}{{\kappa_{0} }} - 1} \right) = C\ln \left( {\frac{T}{{T_{0} }}} \right)$$

(18)

The slope of critical state line in q-$p^{\prime}$ space (${\rm M}_{T}$) is possible to be considered as temperature dependent or independent based on the following group of equations according to ξ values.

$$\begin{aligned} M_{T} & = M - \xi \left( {\frac{T}{{T_{0} }}} \right) \\ M_{T} & = M + \xi \left( {\frac{T}{{T_{0} }}} \right) \\ M_{T} & = M \\ \end{aligned}$$

(19)

The shear modulus $G_{T}$ is considered temperature-dependent by applying D, which is the slope of linear regression line given by Eq. (20).

$$\left( {\frac{{G_{T} }}{{G_{0} }}} \right) = 1 + D\ln \left( {\frac{T}{{T_{0} }}} \right)$$

(20)

Modification of the void ratio in relation to temperature changes is taken into account by $\chi$ which is introduced by Eq. (21).

$$\ln \left( {1 - \frac{{\Delta e_{T} }}{{\Delta e_{\text{max} } }}} \right) = \chi \left( {1 - \frac{T}{{T_{0} }}} \right)$$

(21)

Derivation of the model equations

The elastic stiffness matrix $\left[ {D^{el} } \right]$ is considered based on Eq. (22).

$$\left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {K + 4G/3} \\ {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} {K + 4G/3} \\ {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} {K - 2G/3} \\ {\begin{array}{*{20}c} {K + 4G/3} \\ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} G \\ {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} G \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} 0 \\ G \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \right]$$

(22)

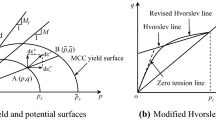
The hardening parameter in the yield surface is a function of plastic volumetric strain. Utilizing the chain rule, derivation of the yield surface with respect to volumetric strain is in the form of Eqs. (23)–(26).

$$H = \frac{\partial f}{{\partial p_{c}^{ '} }}\frac{{\partial p_{c}^{ '} }}{{\partial \varepsilon_{v}^{P} }}\frac{\partial g}{{\partial p^{\prime}}}$$

(23)

$$\frac{\partial f}{{\partial p_{c}^{\prime } }} = - M_{T}^{2} p^{\prime 2} \frac{\beta \left( T \right) - 1}{2\theta - 1}\left[ {\frac{1}{\varOmega }\left( {\frac{{p_{C}^{\prime } }}{{p^{\prime } }}} \right)^{{\frac{1}{\varOmega } - 1}} \left( {\frac{1}{{p^{\prime } }}} \right)} \right]$$

(24)

$$\frac{\partial g}{{\partial p^{\prime}}} = - M_{T}^{2} \frac{{\alpha \left( T \right)^{2} }}{2\theta - 1}\left[ { - \frac{1}{\varOmega }\left( {\frac{{p^{\prime}_{C} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega } - 1}} \left( {p_{c}^{'} } \right) + 2p^{\prime}\left[ {\left( {\frac{{p_{c}^{'} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega }}} - 1} \right]} \right]$$

(25)

$$\left\{ {\begin{array}{*{20}c} {{\text{Increase of }}\psi {\text{ with temperature}} \to \theta = 1 - \omega } \\ {{\text{Independency of }}\psi {\text{ to temperature}} \to \theta = 1} \\ {{\text{Decrease of }}\psi {\text{ with temperature}} \to \theta = 1 + \omega } \\ \end{array} } \right.$$

(26)

In Eq. (26), $\psi$ can be defined as the ratio of plastic volumetric strain ($d\varepsilon_{v}^{p}$) to the plastic shear strain ($d\varepsilon_{s}^{p}$) or $\psi = {{d\varepsilon_{v}^{p} } \mathord{\left/ {\vphantom {{d\varepsilon_{v}^{p} } {d\varepsilon_{s}^{p} }}} \right. \kern-0pt} {d\varepsilon_{s}^{p} }}$. Variations of hardening parameter with respect to plastic volumetric strain can be determined based on Eq. (10).

$$\frac{{\partial p_{c}^{ '} }}{{\partial \varepsilon_{v}^{P} }} = \frac{{\lambda - \kappa_{T} }}{1 + e}\frac{1}{{p_{c}^{'} }} - \frac{{n\Delta e_{T} }}{1 + e}\frac{{M_{T} }}{{M_{T} - \eta }}\frac{1}{{p_{c}^{'} }}$$

(27)

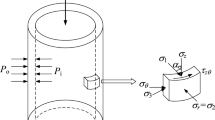
The mean effective stress ($p^{\prime}$) and deviatoric stress ($q$) are defined based on Eqs. (28) and (29), respectively.

$$p^{\prime} = \frac{{(\sigma_{1}^{'} + \sigma_{2}^{'} + \sigma_{3}^{'} )}}{3}$$

(28)

$$q = \left[ {\frac{{(\sigma_{1}^{'} - \sigma_{2}^{'} )^{2} + (\sigma_{2}^{'} - \sigma_{3}^{'} )^{2} + (\sigma_{3}^{'} - \sigma_{1}^{'} )^{2} }}{2}} \right]^{{\frac{1}{2}}}$$

(29)

Deviations of the yield and plastic potential surfaces with respect to principal stresses can be determined based on Eqs. (30)–(41).

$$\frac{\partial f}{\partial \sigma } = \frac{\partial f}{{\partial p^{\prime}}}\frac{{\partial p^{\prime}}}{\partial \sigma } + \frac{\partial f}{\partial q}\frac{\partial q}{\partial \sigma }$$

(30)

$$\frac{\partial f}{\partial q} = 2q$$

(31)

$$\frac{\partial f}{{\partial p^{\prime}}} = - {\rm M}_{T}^{2} \frac{\beta \left( T \right) - 1}{2\theta - 1}\left[ { - \frac{1}{\varOmega }\left( {\frac{{p^{\prime}_{c} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega } - 1}} \left( {p^{\prime}_{c} } \right) + 2p^{\prime}\left[ {\left( {\frac{{p^{\prime}_{c} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega } - 1}} - 1} \right]} \right]$$

(32)

$$\frac{\partial f}{{\partial \sigma_{2} }} = \frac{1}{3}\frac{\partial f}{\partial p} + 3\left( {\sigma_{y} - p} \right)$$

(33)

$$\frac{\partial f}{{\partial \sigma_{3} }} = \frac{1}{3}\frac{\partial f}{\partial p} + 3\left( {\sigma_{z} - p} \right)$$

(34)

$$\frac{\partial f}{{\partial \sigma_{3} }} = \frac{1}{3}\frac{\partial f}{\partial p} + 3\left( {\sigma_{z} - p} \right)$$

(35)

$$\frac{\partial g}{\partial \sigma } = \frac{\partial g}{{\partial p^{\prime}}}\frac{{\partial p^{\prime}}}{\partial \sigma } + \frac{\partial g}{\partial q}\frac{\partial q}{\partial \sigma }$$

(36)

$$\frac{\partial g}{\partial q} = 2q$$

(37)

$$\frac{\partial g}{{\partial p^{\prime}}} = - {\rm M}_{T}^{2} \frac{\alpha \left( T \right) - 1}{2\theta - 1}\left[ { - \frac{1}{\varOmega }\left( {\frac{{p^{\prime}_{c} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega } - 1}} \left( {p^{\prime}_{c} } \right) + 2p^{\prime}\left[ {\left( {\frac{{p^{\prime}_{c} }}{{p^{\prime}}}} \right)^{{\frac{1}{\varOmega } - 1}} - 1} \right]} \right]$$

(38)

$$\frac{\partial g}{{\partial \sigma_{1} }} = \frac{1}{3}\frac{\partial g}{\partial p} + 3\left( {\sigma_{x} - p} \right)$$

(39)

$$\frac{\partial g}{{\partial \sigma_{2} }} = \frac{1}{3}\frac{\partial g}{\partial p} + 3\left( {\sigma_{y} - p} \right)$$

(40)

$$\frac{\partial g}{{\partial \sigma_{3} }} = \frac{1}{3}\frac{\partial g}{\partial p} + 3\left( {\sigma_{z} - p} \right)$$

(41)