RETRACTED ARTICLE: Extentions of neutrosophic cubic sets via complex fuzzy sets with application

Abstract

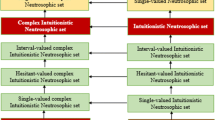
In this paper, we propose that the complex neutrosophic cubic set (internal and external) show, which is a blend of complex fuzzy sets, neutrosophic sets, and cubic sets. We characterize a few set theoretic activities of internal complex neutrosophic sets, for example, union, intersection and complement, and a while later the operational principles. A few ideas identified with the structure of this model are clarified. We present some accumulation administrators and talk about some basic leadership issue with genuine model.

Introduction

Introduction consists of three subsections as by:

Fuzzy sets and its different versions

In 1965 Zadeh [1] first introduced the fuzzy set (FS) theory. After that [2, 3] Atanassov proposed the intuitionstic fuzzy set (IFS). Atanassov included a non-participation work in intuitionistic fuzzy set to diminish the weakness in which the fuzzy set has just enrollment work. Smarandache [4] in 1999 define the theme of ņeutrosophic sets (NS). In ņeutrosophic sets (NS), Smarandache added indeterminacy-membership function, i.e. NS is composed of (truth $truth(l_{11}),$ indeterminacy $in\det er\min acy(l_{11})$ and falsity-membership $False(l_{11}).$ Moreover, the ņeutrosophic sets (NS) are the combination of fuzzy sets (FSs) and intuitionstic fuzzy set (IFSs). The idea of single valued ņeutrosophic sets is given by Wang et al. [6]. Yet, in many real-life problems, the degrees of truth, falsehood, and indeterminacy of a certain statement may be suitably presented by interval forms, instead of real numbers [7]. Multi-criteria basic leadership strategy which depends on a cross-entropy with interim ņeutrosophic sets talked about by Tian et al. [8]. Furthermore, Jun et al. [9] proposed the concept of ņeutrosophic cubic set (NCS) by adding (truth $truth(l_{11}),$ indeterminacy $in\det er\min acy(l_{11})$ and falsity-membership $False(l_{11})$ and neutrosophic set and (truth $truth(l_{11}),$ indeterminacy $in\det er\min acy(l_{11})$ and falsity-membership $False(l_{11})$ and neutrosophic set. Neutrosophic cubic sets (NCSs) which are the generalized form of fuzzy sets, cubic sets and ņeutrosophic sets. Different researchers used the fuzzy sets and extended version such as neutrosophic set, single-valued neutrosophic sets neutrosophic soft sets and neutrosophic refined sets in decision making problems with the help of aggregation operators for detail see [10,11,12,13,14,15].

Complex fuzzy sets and its different versions

Buckly [16] for the first time gave the concept of fuzzy complex numbers, see also [17,18,19]. In 2002 the Ramot et al. [20] generalized the concept of fuzzy set and introduced the notions of complex fuzzy set. In contrast, Ramot et al. [21] displayed an imaginative idea that is entirely unexpected from different analysts, in which the researcher expanded the scope of participation capacity to the unit circle in the complex plane, different from the idea of other researchers. Moreover to leads a unique collaboration, or dependency, between rules, which is improved by the use of vector aggregation in the inference stage of complex fuzzy logic sets. These problems may be very hard or difficult to solve using old techniques of fuzzy logic. There are numerous specialists which have dealt with complex fuzzy set, for example, Nguyen et al. [22] and Zhang et al. [23]. Abd Uazeez et al. [24], added the non-membership term to the idea of complex fuzzy set which is known as complex intuitionistic fuzzy sets, the range of values are extended to the unit circle in complex plan for both membership and non-membership functions instead of [0, 1]. The concept of complex intuitionistic fuzzy set introduced by Salleh [25, 26], which are the generalized form of complex fuzzy set. By the use of complex fuzzy sets different developing systems utilized by neutrosophic sets in present time for better designing and modeling real-life problems. To overcome the information of periodicity and uncertainty at the same time which is related to ‘complex’ functionality. Naveed at al. [27] examined the uses of complex intuitionistic fuzzy charts in cell organize supplier organizations. Additionally observe the possibility of complex intuitionistic fuzzy charts by Naveed and Akram [28].

In recent times, Ali and Smarandache [29] introduced complex neutrosophic set, which complex neutrosophic set is a neutrosophic set whose complex-valued truth membership function, complex-valued indeterminacy membership function, and complex-valued falsehood membership functions are the combination of real-valued truth amplitude term in association with phase term, real-valued indeterminate amplitude term with phase term, and real-valued false amplitude term with phase term, respectively. The complex ņeutrosophic set is a general structure of the various existing models, see [30, 31].

Our approach

In this paper, being motivated from the idea of complex fuzzy sets which sums up the fuzzy sets, we propose the complex ņeutrosophic cubic sets (internal and external), which is a mix of complex fuzzy sets, neutrosophic sets and cubic sets. We characterize a few set theoretic activities of complex ņeutrosophic cubic sets (CNSs), for example, union, intersection and complement, and later the distinctive operational laws. Likewise disclosed a few ideas identified with the structure of this model. We present some collection administrators and talk about some basic leadership issues with genuine precedent.

Preliminaries

In this segment we gathered a portion of the helping material from the current writing.

Definition 1

[4, 5] Let L be a non-empty set. A neutrsophic set in L is a structure of the form $\mathfrak {R}_{1}:=\{l_{11};\mathfrak {R}_{1truth}(l_{11}),\mathfrak {R}_{1In\det er}(l_{11}),\mathfrak {R}_{1False}(l_{11})|l_{11}\in L\},$ is described by truth, $In\det ermacy$ and False, where $\mathfrak {R}_{1truth},\mathfrak {R}_{1In\det er},\mathfrak {R}_{1False}:L\rightarrow \left] 0^{-},1^{+}\right[ .$

Definition 2

[6] Let L be a universe of discourse, with a general element in L denoted by $l_{11}$. A single valued ņeutrosophic set $\mathfrak {R}_{1}$ in L is defined as follows:

$$\begin{aligned} \mathfrak {R}_{1}=\left\{ l_{11}:(\mathfrak {R}_{1truth}(l_{11}),\mathfrak {R}_{1In\det er}(l_{11}),\mathfrak {R}_{1F}(l_{11}))|l_{11}\in L \right\} , \end{aligned}$$

where $\mathfrak {R}_{1truth}$ denote the truth, $\mathfrak {R}_{1In\det er}$ denote the indetermancy and $\mathfrak {R}_{1False}$ denote the falsity-membership function.

For every $l_{11}$ in L, we have $\mathfrak {R}_{1truth}(l_{11}),\mathfrak {R}_{1In\det er}(l_{11}),\mathfrak {R}_{1False}(l_{11})\in [0,1],$ and $0\le \mathfrak {R}_{1truth}(l_{11})+\mathfrak {R}_{1In\det er}(l_{11})+\mathfrak {R}_{1False}(l_{11})\le 3.$

Definition 3

[6] Suppose $l_{11}=(truth_{1},in\det er_{1},false_{1})$ and $l_{22}=(truth_{2},in\det er_{2},False_{2})$ are two SVNNs, then their operational laws are defined as:

• 1. The compliment of $l_{11}$ is ${\bar{l}}_{11}=(False_{1},1-in\det er_{1},truth_{1}).$

• 2. $l_{11}\oplus l_{22}=\big ( truth_{1}+truth_{2}-truth_{1}truth_{2},in\det er_{1}in\det er_{2},False_{1}False_{2}\big ) .$

• 3. $l_{11}\otimes l_{22}=\left( \begin{array}{c} truth_{1}.truth_{2},in\det er_{1}+in\det er_{2}\in \det er_{1}in\det er_{2}, \\ False_{1}+False_{2}-False_{1}False_{2} \end{array} \right) .$

• 4. $nl_{11}=\left( 1-\left( 1-truth_{1}\right) ^{n},\left( in\det er_{1}\right) ^{n},\left( False_{1}\right) ^{n}\right) ,n>0.$

• 5. $l_{11}^{n}=( \left( truth_{1}\right) ^{n},1-\left( 1-in\det er_{1}\right) ^{n},1-( 1-False_{1}) ^{n}) ,n>0.$

Definition 4

[16] Let $\mathring{U}\ne \Phi$ an NCS in L is defined in the form of a pair $\Omega =(\mathfrak {R}_{1},\mathfrak {R}_{2})$, where $\mathfrak {R}_{1}=\{(l_{11};\mathfrak {R}_{1Truth({\bar{l}}_{11})},\mathfrak {R}_{1{\tilde{I}}nd(l_{11})},\mathfrak {R}_{1{\tilde{F}} al(l_{11})})\mid l_{11}\in l_{11}\}$ is an interval ņeutrosophic set in $l_{11}$ and $\mathfrak {R}_{2}=\{(l_{11};\mathfrak {R}_{2truth(l_{11})},\mathfrak {R}_{2{\normalsize {\hat{\imath }}}nd(l_{11})},\mathfrak {R}_{2False(l_{11})})\mid l_{11}\in l_{11})\}$ is a ņeutrosophic set in $l_{11}.$

Definition 5

[30] A ċomplex ņeutrosophic set is defined on a universe of discourse $\mathring{U}$, is described by a truth membership $\left( Truth_{S}(l_{11})\right)$, an indeterminacy membership $\left( In\det er_{S}(l_{11})\right)$, a falsity membership $\left( False_{S}(l_{11})\right)$, and assigning a complex-valued grade of $Truth_{S}(l_{11}),In\det er_{S}(l_{11})$ and $False_{S}(l_{11})$ in S for any $l_{11}\in \mathring{U}.$ The values $Truth_{S}(l_{11}),In\det er_{S}(l_{11}),$$False_{S}(l_{11})$ and their sum may all be with in the unit circle in the ċomplex plane, and so it is of the following form:

$$\begin{aligned} Truth_{S}(l_{11})&=p_{s}(l_{11}).e^{i\mu _{s}(l_{11})}, \\ In\det er_{S}(l_{11})&=q_{s}(l_{11}).e^{i\nu _{s}(l_{11})}, \\ False_{S}(l_{11})&=r_{s}(l_{11}).e^{i\omega _{s}(l_{11})}, \end{aligned}$$

$p_{s}(l_{11}),q_{s}(l_{11})$,$r_{s}(l_{11})$ are respectively real values where $p_{s}(l_{11}),q_{s}(l_{11})$,$r_{s}(l_{11})\in [0,1],$ and $\mu _{s}(l_{11}),\nu _{s}(l_{11}),\omega _{s}(l_{11})\in [0,2\pi ],$ such that the following condition is satisfied: $0\le p_{s}(l_{11})+q_{s}(l_{11})+r_{s}(l_{11})\le 3.$ A complex ņeutrosophic set S can be represented in set form as: $S=\left\{ \left( \begin{array}{c} l_{11},Truth_{S}(l_{11})=s_{Truth},In\det er_{S}(l_{11}) \\ =s_{In\det er},False_{S}(l_{11})=s_{False} \end{array} \right) :l_{11}\in \mathring{U}\right\}$ where $Truth_{S}:X\rightarrow \{s_{Truth}:s_{Truth}\in \mathfrak {R}_{3}|s_{Truth}|\le 1\},$$In\det er_{S}:X\rightarrow \{s_{In\det er}:s_{In\det er}\in \mathfrak {R}_{3}|s_{In\det er}|\le 1\},$$False_{S}:X\rightarrow \{s_{False}:s_{False}\in \mathfrak {R}_{3}|s_{False}|\le 1\}$ and $0 \le |\textit{Truth}_S(l_{11}) + \textit{In } \text {det } \textit{er}_S(l_{11}) + \textit{False}_S(l_{11})| \le 3.$

Complex neutrosophic cubic sets (CNCSs)

In this segment we start the investigation of new kinds of ņeutrosophic sets known as complex ņeutrosophic cubic sets which is the mix of complex sets and ņeutrosophic cubic sets.

Definition 6

A complex ņeutrosophic cubic set is defined on a universe of discourse L is described by a truth membership function $\left( Truth_{{\mathcal {Z}} ^{N}}(l_{11}),truth_{{\mathcal {Z}}^{N}}(l_{11})\right)$, an indeterminacy membership function $\left( In\det er_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{ {\mathcal {Z}}^{N}}(l_{11})\right)$, a falsity membership function $\left( False_{{\mathcal {Z}}^{N}}(l_{11}),false_{{\mathcal {Z}}^{N}}(l_{11})\right)$, and assigning a complex-valued grade of $\left( Truth_{{\mathcal {Z}} ^{N}}(l_{11}),truth_{{\mathcal {Z}}^{N}}(l_{11})\right) ,\left( In\det er_{ {\mathcal {Z}}^{N}}(l_{11}),in\det er_{{\mathcal {Z}}^{N}}(l_{11})\right) ,$ and $\left( False_{{\mathcal {Z}}^{N}}(l_{11}),false_{{\mathcal {Z}}^{N}}(l_{11}) \right) ,$ in ${\mathcal {Z}}^{N}$ for any $l_{11}\in \mathring{U}.$

The values $\left( Truth_{{\mathcal {Z}}^{N}}(l_{11}),truth_{{\mathcal {Z}} ^{N}}(l_{11})\right) ,\left( In\det er_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{ {\mathcal {Z}}^{N}}(l_{11})\right) ,$

$\left( False_{{\mathcal {Z}}^{N}}(l_{11}),false_{{\mathcal {Z}} ^{N}}(l_{11})\right)$ and their sum may all be with in the unit circle in the complex plane, and so it is of the following form:

$$\begin{aligned}&\left( Truth_{{\mathcal {Z}}^{N}}(l_{11}),truth_{{\mathcal {Z}}^{N}}(l_{11}) \right) \\&\quad =\left( P_{{\mathcal {Z}}^{N}}(l_{11}).e^{j{\tilde{\mu }}_{{\mathcal {Z}} ^{N}}(l_{11})},p_{{\mathcal {Z}}^{N}}(l_{11}).e^{i\mu _{{\mathcal {Z}} ^{N}}(l_{11})}\right) , \\&\left( In\det er_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{{\mathcal {Z}} ^{N}}(l_{11})\right) \\&\quad =\left( Q_{{\mathcal {Z}}^{N}}(l_{11}).e^{j{\tilde{\nu }}_{ {\mathcal {Z}}^{N}}(l_{11})},q_{{\mathcal {Z}}^{N}}(l_{11}).e^{i\nu _{{\mathcal {Z}} ^{N}}(l_{11})}\right) , \\&\left( False_{{\mathcal {Z}}^{N}}(l_{11}),false_{{\mathcal {Z}}^{N}}(l_{11}) \right) \\&\quad =\left( R_{{\mathcal {Z}}^{N}}(l_{11}).e^{j{\tilde{\omega }}_{{\mathcal {Z}} ^{N}}(l_{11})},r_{{\mathcal {Z}}^{N}}(l_{11}).e^{i\omega _{{\mathcal {Z}} ^{N}}(l_{11})}\right) , \end{aligned}$$

where

$$\begin{aligned}&\left( P_{{\mathcal {Z}}^{N}}(l_{11}),p_{{\mathcal {Z}}^{N}}(l_{11})\right) ,\left( Q_{{\mathcal {Z}}^{N}}(l_{11}),q_{{\mathcal {Z}}^{N}}(l_{11})\right) ,\\&\quad \left( R_{{\mathcal {Z}}^{N}}(l_{11}),r_{{\mathcal {Z}}^{N}}(l_{11})\right) , \end{aligned}$$

are respectively real values and

$$\begin{aligned}&\left( P_{{\mathcal {Z}}^{N}}(l_{11}),p_{{\mathcal {Z}}^{N}}(l_{11})\right) ,\left( Q_{{\mathcal {Z}}^{N}}(l_{11}),q_{{\mathcal {Z}}^{N}}(l_{11})\right) ,\\&\quad \left( R_{{\mathcal {Z}}^{N}}(l_{11}),r_{{\mathcal {Z}}^{N}}(l_{11})\right) \in [0,1], \end{aligned}$$

where

$$\begin{aligned}&\left( {\tilde{\mu }}_{{\mathcal {Z}}^{N}}(l_{11}),\mu _{{\mathcal {Z}} ^{N}}(l_{11})\right) ,\left( {\tilde{\nu }}_{{\mathcal {Z}}^{N}}(l_{11}),\nu _{ {\mathcal {Z}}^{N}}(l_{11})\right) , \\&\quad \left( {\tilde{\omega }}_{{\mathcal {Z}} ^{N}}(l_{11}),\omega _{{\mathcal {Z}}^{N}}(l_{11})\right) \in [0,2\pi ]. \end{aligned}$$

In set form the complex ņeutrosophic cubic set ${\mathcal {Z}}^{N}$ can be represented as

$$\begin{aligned}&{\mathcal {Z}}^{N}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{{\mathcal {Z}}^{N}}(l_{11}),In\det er_{{\mathcal {Z}} ^{N}}(l_{11}),False_{{\mathcal {Z}}^{N}}(l_{11}), \\ truth_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{{\mathcal {Z}}^{N}}(l_{11}),false_{ {\mathcal {Z}}^{N}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

Example 1

A complex ņeutrosophic cubic set is defined on a universe of discourse L, is described by a truth membership function $\left( \left[ 0.3,0.4 \right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right)$, an indeterminacy membership function $\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.6e^{j\pi 0.4}\right) \right)$, a falsity membership function $\left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right)$, and assigning a complex-valued grade of $\left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5 \right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.6e^{j\pi 0.4}\right) \right) ,$ and $\left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) ,$ in ${\mathcal {Z}}^{N}$ for any $l_{11}\in L.$ Then, the complex neutrosophic cubic set ${\mathcal {Z}}^{N}$ is given as follows:

$$\begin{aligned} {\mathcal {Z}}^{N} =\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.6e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

Definition 7

A complex ņeutrosophic cubic set

$$\begin{aligned}&{\mathcal {Z}}^{N}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{{\mathcal {Z}}^{N}}(l_{11}),In\det er_{{\mathcal {Z}} ^{N}}(l_{11}),False_{{\mathcal {Z}}^{N}}(l_{11}), \\ truth_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{{\mathcal {Z}}^{N}}(l_{11}),false_{ {\mathcal {Z}}^{N}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

in $\mathring{U}$ is said to be

1. Truth-internal complex ņeutrosophic cubic set (TICNCs) if the following is hold $\left( \forall l_{11}\in X\right) \left( Truth_{\mathcal {Z }^{N}}^{-}(l_{11})\le truth_{{\mathcal {Z}}^{N}}(l_{11})\le Truth_{{\mathcal {Z}} ^{N}}^{+}(l_{11})\right)$ and $\left( \forall l_{11}\in circU\right) \left( \mu _{{\mathcal {Z}} ^{N}}^{-}(l_{11})\le \mu _{{\mathcal {Z}}^{N}}(l_{11})\le \mu _{{\mathcal {Z}} ^{N}}^{+}(l_{11})\right) .$

2. Indeterminacy-internal complex ņeutrosophic cubic set (IICNCs) if the following is hold $\left( \forall l_{11}\in circU\right) \left( In\det er_{ {\mathcal {Z}}^{N}}^{-}(l_{11})\le in\det er_{{\mathcal {Z}}^{N}}(l_{11})\le In\det er_{{\mathcal {Z}}^{N}}^{+}(l_{11})\right)$ and $\left( \forall l_{11}\in circU\right) \left( \nu _{{\mathcal {Z}} ^{N}}^{-}(l_{11})\le \nu _{{\mathcal {Z}}^{N}}(l_{11})\le \nu _{{\mathcal {Z}} ^{N}}^{+}(l_{11})\right) .$

3. Falsity-internal complex ņeutrosophic cubic set (FICNCs) if the following is hold $\left( \forall l_{11}\in circU\right) \left( False_{ {\mathcal {Z}}^{N}}^{-}(l_{11})\le false_{{\mathcal {Z}}^{N}}(l_{11})\le False_{ {\mathcal {Z}}^{N}}^{+}(l_{11})\right)$ and $\left( \forall l_{11}\in circU\right) \left( \omega _{{\mathcal {Z}} ^{N}}^{-}(l_{11})\le \omega _{{\mathcal {Z}}^{N}}(l_{11})\le \omega _{ {\mathcal {Z}}^{N}}^{+}(l_{11})\right) .$

If a complex ņeutrosophic cubic set (CNCs) satisfy 1, 2, 3, then it is said to be internal complex ņeutrosophic cubic set (ICNCs).

Definition 8

A complex ņeutrosophic cubic set

$$\begin{aligned}&{\mathcal {Z}}^{N}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{{\mathcal {Z}}^{N}}(l_{11}),In\det er_{{\mathcal {Z}} ^{N}}(l_{11}),False_{{\mathcal {Z}}^{N}}(l_{11}), \\ truth_{{\mathcal {Z}}^{N}}(l_{11}),in\det er_{{\mathcal {Z}}^{N}}(l_{11}),false_{ {\mathcal {Z}}^{N}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

in $l_{11}$ is said to be

1. Truth-external complex ņeutrosophic cubic set (TECNCs) if the following is hold $\left( \forall l_{11}\in circU\right) \left( truth_{ {\mathcal {Z}}^{N}}(l_{11})\notin \left( Truth_{{\mathcal {Z}} ^{N}}^{-}(l_{11}),Truth_{{\mathcal {Z}}^{N}}^{+}(l_{11})\right) \right)$ and $\left( \forall l_{11}\in circU\right) \left( \mu _{{\mathcal {Z}} ^{N}}(l_{11})\notin \left( \mu _{{\mathcal {Z}}^{N}}^{-}(l_{11}),\mu _{\mathcal { Z}^{N}}^{+}(l_{11})\right) \right) .$

2. Indeterminacy-external complex ņeutrosophic cubic set (IECNCs) if the following is hold $\left( \forall l_{11}\in circU\right) \left( in\det er_{ {\mathcal {Z}}^{N}}(l_{11})\notin \left( In\det er_{{\mathcal {Z}} ^{N}}^{-}(l_{11}),In\det er_{{\mathcal {Z}}^{N}}^{+}(l_{11})\right) \right)$ and $\left( \forall l_{11}\in circU\right) \left( \nu _{{\mathcal {Z}} ^{N}}(l_{11})\notin \left( \nu _{{\mathcal {Z}}^{N}}^{-}(l_{11}),\nu _{\mathcal { Z}^{N}}^{+}(l_{11})\right) \right) .$

3. Falsity-external complex ņeutrosophic cubic set (FECNCs) if the following is hold $\left( \forall l_{11}\in X\right) \left( false_{\mathcal {Z }^{N}}(l_{11})\notin \left( False_{{\mathcal {Z}}^{N}}^{-}(l_{11}),False_{ {\mathcal {Z}}^{N}}^{+}(l_{11})\right) \right)$ and $\left( \forall l_{11}\in X\right) \left( \omega _{{\mathcal {Z}} ^{N}}(l_{11})\notin \left( \omega _{{\mathcal {Z}}^{N}}^{-}(l_{11}),\omega _{ {\mathcal {Z}}^{N}}^{+}(l_{11})\right) \right) .$

If a complex ņeutrosophic cubic set (CNCs) satisfy 1, 2, 3 then it is said to be external complex ņeutrosophic cubic set (ECNCs).

Definition 9

Let

$$\begin{aligned}&\mathfrak {R}_{1}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{1}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

and

$$\begin{aligned}&\mathfrak {R}_{2}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11}),False_{ \mathfrak {R}_{2}}(l_{11}), \\ truth_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2} }(l_{11}),false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

be two complex ņeutrosophic cubic sets (CNCSs). We define

1. The complement of $\mathfrak {R}_{1}$, denoted as $\mathfrak {R}_{3}(\mathfrak {R}_{1})$, is specified by functions:

$$\begin{aligned} Truth_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=P_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j{\tilde{\mu }}_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=R_{\mathfrak {R}_{1}}(l_{11}).e^{j\left( 2\pi -{\tilde{\mu }}_{\mathfrak {R}_{1}}(l_{11})\right) } \\ In\det er_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=Q_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j{\tilde{\nu }}_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=\left( 1-Q_{\mathfrak {R}_{1}}(l_{11})\right) .e^{j\left( 2\pi -{\tilde{\nu }}_{\mathfrak {R}_{1}}(l_{11})\right) } \\ False_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=R_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j{\tilde{\omega }}_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=P_{\mathfrak {R}_{1}}(l_{11}).e^{j\left( 2\pi -{\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11})\right) } \\ truth_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=p_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j\mu _{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=r_{\mathfrak {R}_{1}}(l_{11}).e^{j\left( 2\pi -\mu _{\mathfrak {R}_{1}}(l_{11})\right) } \\ in\det er_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=q_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j\nu _{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=\left( 1-q_{\mathfrak {R}_{1}}(l_{11})\right) .e^{j\left( 2\pi -\nu _{\mathfrak {R}_{1}}(l_{11})\right) } \\ false_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})&=r_{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11}).e^{j\omega _{\mathfrak {R}_{3}\left( \mathfrak {R}_{1}\right) }(l_{11})} \\&=p_{\mathfrak {R}_{1}}(l_{11}).e^{j\left( 2\pi -\omega _{\mathfrak {R}_{1}}(l_{11})\right) } \end{aligned}$$

2. $\mathfrak {R}_{1}\subseteq \mathfrak {R}_{2}$ if, (i) $Truth_{\mathfrak {R}_{1}}(l_{11})\le Truth_{\mathfrak {R}_{2}}(l_{11})$ such that $P_{\mathfrak {R}_{1}}(l_{11})\le P_{\mathfrak {R}_{2}}(l_{11})$ and ${\tilde{\mu }}_{\mathfrak {R}_{1}}(l_{11})\le {\tilde{\mu }}_{\mathfrak {R}_{2}}(l_{11}),$

(ii) $In\det er_{\mathfrak {R}_{1}}(l_{11})\ge In\det er_{\mathfrak {R}_{2}}(l_{11})$

such that $Q_{\mathfrak {R}_{1}}(l_{11})\ge Q_{\mathfrak {R}_{2}}(l_{11})$ and ${\tilde{\nu }} _{\mathfrak {R}_{1}}(l_{11})\ge {\tilde{\nu }}_{\mathfrak {R}_{2}}(l_{11}),$

(iii) $False_{\mathfrak {R}_{1}}(l_{11})\ge False_{\mathfrak {R}_{2}}(l_{11})$

such that $R_{\mathfrak {R}_{1}}(l_{11})\ge R_{\mathfrak {R}_{2}}(l_{11})$ and $\tilde{\omega }_{\mathfrak {R}_{1}}(l_{11})\ge {\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11}),$

(iv) $T_{\mathfrak {R}_{1}}(l_{11})\le T_{\mathfrak {R}_{2}}(l_{11})$

such that $p_{\mathfrak {R}_{1}}(l_{11})\le p_{\mathfrak {R}_{2}}(l_{11})$ and $\mu _{\mathfrak {R}_{1}}(l_{11})\le \mu _{\mathfrak {R}_{2}}(l_{11}),$

(v) $in\det er_{\mathfrak {R}_{1}}(l_{11})\ge in\det er_{\mathfrak {R}_{2}}(l_{11})$

such that $q_{\mathfrak {R}_{1}}(l_{11})\ge q_{\mathfrak {R}_{2}}(l_{11})$ and $\nu _{\mathfrak {R}_{1}}(l_{11})\ge \nu _{\mathfrak {R}_{2}}(l_{11}),$

(vi) $false_{\mathfrak {R}_{1}}(l_{11})\ge false_{\mathfrak {R}_{2}}(l_{11})$

such that $r_{\mathfrak {R}_{1}}(l_{11})\ge r_{\mathfrak {R}_{2}}(l_{11})$ and $\omega _{\mathfrak {R}_{1}}(l_{11})\ge \omega _{\mathfrak {R}_{2}}(l_{11}).$

3. The union (intersection) of $\mathfrak {R}_{1}$ and $\mathfrak {R}_{2}$, denoted as $\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}$ and the truth membership function $\left( Truth_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) ,truth_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \right) ,$ the indeterminacy membership function $\left( In\det er_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) ,in\det er_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \right)$ and the falsity membership function $\left( False_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) ,false_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \right)$ are defined as:

$$\begin{aligned}&Truth_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ P_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) P_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( {\tilde{\mu }}_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) {\tilde{\mu }}_{\mathfrak {R}_{2}}(l_{11})\right) } \\&In\det er_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ Q_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) Q_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( {\tilde{\nu }}_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) {\tilde{\nu }}_{\mathfrak {R}_{2}}(l_{11})\right) } \\&False_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ R_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) R_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) {\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11})\right) } \\&truth_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ p_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) p_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( \mu _{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) \mu _{\mathfrak {R}_{2}}(l_{11})\right) } \\&in\det er_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ q_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) q_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( \nu _{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) \nu _{\mathfrak {R}_{2}}(l_{11})\right) } \\&false_{\mathfrak {R}_{1}\cup \left( \cap \right) \mathfrak {R}_{2}}\left( l_{11}\right) \\&\quad = \left[ r_{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) r_{\mathfrak {R}_{2}}(l_{11}) \right] .e^{j\left( \omega _{\mathfrak {R}_{1}}(l_{11})\vee \left( \wedge \right) \omega _{\mathfrak {R}_{2}}(l_{11})\right) } \end{aligned}$$

where $\vee =\max$ and $\wedge =\min .$

Definition 10

Let

$$\begin{aligned}&\mathfrak {R}_{1}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{1}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

and

$$\begin{aligned}&\mathfrak {R}_{2}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11}),False_{\mathfrak {R}_{2}}(l_{11}), \\ T_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

be two complex ņeutrosophic cubic sets (CNCSs) over $\mathring{U}$. The union of $\mathfrak {R}_{1}$ and $\mathfrak {R}_{2}$ is denoted as follows: $\mathfrak {R}_{1}\cup \mathfrak {R}_{2}=$

$$\begin{aligned}&Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \\&In\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf {\tilde{\imath }}_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup {\tilde{\imath }}_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \\&False_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf False_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup False_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \\&Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf t_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup t_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi \omega _{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \\&in\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf in\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup in\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \right] \\&\qquad .e^{j\pi \psi _{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \\&false_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf false_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}),\sup false_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi \phi _{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})} \end{aligned}$$

where

$$\begin{aligned}&\inf Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf Truth_{\mathfrak {R}_{1}}(l_{11}),\inf Truth_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup Truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\vee \left( \sup Truth_{\mathfrak {R}_{1}}(l_{11}),\sup Truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf In\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf In\det er_{\mathfrak {R}_{1}}(l_{11}),\inf In\det er_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup In\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\wedge \left( \sup In\det er_{\mathfrak {R}_{1}}(l_{11}),\sup In\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf False_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf False_{\mathfrak {R}_{1}}(l_{11}),\inf False_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup False_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\wedge \left( \sup False_{\mathfrak {R}_{1}}(l_{11}),\sup False_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf truth_{\mathfrak {R}_{1}}(l_{11}),\inf truth_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup truth_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\vee \left( \sup truth_{\mathfrak {R}_{1}}(l_{11}),\sup truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf in\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf in\det er_{\mathfrak {R}_{1}}(l_{11}),\inf in\det er_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup in\det er_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\wedge \left( \sup in\det er_{\mathfrak {R}_{1}}(l_{11}),\sup in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf false_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf false_{\mathfrak {R}_{1}}(l_{11}),\inf false_{\mathfrak {R}_{2}}(l_{11})\right) ,\sup false_{\mathfrak {R}_{1}\cup \mathfrak {R}_{2}}(l_{11}) \\&\quad =\wedge \left( \sup false_{\mathfrak {R}_{1}}(l_{11}),\sup false_{\mathfrak {R}_{2}}(l_{11})\right) \end{aligned}$$

$\forall l_{11}\in \mathring{U}.$ The union of the phase terms remains the same.

Example 2

Let

$$\begin{aligned} \mathfrak {R}_{1} =\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.7e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

and

$$\begin{aligned} \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.6\right] },\left( 0.7e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

be two CNCSs, then their union is defined as

$$\begin{aligned} \mathfrak {R}_{1}\cup \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.6\right] },\left( 0.7e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.7e^{j\pi 0.5}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

Definition 11

Let

$$\begin{aligned}&\mathfrak {R}_{1}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{1}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

and

$$\begin{aligned}&\mathfrak {R}_{2}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{2}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11}),False_{\mathfrak {R}_{2}}(l_{11}), \\ truth_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

be two complex ņeutrosophic cubic sets (CNCSs) over $l_{11}.$ The intersection of $\mathfrak {R}_{1}$ and $\mathfrak {R}_{2}$ is denoted as $\mathfrak {R}_{1}\cap \mathfrak {R}_{2}=$

$$\begin{aligned}&Truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf Truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup Truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \\&In\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf In\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup In\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \right] \\&\qquad .e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \\&False_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf False_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup False_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \\&truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi \omega _{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \\&in\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf in\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup in\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \right] \\&\qquad .e^{j\pi \psi _{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \\&false_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\left[ \inf false_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}),\sup false_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\right] \\&\qquad .e^{j\pi \phi _{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})} \end{aligned}$$

where

$$\begin{aligned}&\inf Truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf Truth_{\mathfrak {R}_{1}}(l_{11}),\inf Truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup Truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\wedge \left( \sup Truth_{\mathfrak {R}_{1}}(l_{11}),\sup Truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf In\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf In\det er_{\mathfrak {R}_{1}}(l_{11}),\inf In\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup In\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\vee \left( \sup In\det er_{\mathfrak {R}_{1}}(l_{11}),\sup In\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf False_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf False_{\mathfrak {R}_{1}}(l_{11}),\inf False_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup False_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\vee \left( \sup False_{\mathfrak {R}_{1}}(l_{11}),\sup False_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\wedge \left( \inf truth_{\mathfrak {R}_{1}}(l_{11}),\inf truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup truth_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\wedge \left( \sup truth_{\mathfrak {R}_{1}}(l_{11}),\sup truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf in\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf in\det er_{\mathfrak {R}_{1}}(l_{11}),\inf in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup in\det er_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\vee \left( \sup in\det er_{\mathfrak {R}_{1}}(l_{11}),\sup in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\inf false_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11})\\&\quad =\vee \left( \inf false_{\mathfrak {R}_{1}}(l_{11}),\inf false_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad ,\sup false_{\mathfrak {R}_{1}\cap \mathfrak {R}_{2}}(l_{11}) \\ \\&\quad =\vee \left( \sup false_{\mathfrak {R}_{1}}(l_{11}),\sup false_{\mathfrak {R}_{2}}(l_{11})\right) \end{aligned}$$

$\forall l_{11}\in \mathring{U}.$ The intersection of the phase terms remains the same.

Example 3

Let

$$\begin{aligned} \mathfrak {R}_{1}=\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.7e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

and

$$\begin{aligned} \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.6\right] },\left( 0.7e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

then

$$\begin{aligned} \mathfrak {R}_{1}\cap \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

Proposition 1

Let

$$\begin{aligned}&\mathfrak {R}_{1}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{1}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} , \\&\mathfrak {R}_{2}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11}),False_{\mathfrak {R}_{2}}(l_{11}), \\ truth_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} , \\&\mathfrak {R}_{3}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{3}}(l_{11}),In\det er_{\mathfrak {R}_{3}}(l_{11}),False_{\mathfrak {R}_{3}}(l_{11}), \\ truth_{\mathfrak {R}_{3}}(l_{11}),in\det er_{\mathfrak {R}_{3}}(l_{11}),false_{l_{22}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} \end{aligned}$$

be three complex ņeutrosophic cubic sets over $\mathring{U}$. Then

• 1. $\mathfrak {R}_{1}\cup \mathfrak {R}_{2}=\mathfrak {R}_{2}\cup \mathfrak {R}_{1},$

• 2. $\mathfrak {R}_{1}\cap \mathfrak {R}_{2}=\mathfrak {R}_{2}\cap \mathfrak {R}_{1},$

• 3. $\mathfrak {R}_{1}\cup \mathfrak {R}_{1}=\mathfrak {R}_{1},$

• 4. $\mathfrak {R}_{1}\cap \mathfrak {R}_{1}=\mathfrak {R}_{1},$

• 5. $\mathfrak {R}_{1}\cup \left( \mathfrak {R}_{2}\cup \mathfrak {R}_{3}\right) =\left( \mathfrak {R}_{1}\cup \mathfrak {R}_{2}\right) \cup \mathfrak {R}_{3},$

• 6. $\mathfrak {R}_{1}\cap \left( \mathfrak {R}_{2}\cap \mathfrak {R}_{3}\right) =\left( \mathfrak {R}_{1}\cap \mathfrak {R}_{2}\right) \cap \mathfrak {R}_{3},$

• 7. $\mathfrak {R}_{1}\cup \left( \mathfrak {R}_{2}\cap \mathfrak {R}_{3}\right) =\left( \mathfrak {R}_{1}\cup \mathfrak {R}_{2}\right) \cap \left( \mathfrak {R}_{1}\cup \mathfrak {R}_{3}\right) .$

• 8. $\mathfrak {R}_{1}\cap \left( \mathfrak {R}_{2}\cup \mathfrak {R}_{3}\right) =\left( \mathfrak {R}_{1}\cap \mathfrak {R}_{2}\right) \cup \left( \mathfrak {R}_{1}\cap \mathfrak {R}_{3}\right) ,$

• 9. $\mathfrak {R}_{1}\cup \left( \mathfrak {R}_{1}\cap \mathfrak {R}_{2}\right) =\mathfrak {R}_{1},$

• 10. $\mathfrak {R}_{1}\cap \left( \mathfrak {R}_{1}\cup \mathfrak {R}_{2}\right) =\mathfrak {R}_{1},$

• 11. $\left( \mathfrak {R}_{1}\cup \mathfrak {R}_{2}\right) ^{C}=\mathfrak {R}_{1}^{^{C}}\cap \mathfrak {R}_{2}^{^{C}},$

• 12. $\left( \mathfrak {R}_{1}\cap \mathfrak {R}_{2}\right) ^{^{C}}=\mathfrak {R}_{1}^{^{C}}\cup \mathfrak {R}_{2}^{^{C}},$

• 13. $\left( \mathfrak {R}_{1}^{^{C}}\right) ^{^{C}}=\mathfrak {R}_{1}.$

Proof

All these statements can be easily proved. $\square$

Operational rules of complex neutrosophic cubic sets

In this section we define some basic operational rules which are helpful in the manipulations between the complex ņeutrosophic cubic sets.

Definition 12

Let

$$\begin{aligned}&\mathfrak {R}_{1}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{1}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} , \\&\mathfrak {R}_{2}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11}),False_{\mathfrak {R}_{2}}(l_{11}), \\ truth_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) :l_{11}\in \mathring{U}\right\} , \end{aligned}$$

be two complex ņeutrosophic cubic sets over $\mathring{U}$ which are defined by

$$\begin{aligned}&\left( Truth_{\mathfrak {R}_{1}}(l_{11}),truth_{\mathfrak {R}_{1}}(l_{11})\right) \\&\quad =\left( Truth_{\mathfrak {R}_{1}}(l_{11}),truth_{\mathfrak {R}_{1}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11})},e^{j\pi \omega _{\mathfrak {R}_{1}}(l_{11})}\right) , \\&\left( In\det er_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11})\right) \\&\quad =\left( In\det er_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{1}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{1}}(l_{11})},e^{j\pi \psi _{\mathfrak {R}_{1}}(l_{11})}\right) , \\&\left( False_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11})\right) \\&\quad =\left( False_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{1}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{1}}(l_{11})},e^{j\pi \phi _{\mathfrak {R}_{1}}(l_{11})}\right) \end{aligned}$$

and

$$\begin{aligned}&\left( Truth_{\mathfrak {R}_{2}}(l_{11}),truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( Truth_{\mathfrak {R}_{2}}(l_{11}),truth_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11})},e^{j\pi \omega _{\mathfrak {R}_{2}}(l_{11})}\right) , \\&\left( In\det er_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( In\det er_{\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{2}}(l_{11})},e^{j\pi \psi _{\mathfrak {R}_{2}}(l_{11})}\right) , \\&\left( False_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( False_{\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11})\right) \\&\qquad .\left( e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{2}}(l_{11})},e^{j\pi \phi _{\mathfrak {R}_{2}}(l_{11})}\right) . \end{aligned}$$

respectively. Then, the operational rules of complex ņeutrosophic cubic sets (CNCSs) are defined as follows:

1. The product of $\mathfrak {R}_{1}$ and $\mathfrak {R}_{2}$, is denoted as $\mathfrak {R}_{1}\times \mathfrak {R}_{2},$ is:

$$\begin{aligned}&\left( Truth_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11}),truth_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( Truth_{\mathfrak {R}_{1}}(l_{11}),Truth_{\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( t_{\mathfrak {R}_{1}}(l_{11}),t_{\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\qquad .\left( \begin{array}{c} e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \omega _{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \\&\left( In\det er_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( In\det er_{\mathfrak {R}_{1}}(l_{11}),In\det er_{\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( in\det er_{\mathfrak {R}_{1}}(l_{11}),in\det er_{\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\qquad .\left( \begin{array}{c} e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \psi _{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \\&\left( False_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( False_{\mathfrak {R}_{1}}(l_{11}),False_{\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( false_{\mathfrak {R}_{1}}(l_{11}),false_{\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\qquad .\left( \begin{array}{c} e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \phi _{\mathfrak {R}_{1}*\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \end{aligned}$$

The product of the phase term is defined as follows:

$$\begin{aligned}&\left( {\tilde{\omega }}_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11}), {\tilde{\omega }}_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \omega _{\mathfrak {R}_{1}}(l_{11})\omega _{\mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}}(l_{11})\omega _{\mathfrak {R}_{2}}(l_{11})\right) \\&\left( {\tilde{\psi }}_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\psi }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\psi }}_{\mathfrak {R}_{2}}(l_{11}),\tilde{ \psi }_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \psi _{\mathfrak {R}_{1}}(l_{11})\psi _{\mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\psi }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\psi }}_{\mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}}(l_{11})\psi _{\mathfrak {R}_{2}}(l_{11})\right) \\&\left( {\tilde{\phi }}_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\phi }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\phi }}_{\mathfrak {R}_{2}}(l_{11}),\tilde{ \phi }_{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \phi _{\mathfrak {R}_{1}}(l_{11})\omega _{\mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}\times \mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\phi }}_{\mathfrak {R}_{1}}(l_{11}){\tilde{\phi }}_{\mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}}(l_{11})\phi _{\mathfrak {R}_{2}}(l_{11})\right) \end{aligned}$$

Example 4

Let

$$\begin{aligned} \mathfrak {R}_{1} =\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.7e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

and

$$\begin{aligned} \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.6\right] },\left( 0.7e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

then

$$\begin{aligned} \mathfrak {R}_{1}\times \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

2. The addition of $\mathfrak {R}_{1}$ and $\mathfrak {R}_{2}$, is denoted as $\mathfrak {R}_{1}+\mathfrak {R}_{2},$ is:

$$\begin{aligned}&\left( Truth_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),Truth_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} Truth_{\mathfrak {R}_{1}}(l_{11})+Truth_{\mathfrak {R}_{2}}(l_{11}) \\ -Truth_{\mathfrak {R}_{1}}(l_{11})Truth_{\mathfrak {R}_{2}}(l_{11}), \\ truth_{\mathfrak {R}_{1}}(l_{11})+truth_{\mathfrak {R}_{2}}(l_{11}) \\ -truth_{\mathfrak {R}_{1}}(l_{11})truth_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) \\&\qquad .\left( \begin{array}{c} e^{j\pi {\tilde{\omega }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \omega _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \\&\left( In\det er_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),in\det er_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} In\det er_{\mathfrak {R}_{1}}(l_{11})+In\det er_{\mathfrak {R}_{2}}(l_{11}) \\ -In\det er_{\mathfrak {R}_{1}}(l_{11})In\det er_{\mathfrak {R}_{2}}(l_{11}), \\ in\det er_{\mathfrak {R}_{1}}(l_{11})+in\det er_{\mathfrak {R}_{2}}(l_{11}) \\ -in\det er_{\mathfrak {R}_{1}}(l_{11})in\det er_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) .\left( \begin{array}{c} e^{j\pi {\tilde{\psi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \psi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \\&\left( False_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),false_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} False_{\mathfrak {R}_{1}}(l_{11})+False_{\mathfrak {R}_{2}}(l_{11}) \\ -False_{\mathfrak {R}_{1}}(l_{11})False_{\mathfrak {R}_{2}}(l_{11}), \\ false_{\mathfrak {R}_{1}}(l_{11})+false_{\mathfrak {R}_{2}}(l_{11}) \\ -false_{\mathfrak {R}_{1}}(l_{11})false_{\mathfrak {R}_{2}}(l_{11}) \end{array} \right) .\left( \begin{array}{c} e^{j\pi {\tilde{\phi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})}, \\ e^{j\pi \phi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})} \end{array} \right) \end{aligned}$$

The addition of the phase term is defined as follows:

$$\begin{aligned}&\left( {\tilde{\omega }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11}), {\tilde{\omega }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \omega _{\mathfrak {R}_{1}}(l_{11})+\omega _{\mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\omega }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\omega }}_{\mathfrak {R}_{2}}(l_{11}),\omega _{\mathfrak {R}_{1}}(l_{11})+\omega _{\mathfrak {R}_{2}}(l_{11})\right) \\&\left( {\tilde{\psi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\psi }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\psi }}_{\mathfrak {R}_{2}}(l_{11}), {\tilde{\psi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \psi _{\mathfrak {R}_{1}}(l_{11})+\psi _{\mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\psi }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\psi }}_{\mathfrak {R}_{2}}(l_{11}),\psi _{\mathfrak {R}_{1}}(l_{11})+\psi _{\mathfrak {R}_{2}}(l_{11})\right) \\&\left( {\tilde{\phi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \\&\quad =\left( \begin{array}{c} \left( {\tilde{\phi }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\phi }}_{\mathfrak {R}_{2}}(l_{11}), {\tilde{\phi }}_{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) , \\ \left( \phi _{\mathfrak {R}_{1}}(l_{11})+\omega _{\mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}+\mathfrak {R}_{2}}(l_{11})\right) \end{array} \right) \\&\quad =\left( {\tilde{\phi }}_{\mathfrak {R}_{1}}(l_{11})+{\tilde{\phi }}_{\mathfrak {R}_{2}}(l_{11}),\phi _{\mathfrak {R}_{1}}(l_{11})+\phi _{\mathfrak {R}_{2} }(l_{11})\right) \end{aligned}$$

Example 5

Let

$$\begin{aligned} \mathfrak {R}_{1}=\left\{ \left( \begin{array}{c} \left( \left[ 0.3,0.4\right] e^{j\pi \left[ 0.4,0.5\right] },\left( 0.5e^{j\pi 0.4}\right) \right) ,\left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.7e^{j\pi 0.4}\right) \right) , \\ \left( \left[ 0.4,0.6\right] e^{j\pi \left[ 0.4,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) \end{array} \right) \right\} \end{aligned}$$

and

$$\begin{aligned} \mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.6\right] },\left( 0.7e^{j\pi 0.6}\right) \right) ,\left( \left[ 0.4,0.5\right] e^{j\pi \left[ 0.5,0.7\right] },\left( 0.6e^{j\pi 0.5}\right) \right) , \\ \left( \left[ 0.3,0.5\right] e^{j\pi \left[ 0.3,0.6\right] },\left( 0.5e^{j\pi 0.4}\right) \right) \end{array} \right) \right\} \end{aligned}$$

then

$$\begin{aligned} \mathfrak {R}_{1}+\mathfrak {R}_{2} =\left\{ \left( \begin{array}{c} \left( \left[ 0.58,0.7\right] e^{j\pi \left[ 0.7,0.8\right] },\left( 0.85e^{j\pi 0.8}\right) \right) ,\left( \left[ 0.58,0.75\right] e^{j\pi \left[ 0.7,0.91\right] },\left( 0.88e^{j\pi 0.7}\right) \right) , \\ \left( \left[ 0.58,0.8\right] e^{j\pi \left[ 0.58,0.88\right] },\left( 0.8e^{j\pi 0.7}\right) \right) \end{array} \right) \right\} \end{aligned}$$

Multi-criteria group decision-making model in complex neutrosophic cubic set

In this area we will acquaint the methodology with different characteristic collective choice making with the assistance of the complex ņeutrosophic cubic set (CNCSs). We apply complex ņeutrosophic cubic set administrator to manage the characteristic basic leadership issue under the complex neutrosophic cubic set situations then we represent our methodology with a model.

Application in multiple attribute group decision making problem

In a problem of multiple attribute group decision making, Suppose $U=\{U_{1},U_{2},\ldots ,U_{m}\}$ is a set of alternatives. $A_{j}= \{A_{1},A_{2},\ldots ,A_{n}\}$ is a set of attributes and ${\hat{w}}=\left( {\hat{w}} _{1},{\hat{w}}_{2},\ldots ,{\hat{w}}_{n}\right)$ is the weighted vector of the criteria, where, ${\hat{w}}_{i}\epsilon \left[ 0,1\right]$ and $\sum {\hat{w}} _{i}=1.$ The evaluation value of an attribute $A_{j}$$\left( j=1,2,\ldots , n\right)$ with respect to an alternatives $U_{i}$$\left( i=1,2,\ldots ,m\right)$ is express by a CNCS

$$\begin{aligned}&S_{ijk}\\&\quad =\left\{ \left( \begin{array}{c} l_{11},Truth_{S_{ijk}}(l_{11}),In\det er_{S_{ijk}}(l_{11}),False_{S_{_{ijk}}}(l_{11}), \\ truth_{S_{ijk}}(l_{11}),in\det er_{S_{ijk}}(l_{11}),false_{S_{_{ijk}}}(l_{11}) \end{array} \right) :l_{11}\in L\right\} \\&\qquad \left( j=1,2,\ldots ,n;i=1,2,\ldots ,m;k=1,2,\ldots ,h\right) , \end{aligned}$$

so, the decision matrix is obtained: $D=\left( S_{ij}\right) _{m\times n}.$

The step of the decision making based on complex ņeutrosophic cubic sets is proposed as follows:

Step 1, 2 : Using the operational rules of the complex neutrosophic cubic sets (CNCSs), the average suitability rating

$$\begin{aligned} S_{i_{j}}=\left( \begin{array}{c} \left( Truth_{S_{i_{j}}}(l_{11}),In\det er_{S_{i_{j}}}(l_{11}),False_{S_{i_{j}}}(l_{11})\right) , \\ \left( truth_{S_{i_{j}}}(l_{11}),in\det er_{S_{i_{j}}}(l_{11}),false_{S_{i_{j}}}(l_{11})\right) \end{array} \right) \end{aligned}$$

can be evaluated as:

$$\begin{aligned} S_{ij}=\frac{1}{h}\otimes \left( S_{ij}\oplus S_{ij}\oplus ...\oplus S_{ijk}\oplus ...\oplus S_{ijh}\right) \end{aligned}$$

where

$$\begin{aligned}&Truth_{S_{i_{j}}}\\&\quad =\left[ \wedge \left( \frac{1}{h}\sum \limits _{k=1}^{h}Truth_{S_{ijk}},1\right) ,\wedge \left( \frac{1}{h}\sum \limits _{k=1}^{h}truth_{S_{ijk}},1\right) \right] \\&\qquad e^{j\pi \left[ \frac{1}{h} \sum \limits _{k=1}^{h}w_{k}\left( l_{11}\right) \right] } \\&In\det er_{S_{i_{j}}}\\&\quad =\left[ \wedge \left( \frac{1}{h}\sum \limits _{k=1}^{h}In\det er_{S_{ijk}},1\right) ,\wedge \left( \frac{1}{h} \sum \limits _{k=1}^{h}in\det er_{S_{ijk}},1\right) \right] \\&\qquad e^{j\pi \left[ \frac{1}{h}\sum \limits _{k=1}^{h}\Psi _{k}\left( l_{11}\right) \right] } \\&False_{S_{i_{j}}}\\&\quad =\left[ \wedge \left( \frac{1}{h}\sum \limits _{k=1}^{h}False_{S_{ijk}},1\right) ,\wedge \left( \frac{1}{h}\sum \limits _{k=1}^{h}false_{S_{ijk}},1\right) \right] \\&\qquad e^{j\pi \left[ \frac{1}{h} \sum \limits _{k=1}^{h}\Phi _{k}\left( l_{11}\right) \right] } \end{aligned}$$

Step 3: To aggregate the weighted rating of alternatives according to the following formula,

$$\begin{aligned} V_{0}=\frac{1}{p}\sum \limits _{p=1}^{h}s_{ij}\times w,0=1,p=1,\ldots ,h \end{aligned}$$

Step 4: To rank the alternatives (Fig. 1)

Fig. 1

A flow chart of CNCSs based on MADM problem

Numerical example

Step 1: An investment company intends to choose one product to invest his/her money from three candidates $\left( U_1-U_3\right)$. Three criteria $A_1$ = price, $A_2$ = quality and $A_3$ = model have been evaluated. They are shown as follows:

Step 2: To calculate the average suitability rate of each alternatives using  5.1

$$\begin{aligned}&U_{1}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.2435,0.3984\right] e^{j\pi \left[ 0.2175,0.44418\right] }, \\ \left[ 0.35,0.49\right] e^{j\pi \left[ 0.2160,0.4214\right] }, \\ \left[ 0.2004,0.4680\right] e^{j\pi \left[ 0.3235,0.5098\right] } \end{array} \right) , \\ \left( 0.6334e^{j\pi \left( 0.3327\right) },0.6333e^{j\pi \left( 0.333\right) },0.433e^{j\pi \left( 0.366\right) }\right) \end{array} \right) \\&U_{2}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.2160,0.4234\right] e^{j\pi \left[ 0.2170,0.3519\right] }, \\ \left[ 0.3235,0.5307\right] e^{j\pi \left[ 0.2977,0.4451\right] }, \\ \left[ 0.16216,0.25003\right] e^{j\pi \left[ 0.352,0.5488\right] } \end{array} \right) , \\ \left( 0.6667e^{j\pi \left( 0.3664\right) },0.566e^{j\pi \left( 0.4\right) },0.399e^{j\pi \left( 0.399\right) }\right) \end{array} \right) \\&U_{3}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.2440,0.769\right] e^{j\pi \left[ 0.0958,0.3497\right] }, \\ \left[ 0.3483,0.5099\right] e^{j\pi \left[ 0.271,0.4680\right] }, \\ \left[ 0.25003,0.3064\right] e^{j\pi \left[ 0.3483,0.46735\right] } \end{array} \right) , \\ \left( 0.499e^{j\pi \left( 0.3667\right) },0.5667e^{j\pi \left( 0.3997\right) },0.5996e^{j\pi \left( 0.4663\right) }\right) \end{array} \right) \end{aligned}$$

Step 3: To aggregate the weighted rating of alternatives using the where $w=\left( 0.5,0.3,0.2\right)$

$$\begin{aligned}&U_{1}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.1218,0.1992\right] e^{j\pi \left[ 0.1088,0.2209\right] }, \\ \left[ 0.175,0.245\right] e^{j\pi \left[ 0.108,0.2107\right] }, \\ \left[ 0.1002,0.234\right] e^{j\pi \left[ 0.1618,0.2549\right] } \end{array} \right) , \\ \left( 0.3167e^{j\pi \left( 0.1664\right) },0.3167e^{j\pi \left( 0.1665\right) },0.2165e^{j\pi \left( 0.183\right) }\right) \end{array} \right) \\&U_{2}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.0648,0.1270\right] e^{j\pi \left[ 0.0651,0.1056\right] }, \\ \left[ 0.0971,0.1592\right] e^{j\pi \left[ 0.0893,0.1335\right] }, \\ \left[ 0.04865,0.07501\right] e^{j\pi \left[ 0.1056,0.1646\right] } \end{array} \right) , \\ \left( 0.2000e^{j\pi \left( 0.1099\right) },0.1698e^{j\pi \left( 0.12\right) },0.1197e^{j\pi \left( 0.1197\right) }\right) \end{array} \right) \\&U_{3}\\&\quad =\left( \begin{array}{c} \left( \begin{array}{c} \left[ 0.0488,0.1538\right] e^{j\pi \left[ 0.0192,0.0699\right] }, \\ \left[ 0.0697,0.1019\right] e^{j\pi \left[ 0.0542,0.0936\right] }, \\ \left[ 0.05001,0.0613\right] e^{j\pi \left[ 0.0697,0.1135\right] } \end{array} \right) , \\ \left( 0.0998e^{j\pi \left( 0.07334\right) },0.1133e^{j\pi \left( 0.0799\right) },0.1199e^{j\pi \left( 0.0933\right) }\right) \end{array} \right) \end{aligned}$$

Step 4: To find out the rank of the alternatives

$$\begin{aligned} \begin{array}{ccc} &{} \text {Amplitude term} &{} \text {Phase term} \\ U_{1} &{} 0.5945 &{} -0.4057\pi \\ U_{2} &{} 0.6353 &{} -0.3223\pi \\ U_{3} &{} 0.6533 &{} -0.2419\pi \end{array} \end{aligned}$$

$U_{3}\succ U_{2}\succ U_{1}$

Step 5: end.

Comparison and conclusions

This paper sums up the possibility of ņeutrosophic cubic sets given by Jun et al. [9]. The possibility of complex ņeutrosophic cubic sets gives us a wide range for reality, uncertain and deception capacities where one can talk about more parameters. We propose the complex ņeutrosophic cubic sets (internal and external) show, which is a mix of complex fluffy sets, ņeutrosophic sets and cubic sets. Additionally we talked about various properties. Toward the end, with the assistance of the complex ņeutrosophic cubic set (CNCSs) we build up a way to deal with different characteristic cooperative choice making. In future our proposed structure might be use in numerous ways, for example, master frameworks, flag handling and in logarithmic structures.