Vibration frequency analysis of an electrically-actuated microbeam resonator accounting for thermoelastic coupling effects

Abstract

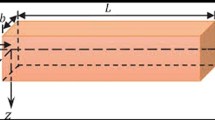
The paper deals with a thermo-mechanical problem for a slender microbeam subjected to an electric actuation; the purpose of the study is to improve the knowledge of loss mechanisms in micro-electro-mechanical devices and to predict accurately the dynamical behaviour. The thermoelastic damping in microbeam resonators is strictly correlated to the mechanical behaviour, and the thermoelastic response of the structure changes significantly near critical frequencies. To fully understand the thermoelastic coupling effects, we add the description of the thermal phenomena to the mechanical problem obtaining a system of two coupled PDEs. The proposed governing equations, by making use of a unified model, are able to describe the response by using the classical thermoelastic formulation and two distinct generalized theories, namely, the Lord–Shulman and the Green–Lindsay models. The study is carried out by means of a spectral approximation method and numerical simulations. The results show the influence of the relaxation times and the presence of dissipation peaks in the different formulations.

Appendix

1.1 Appendix 1

We report here the dimensionless parameters used in the dimensionless system (25):

$$\begin{aligned}&\alpha _3=\dfrac{D_{2}}{D_{1} L^{2}}, \quad c=\dfrac{\hat{c}L^{2}}{\sqrt{\rho A D_{1}}}, \quad N=\dfrac{EAL^{2}}{D_{1}}N_{0}, \nonumber \\&t_{0,1,2}=\sqrt{\dfrac{D_{1}}{\rho A L^{4}}}\hat{t}_{0,1,2},\quad \beta =\dfrac{h b L^{2}}{D_{1}}T_{0}\hat{\beta },\nonumber \\&\gamma =\dfrac{h}{g},\quad \alpha _{2} V^{2}=\dfrac{L^{4} \varepsilon _{0} \varepsilon _{r} b}{2 D_{1} g^{3}}V^{2}, \quad \fancyscript{L}=\dfrac{h}{L},\nonumber \\&\alpha _{1}=\dfrac{EA}{2 D_{1}}\left( \dfrac{g}{L} \right) ^{2}, \quad \eta =\dfrac{g}{b},\quad \xi =\dfrac{h}{b}, \nonumber \\&k=\dfrac{h b L^{2}}{D_{1}}\sqrt{\dfrac{\rho A L^{4}}{D_{1}}}\dfrac{T_{0}}{h^{2}}\hat{k},\quad \fancyscript{C}=\rho {c}_{\varepsilon }T_{0}\dfrac{h b L^{2}}{D_{1}},\nonumber \\&\alpha _{4}=\dfrac{D_{3}}{D_{1}}\left( \dfrac{g}{L} \right) ^{2}. \end{aligned}$$

(60)

1.2 Appendix 2

$$\begin{aligned}&F_{e}\left[ r \right] =\alpha _{2} \left( 2 V_{dc}V_{ac}+V_{ac}^{2}\right) \nonumber \\&\qquad \frac{ \left( 1 +0.265 (1-w_{s})^{0.75} \eta ^{0.75}+0.53 \sqrt{1-w_{s}} \sqrt{\gamma } \eta \right) }{(1-w_{s})^{2}}\nonumber \\&\qquad +\,\alpha _{2} \left( V_{ac}+V_{dc}\right) ^{2} r^{3} \left( \frac{-\frac{0.0103516 \eta ^{0.75}}{(1-w_s)^{2.25}}-\frac{0.033125 \sqrt{\gamma } \eta }{(1-w_{s})^{5/2}}}{(1-w_{s})^2}\right. \nonumber \\&\qquad \left. -\frac{2 \left( -\frac{0.0248438 \eta ^{0.75}}{(1-w_{s})^{1.25}}-\frac{0.06625 \sqrt{\gamma } \eta }{(1-w_{s})^{3/2}}\right) }{(-1+w_{s})^{3}} \right. \nonumber \\&\qquad +\frac{3 \left( -\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}\right) }{(-1+w_{s})^4}\nonumber \\&\qquad \left. -\frac{4 \left( 1\!+\!0.265 (1-w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1\!-\!w_{s}} \sqrt{\gamma } \eta \right) }{(-1\!+\!w_{s})^5}\right) \nonumber \\&\qquad +\,\alpha _{2} \left( V_{ac}+V_{dc}\right) ^{2} r^{2} \left( \frac{-\frac{0.0248438 \eta ^{0.75}}{(1-w_{s})^{1.25}}-\frac{0.06625 \sqrt{\gamma } \eta }{(1-w_{s})^{3/2}}}{(1-w_{s})^2}\right. \nonumber \\&\qquad \left. -\frac{2 \left( -\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}\right) }{(-1+w_{s})^3} \right. \nonumber \\&\qquad +\left. \frac{3 \left( 1\!+\!0.265 (1\!-\!w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1\!-\!w_{s}} \sqrt{\gamma } \eta \right) }{(-1+w_{s})^4}\right) \nonumber \\&\qquad +\,\alpha _{2}\left( V_{ac}+V_{dc}\right) ^{2} r \left( \frac{-\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}}{(1-w_{s})^2}\right. \nonumber \\&\qquad \left. -\frac{2 \left( 1\!+\!0.265 (1\!-\!w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1\!-\!w_{s}} \sqrt{\gamma } \eta \right) }{(-1+w_{s})^{3}}\right) .\nonumber \\ \end{aligned}$$

(61)

1.3 Appendix 3

$$\begin{aligned}&f_{1}(t)=\omega _{1}^{2}-\alpha _1\int _{0}^{1}\left( 2 w_{s}^{\prime }\phi _{1}^{\prime } \right) dx\int _{0}^{1}\left( w_{s}^{\prime \prime }\phi _1 \right) dx\nonumber \\&\quad -\alpha _{1}\int _{0}^ {1}\left( w_{s}^{\prime } \right) ^{2}dx\int _{0}^{1}\left( \phi _{1}^{\prime \prime }\phi _{1} \right) dx\nonumber \\&\quad -\alpha _{2}\left( v_{ac}\cos \varOmega t+V_{dc} \right) ^{2}\int _{0}^{1}\left( \frac{-\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}}{(1-w_{s})^{2}}\right. \nonumber \\&\quad \left. -\frac{2 \left( 1\!+\!0.265 (1\!-\!w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1-w_{s}} \sqrt{\gamma } \eta \right) }{(-1\!+\!w_{s})^3}\right) \phi _{1}^{2} dx,\nonumber \\ \end{aligned}$$

(62)

$$\begin{aligned}&f_{2}(t)=-\alpha _{1}\int _{0}^{1}\left( \phi _{1}^{\prime } \right) ^{2} dx\int _{0}^{1}\left( w_{s}^{\prime \prime }\phi _{1} \right) dx\nonumber \\&\quad -\alpha _{1}\int _{0}^{1}\left( 2 w_{s}^{\prime }\phi _{1}^{'} \right) dx\int _{0}^{1}\left( \phi _{1}^{''}\phi _{1} \right) dx\nonumber \\&\quad -\alpha _{2}\left( v_{ac}\cos \varOmega t+V_{dc} \right) ^{2}\int _{0}^{1} \left( \frac{-\frac{0.0248438 \eta ^{0.75}}{(1-w_{s})^{1.25}}-\frac{0.06625 \sqrt{\gamma } \eta }{(1-w_{s})^{3/2}}}{(1-w_{s})^2}\right. \nonumber \\&\left. \quad -\frac{2 \left( -\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}\right) }{(-1+w_{s})^{3}} \right. \nonumber \\&\quad +\left. \frac{3 \left( 1\!+\!0.265 (1\!-\!w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1-w_{s}} \sqrt{\gamma } \eta \right) }{(-1+w_{s})^{4}}\right) \phi _{1}^{3} dx,\nonumber \\ \end{aligned}$$

(63)

$$\begin{aligned}&f_{3}(t)=-\alpha _{1}\int _{0}^{1}\left( \phi _{1}^{'} \right) ^{2} dx\int _{0}^{1}\left( \phi _{1}^{''}\phi _{1} \right) dx\nonumber \\&\quad -\alpha _{2}\left( v_{ac}\cos \varOmega t+V_{dc} \right) ^{2}\int _{0}^{1} \left( \frac{-\frac{0.0103516 \eta ^{0.75}}{(1-w_{s})^{2.25}}-\frac{0.033125 \sqrt{\gamma } \eta }{(1-w_{s})^{5/2}}}{(1-w_{s})^2}\right. \nonumber \\&\quad \left. -\frac{2 \left( -\frac{0.0248438 \eta ^{0.75}}{(1-w_{s})^{1.25}}-\frac{0.06625 \sqrt{\gamma } \eta }{(1-w_{s})^{3/2}}\right) }{(-1+w_{s})^{3}} \right. \nonumber \\&\quad +\left. \frac{3 \left( -\frac{0.19875 \eta ^{0.75}}{(1-w_{s})^{0.25}}-\frac{0.265 \sqrt{\gamma } \eta }{\sqrt{1-w_{s}}}\right) }{(-1+w_{s})^4}\right. \nonumber \\&\left. \quad -\frac{4 \left( 1\!+\!0.265 (1\!-\!w_{s})^{0.75} \eta ^{0.75}\!+\!0.53 \sqrt{1\!-\!w_{s}} \sqrt{\gamma } \eta \right) }{(-1+w_{s})^{5}}\right) \phi _{1}^{4} dx,\nonumber \\ \end{aligned}$$

(64)

$$\begin{aligned}&f_{0}(t)=-\alpha _2\left( 2 V_{dc} v_{ac}\cos \varOmega t\right. \nonumber \\&\quad \left. +\left( v_{ac}\cos \varOmega t \right) ^{2} \right) \int _{0}^{1}\nonumber \\&\quad \frac{ \left( 1 +0.265 (1-w_{s})^{0.75} \eta ^{0.75}+0.53 \sqrt{1-w_{s}} \sqrt{\gamma } \eta \right) }{(1-w_{s})^2} \phi _{1} dx,\nonumber \\ \end{aligned}$$

(65)

$$\begin{aligned}&{f_{T}}_{m}(t)=-\gamma \beta m^{2} \pi ^{2} \int _{0}^{1}\sin m\pi x \phi _{1} dx,\end{aligned}$$

(66)

$$\begin{aligned}&g_{0}=-\fancyscript{C}\left( t_{0}+t_{2}\right) /2 \quad {g_{1}}=-\fancyscript{C}/2,\nonumber \\&{g_{2}}_{m}=-k\pi ^{2}\left( \fancyscript{L}^{2} m^{2}+1 \right) /2,\end{aligned}$$

(67)

$$\begin{aligned}&{g_{3}}_{m}=\left( \dfrac{\fancyscript{L}^{2}}{12 \gamma } \right) \int _{0}^{1}\phi _{1}^{\prime \prime }\sin m\pi x dx \quad {g_{4}}_{m}=t_{0} {g_{3}}_{m}. \end{aligned}$$

(68)