Tight carbonate gas well deliverability evaluation and reasonable production proration analysis

Abstract

It is very important to accurately predict the gas well productivity and reasonably allocate the gas production at the early development stage of gas reservoirs. However, both the non-Darcy and stress sensitivity effects have not been investigated in dual-porosity model of tight carbonate gas reservoirs. This paper proposed a new dual-porosity binomial deliverability model and single-well production proration numerical model, which consider the effects of non-Darcy and stress sensitivity. The field gas well deliverability tests data validated the accuracy of the new analytical model, which is a very helpful deliverability method when lacking deliverability test. A geological model was built on the results of the well log, well testing, and well production analysis. Then, a reasonable production proration analysis was conducted based on history matched single-well numerical model. The gas productivity index curve and production–prediction of MX22 several simulation cases were adopted to analyze the reasonable production proration. The results indicate that 1/6 may be suitable for high productivity gas well proration. In addition, the absolute open flow rate from the numerical simulation is higher than that from the new deliverability equation, which also shows that the pressure transient analysis sometimes has some deviation in formation property prediction. It is suggested comprehensively utilizing the analytical binomial model and the single-well numerical model in tight carbonate gas well deliverability evaluation.

Introduction

The development of tight carbonate gas reservoirs usually goes through three stages: early production building, middle stable yield, and late production decline. In early production stage, gas well deliverability evaluation and reasonable production proration analysis play an important role in reservoir production management (Jia, 2017). The tight carbonate Gaoshiti-Moxi gas reservoir, which is located in the middle of the Sichuan Basin, is just at the early production building stage (Wei, 2015). For carbonate gas reservoirs, complex natural fractures intensify the formation heterogeneity. The reservoir characteristics make it difficult to accurately describe the gas flow of tight carbonate (Feng et al. 2019; Tan et al. 2021).

Both the non-Darcy and stress sensitivity effects are key in evaluating tight carbonate gas well productivity. Owing to the non-Darcy effect, the productivity index may be reduced up to 20% in a high rate gas well (Smith et al. 2004). The non-Darcy effect is still obvious even for low flow rate in the tight fractured reservoir or unconventional reservoir (Pereira et al. 2006). The stress sensitivity effect can also decrease the fracture permeability as the tight gas reservoir is depleting (Cho et al. 2013). It can not only affect the pressure transient analysis (Pedrosa Jr, 1986) but also damage the well productivity in sandstone or shale gas reservoirs (Deng et al. 2013; Yang et al. 2008).

The gas well deliverability equation is often used in productivity evaluation. Houpeurt (1959) proposed a binomial deliverability equation that has been widely used in field deliverability tests. A novel binomial deliverability equation was presented for fractured gas well considering the non-Darcy effect (Wang et al. 2014). Several deliverability models have also been built to consider the stress sensitivity effect (Deng et al. 2013; Yang et al. 2008). In addition, a production–prediction model for CBM wells was presented considering the effect of pressure-propagation behavior (Sun et al. 2019). However, these gas deliverability studies are single porosity models. Few studies incorporate the effects of non-Darcy and stress sensitivity into dual-porosity naturally fractured reservoirs (Brohi et al. 2011). Some dual-porosity models were proposed to predict well rate behavior (Olarewaju and Lee, 1991; Ramirez et al. 2007; Xue et al. 2020). The Laplace solutions to the semi-analytical models were also presented to predict the vertical or deviated well performance in composite stress-sensitive carbonate gas reservoirs (Zhang et al. 2015; Wang et al. 2017; Meng, 2018; Shi et al. 2018). Nevertheless, it is not convenient to calculate the absolute open flow rate in the field application.

There are several methods developed for gas well reasonable production proration, such as empirical allocation method, productivity index curve method, node analysis method, and numerical simulation method (Zonglin et al. 2006;). The empirical allocation method usually allocates the gas well production according to 1/3 ~ 1/6 of the absolute open flow (AOF) rate. The production index curve method considers the turning point from linear trend due to the non-Darcy effect (Lyons and Plisga, 2011). The node analysis method pays more attention to the gas lifting parameters. The numerical simulation method is the most accurate approach for gas well reasonable production proration (Lu et al. 2017). However, the accuracy of numerical simulation relies on the quality of geologic models and production data. Natural fracture modeling is a challenging task for carbonate reservoirs (Nelson, 2001; Benetatos et al. 2019). Although the multi-porosity model can be used to simulation the carbonate reservoir, dual-porosity model is currently the most popular model that considers the stress sensitivity effect (Chaudhri, 2012; Hinkley et al. 2013).

Few wells of Gaoshiti-Moxi gas reservoir have been conducted deliverability test due to the high cost for ultra-deep wells (> 5000 m). The conventional single-point test is not applicable to tight carbonate gas wells (Lei et al. 2017). However, almost all the wells have implemented pressure build-up tests after a period of early production. Hence, transient pressure analysis results can be directly adopted to evaluate gas well deliverability (Rafi and Neil, 1996). In addition, there are not enough data to build the field geologic model to run simulation cases to analyze the proper well production proration. However, the well log, well pressure build-up test, and well production data are enough to build a single-well numerical model to conduct well production proration analysis.

In this paper, a new dual-porosity binomial deliverability model was proposed to consider the effects of non-Darcy and stress sensitivity. The field gas well deliverability test data were used to validate the accuracy of the new analytical model. Then, a single-well numerical model was built on the results of logging, well testing, and production analysis. After the history match, several simulation cases are adopted to analyze the reasonable production proration. Finally, we point out the implications of the new deliverability model and single-well model, and some research points to be conducted in future field development.

Tight carbonate gas well deliverability evaluation

Conventional binomial deliverability equation

The typical oil flow follows linear Darcy law, while the gas flow follows the nonlinear Forchheimer law that results from the high-velocity inertial effect. The motion equation with a second proportionality constant could truly describe the high-velocity gas flow (Forchheimer, 1901):

$$\frac{{dP}}{{dx}} = av + bv^{2}$$

(1)

where $v$ is the gas velocity and $P$ is the formation pressure.

A more detailed equation with inertial effect is (Cornell and Katz, 1953)

$$\frac{{dP}}{{dx}} = \frac{\mu }{K}v + \beta \rho v^{2}$$

(2)

where $\mu$ is the gas viscosity, $K$ is the formation permeability, $\rho$ is the gas density, $\beta$ is the non-Darcy coefficient.

Based on the above motion equation, a binominal deliverability equation was proposed for vertical gas well productivity evaluation considering the inertial effects around the wellbore (Houpeurt, 1959).

$$P_{e}^{2} - P_{{wf}}^{2} = Aq_{{sc}} + Bq_{{sc}}^{2}$$

(3)

where $P_{e}$ is the outer boundary pressure, $P_{{wf}}$ is the bottom hole pressure, $A$ is the linear coefficient, $A = \frac{{T\mu Z}}{{Kh}}\left( {\ln \frac{{r_{e} }}{{r_{w} }} + S} \right)$, $B$ is the nonlinear coefficient, $B = \frac{{\beta \gamma _{g} ZT}}{{h^{2} }}\left( {\frac{1}{{r_{w} }} - \frac{1}{{r_{e} }}} \right)$.

This conventional binomial deliverability equation was widely used for conventional gas reservoir well productivity calculation. The coefficients A and B are usually obtained by linear matching for the rearranged equation, and then, the absolute open flow rate is calculated by

$$q_{{AOF}} = \frac{{ - A + \sqrt {A^{2} - 4B\left( {P_{e} - 0.101} \right)} }}{{2B}}$$

(4)

Dual-porosity binomial deliverability equation

The conventional binomial deliverability equation is obtained for a single porosity reservoir, and it is not suitable for dual-porosity reservoirs such as carbonate gas reservoirs. Firstly, the non-Darcy coefficient correlation was different for tight carbonate gas reservoir. Here a regressed equation was adopted for the Gaoshiti-Moxi gas reservoir (Shusheng et al. 2015).

$$\beta {\text{ = }}\frac{{7.0 \times 10^{9} }}{{K^{{1.42}} }}$$

(5)

Secondarily, the stress sensitivity effect is also important for tight carbonate gas reservoirs. Here a matched fracture dynamic permeability equation was used to describe this effect (Shusheng et al. 2015). They proposed a stress-sensitive relationship against crack-type carbonate reservoirs. In their study, the stress-sensitive experiment of typical rock samples of tight carbonate reservoirs was obtained, and three carbonate rock stresses were obtained. To facilitate the application, the stress-sensitive curve is normalized, thereby obtaining a mathematical model of the stress sensitivity of crack-type carbonate rock gas reservoir stress:

$$K_{f} = K_{{fi}} \left( {\frac{{P_{{ob}} - P}}{{P_{{ob}} - P_{i} }}} \right)^{{ - \gamma }}$$

(6)

where $K_{{fi}}$ is the initial fracture permeability, $P_{i}$ is the initial formation pressure, $P_{{ob}}$ is the overburden pressure, $\gamma$ is the stress sensitivity coefficient, in this case, $\gamma$ equals 0.6.

A modified pseudo-pressure for stress sensitivity is defined as

$$m_{f} ^{ * } = 2\int_{0}^{P} {\frac{P}{{\mu Z}}} \left( {\frac{{P_{{ob}} - P}}{{P_{{ob}} - P_{i} }}} \right)^{{ - \gamma }} dP$$

(7)

The gas radial flow rate is calculated as

$$v = \frac{{Q_{{sc}} }}{{2\pi rh}}\frac{{ZP_{{sc}} T}}{{PT_{{sc}} }}$$

(8)

where $Z$ is the gas deviation factor, $P_{{sc}}$ is the pressure at the surface condition, $T_{{sc}}$ is the temperature at the surface condition, $P$ is the formation pressure, and $T$ is the formation temperature.

The gas density under reservoir condition is obtained by

$$\rho _{g} = \frac{{T_{{sc}} \rho _{{gsc}} }}{{P_{{sc}} }} \cdot \frac{P}{{TZ}}$$

(9)

where $\rho _{{gsc}}$ is the gas density at surface normal condition.

Usually $\phi _{f} \ll \phi _{m}$, $K_{m} \ll K_{f}$, dual-porosity governing equation is

$$\left\{ {\begin{array}{*{20}c} { - \frac{1}{r}\frac{\partial }{{\partial r}}\left( {r\rho _{g} \delta \frac{{K_{f} }}{\mu }\frac{{\partial P_{f} }}{{\partial r}}} \right) + \frac{{\rho _{g} \alpha K_{m} }}{\mu }\left( {P_{m} - P_{f} } \right) = 0} \\ { - \frac{{\rho _{g} \alpha K_{m} }}{\mu }\left( {P_{m} - P_{f} } \right) = \rho _{g} \phi _{m} C_{m} \frac{{\partial P_{m} }}{{\partial t}}} \\ \end{array} } \right.$$

(10)

where r is the radius of the reservoir, δ is the turbulence parameter, $\delta {\text{ = }}\frac{1}{{1 + \frac{{\beta \rho K_{f} v}}{\mu }}}$, Ф_m is the matrix porosity, C_m is the matrix compression coefficient, the subscript m represents the matrix system, subscript f represents the fracture system.

Combining Eq. (2) and Eq. (5) ~ (10), a new dual-porosity binomial deliverability equation is obtained.

$$m^{*} \left( {P_{{fe}} } \right) - m^{*} \left( {P_{{fw}} } \right){\text{ = }}A \cdot Q_{{sc}} + B \cdot Q_{{sc}}^{2}$$

(11)

where $A = D\left( {\ln \frac{{r_{e} }}{{r_{w} }}{\text{ + }}S} \right) - 2EG\left( {r_{e} - r_{w} } \right) + \frac{E}{2}\left( {r_{e}^{2} - r_{w}^{2} } \right)$

$$\begin{gathered} B{\text{ = }}\frac{{D^{2} }}{{2F}}\left( {\frac{1}{{r_{w} }} - \frac{1}{{r_{e} }}} \right) - \frac{{2DEG}}{F}\ln \frac{{r_{e} }}{{r_{w} }} + \left( {\frac{{DE}}{{4F}} + \frac{{2E^{2} G^{2} }}{F}} \right)\left( {r_{e} - r_{w} } \right) \hfill \\ \;\;\;\; - \frac{{E^{2} G}}{F}\left( {r_{e}^{2} - r_{w}^{2} } \right) + \frac{{E^{2} }}{{6F}}\left( {r_{e}^{3} - r_{w}^{3} } \right) \hfill \\ \end{gathered}$$

$D = \frac{{P_{{sc}} T}}{{\pi K_{{fi}} hT_{{sc}} }}\left[ {1 - \frac{{\left( {1 - \omega } \right)r_{w}^{2} }}{{r_{e}^{2} }}\left( {1 - \frac{{2\overline{\delta } }}{\lambda }\ln r_{w} } \right)} \right]$, $\omega$ is the elastic storativity ratio.

$$E = \frac{{TP_{{sc}} \left( {1 - \omega } \right)}}{{\pi K_{{fi}} \left( {r_{e}^{2} - r_{w}^{2} } \right)hT_{{sc}} }}$$

$$F \approx \frac{{\overline{\mu } P_{{sc}} T}}{{7.0 \times 10^{9} T_{{sc}} \rho _{{gsc}} K_{{fi}}^{{0.58}} }}\left( {\frac{{P_{{ob}} {\text{ - }}\overline{{P_{f} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }}$$

$G = \left( {{{r_{w}^{2} } \mathord{\left/ {\vphantom {{r_{w}^{2} } \lambda }} \right. \kern-\nulldelimiterspace} \lambda }} \right)\overline{\delta } \frac{{\ln \bar{r}}}{{\bar{r}}}$, $\lambda$ is the inter porosity flow parameter, $\overline{\delta }$ is the average turbulence at the average radius, $\overline{\delta } {\text{ = }}\frac{1}{{1 + \frac{{3.5 \times 10^{9} \rho _{{gsc}} Q_{{sc}} }}{{\overline{\mu } \pi hK_{{fi}}^{{0.42}} \left( {\frac{{P_{{ob}} - \overline{{P_{f} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }} }}\frac{1}{{\overline{r} }}}}$.

Then, the absolute open flow can be calculated as

$$Q_{{AOF}} = \frac{{ - A + \sqrt {A^{2} + 4B\left[ {m^{*} \left( {P_{{fe}} } \right) - m^{*} \left( {0.101} \right)} \right]} }}{{2B}}$$

(12)

If the reservoir is a two-area composite reservoir, the deliverability equation becomes

$$m^{ * } \left( {P_{{fe}} } \right) - m^{ * } \left( {P_{{fw}} } \right){\text{ = }}A \cdot Q_{{sc}} + B \cdot Q_{{sc}}^{2}$$

(13)

where$A = A_{1} + A_{2}$, $B = B_{1} + B_{2}$

$$A_{1} {\text{ = }}D_{1} \left( {\ln \frac{{r_{1} }}{{r_{w} }} + S} \right) - 2E_{1} G_{1} \left( {r_{1} - r_{w} } \right) + \frac{{E_{1} }}{2}\left( {r_{1}^{2} - r_{w}^{2} } \right)$$

$$A_{2} {\text{ = }}D_{2} \ln \frac{{r_{e} }}{{r_{1} }} - 2E_{2} G_{2} \left( {r_{e} - r_{1} } \right) + \frac{{E_{2} }}{2}\left( {r_{e}^{2} - r_{1}^{2} } \right)$$

$$\begin{aligned} B_{1} & = \frac{{D_{1} ^{2} }}{{2F_{1} }}\left( {\frac{1}{{r_{w} }} - \frac{1}{{r_{1} }}} \right) - \frac{{2D_{1} E_{1} G_{1} }}{{F_{1} }}\ln \frac{{r_{1} }}{{r_{w} }} \\ &\quad+ \left( {\frac{{D_{1} E_{1} }}{{4F_{1} }} + \frac{{2E_{1} ^{2} G_{1} ^{2} }}{{F_{1} }}} \right)\left( {r_{1} - r_{w} } \right) \hfill \\&\quad - \frac{{E_{1} ^{2} G_{1} }}{{F_{1} }}\left( {r_{1}^{2} - r_{w}^{2} } \right) + \frac{{E_{1} ^{2} }}{{6F_{1} }}\left( {r_{1}^{3} - r_{w}^{3} } \right) \hfill \\ \end{aligned}$$

$$\begin{aligned} B_{2} &= \frac{{D_{2} ^{2} }}{{2F_{2} }}\left( {\frac{1}{{r_{1} }} - \frac{1}{{r_{e} }}} \right) - \frac{{2D_{2} E_{2} G_{2} }}{{F_{2} }}\ln \frac{{r_{e} }}{{r_{1} }} \\ &\quad+ \left( {\frac{{D_{2} E_{2} }}{{4F_{2} }} + \frac{{2E_{2} ^{2} G_{2} ^{2} }}{{F_{2} }}} \right)\left( {r_{e} - r_{1} } \right) \hfill \\ &\quad - \frac{{E_{2} ^{2} G_{2} }}{{F_{2} }}\left( {r_{e}^{2} - r_{1}^{2} } \right) + \frac{{E_{2} ^{2} }}{{6F_{2} }}\left( {r_{e}^{3} - r_{1}^{3} } \right) \hfill \\ \end{aligned}$$

$$D_{1} = \frac{{P_{{sc}} T}}{{\pi K_{{f1i}} hT_{{sc}} }}\left[ {1 - \frac{{\left( {1 - \omega _{1} } \right)r_{w}^{2} }}{{r_{1}^{2} }}\left( {1 - \frac{{2\overline{{\delta _{1} }} }}{{\lambda _{1} }}\ln r_{w} } \right)} \right]$$

$$D_{2} = \frac{{P_{{sc}} T}}{{\pi K_{{f2i}} hT_{{sc}} }}\left[ {1 - \frac{{\left( {1 - \omega _{2} } \right)r_{1}^{2} }}{{r_{e}^{2} }}\left( {1 - \frac{{2\overline{{\delta _{2} }} }}{{\lambda _{2} }}\ln r_{1} } \right)} \right]$$

$$\overline{{\delta _{1} }} {\text{ = }}\frac{1}{{1 + \frac{{3.5 \times 10^{9} \rho _{{gsc}} Q_{{sc}} }}{{\overline{\mu } _{1} \pi hK_{{f1i}}^{{0.42}} \left( {\frac{{P_{{ob}} - \overline{{P_{{f_{1} }} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }} }}\frac{1}{{\overline{{r_{1} }} }}}}$$

$$\overline{{\delta _{2} }} {\text{ = }}\frac{1}{{1 + \frac{{3.5 \times 10^{9} \rho _{{gsc}} Q_{{sc}} }}{{\overline{\mu } _{2} \pi hK_{{f2i}}^{{0.42}} \left( {\frac{{P_{{ob}} - \overline{{P_{{f2}} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }} }}\frac{1}{{\overline{{r_{2} }} }}}}$$

$$E_{1} = \frac{{TP_{{sc}} \left( {1 - \omega _{1} } \right)}}{{\pi K_{{f1i}} \left( {r_{1}^{2} - r_{w}^{2} } \right)hT_{{sc}} }}$$

$$E_{2} = \frac{{TP_{{sc}} \left( {1 - \omega _{2} } \right)}}{{\pi K_{{f2i}} \left( {r_{e}^{2} - r_{1}^{2} } \right)hT_{{sc}} }}$$

$$F_{1} = \frac{{\overline{{\mu _{1} }} P_{{sc}} T}}{{7.0 \times 10^{9} T_{{sc}} \rho _{{gsc}} K_{{f1i}}^{{0.58}} }}\left( {\frac{{P_{{ob}} - \overline{{P_{{f1}} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }}$$

$$F_{2} = \frac{{\overline{{\mu _{2} }} P_{{sc}} T}}{{7.0 \times 10^{9} T_{{sc}} \rho _{{gsc}} K_{{f2i}}^{{0.58}} }}\left( {\frac{{P_{{ob}} - \overline{{P_{{f2}} }} }}{{P_{{ob}} - P_{i} }}} \right)^{{ - 0.42\gamma }}$$

$$G_{1} = \left( {{{r_{w}^{2} } \mathord{\left/ {\vphantom {{r_{w}^{2} } {\lambda _{1} }}} \right. \kern-\nulldelimiterspace} {\lambda _{1} }}} \right)\overline{{\delta _{1} }} \frac{{\ln \bar{r}_{1} }}{{\bar{r}_{1} }}$$

$$G_{2} = \left( {{{r_{1}^{2} } \mathord{\left/ {\vphantom {{r_{1}^{2} } {\lambda _{2} }}} \right. \kern-\nulldelimiterspace} {\lambda _{2} }}} \right)\overline{{\delta _{2} }} \frac{{\ln \bar{r}_{2} }}{{\bar{r}_{2} }}$$

.

Tight carbonate gas well deliverability evaluation

Deliverability testing is the most accurate method for well productivity evaluation. It can also be calculated by directly taking the pressure transient analysis results into the deliverability equation. In addition, the single-point method is a widely used empirical equation for well deliverability equation as it only needs one steady test point with the gas flow rate and bottom hole pressure. Here four wells from Gaoshiti-Moxi tight carbonate gas reservoir are chosen  for deliverability evaluation. The gas well deliverability results are shown in Table 1.

Table 1 Absolute open flow rate comparison for different gas well deliverability evaluation methods

Based on the comparison for different gas well deliverability evaluation methods (Table 1), it was found that the Mean Absolute Percentage Error (MAPE) of the deliverability equation is as low as 2.15%, and that of one-point is 4.02% (Fig. 1). The deliverability equation results are close to the deliverability test that shows the new model has high accuracy in gas well deliverability evaluation. It can be also seen that the MAPE of one-point results are distinctly higher than the results of the deliverability equation. That is because the empirical parameter may not be suitable for the fractured carbonate reservoir. The new dual-porosity gas well deliverability model can make a reasonable prediction based on the well build-up test interpretation result, which is a very helpful deliverability method when lacking the deliverability test data.

Fig. 1

MAPE of different deliverability methods

The deliverability test results are close to the actual well productivity that shows the new model has high accuracy in gas well deliverability evaluation. It can be also seen that one-point results are distinctly smaller than the results of the deliverability test. That is because the empirical parameter may not be suitable for the fractured carbonate reservoir. The new dual-porosity gas well deliverability model can make a reasonable prediction based on the well build-up test interpretation result, which is a very helpful deliverability method when lacking the deliverability test data.

Tight carbonate gas well reasonable production proration analysis

At the early stage of gas reservoirs, it is important to set reasonable production proration for each well. After the accurate well deliverability evaluation, the common method is just to use the empirical proportion to guide the gas well production proration. However, there is less theory foundation on how to determine the reasonable proportion parameter. Based on pressure transient analysis, a single-well numerical simulation could accurately describe the well productivity. Here, we take MX22 well as an example to analyze the gas well reasonable production proration.

Tight carbonate single gas well numerical model setup

MX22 is an early development well in Gaoshiti-Moxi tight carbonate gas reservoir. The production water belongs to condensed water and there is very little water production. Therefore, the gas–water two-phase (water stays under irreducible water state) dual-porosity model is chosen for a single-well simulation study. The model grid dimension is 74 × 74 × 42. The grid size is 33.81 m in the horizontal direction. According to the transient pressure analysis, the inner stimulation radius is 98.47 m and the outer drainage radius is 1412 m. The corresponding square side lengths are 174.53 m for the inner area and 2502 m for the outer area. There are three gas-bearing layers and two interlayers. The permeability of gas-bearing layers comes from well testing, and the porosity of gas-bearing layers comes from the well logging average value. The inner and outer area formation property parameters are shown in Table 2.

Table 2 Inner and outer area formation property parameters

The shape factor can be calculated according to the pressure transient analysis. The shape factor of the inner area is

$$\alpha _{1} {\text{ = }}\frac{{\lambda _{1} }}{{r_{w}^{2} }}\frac{{K_{{f1i}} }}{{K_{{m1i}} }} \approx 184.4$$

(14)

The shape factor of the outer area is

$$\alpha _{2} {\text{ = }}\frac{{\lambda _{2} }}{{r_{w}^{2} }}\frac{{K_{{f2i}} }}{{K_{{m1i}} }} \approx 0.0466$$

(15)

The gas component data come from GS3 well gas sample analysis for the same formation as shown in Table 3.

Table 3 Gas component analysis of GS3 well

The formation temperature is 152.62 °C. The gas formation volume factor and viscosity are shown in Table 4 under different formation pressure.

Table 4 Gas PVT under different pressures

According to the MDT water sample analysis from MX22, the water density is 1.0687 g/cm³. The water formation volume factor is 1.006 under reference pressure 27.85 MPa. In addition, the water compressibility coefficient is 4.65 × 10^–4 MPa⁻¹, and the water viscosity is 0.25 mPa•s under reservoir conditions. The rock compressibility coefficient is 1.62 × 10^–4 MPa⁻¹ under 27.85 MPa. In order to consider the stress sensitivity, a permeability multiplying factor table is achieved by using Eq. (6) with overburden pressure 120 MPa and initial formation pressure 60 MPa (Table 5).

Table 5 Permeability multiplying factor under different pressures

The relative permeability curves come from MX13 well for the same formation core test, which is shown in Fig. 2.

Fig. 2

Gas–water two-phase relative permeability curves from MX13 well core test

The equilibrium initialization is adopted to get the reservoir reserve. The single-well reservoir reserve is 60.33 × 10⁸ m³, while the single-well control reserve is only 30.7 × 10⁸ m³ from gas rate dynamic analysis. Hence, the pore volume is multiplied by 0.5089 to match the dynamic reserve. The geologic model of MX22 is shown in Fig. 3.

Fig. 3

MX22 single-well geologic model: a fracture permeability of the inner area and the outer area; b initial matrix gas saturation distribution

Tight carbonate single gas well history match

The three gas-bearing layers are perforated for Well MX22. Because of very little water production, MX22 can be seen as a single-phase production vertical well. The well history data have the tubing head pressure, so a VFP table is needed to calculate the vertical tubing flow pressure drop. The VFP table is generated by using the VFPi module for dry gas flow. The tubing inner diameter is 74.6 mm. The tubing of well MX22 is sulfate resistant as there is a hydrogen sulfide component. The tubing roughness is 0.0016 mm, which is much less than that of common tubing. The well bottom hole temperature is 153.51 ℃, and the wellhead temperature is 60.2 ℃. The formation pressure is 60.4 MPa at the middle gas-bearing layer depth 5453.30 m.

The well MX22 is producing at a constant gas rate, and the formation parameters are adjusted to match the tubing head pressure in the history match process. The tubing head pressure well-matched result is shown in Fig. 3. The inner area permeability is 6.426 mD and the outer area permeability is 0.378 mD after the history match adjusting procedure. The adjusted model is then used for reasonable production proration analysis.

Tight carbonate single gas well reasonable production proration analysis

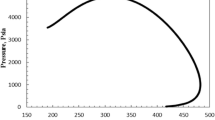
Based on the history matched single-well model, the well MX22 stable gas flow rate is obtained under different constant bottom hole pressure. The inflow performance relationship curve of MX22 is shown in Fig. 4. The absolute open flow is about 184.21 × 10⁴ m³ by extrapolating the inflow performance relationship to the horizontal axis. This can be used for further numerical simulation analysis. In addition, the gas productivity index curve can also be obtained from the simulation results. The horizontal axis is the gas flow rate, while the vertical axis is the square deviation between boundary pressure and bottom hole pressure in the gas productivity index curve. The reasonable gas production rate is the point where the curve deviates from a straight line. The gas productivity index curve of MX22 is illustrated in Fig. 5, and the reasonable gas flow rate is about 26 × 10⁴ m³ as shown in the turning point. The sand production and bottom water are not considered when analyzing the production proration. The other only limiting condition is the lowest gas transportation pressure that is 2 MPa here. The corresponding highest gas flow rate is 123.464 × 10⁴ m³ by conducting a simulation case with a 2 MPa tubing head pressure limit (Fig. 6).

Fig. 4

Tubing head pressure matched result for MX22 well

Fig. 5

Inflow performance relationship curve of MX22 well

Fig. 6

Gas well productivity index curve of MX22 well

In order to find the reasonable proportion of the absolute open flow rate, five cases are designed to compare the stable production period and cumulative gas production. The five proration gas rates are obtained by multiplying the absolute open flow rate by 1/4, 1/5, 1/6, 1/7, and 1/8. The stable production period under different allocating gas rates is illustrated in Fig. 7. It can be seen that the stable period keeps 20 years when the allocating gas flow rate is no bigger than 1/7. The stable period decreases when the proration gas rate increases from 1/6 to 1/4. The cumulative gas flow rate will increase as the allocating gas flow rate increases as is shown in Fig. 8. However, the cumulative gas flow rate increases slower when the gas flow rate proportion ratio is bigger than 1/6.

Fig. 7

Stable production period under different allocating gas rates of MX22 well

Fig. 8

Cumulative gas production under different allocating gas rates of MX22 well

Discussion

The absolute open flow rates of the new gas well deliverability equation are more accurate than those of the single-point method as shown in Table 1. The results of the new deliverability equation are higher than those of the deliverability test for some wells such as MX 108 and MX105. However, the result of the new deliverability equation is lower than that of the deliverability test for GS3. This abnormal phenomenon shows that the new gas well deliverability equation may have some bias in absolute open flow rate evaluation. On the one side, this may result from deliverability test inaccuracy such as abnormal data from instrumental error. On the other side, this may result from the inaccuracy of transient pressure analysis such as unreasonable interpretation. In other words, the new workflow based on the well build-up test interpretation result has two sides. It can make a reasonable prediction when the deliverability test data lacks. The accuracy of the new equation relies on the accuracy of the pressure transient analysis. In addition, the absolute open flow from the numerical simulation is higher than that from the new deliverability equation, which also shows that the pressure transient analysis has some deviation in formation property prediction as the numerical simulation modified the permeability for history match. It is suitable to comprehensively utilize the above methods in tight carbonate gas well deliverability evaluation.

The single-well simulation could predict the cumulative gas production under different allocating gas rates as shown in Fig. 7. The turning point 1/6 (30.70 × 10⁴ m³) may be the reasonable allocation proportion for MX22 which indicates that 1/6 may be suitable for high productivity gas well proration. The stable production period for 1/6 is 210 months that are also acceptable for actual well management. It should be mentioned that the tight carbonate gas reservoir has few sandstone production problems. The water production is not involved at the early stage; however, the bottom or edge water has a huge effect on gas production. Similarly, the stress sensitivity effect has a small influence at the early production stage and a great effect on the well productivity at the later production stage. What is more, the core test stress sensitivity parameters may not suitable for actual well production performance.

The new gas deliverability equation and the single-well numerical simulation may be helpful at the early gas production evaluation and proration. As the production goes on, some research should be suggested to conduct in future. Firstly, the new model for deviated wells or horizontal wells should be studied in future. The vertical wells are commonly used at the early stage; however, deviated or horizontal wells are widely used at the development adjustment stage. Secondarily, edge water or bottom water must be considered in future reservoir management. Actually, there is bottom water under the main gas-bearing layers and the water channeling or invasion will hugely damage the gas well production. Hence, new two-phase deliverability equation and whole field simulation model are needed for water encroachment evaluation and production optimization. At last, the stress sensitivity effect should be carefully evaluated by combing lab core tests and field dynamic data analysis, which should also be considered in the field development plan in future.

Conclusions

• 1. The conventional binomial deliverability equation is not suitable for dual-porosity gas well productivity evaluation. A new dual-porosity binomial deliverability equation was developed with considering both the non-Darcy and the stress sensitivity effects. The field well deliverability calculation cases validated the reasonableness.

• 2. The empirical one-point method may not be suitable for the fractured carbonate gas reservoir deliverability evaluation. The new dual-porosity tight carbonate gas well deliverability model can make a reasonable prediction based on the well build-up test interpretation result, which is a very helpful deliverability method when lacking deliverability test.

• 3. The geological model was built on the results of logging, well testing, and production analysis. A VFP table was generated to consider the pressure drop during the flow in vertical tubing, which should carefully choose the toughness according to the tubing type. A reasonable production proration analysis can be conducted based on history matched single-well model.

• 4. The gas productivity index curve of MX22 was obtained after several simulation cases, and five cases were designed to compare the stable production period and cumulative gas production. The turning point 1/6 (30.70 × 10⁴ m³) may be the reasonable allocation proportion for MX22 which indicates that 1/6 may be suitable for high productivity gas well proration.

Appendix A

The derivation process of the new dual-porosity binomial deliverability equation.

The derivation process from Eq. 11 is shown here.

Using the first equation of Eq. 10, we can obtain differential equation of fracture system

$$C_{0} \xi \frac{\partial }{{\partial t}}\left[ { - \frac{1}{r}\frac{\partial }{{\partial r}}\left( {\delta r\frac{{\partial m_{f} }}{{\partial r}}} \right)} \right] + \frac{{K_{f} }}{\mu }\left[ { - \frac{1}{r}\frac{\partial }{{\partial r}}\left( {\delta r\frac{{\partial m_{f} }}{{\partial r}}} \right)} \right] = C_{0} \frac{{\partial m_{f} }}{{\partial t}}$$

(A.1)

where $C_{0} {\text{ = }}\phi _{m} C_{m}$, $\xi {\text{ = }}\frac{{\alpha K_{f} }}{{K_{m} }}{\text{ = }}\frac{{r_{w}^{2} }}{\lambda }$, $\lambda = \frac{{\alpha r_{w}^{2} K_{m} }}{{K_{i} }}$.

If the crossflow is not large, the fracture control equation can be rewritten as

$$\frac{\xi }{\eta }\frac{\partial }{{\partial r}}\left[ { - \frac{1}{r}\frac{\partial }{{\partial r}}\left( {r\delta \frac{{\partial m_{f} }}{{\partial t}}} \right)} \right] - \frac{1}{r}\frac{\partial }{{\partial r}}\left( {\delta r\frac{{\partial m_{f} }}{{\partial r}}} \right) = \frac{1}{\eta }\frac{{\partial m_{f} }}{{\partial t}}$$

(A.2)

where η is pressure coefficient, $\eta {\text{ = }}\frac{{K_{f} }}{{\mu \phi _{m} C_{m} }}$.

Using the pseudo-pressure function and material balance equation, we can obtain

$$\frac{{\partial m_{f} }}{{\partial t}}{\text{ = }}\frac{{2P_{f} }}{{\mu Z}}\frac{{\partial P_{f} }}{{\partial t}} = - \frac{{2TP_{{sc}} }}{{\left( {\left( {\phi C_{t} } \right)_{f} + \left( {\phi C_{t} } \right)_{m} } \right)\mu \pi \left( {r_{e}^{2} - r_{w}^{2} } \right)hT_{{sc}} }}Q_{{sc}}$$

(A.3)

Using average turbulence coefficient, separation variables and integral, we can obtain

$$r\delta \frac{{\partial m_{f} }}{{\partial r}} = 2EQ_{{sc}} \left( {\frac{{r^{2} }}{2} - \frac{{r_{w}^{2} \overline{\delta } }}{\lambda }\ln r} \right) + C$$

(A.4)

where $E = \frac{{TP_{{sc}} \left( {1 - \omega } \right)}}{{\pi K_{f} \left( {r_{e}^{2} - r_{w}^{2} } \right)hT_{{sc}} }}$, C is integration constant.

Using the inner boundary conditions, we can obtain

$$C = \frac{{P_{{sc}} T}}{{\pi K_{f} hT_{{sc}} }}\left[ {1 - \frac{{\left( {1 - \omega } \right)r_{w}^{2} }}{{r_{e}^{2} }}\left( {1 - \frac{{2\overline{\delta } }}{\lambda }\ln r_{w} } \right)} \right]Q_{{sc}}$$

(A.5)

Using internal boundary conditions and the definition of the seepage speed, we can obtain

$$\begin{aligned} \frac{{\partial m_{f} }}{{\partial r}}& = \frac{{D^{2} Q_{{sc}}^{2} }}{{2F}}\frac{1}{{r^{2} }} + \left( {DQ_{{sc}} - \frac{{2DEG}}{F}Q_{{sc}}^{2} } \right)\frac{1}{r} \\ & \quad+ \left( {\frac{{DE}}{{4F}}Q_{{sc}}^{2} - 2EGQ_{{sc}} + \frac{{2E^{2} G^{2} }}{F}Q_{{sc}}^{2} } \right) \hfill \\& \quad + \left( {EQ_{{sc}} - \frac{{2E^{2} G}}{F}Q_{{sc}}^{2} } \right)r + \frac{{E^{2} Q_{{sc}}^{2} }}{{2F}}r^{2} \hfill \\ \end{aligned}$$

(A.6)

where $D = \frac{{P_{{sc}} T}}{{\pi K_{f} hT_{{sc}} }}\left[ {1 - \frac{{\left( {1 - \omega } \right)r_{w}^{2} }}{{r_{e}^{2} }}\left( {1 - \frac{{2\overline{\delta } }}{\lambda }\ln r_{w} } \right)} \right]$, $F = \frac{{\mu P_{f} }}{{K_{f}^{2} \beta \rho Z}}$, $G = \left( {{{r_{w}^{2} } \mathord{\left/ {\vphantom {{r_{w}^{2} } \lambda }} \right. \kern-\nulldelimiterspace} \lambda }} \right)\overline{\delta } \frac{{\ln \bar{r}}}{{\bar{r}}}$.

Separating the variables and integral to the above formula, a new dual-porosity binomial deliverability equation is obtained as

$$m\left( {P_{{fe}} } \right) - m\left( {P_{{fw}} } \right){\text{ = }}A \cdot Q_{{sc}} + B \cdot Q_{{sc}}^{2}$$

(A.7)