Introduction

Food webs are networks of species linked via trophic interactions that in a simple way describe the biodiversity and feeding relations in ecosystems. To find universal laws that aid in understanding what maintains this biodiversity, several descriptors of food webs have emerged over the past decades, such as link density (the number of links per species; Levins 1977), trophic chain length (e.g. Pimm and Lawton 1977) and lengths and weights of trophic interaction loops (chains of trophic links that start and end with the same species; Levins 1977; Neutel et al. 2002).

The most studied food web descriptor is probably (unweighted) connectance (Gardner and Ashby 1970), which is the proportion of realized links in a food web. It was this food web descriptor that May (1972, 1973) used in his analysis to show that an increase in food web complexity, described in terms of connectance, number of species, and average interaction strength, does not necessarily lead to an increase in stability. Up till then it was considered true that ‘complexity begets stability’, an idea formulated amongst others by MacArthur (1955). The result of May led to a wealth of research that investigated the complexity-stability relation, often using connectance as a derivative of food web complexity (e.g. DeAngelis 1975; Pimm 1979; Martinez 1992; Chen and Cohen 2001; Dunne et al. 2002). Depending on definitions of stability, methods to construct theoretical food webs, or usage of empirical food web data or structures, some studies confirmed the negative relation between food web complexity and stability (Gardner and Ashby 1970; May 1972; Pimm 1979; Chen and Cohen 2001), but others found that highly connected food webs could still be stable (DeAngelis 1975; Haydon 2000; Dunne et al. 2002).

Connectance is a food web property that can be determined from the number of species and number of feeding links. Unweighted food web measures, such as connectance, treat all links as if they are equally important to the food web. However, it is very common in food webs, or even in networks in general (Csermely 2006), that material fluxes associated with the links (i.e. feeding rates) vary considerably in magnitude. To account for these differences in link ‘weights’, weighted food web descriptors have been introduced (Ulanowicz and Wolff 1991; Ulanowicz 1997; Bersier et al. 2002). These descriptors are based on principles from information theory (Shannon 1948) and often use Shannon’s diversity index. They assign more importance to strong links than to weak links and in that way take into account the unequal distribution of link weights in the food web.

Studies that use weighted food web measures focussed mainly on the weighted link density, which is the number of links per species, taking link weights into account (Ulanowicz 1997; Bersier et al. 2002; Banasek-Richter et al. 2009). Using this measure, Ulanowicz (1997, 2002) proposed a weighted equivalent to the complexity-stability criterion of May (1972). Ulanowicz (1997, 2002) showed that the weighted link densities of real food webs complied with the weighted complexity-stability criterion, while those of randomly constructed networks did not.

Instead of using the weighted link density as in the analysis of Ulanowicz (1997, 2002), the relation between food web complexity and stability can also be re-examined by looking at weighted connectance (Bersier et al. 2002; Boit and Gaedke 2014). Weighted connectance is the number of links in the whole web relative to the total number of links, in which each link is weighed on the basis of the flux rate (in case of food webs) associated with the link. Weighted connectance has been used before in food web studies (Bersier et al. 2002; Banasek-Richter et al. 2009; Boit and Gaedke 2014), but not yet in the complexity-stability context.

Investigating the relation between weighted connectance and food web stability is interesting for two reasons. First, there is no clear pattern in how unweighted connectance relates to food web stability, even though this relation has been studied for decades. Studies have shown different possibilities (e.g. Pimm 1979; Haydon 2000; Chen and Cohen 2001; Dunne et al. 2002) and it would be interesting to see what the weighted version of this relation would add to the complexity-stability debate. Second, it is difficult to predict what the relation between weighted connectance (based on patterns in flux rates) and food web stability (based on patterns in interaction strengths) would be. Interaction strengths are the elements of the Jacobian matrix which is calculated from the system of differential equations that describe food web dynamics (May 1972). These strengths are the per capita fluxes in equilibrium between consumers and resources. Thus, the interaction strengths are derived from the material flux rates, but the relation between flux rate and interaction strength is not one to one: a small flux (small link weight) does not necessarily lead to a weak interaction strength (de Ruiter et al. 1995).

Food web theoreticians emphasize the importance of patterns in interaction strengths to food web stability (de Ruiter et al. 1995; McCann et al. 1998; Neutel et al. 2002; McCann 2012). Studies in which the interaction strengths are derived from empirical information show patterns that are important to stability. McCann et al. (1998) linked food web stability to the occurrence of a few strong links embedded in a majority of weak links. Emmerson and Yearsley (2004) found that the probability of a food web to be stable is larger for interaction strength distributions that are skewed towards weak interaction strengths. De Ruiter et al. (1995) found that the patterning of the interaction strengths is trophic level dependent, and the stabilising effect of such patterns can be understood from its prevention of destabilizing strong (‘heavy’) trophic interaction loops (Neutel et al. 2002).

Different expectations can be formulated on the relation between weighted connectance and stability. From a ‘complexity begets stability’ point of view (MacArthur 1955), we would expect that an increase in weighted connectance (as a measure of complexity) would lead to an increase in food web stability. Boit and Gaedke (2014) found that weighted connectance increased during succession and hypothesized that this could “imply an insurance effect enhancing response diversity and robustness against disturbances” (p. 19). However, we could also reason that an increase in weighted connectance might be destabilizing for the food web. An increase in weighted connectance reflects a more even distribution of the fluxes (Ulanowicz 2002; Boit and Gaedke 2014). A more even distribution of fluxes could result in a more even distribution in interaction strengths (which is not necessarily the case, as mentioned above). The loss in skew towards weak interaction strengths could mean a loss of stabilizing interaction strength patterns, because these skewed distributions are associated with stable food webs (McCann et al. 1998; Emmerson and Yearsley 2004). But it might also be possible that a more even distribution in interaction strengths implies that extremely strong links become less strong and in that way prevent destabilizing heavy trophic interaction loops (Neutel et al. 2002).

In this study, we will revisit the relation between complexity and food web stability for 15 real soil food webs (Hendrix et al. 1986; Hunt et al. 1987; Andrén et al. 1990; de Ruiter et al. 1993; Neutel et al. 2007).We used the soil food web data to determine the fluxes in terms of the amount of biomass that is transferred from one trophic group to another. Subsequently, we used these fluxes to calculate weighted connectance. Furthermore, these fluxes were used to calculate the interaction strengths and the interaction strength matrices (i.e. the Jacobian matrices, see Methods), cf. de Ruiter et al. (1995), from which we determined food web stability with the diagonal strength metric, s. This measure was introduced by Neutel et al. (2002) and represents the minimal amount of self-damping needed for stability. Thus, similar to the approach of May (1972), we determined food web stability and connectance, with the difference that in our study, food web stability and connectance were based on distributions of fluxes and interactions strengths that were derived from empirical data. To see how the skewness of the distributions of fluxes and interaction strengths was related to weighted connectance and food web stability, we determined skewness with the Gini coefficient (Gini 1912).

Methods

Food web data and fluxes

We used data of 15 real soil food webs, which have been sampled before and described in a number of publications (Hendrix et al. 1986; Hunt et al. 1987; Andrén et al. 1990; de Ruiter et al. 1993; Neutel et al. 2007). Four food webs were sampled on the island of Schiermonnikoog, The Netherlands, and represent a chronosequence of primary succession (Neutel et al. 2007). Each web was originally represented by four food web replications (Neutel et al. 2007), but these were averaged here. Another four food webs (each web averaged over four replicates) were sampled from Hulshorsterzand, The Netherlands, and also represent a chronosequence of primary succession (Neutel et al. 2007). Again, each web was originally represented by four food web replications (Neutel et al. 2007) , but these were averaged here. Three sites (Horseshoe bend in Georgia, USA, Hendrix et al. 1986; Kjettslinge in Uppsala, Sweden, Andrén et al. 1990; and Lovinkhoeve in Marknesse, The Netherlands, de Ruiter et al. 1993) consisted of two treatments (agricultural management practices) and for each treatment the food webs were established. Finally, the present data-set also included the soil food web from the native prairie of the Central plains experimental range (Colorado, USA, Hunt et al. 1987).

Functionally similar species of the food webs were aggregated in groups (Hendrix et al. 1986; Moore et al. 1988; Andrén et al. 1990). The number of trophic groups varied between 12 and 19 (see Table A1). These trophic groups form the nodes of the food web, and the links between the groups represent the feeding rates or fluxes of biomass F ij (kg C ha−1 yr−1) from resources j to consumers i. These fluxes were constructed via mass balancing (O’Neill 1969; Hunt et al. 1987; de Ruiter et al. 1993) by using the measured yearly-averaged biomasses of the trophic groups (see Table A1), which were assumed to represent equilibrium biomasses B i , and values from the literature for biomass conversion efficiencies e i , feeding preferences w i , and death rates d i (de Ruiter et al. 1993 and references therein; Neutel et al. 2007).

Food web stability

To determine food web stability, Jacobian matrices, or interaction strength matrices (May 1972), were constructed from the system of generalized Lotka-Volterra differential equations that describe for each food web its dynamics, cf. de Ruiter et al. (1995) and Neutel et al. (2007). The off-diagonal elements, α ij , or the interspecific interaction strengths, represent the per capita effects of species j (i.e. trophic group j) on species i. The effects of consumers j on resources i are given by \( {\alpha}_{ij}=-\frac{F_{ij}}{B_j} \), and the effects of resources i on consumers j are given by \( {\alpha}_{ji}=\frac{e_j{F}_{ij}}{B_i} \). Non-assimilated biomass was returned to the detritus pool, leading to positive interaction strengths, α Di , of species i on detritus D (see also Supplementary Information of Neutel et al. 2007).

The diagonal elements, α ii , were used to quantify food web stability, cf. Neutel et al. (2002). They were defined as: α ii  = − sd i , where s represents the fraction of deaths caused by density dependence (Neutel et al. 2002). We used s as a measure for stability, which was defined by Neutel et al. (2002) as the minimum value needed for the interaction strength matrix to be stable, i.e. it is the value where the maximum real part of all eigenvalues is equal to zero. The lower the value of s, the ‘more stable’ the food web is, in the sense that the food web requires less self-damping to remain stable. The diagonal value of detritus, α DD , can be determined directly from the system of differential equations (see Supplementary Information of Neutel et al. 2007). This method only works if the original interaction strength matrices, with diagonal elements α ii  = 0 for i ≠ D, are unstable, which was the case for our 15 food webs.

Connectance, topological and effective connectance per node, and weighted connectance

We determined for each food web unweighted connectance, C, as the number of realized links, L, divided by the total number of possible links, S 2 (Martinez 1991), with S the number of trophic groups in the food web.

We followed the approach of Boit and Gaedke (2014) to determine weighted connectance, which is based on the information theory metrics of Ulanowicz (1997). First, Shannon’s formula is used to describe the diversity in flux weights (feeding rates F ij  ≠ 0):

$$ H=-{\displaystyle \sum_{\begin{array}{c}\hfill i,j\hfill \\ {}\hfill i\ne j\hfill \end{array}}}\left(\frac{F_{ij}}{F}\right) \ln \left(\frac{F_{ij}}{F}\right), $$
(1)

where F is the total sum of fluxes, calculated as the sum of all feeding rates, plus fluxes to detritus from each trophic group caused by egestion or mortality. In theory, the summation holds for i = j, but here we exclude this value for i because there are no cannibalistic species in our food webs. Second, the average mutual information A is calculated as:

$$ A={\displaystyle \sum_{\begin{array}{c}\hfill i,j\hfill \\ {}\hfill i\ne j\hfill \end{array}}}\left(\frac{F_{ij}}{F}\right) \ln \left(\frac{F_{ij}F}{{\displaystyle {\sum}_k}{F}_{ik}{\displaystyle {\sum}_m}{F}_{mj}}\right), $$
(2)

These measures are used to calculate the ‘effective connectance per node’, m (Ulanowicz 1997):

$$ m= \exp \left(\frac{H-A}{2}\right). $$
(3)

This is the weighted version of link density. A special case is when all links have equal weights. The resulting m is then denoted by m *, and termed the ‘topological connectance per node’ (Ulanowicz 1997). Because H − A is actually a sum of Shannon indices (Ulanowicz and Wolff 1991), and because the Shannon index is maximal when all links have equal weights (Shannon 1948), m increases when the flux distribution becomes more even. If links have unequal weights, then 1 ≤ m < m* (Ulanowicz and Wolff 1991). The topological connectance per node, m *, is not equal to qualitative link density, L/S (Bersier et al. 2002 use a different weighting of the links, so that their measure of weighted link density is indeed equal to qualitative link density if all links have equal weights).

The criterion of May (1972) states that food webs should be stable if

$$ a<\sqrt{SC}, $$
(4)

where a is the average interaction strength. Ulanowicz (1997) expressed a in terms of m and m *, and stated that stable food webs (with m ≤ m*) should satisfy the following inequality:

$$ m< \exp \left(\frac{3 \ln \left({m}^{\ast}\right)}{2\sqrt{m^{\ast }}}\right). $$
(5)

We tested whether the 15 real soil food webs also comply to this inequality.

Finally, weighted connectance, C w , is obtained by dividing m by the number of trophic groups, S (Boit and Gaedke 2014):

$$ {C}_w=\frac{m}{S}. $$
(6)

Weighted connectance C w thus captures how connected the species in a food web are, taking the distribution of the flux weights into account. A skewed flux distribution towards small fluxes (i.e. many small fluxes, few strong fluxes) results in low values for C w , while a more even flux distribution results in high values for C w . Because m can vary between 1 and m * (Ulanowicz and Wolff 1991), and m * cannot be greater than S (when all species are connected to all species and links have equal link weights), C w can vary between 1/S and 1.

Gini coefficients of fluxes and interaction strengths

Weighted connectance takes the distribution of flux weights into account, but it is also influenced by the number of species and the number of links between species. To determine the skewness (towards weak links) in the distribution of the fluxes and interaction strengths independent of number of species and links, we used the Gini coefficient (Gini 1912). This coefficient is often used in social sciences, mostly to determine the inequality in distribution of income in a society. However, it can be used in other fields as well, including ecology (e.g. Arenas and Fernandez 2000; Wittebolle et al. 2009; Jiang et al. 2013). The Gini coefficient can take values between zero and one, where a (theoretical) value of zero means complete equality (here: all fluxes are of equal size) and a (theoretical) value of one means complete inequality (here: there is only one flux with a non-zero value, all other fluxes are zero). Thus, the higher the value, the more skewed the distribution (towards weak links), i.e. there are only a few links that account for a large proportion of the total sum of fluxes. We used the definition of Sen (1973) to calculate the Gini coefficients for both flux (G F ) and interaction strength (G I ) distributions:

$$ {G}_F=\frac{{\displaystyle {\sum}_{m=1}^S}{\displaystyle {\sum}_{n=1}^S}\left|{F}_m-{F}_n\right|}{2{S}^2{\mu}_F}, $$
(7)
$$ {G}_I=\frac{{\displaystyle {\sum}_{m=1}^S}{\displaystyle {\sum}_{n=1}^S}\left|{\alpha}_m-{\alpha}_n\right|}{2{S}^2{\mu}_I}, $$
(8)

where F m represents a flux, μ F is the mean of all fluxes, α m represents the absolute value of an interaction strength (excluding diagonal values, except the diagonal value for detritus), and μ I is the mean of all absolute values of interaction strengths (excluding diagonal values, except the diagonal value for detritus).

Results

We found no relation between unweighted connectance, C, and food web stability expressed as diagonal strength, s, in the 15 real soil food webs (Spearman rank correlation test: ρ = 0.11, P > 0.5, corrected for 5 ties). There was also no statistically significant relation between the topological connectance per node, m *, and s (Spearman rank correlation test: ρ = 0.93, 0.1 < P < 0.2). The effective connectance per node, m (i.e. weighted link density), and the topological connectance per node, m * (i.e. weighted link density where links have equal weights), of the food webs did satisfy the proposed inequality (5) of Ulanowicz (1997), as shown in Fig. 1. Some food webs had a value of m that was very close to the minimum value of 1 (Fig. 1), that is, these webs had practically one link per node when link weights were taken into account. Both low and high values of stability could occur close to the line m = 1 (Fig. 1).

Fig. 1
figure 1

Topological connectance per node, m *, and the effective connectance per node, m, for the 15 real soil food webs. Only values of m that are below the dotted line m = m * are feasible values. The solid line represents inequality (5). Black dots: s < 0.01; grey squares: 0.01 ≤ s < 0.1; white triangles: s ≥ 0.1. Stability and s are inversely related. Label numbers refer to the 15 soil food webs as follows: (1) Central Plains Experimental Range (Hunt et al. 1987), (2) Horseshoe Bend Experimental Farm Conventional Tillage (CT) and (3) No Tillage (NT) (Hendrix et al. 1986), (4) Kjettslinge Experimental Farm Barley field with no fertilizer (B0) and (5) with fertilizer (B120) (Andrén et al. 1990), (6) Lovinkhoeve Experimental Farm Integrated Farming (Int) and (7) Convential Farming (Con) (de Ruiter et al. 1993), (8)-(11) Schiermonnikoog Primary Succession Stages 1–4 (Neutel et al. 2007), (12)-(15) Hulsthogerzand Primary Succession Stages 1–4 (Neutel et al. 2007)

Full size image

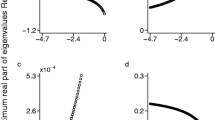
When connectance was based on the feeding rates, F ij , this weighted connectance, C w , was negatively correlated with s (Spearman rank correlation test: ρ = −0.83, P < 0.001; Fig. 2), i.e. positively correlated with food web stability. Thus, where unweighted connectance and stability did not show any relation at all, the relation between weighted connectance and stability showed an opposite relation from what May (1972) found. The values for weighted connectance (range of C w between 0.07 and 0.12, median = 0.08) were much lower than the values for unweighted connectance (range of C between 0.16 and 0.23, median = 0.18), implying that the flux distributions of the food webs showed skewed distributions of many links with small values and only a few links with large values. Figure 2 shows that diagonal strength, s, decreased very rapidly for increasing C w .

Fig. 2
figure 2

Weighted connectance, C w , and food web stability expressed as diagonal strength, s, for the 15 real soil food webs. Stability and s are inversely related. Label numbers refer to the 15 soil food webs, see legend of Fig. 1

Full size image

The Gini coefficient that we used to express the skewness in the distributions of fluxes, G F , was negatively correlated with weighted connectance (Spearman rank correlation test: ρ = −0.90, P < 0.001; Fig. 3). This is to be expected as weighted connectance, amongst others, takes the skew in distributions into account: the more skewed the data, the lower weighted connectance. The Gini coefficient showed that the flux distributions of the food webs were highly skewed towards small fluxes (see also Table A2), because the Gini coefficients of the fluxes for the 15 investigated food webs took values between 0.86 and 0.97 (median = 0.94).

Fig. 3
figure 3

Skewness of fluxes expressed as Gini coefficient, G F , and weighted connectance, C w , for the 15 real soil food webs. Label numbers refer to the 15 soil food webs, see legend of Fig. 1

Full size image

We also found that the Gini coefficient of the fluxes was positively correlated with the Gini coefficient of the per capita interaction strengths, G I (Spearman rank correlation test: ρ = 0.58, P = 0.03). The Gini coefficients of these interaction strength distributions were lower (median = 0.77, range = 0.64–0.86) than the Gini coefficients of the flux distributions, but still relatively high and indicated that the interaction strength distributions were also highly skewed towards weak interactions (see also Table A3).

The Gini coefficients of both the interaction strengths and the fluxes were positively correlated with s (Spearman rank correlation test for G I and stability: ρ = 0.60, P = 0.02, Fig. 4; Spearman rank correlation test for G F and stability: ρ = 0.78, P < 0.001), i.e. negatively correlated with food web stability. Thus, the relations between the Gini coefficients and stability confirm that the higher the skewness of the distribution of either the fluxes or the interaction strengths towards weak links, the lower food web stability.

Fig. 4
figure 4

Skewness of interaction strengths expressed as Gini coefficient, G I , and food web stability expressed as diagonal strength, s, for the 15 real soil food webs. Stability and s are inversely related. Label numbers refer to the 15 soil food webs, see legend of Fig. 1

Full size image

Discussion

The relation between food web connectance and food web stability has been extensively studied on a wide variety of ecosystems, using empirical and theoretical approaches. The outcomes of these studies gave a scattered picture: some found a positive relation between connectance and stability, others found a negative relation, and some found no relation. In our study, we restricted to soil food webs that were constructed using similar methods (Bersier et al. 2002; Dunne et al. 2002), and in which fluxes and interaction strengths were calculated using the same models as in previous studies (Pimm 1979; Neutel et al. 2007). For these food webs, we did not see any clear pattern of how unweighted connectance relates to food web stability. But we did find a clear positive correlation between weighted connectance and stability. We also found that the more skewed the distribution of fluxes or interaction strengths was towards small fluxes or weak interactions, the less stable the food web was.

When food web complexity is expressed in terms of weighted connectance, our results confirm the notion of ‘complexity begets stability’ (MacArthur 1955), and our results challenge the notion of May (1972) that connectance constrains stability. In the approach of May, unweighted connectance was not dependent on the flux distribution, and food web stability was based on matrices of which the elements were drawn randomly from normal distributions. In real food webs, however, fluxes are not evenly distributed, and distributions of interaction strengths are skewed towards weak interactions (Paine 1980; de Ruiter et al. 1995; McCann et al. 1998; Emmerson and Yearsley 2004; Neutel et al. 2007). The distributions of both our fluxes and interaction strengths were skewed, and this empirical information was used when we calculated both weighted connectance and food web stability. Thus, although we adhered to the approach of May (1972), differences in outcomes can be expected.

Studies on the same soil food webs (Neutel et al. 2002, 2007) have shown that there is no relation between food web complexity and stability. In these studies, food web stability was based on empirically derived interaction strengths, while complexity was based on number of species and unweighted connectance. In our study, when both stability and connectance are based on observations, a positive relation between complexity (expressed as weighted connectance) and stability emerges. Testing this relation for food webs from other biomes could show whether this result can be generalized.

The present results evoke the question of why there is a positive relation between weighted connectance and stability. The analysis with the Gini coefficients showed that the more skewed the distribution of either fluxes or interaction strengths were (many weak links, few strong links), the more stable the food web was. Thus, skewed distributions towards weak links seem to be the driving force behind this positive relation. Neutel et al. (2002; 2007) used the same soil food webs and found a negative relation between the maximum loop weight and stability, which together with the present results implies a negative relation between weighted connectance and maximum loop weight. A more even distribution of feeding rates could then prevent heavy loops. However, the precise nature of this relation is not yet clear, as we did not find any relation between the evenness of feeding rates and that of interaction strength.

Interesting here is that we find that very skewed distributions towards weak links are associated with the less stable food webs, while food web theoreticians find that skewed distributions towards weak interactions are associated with stability. Thus, it might be that there is an optimum in skewness. Ulanowicz (1997) also suggested that an extremely skewed link (here, flux) weight distribution towards weak links might make the food web become sparsely connected. He proposed that there is a ‘window of vitality’ (Ulanowicz 2002) in the plane defined by the topological connectance per node m * and the effective connectance per node m, which is bounded by the line m = 1, the line m = m *, and the line defined by inequality (5). Ulanowicz (2002) found that 41 observed food webs were positioned within this window of vitality, and that most of the 41 observed webs were relatively far from the ‘edges of chaos’, i.e. were positioned in the middle of the window. All 15 soil food webs that we investigated were also positioned within the window of vitality, but quite a few food webs had values of m that were close to m = 1. Based on the hypothesis of Ulanowicz (2002), it might be expected that food webs with m values close to 1 are less stable than food webs with m values that are positioned more in the centre of the window of vitality. This was indeed the case for most food webs, but some food webs actually had very low values for s, i.e. were very stable. These very stable food webs positioned close to the ‘edge of chaos’ might provide clues on what other factors than factors accounted for in weighted connectance are important for food web stability, such as the distribution of weak links in loops (Neutel et al. 2002), or specific biomass ratios between predators and their prey (Brose et al. 2006).

Should we prefer the use of weighted connectance over the use of unweighted connectance? Weighted connectance captures food web properties that are considered to be important for food webs: the number of interacting species, the number of links, and the weights of links. These properties have often been related to food web stability, but separately. Weighted connectance combines these properties, which can be seen as an advantage when one wants to use a summary statistic or as a disadvantage when actually only one of these properties is relevant to stability. By weighing fluxes, large fluxes are considered to be more important than small fluxes in terms of quantity. But it could be that the magnitude of a flux is not its most important feature. For example, it has been suggested that weak links can serve as a ‘back-up link’ for species when other links are lost (Paine 1980) and thus a link may be quantitatively redundant, but not functionally. In that case, unweighted connectance would be more suitable to use.

We used weighted connectance here to contribute to the complexity-stability debate, and used empirical information to calculate both weighted connectance, as well as stability. By using realistic distributions of fluxes and interaction strengths, i.e. distributions that are skewed towards weak fluxes and interaction strengths, we confirmed the notion of ‘complexity begets stability’. Using weighted instead of unweighted food web measures can thus shed new light on classical theories, and possibly aid in finding what biological characteristics drive the stability of food webs.