Coexistence of multiple parasitoids on a single host due to differences in parasitoid phenology

Abstract

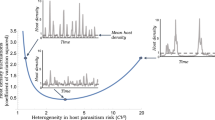
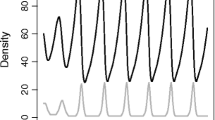
There are many well-documented cases in which multiple parasitoids can coexist on a single host species. We examine a theoretical framework to assess whether parasitoid coexistence can be explained through differences in timing of parasitoid oviposition and parasitoid emergence. This study explicitly includes the phenology of host and parasitoid development and explores how this mechanism affects the population dynamics. Coexistence of the host with two parasitoids requires a balance between parasitoid fecundity and survival and occurs most readily if one parasitoid attacks earlier but emerges later than the other parasitoid. The host density can either be decreased or increased when a second coexisting parasitoid is introduced into the system. However, there always exists a single parasitoid type that is most effective at depressing the host density, although this type may not be successful due to parasitoid competition. The coexistence of multiple parasitoids also affects the population dynamics. For instance, population oscillations can be removed by the introduction of a second parasitoid. In general, subtle differences in parasitoid phenology can give rise to different outcomes in a host–multi-parasitoid system, and this may offer some insight into why establishing criteria for the ‘ideal’ biological control agent has been so challenging.

Appendices

Appendix A: Deriving the host-multi-parasitoid model

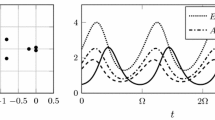
In this section, a continuum analogy, involving a system of ordinary differential equations, is used to show how the model in Section ‘Host–multiple-parasitoid model’ for two parasitoids, P and Q, and one host, H, is obtained. For concreteness, we assume that the order of attack is exactly as shown in Fig. 1, and that density–dependence acts for the whole season, i.e. T _dd  = 1. Other arrangements of attack window and choices of T _dd can be applied in a similar way.

The equations for the continuum model are

$$\label{Ht} \begin{array}{lll} \frac{dH(t)}{dt} &=& - \overbrace{a_p H(t) P_n}^{\stackrel{\text{\footnotesize{Host is parasitised }}}{\text{\footnotesize{by parasitoid $P$}}}} - \overbrace{a_q H(t) Q_n}^{\stackrel{\text{\footnotesize{Host is parasitised }}}{\text{\footnotesize{by parasitoid $Q$}}}}\\\\ &&- \overbrace{g H_n H(t)}^{\stackrel{\text{\footnotesize{Mortality due to}}}{\text{\footnotesize{intraspecific competition}}}} \end{array} $$

(9)

$$ \frac{dP(t)}{dt} = a_p H(t) P_n - g H_n P(t)\label{Pconteqn} $$

(10)

$$ \frac{dQ(t)}{dt} = a_q H(t) Q_n - g H_n Q(t)\label{Qconteqn} $$

(11)

Here, H _n , P _n and Q _n are constants which correspond to the density of hosts and adult parasitoids of type (P, Q) at the start of the season, which we take to be the density in year n. It is important to make clear that by P(t) and Q(t) we actually mean hosts parasitised by parasitoid P or Q. On the day the parasitoid emerges, the host is killed, so the parasitoid density instantaneously becomes equal to the parasitised host density. The equations above govern the most general situation when both P and Q are attacking the host. For periods where one (or both) of the parasitoids is not attacking, we should set the associated searching efficiency to zero. The host mortality term is due to intraspecific competition for resources. We assume that parasitised hosts compete in the same way as unparasitised hosts. Mortality depends on the density of hosts at the start of the season. One interpretation of this term is that hosts have reduced fitness if the parent generation was at a high density and experienced strong competition.

Solving Eq. 9 for H(t), and then substituting into the equations for P(t) and Q(t), gives the following general solutions:

$$ H(t) = c_1 e^{-(a_p P_n + a_q Q_n + g H_n)t}\label{Htsol} $$

(12)

$$P(t) = - \frac{a_p P_n}{a_p P_n + a_q Q_n} c_1 e^{-(a_p P_n + a_q Q_n + g H_n)t} + c_2 e^{-g H_n t} \label{Ptsol} $$

(13)

$$ Q(t) = - \frac{a_q Q_n}{a_p P_n + a_q Q_n} c_1 e^{-(a_p P_n + a_q Q_n + g H_n)t} + c_3 e^{- g H_n t}, \label{Qtsol} $$

(14)

where c ₁, c ₂, c ₃ are integration constants. We now apply the boundary conditions in each segment of the year indicated in Fig. 1 to work out H(n + 1), P(n + 1), Q(n + 1), i.e. the densities at the start of year n + 1.

First, we consider the period 0 < t < t _ps . The initial host density is H(0) = H _n . Since no parasitoid attacks the host in this period, we should set a _p  = a _q  = 0 in Eqs. 12–14 and the boundary conditions are P(0) = P(t _ps ) = 0 and Q(0) = Q(t _ps ) = 0. Therefore, at the end of the first period, we obtain

$$ H(t_{ps}) = H_n e^{-g H_n t_{ps}}, \qquad P(t_{ps}) = 0, \qquad Q(t_{ps})=0\;. \label{initial1} $$

(15)

These will be initial conditions for the solutions in the next time period.

We now consider round 1, namely, t _ps  < t < t _qs . Since Q has not begun its attack, we set a _q  = 0 in Eqs. 12–14. Our initial conditions are Eq. 15, which allow us to determine the constants as follows:

$$ c_1 = H_n e^{a_p P_n t_{ps}}, \qquad c_2 = H_n, \qquad c_3 = 0. $$

Therefore, at the end of round 1, we have densities

$$ H(t_{qs}) = H_n e^{-a_p P_n (t_{qs} - t_{ps})} e^{-g H_n t_{qs}} $$

$$ P(t_{qs}) = H_n ( 1 - e^{-a_p P_n(t_{qs} - t_{ps})}) e^{-g H_n t_{qs}} $$

$$ Q(t_{qs}) = 0\;. \label{initial2} $$

(16)

These give the initial conditions for round 2.

In round 2, i.e. t _qs  < t < t _pf , both P and Q are attacking. Using the initial conditions Eq. 16 at time t _qs in the solutions Eqs. 12–14, we find

$$ \begin{array}{lll} c_1 &=& H_n e^{a_p P_n t_{ps} + a_q Q_n t_{qs}}\\ c_2 &=& H_n \left( 1 - \frac{a_q Q_n}{a_p P_n + a_q Q_n}e^{-a_p P_n(t_{qs} - t_{ps})}\right)\\ c_3 &=& \frac{a_q Q_n}{a_p P_n + a_q Q_n}\ H_n e^{-a_p P_n(t_{qs} - t_{ps})}\;. \end{array} $$

So, using these values for the constants, at the end of round 2, we have

$$ H(t_{pf}) = H_n e^{-a_p P_n (t_{pf} - t_{ps})} e^{-a_q Q_n(t_{pf} - t_{qs})} e^{-g H_n t_{pf}} $$

$$ \begin{array}{lll} P(t_{pf})&=& H_n e^{-gH_n t_{pf}} \left( 1 - \frac{1}{a_p P_n + a_q Q_n} e^{-a_p P_n (t_{qs} - t_{ps})}\right.\\ &&{\kern50pt}\left. \times\left( a_q Q_n + a_p P_n e^{- a_q Q_n (t_{pf} - t_{qs})}\right)\right) \end{array} $$

$$\label{initial3} \begin{array}{lll} Q(t_{pf}) &=& \frac{a_q Q_n}{a_p P_n + a_q Q_n} H_n e^{-gH_n t_{pf}}\\ &&\times \left( e^{-a_p P_n (t_{qs} - t_{ps})} - e^{-a_p P_n (t_{pf} -t_{ps}) - a_q Q_n(t_{pf} - t_{qs})} \right) \end{array} $$

(17)

which become the initial conditions for the next round.

In round 3, t _pf  < t < t _qf , P has stopped attacking, so we should set a _p  = 0 in Eqs. 12–14. Using Eq. 17 as initial conditions, we obtain

$$ \begin{array}{lll} c_1 &=& H_n e^{-a_p P_n (t_{pf}- t_{ps})} e^{a_q Q_n t_{qs}}\\\\ c_2 &=& H_n \left( 1 - \frac{1}{a_p P_n + a_q Q_n} e^{-a_p P_n (t_{qs} - t_{ps})}\right.\\\\ &&{\kern20pt}\left.\times\left( a_q Q_n + a_p P_n e^{- a_q Q_n (t_{pf} - t_{qs})}\right)\right)\\\\ c_3 &=& \frac{H_n}{a_p P_n + a_q Q_n}\left( a_q Q_n e^{-a_p P_n (t_{qs} - t_{ps})} \right.\\\\ &&{\kern65pt} \left.+\, a_p P_n e^{-a_p P_n (t_{qs} - t_{ps}) - a_q Q_n(t_{pf} - t_{qs})}\right)\;. \end{array} $$

So by the end of round 3, the densities are

$$ \begin{array}{lll} H(t_{qf})&=& H_n e^{-a_p P_n (t_{pf} - t_{ps})}e^{-a_q Q_n (t_{qf} - t_{qs})} e^{-g H_n t_{qf}} \\\\ P(t_{qf}) &=& H_n e^{-g H_n t_{qf}}\left( 1 - e^{-a_p P_n (t_{qs} - t_{ps})}\right) \\\\ && + H_n e^{-g H_n t_{qf}} e^{-a_p P_n (t_{qs} - t_{ps})} \frac{a_p P_n}{a_p P_n + a_q Q_n}\\\\ &&\times \left( 1 - e^{-a_p P_n(t_{pf} - t_{qs}) - a_q Q_n (t_{pf} - t_{qs})}\right) \end{array} $$

$$ \begin{array}{lll} Q(t_{qf}) &=& H_n e^{-g H_n t_{qf}} e^{-a_p P_n (t_{qs} - t_{ps})} \frac{a_q Q_n}{a_p P_n + a_q Q_n} \\\\ &&\times \left( 1 - e^{-(a_p P_n+a_q Q_n)(t_{pf} - t_{qs})}\right)\\\\ && + H_n e^{-g H_n t_{qf}} e^{-a_p P_n (t_{pf} - t_{ps}) - a_q Q_n (t_{pf} - t_{qs})}\\\\ &&\times\left( 1 - e^{-a_q Q_n (t_{qf} - t_{pf})}\right)\;. \end{array} $$

(18)

For t > t _qf , both parasitoids have finished attacking (ovipositing on) the hosts. Density-dependence continues to affect the parasitised host until the parasitoids emerge from the host at α _p , α _q . Unparasitised hosts continue to experience density-dependence for the remainder of the season. Therefore, the densities at t = n + 1 are

$$ \begin{array}{lll} H(n+1 ) &=& H_n e^{-a_pP_n(t_{pf} - t_{ps}) - a_q Q_n (t_{qf} - t_{qs}) - g H_n}\\\\ P(n+1) &=& H_n e^{-g H_n \alpha_p}\\\\ &&\times\left[ \left(1 - e^{-a_p P_n (t_{qs} - t_{ps})}\right) + \frac{a_p P_n}{a_p P_n + a_q Q_n}\right.\\\\ &&\left.\times\, e^{-a_p P_n(t_{qs} - t_{ps})}\left(1 - e^{-(a_p P_n + a_q Q_n)(t_{pf}-t_{qs})}\right)\right] \end{array} $$

$$\label{final} \begin{array}{lll} Q(n+1)&=& H_n e^{-g H_n \alpha_q}\\\\ &&\times \left[ \frac{a_p Q_n}{a_p P_n + a_q Q_n}e^{-a_p P_n(t_{qs} - t_{ps})}\right.\\\\ &&{\kern8pt}\times \left(1 - e^{-(a_p P_n + a_q Q_n)(t_{pf}-t_{qs})}\right)\\\\ &&{\kern8pt} + e^{-a_p P_n(t_{qs} - t_{ps})} e^{-(a_p P_n + a_q Q_n)(t_{pf} - t_{qs})}\\\\ &&{\kern8pt}\left.\times\left( 1 - e^{-a_q Q_n(t_{qf} - t_{pf})}\right)\right] \end{array} $$

(19)

To obtain the model in Section ‘Host–multiple-parasitoid model’, we assume that, just prior to the start of the next season, adult hosts reproduce with, on average, e ^r offspring per host, which means we multiply the equation for H by e ^r. In a more general setting where the density–dependent phase has length T _dd , we can obtain the corresponding model by scaling g →g T _dd . (Note that in this model framework, density dependence always appears in the equations as an overall multiplicative factor.)

Appendix B: Host–N-parasitoid model for equal attack windows

In the special case when all attack windows are equal, the equations for N parasitoids, P ⁽ⁱ⁾, are described by the following equations:

$$ H_{n+1} = H_n e^r e^{-g H_n T_{dd}} e^{-(t_f - t_s)\sum_i a_i P_n^{(i)}} $$

(20)

$$ P_{n+1}^{(i)} = H_n e^{-\alpha_i g H_n T_{dd}} \frac{a_i P_n^{(i)}}{\sum_i a_i P_n^{(i)}} \left( 1 - e^{-(t_f - t_s)\sum_i a_i P_n^{(i)}}\right)\ , $$

(21)

where t _s , t _f are the start and finish times of the attack window and i = 1, ..., N. Each parasitoid species P ⁽ⁱ⁾ has an associated searching efficiency, a _i , and fraction of host density-dependence which it experiences, α _i .