Abstract
A series of gas nitriding and gas nitrocarburizing experiments was performed at 823 K (550 °C) to investigate the growth kinetics of ε-Fe3(N,C)1+x /γ′-Fe4N1-z -double layers on pure α-iron substrates. The growth rate and composition of the (sub)layers were determined by (sub)layer-thickness measurements using light optical microscopy and electron-probe microanalyses (EPMA), respectively. Models for the growth of bilayers into a substrate, controlled by the interstitial diffusion of two elements (N and C), were applied to the experimental data to determine the intrinsic diffusion coefficients of N and C in ε-Fe3(N,C)1+x as well as the self-diffusion coefficient of N in γ′-Fe4N1−z . For ε-Fe3(N,C)1+x , it was found that the four components of the diffusion matrix, \( D_{\text{NN}}^{\varepsilon } ,\,D_{\text{CC}}^{\varepsilon } ,\,D_{\text{NC}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \), are all positive. The significant values of the off-diagonal diffusivities \( D_{\text{NC}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \) indicate profound interaction of both interstitial species. Thereby, additional information is obtained about the thermodynamic properties of the ε phase in the ternary Fe-N-C system.








Similar content being viewed by others
Notes
The chemical potentials of nitrogen and carbon in the nitriding/nitrocarburizing atmosphere are directly related to the corresponding activities by the equation \( \mu_{k} = \mu_{k}^{0} + RT\ln (a_{k} ) \), where \( k \in \left\{ {\text{N, C}} \right\},\,\mu_{k} \) is the chemical potential of species k and \( \mu_{k}^{0} \) is the chemical potential of k in the reference state (nitrogen gas at 1 atm for a N and graphite at 1 atm for a C, at the temperature concerned).
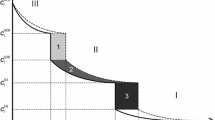
Local equilibrium, for example, at the ε/γ′ interface, does not necessarily require constancy of the position of the diffusion path[1,23,24] in ε (see Section IV–B; Fig. 7) and the corresponding concentrations \( c_{\text{N}}^{\varepsilon /\gamma '} \) and \( c_{\text{C}}^{\varepsilon /\gamma '} \) in ε at the ε/γ′ interface. Diffusion path and interface concentrations may change as a function of time according to the tie-lines in the ε+γ′ two-phase region of the Fe-N-C phase diagram. If such changes occurred and/or if the surface concentration were not constant (no local equilibrium between gas phase and solid) it is at least required that the concentration changes occur sufficiently slowly.
Quasi-steady-state diffusion of N in γ′ was confirmed by numerical calculations[27] for a γ′ (sub)layer growing into a ferrite substrate, after lifting the constraint of a constant (stoichiometric) composition of γ′ as assumed in the current article, and is attributed to the very small compositional range of γ′ iron nitride and the large concentration difference between the γ′ sublayer and the α-iron substrate.
The Boltzmann transformation \( \lambda = {x \mathord{\left/ {\vphantom {x {\sqrt t }}} \right. \kern-0pt} {\sqrt t }} \)[28] can only be applied for the extreme cases of a nitrogen presaturated substrate or an infinitely thick substrate provided that the surface concentrations of N and C in ε are constant. Under these boundary conditions, the diffusion process is invariant to \( \lambda = {x \mathord{\left/ {\vphantom {x {\sqrt t }}} \right. \kern-0pt} {\sqrt t }} \) (i.e., the boundary conditions can be expressed in terms of \( \lambda = {x \mathord{\left/ {\vphantom {x {\sqrt t }}} \right. \kern-0pt} {\sqrt t }} \)), and the equations for the concentration profile can be expressed in terms of error functions as shown in Refs. 1,12.
In contrast with, e.g., the ε phase, with a wide composition range, the very narrow compositional range of N in γ′ cannot be easily determined experimentally. As compared with the nitrogen-content variation, the nitrogen-activity range over the γ′ (sub)layer is not small and can straightforwardly be derived from nitriding experiments.[31] For that reason, the flux equation for γ′ is related here to the self-diffusion coefficient and the nitrogen-activity range over the γ′-phase (cf. Eq. [5]).
The hypothetical, initial squared layer thickness is in general given by \( \left( {S_{0}^{I} } \right)^{2} = -k^{I} t_{0} \), where t 0 can be conceived as an effective incubation time for the (carbo)nitride formation if t 0 > 0. In the case of t 0 < 0 the layer growth is initially faster than at later stages when the growth is given as a function of k I.
Validity of this treatment for estimating uncertainties of the diffusion coefficients should not be affected by, in the calculation, the intermediate determination of interface velocities and fluxes in the main article.
References
J.S. Kirkaldy, D.J. Young. Diffusion in the Condensated State. London: The Institute of Metals, 1987.
H. Mehrer: Diffusion in Solids: Fundamentals, Methods, Materials, Diffusion-Controlled Processes, Springer, Berlin, 2007.
M.E. Glicksman. Diffusion in Solids. New York: John Wiley & Sons, 2000.
R. Taylor, R. Krishna. Multicomponent Mass Transfer: Wiley, New York, 1993.
P. Shewmon: Diffusion in Solids, Minerals, Metals & Materials Society, Warrendale, 1989.
E.J. Mittemeijer, M.A.J. Somers: Surf. Eng. 1997;13:483-497.
P.F. Colijn, E.J. Mittemeijer, H.C.F. Rozendaal: Z. Metallkd. 1983;74:620-627.
T. Woehrle, A. Leineweber, E.J. Mittemeijer: HTM J. Heat Treatm. Mater. 2010;65:243-248.
M.A.J. Somers, E.J. Mittemeijer: Surf. Eng. 1987;3:123-137.
T. Gressmann, M. Nikolussi, A. Leineweber, E.J. Mittemeijer: Scripta Mater. 2006;55:723-726.
M.A.J. Somers, E.J. Mittemeijer: Metall. Mater. Trans. A 1995;26A:57-74.
H. Du, J. Ågren: Metall. Mater. Trans. A 1996;27A:1073-1080.
J. Kunze. Nitrogen and Carbon in Iron and Steel: Akademie-Verlag Berlin, 1990.
H. Du: J. Phase Equilib. 1993;14:682-693.
J. Slycke, L. Sproge: Surf. Eng. 1989;5:125-140.
M.A.J. Somers, P.F. Colijn, W.G. Sloof, E.J. Mittemeijer: Z. Metallkd. 1990;81:33-43.
H. Du, M.A.J. Somers, J. Ågren: Metall. Mater. Trans. A 2000;31A:195-211.
J. Slycke, L. Sproge, J. Ågren: Scand. J. Metall. 1988;17:122-126.
A. Leineweber, T. Gressmann, E.J. Mittemeijer: Surf. Coat. Technol. 2012;206:2780-2791.
A. Wells: J. Mat. Sci. 1985;20:2439-2445.
J.L. La Pouchou and F. Pichoir: Rech. Aerospatial, 1984, 167–92.
E. Lehrer: Z. Elektrochem. 1930;36:383-392.
T. Woehrle, A. Leineweber, E.J. Mittemeijer: Metall. Mater. Trans. A 2012;43A:2401-2413.
A.A. Kodentsov, G.F. Bastin, F.J.J. Van Loo: J. Alloys Compd. 2001;320:207-217.
H. Du: Thesis, Royal Institute of Technology, 1994.
H. Du, J. Ågren: Z. Metallkd. 1995;86:522-529.
T. Woehrle, A. Leineweber, E.J. Mittemeijer: Metall. Mater. Trans. A 2012;43A:610-618.
J. Crank: The Mathematics of Diffusion, Oxford Science Publications, Oxford, 1975.
M. Weller: Mater. Sci. Forum 2001;366-368:95-137.
M. Hillert, L. Höglund, and J. Ågren: J. Appl. Phys. 2005, vol. 98, pp. 053511-1–6.
K. Schwerdtfeger, P. Grieveson, E.T. Turkdogan: Trans. Metall. Soc. AIME 1969;245:2461-2466.
E.L. Cussler: Diffusion: Mass Transfer in Fluid Systems, Cambridge University Press, Cambridge, 1997.
J.-O. Andersson, J. Ågren: J. Appl. Phys. 1992;72:1350-1355.
L. Torchane, P. Bilger, J. Dulcy, M. Gantois: Metall. Mater. Trans. A 1996;27A:1823-1835.
B. Prenosil: Kovove Mater. 1965;3:69-87.
M.A.J. Somers, B.J. Kooi, L. Maldzinski, E.J. Mittemeijer, A.A. van der Horst, A.M. van der Kraan, N.M. van der Pers: Acta Mater. 1997;45:2013-2025.
B.J. Kooi, M.A.J. Somers, E.J. Mittemeijer: Metall. Mater. Trans. A 1996;27A:1063-1071.
H. Du, M. Hillert: Z. Metallkunde 1991;82:310-316.
J. Slycke: Berichtsband AWT-VWT-Tagung “Nitrieren und Nitrocarburieren” 1996, pp. 19–28.
J. Kunze: Härterei-Tech. Mitt. 1996;51:348-355.
M. Hillert. Phase Equilibria, Phase Diagrams, and Phase Transformations: Cambridge University Press, Cambridge, 2007.
P. Fornasini: The Uncertainty in Physical Measurements: An Introduction to Data Analysis in the Physics Laboratory, Springer, New York, 2008.
Author information
Authors and Affiliations
Corresponding author
Additional information
Manuscript submitted January 25, 2012.
Appendices
Appendix A
According to the Hillert–Staffansson approach,[41] the ε phase can be conceived as an interstitial subregular solid solution of formula unit \( \left( {\text{Fe}} \right)_{a} \left( {\text{N,C,Va}} \right)_{c} \) composed of two separate sublattices: one substitutional sublattice with a number of a sites per formula unit occupied by Fe and an interstitial sublattice with a number of c sites per formula unit occupied by N, C, and vacancies (Va). The number of sites per formula unit assumed in the literature for the ε phase is a = 1, c = 1 according to Reference 13 and a = 1, c = 1/2 according to Reference 14, i.e., the site fractions of component k (N, C) in the interstitial sublattice, \( y_{k}^{\varepsilon } \), given in References 13 and 14 differ by a factor of 2.
The thermodynamic factors, \( \vartheta_{kj}^{\varepsilon } \), can be calculated from the Gibbs energy per formula unit, \( G_{\text{m}}^{\varepsilon } \),[13,14] as
It thus is obtained that
where \( \Updelta L^{\varepsilon } = \left[{{}^{0}L_{\text{Fe:N,Va}}^{\varepsilon } + 2\left({3y_{\text{N}}^{\varepsilon } + y_{\text{C}}^{\varepsilon } - 1}\right){}^{1}L_{\text{Fe:N,Va}}^{\varepsilon } } \right] +{}^{0}L_{\text{Fe:C,Va}}^{\varepsilon } -{}^{0}L_{\text{Fe:N,C}}^{\varepsilon } \), \( y_{k}^{\varepsilon } \) is the site fraction of component k in the interstitial sublattice,\( {}^{0}L_{\text{Fe:N,Va}}^{\varepsilon } \), \( {}^{0}L_{\text{Fe:N,C}}^{\varepsilon } \) and \( {}^{0}L_{\text{Fe:C,Va}}^{\varepsilon } \) are the regular solid solution parameters and \( {}^{1}L_{\text{Fe:N,Va}}^{\varepsilon } \) is the subregular solid solution parameter. The first term of the thermodynamic factor \( \vartheta_{kj}^{\varepsilon } \) given at the right-hand side of Eqs. [A2] through [A5] represents the contribution of the configurational entropy, and the second term represents the contribution of the excess enthalpy, describing the non-ideality of the system, i.e., the interaction of nitrogen and carbon. The (sub)regular parameters can be obtained on the basis of an assessments of the ternary Fe-N-C system.[13,14,38] Note that the thermodynamic model assumed in Reference 14 provides a more distinct contribution of the configurational entropy to the thermodynamic factor compared with the model used in Reference 13.
Note that also for ideal systems (then the (sub)regular parameters and thus the excess enthalpy is zero) with a wide composition range, the off-diagonal thermodynamic factors can be significant because of the configurational entropy, i.e., \( \vartheta_{kj}^{\varepsilon } /\vartheta_{kk}^{\varepsilon } = y_{k}^{\varepsilon } /\left( {1 - y_{j}^{\varepsilon } } \right) \), reflecting the geometrical interaction of nitrogen and carbon. It holds that \( \vartheta_{kj}^{\varepsilon } \left( {k \ne j} \right) \to 0 \) for \( y_{k}^{\varepsilon } \to 0 \), which, obviously, is not the case for the diagonal components.
Appendix B
The gas composition used for the nitriding and nitrocarburizing experiments of series A1, A2, B1, and B2 has been given in Table IX. These gas compositions as claimed to impose specific combinations of a N and a C at 823 K (550 °C), were calculated as described in Reference 19.
Appendix C
In order to arrive at estimates for the uncertainties/errors of the values given for the intrinsic diffusion coefficients \( D_{\text{NN}}^{\varepsilon } ,\,D_{\text{CC}}^{ \varepsilon } ,\,D_{\text{NC}}^{ \varepsilon } \) and \( D_{\text{CN}}^{ \varepsilon } \) a simplified calculation is performed here. These errors are believed to be mainly caused by the uncertainties of the values of the concentration differences \( c_{j}^{\varepsilon /{\text{gas}}} - c_{j}^{\varepsilon /\gamma'} \) with \( j \in \left\{ {\text{N,C}} \right\} \) over the thickness of the ε sublayers as well as of the parabolic growth rate constants \( k^{\xi } \), whereas the uncertainties of the absolute concentration values and of initial squared layer thicknesses are believed to be comparably small.
In order to arrive at an estimate for the effect of the errors of these quantities on the diffusion coefficients determined on the basis of these quantities, consider the growth of a single, close-to-stoichiometric layer of a phase ξ on a substrate (sub.), exhibiting a linear evaluation in the X content from \( c_{X}^{{\xi /{\text{gas}}}} \) at the surface and \( c_{X}^{{\xi / {\text{sub}} .}} \) at the interface to the substrate. The growth of that layer is controlled by diffusion of an interstitially diffusing component X supplied by a gas phase. This component X shows negligible solubility in the substrate. Thus, one finds for the average X content \( {1}/{2}\left( {c_{X}^{{\xi /{\text{gas}}}} + c_{X}^{{\xi /{\text{sub}} .}} } \right) \) in the layer \( {1}/{2}\left( {c_{X}^{{\xi /{\text{gas}}}} + c_{X}^{{\xi /{\text{sub}} .}} } \right) \gg c_{X}^{{\xi /{\text{gas}}}} -c_{X}^{{\xi / {\text{sub}} .}} \), where \( {c_{X}^{{\xi /{\text{gas}}}} - c_{X}^{{\xi / {\text{sub}} .}} } \) is the concentration difference of X over the thickness of the layer, which is time independent if local equilibrium at the two interfaces of the layer is assumed. In such a case, the assumedly ideal parabolic layer growth leads to a simple relation for diffusion coefficient \( D_{X}^{\xi } \) as
where \( k^{\xi } \) is the parabolic growth-rate constant for the growth of the ξ phase. One, e.g., finds a corresponding equation for the growth of the pure γ′-iron nitride phase on α-Fe if solubility of N in α is neglected.[11] In such a case, employing the method of relative errors,[42] the error of \( D_{X}^{\xi } \), \( \sigma (D_{X}^{\xi } ) \), results from those of \( {c_{X}^{{\xi /{\text{gas}}}} - c_{X}^{{\xi / {\text{sub}} .}} } \) and \( k^{\xi } \) as:
In the current study, values for \( D_{\text{NN}}^{\varepsilon } ,\,D_{\text{CC}}^{\varepsilon } ,\,D_{\text{NC}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon} \) have been determined by fitting on the basis of the experimental data pertaining to nitrocarburizing conditions of various combinations of a N and a C. Their simultaneous determination from the layer-growth kinetics and concentration data pertaining to only one set of (a N, a C) values in the atmosphere is not possible. Nevertheless, as an approximation Eq. [C2] with ξ = ε and X = N, C may be employed for estimating the impact of the errors of \( k^{\xi } ,\,c_{\text{N}}^{\varepsilon /{\text{gas}}} -c_{\text{N}}^{\varepsilon /\gamma '} \), and \( c_{\text{C}}^{\varepsilon /{\text{gas}}} -c_{\text{C}}^{\varepsilon /\gamma '} \) on the relative errors of the diffusion coefficients expected for that set of (a N, a C).Footnote 8 According to Eq. [6], the relative errors of \( D_{\text{NN}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \) should be associated with the error of \( c_{\text{N}}^{\varepsilon /{\text{gas}}} -c_{\text{N}}^{\varepsilon /\gamma '} \), whereas the relative errors of \( D_{\text{NN}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \) should be associated with the error of \( c_{\text{C}}^{\varepsilon /{\text{gas}}} -c_{\text{C}}^{\varepsilon /\gamma '} \). Since the value of \( c_{\text{N}}^{\varepsilon /{\text{gas}}} - c_{\text{N}}^{\varepsilon /\gamma '} \) decreases considerably with increasing a C (see Table IV), the relative error of \( {c_{\text{N}}^{\varepsilon /{\text{gas}}} - c_{\text{N}}^{\varepsilon /\gamma '} } \) increases and thus the relative errors of \( D_{\text{NN}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \) increase. The situation is opposite for \( D_{\text{CC}}^{\varepsilon} \) and \( D_{\text{NC}}^{\varepsilon} \): \( {c_{\text{C}}^{\varepsilon /{\text{gas}}} - c_{\text{C}}^{\varepsilon /\gamma '} } \) increases with increasing a C, leading to a decrease of the relative error of \( {c_{\text{C}}^{\varepsilon /{\text{gas}}} - c_{\text{C}}^{\varepsilon /\gamma '} } \) and thus of \( D_{\text{CC}}^{\varepsilon} \) and \( D_{\text{NC}}^{\varepsilon} \).
For all four diffusion coefficients, the minimum values of the relative errors calculated with Eq. [19] are found to amount to 20 pct. One may justify adopting these minimum relative errors for the refined values \( D_{\text{NN}}^{\varepsilon } ,\,D_{\text{CC}}^{\varepsilon } ,\,D_{\text{NC}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \) determined on the basis of all employed sets of (a N, a C) in view of the outcome of Figure 7: Large fluxes \( J_{\text{NN}}^{\varepsilon } \) and \( J_{\text{CN}}^{\varepsilon } \) (associated via Eq. [6] with \( D_{\text{NN}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \)) are only found for small values of a C, whereas large fluxes \( J_{\text{CC}}^{\varepsilon } \) and \( J_{\text{NC}}^{\varepsilon } \)(associated with \( D_{\text{NN}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \)) for large a C. These are the ranges of a C values for which small relative errors are obtained using Eq. [19]. In the course of the fitting procedure, the data with the large fluxes will receive the main weight when refining the values for \( D_{\text{NN}}^{\varepsilon } ,\,D_{\text{CC}}^{\varepsilon } ,\,D_{\text{NC}}^{\varepsilon } \) and \( D_{\text{CN}}^{\varepsilon } \), so that above-mentioned minimum 20 pct relative uncertainty will be a reasonable estimate of the uncertainty of the refined diffusion coefficients.
Rights and permissions
About this article
Cite this article
Woehrle, T., Leineweber, A. & Mittemeijer, E.J. Multicomponent Interstitial Diffusion in and Thermodynamic Characteristics of the Interstitial Solid Solution ε-Fe3(N,C)1+x : Nitriding and Nitrocarburizing of Pure α-Iron. Metall Mater Trans A 44, 2548–2562 (2013). https://doi.org/10.1007/s11661-013-1640-z
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11661-013-1640-z