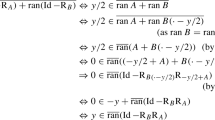Abstract
Maximally monotone operators and firmly nonexpansive mappings play key roles in modern optimization and nonlinear analysis. Five years ago, it was shown that if finitely many firmly nonexpansive operators are all asymptotically regular (i.e., they have or “almost have” fixed points), then the same is true for compositions and convex combinations. In this paper, we derive bounds on the magnitude of the minimal displacement vectors of compositions and of convex combinations in terms of the displacement vectors of the underlying operators. Our results completely generalize earlier works. Moreover, we present various examples illustrating that our bounds are sharp.


Similar content being viewed by others
Notes
We shall write \({\text {dom}}A = \big \{{x\in X}~\big |~{Ax\ne \varnothing }\big \}\) for the domain of A, \({\text {ran}}A = A(X) = \bigcup _{x\in X}Ax\) for the range of A, and \({\text {gra}}A=\big \{{(x,u)\in X\times X}~\big |~{u\in Ax}\big \}\) for the graph of A.
Here and elsewhere, \({\text {Id}}\) denotes the identity operator on X.
Given a nonempty closed convex subset C of X, we denote its projection mapping or projector by \(P_C\).
Let \(S:X\rightarrow X\). Then S is \(\alpha \)-averaged if there exists \(\alpha \in [0,1[\) such that \(S=(1-\alpha ){\text {Id}}+\alpha N\) and \(N:X\rightarrow X\) is nonexpansive.
We recall that a monotone operator \(B:X\rightrightarrows X\) is 3* monotone (see [9]) (this is also known as rectangular) if \((\forall (x,y^*)\in {\text {dom}}B\times {\text {ran}}B)\) \(\sup _{(z,z^*)\in {\text {gra}}B} \left\langle {x-z},{z^*-y^*} \right\rangle <+\infty \).
References
Bauschke, H.H.: The composition of finitely many projections onto closed convex sets in Hilbert space is asymptotically regular. Proc. Am. Math. Soc. 131, 141–146 (2003)
Bauschke, H.H., Borwein, J.M.: Dykstra’s alternating projection algorithm for two sets. J. Approx. Theory 79, 418–443 (1994)
Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces, Second edn. Springer, Berlin (2017)
Bauschke, H.H., Hare, W.L., Moursi, W.M.: Generalized solutions for the sum of two maximally monotone operators. SIAM J. Control Optim. 52, 1034–1047 (2014)
Bauschke, H.H., Martin-Marquez, V., Moffat, S.M., Wang, X.: Compositions and convex combinations of asymptotically regular firmly nonexpansive mappings are also asymptotically regular. Fixed Point Theory Appl. 2012, 53 (2012)
Bauschke, H.H., Moursi, W.M.: The Douglas–Rachford algorithm for two (not necessarily intersecting) affine subspace. SIAM J. Optim. 26, 968–985 (2016)
Borwein, J.M., Vanderwerff, J.D.: Convex Functions. Cambridge University Press, Cambridge (2010)
Brezis, H.: Operateurs Maximaux Monotones et Semi-Groupes de Contractions dans les Espaces de Hilbert. North-Holland/Elsevier, Amsterdam (1973)
Brezis, H., Haraux, A.: Image d’une Somme d’opérateurs Monotones et Applications. Israel J. Math. 23, 165–186 (1976)
Burachik, R.S., Iusem, A.N.: Set-Valued Mappings and Enlargements of Monotone Operators. Springer, Berlin (2008)
De Pierro, A.: From parallel to sequential projection methods and vice versa in convex feasibility: results and conjectures. In: Inherently parallel algorithms in feasibility and optimization and their applications (Haifa, 2000). Studies in Computational Mathematics, vol. 8, pp. 187–201. North-Holland, Amsterdam (2001)
Eckstein, J., Bertsekas, D.P.: On the Douglas–Rachford splitting method and the proximal point algorithm for maximal monotone operators. Math. Program. 55, 293–318 (1992)
GeoGebra. http://www.geogebra.org. Accessed 4 Dec 2017
Goebel, K., Kirk, W.A.: Topics in Metric Fixed Point Theory. Cambridge University Press, Cambridge (1990)
Goebel, K., Reich, S.: Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. Marcel Dekker, New York (1984)
Kohlenbach, U.: A polynomial rate of asymptotic regularity for compositions of projections in Hilbert space. Found. Comput. Math. (2018). https://doi.org/10.1007/s10208-018-9377-0
Kohlenbach, U., López-Acedo, G., Nicolae, A.: Quantitative asymptotic regularity results for the composition of two mappings. Optimization 66, 1291–1299 (2017)
Minty, G.J.: Monotone (nonlinear) operators in Hilbert spaces. Duke Math. J. 29, 341–346 (1962)
Moursi, W.M.: The forward–backward algorithm and the normal problem. J. Optim. Theory Appl. 176, 605–624 (2018)
Pennanen, T.: On the range of monotone composite mappings. J. Nonlinear Convex Anal. 2, 193–202 (2001)
Rockafellar, R.T.: Convex Analysis. Princeton University Press, Princeton (1970)
Rockafellar, R.T., Wets, R.J.-B.: Variational Analysis. Springer, Berlin (2009). (corrected 3rd printing)
Simons, S.: From Hahn–Banach to Monotonicity. Springer, Berlin (2008)
Simons, S.: Minimax and Monotonicity. Springer, Berlin (1998)
Zălinescu, C.: Convex Analysis in General Vector Spaces. World Scientific Publishing, Singapore (2002)
Zeidler, E.: Nonlinear Functional Analysis and Its Applications I: Fixed Point Theorems. Springer, Berlin (1993)
Zeidler, E.: Nonlinear Functional Analysis and Its Applications II/A: Linear Monotone Operators. Springer, Berlin (1990)
Zeidler, E.: Nonlinear Functional Analysis and Its Applications II/B: Nonlinear Monotone Operators. Springer, Berlin (1990)
Acknowledgements
The research of HHB was partially supported by a Discovery Grant of the Natural Sciences and Engineering Research Council of Canada. WMM was was partially supported by the DIMACS/Simons Collaboration on Bridging Continuous and Discrete Optimization through NSF Grant # CCF-1740425.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Bauschke, H.H., Moursi, W.M. The magnitude of the minimal displacement vector for compositions and convex combinations of firmly nonexpansive mappings. Optim Lett 12, 1465–1474 (2018). https://doi.org/10.1007/s11590-018-1259-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11590-018-1259-5