Skip to main content
SpringerLink
Log in

Fundamental finite key limits for one-way information reconciliation in quantum key distribution

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

The security of quantum key distribution protocols is guaranteed by the laws of quantum mechanics. However, a precise analysis of the security properties requires tools from both classical cryptography and information theory. Here, we employ recent results in non-asymptotic classical information theory to show that one-way information reconciliation imposes fundamental limitations on the amount of secret key that can be extracted in the finite key regime. In particular, we find that an often used approximation for the information leakage during information reconciliation is not generally valid. We propose an improved approximation that takes into account finite key effects and numerically test it against codes for two probability distributions, that we call binary–binary and binary–Gaussian, that typically appear in quantum key distribution protocols.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Notes

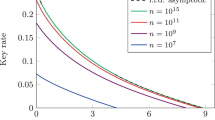
  1. Consider, for example, BB84 with asymmetric basis choice [25] on a channel with quantum bit error rate Q. Here, contributions (1) and (2) vanish asymptotically while contributions (3) and (4) converge to h(Q).

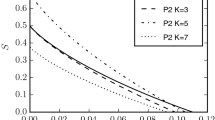
  2. Recent works analyzing the finite block length behavior using this approximation include [1, 5, 7, 17, 24, 35, 43].

  3. We here apply Theorem 1 to distributions that are continuous in Y. Note that the proofs leading to Theorem 1 can easily be generalized to this setting.

References

  1. Abruzzo, S., Mertz, M., Kampermann, H., Bruss. D.: Finite-key analysis of the six-state protocol with photon number resolution detectors. In: Proceedings of SPIE, pp. 818917. Prague (2011)

  2. Altug, Y., Wagner, A. B.: The third-order term in the normal approximation for singular channels. In: IEEE International Symposium on Information Theory (ISIT), 2014, pp. 1897–1901. IEEE, (2014)

  3. Beigi, S., Gohari, A.: Quantum achievability proof via collision relative entropy. IEEE Trans. Inf. Theory 60(12), 7980–7986 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  4. Bennett, C. H., Brassard, G.: Quantum cryptography: public key distribution and coin tossing. In: Proceedings of IEEE International Conference on Computer System Signal Processing, pp. 175–179, IEEE, Bangalore (1984)

  5. Bratzik, S., Mertz, M., Kampermann, H., Bruß, D.: Min-entropy and quantum key distribution: nonzero key rates for small numbers of signals. Phys. Rev. A 83(2), 022330 (2011)

    Article  ADS  Google Scholar 

  6. Bruß, D.: Optimal eavesdropping in quantum cryptography with six states. Phys. Rev. Lett. 81(14), 3018–3021 (1998)

    Article  ADS  Google Scholar 

  7. Cai, R.Y.Q., Scarani, V.: Finite-key analysis for practical implementations of quantum key distribution. New J. Phys. 11(4), 045024 (2009)

    Article  ADS  Google Scholar 

  8. Chung, S.-Y., Forney, G.D., Richardson, T.J., Urbanke, R.: On the design of low-density parity-check codes within 0.0045 dB of the Shannon limit. IEEE Commun. Lett. 5(2), 58–60 (2001)

    Article  Google Scholar 

  9. Dupuis, F., Kraemer, L., Faist, P., Renes, J. M., Renner, R.: Generalized entropies. In: Proceedings of XVIIth International Congress on Mathematical Physics, pp. 134–153, Aalborg, Denmark (2012)

  10. Ekert, A.K.: Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 67(6), 661–663 (1991)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  11. Elkouss, D., Martinez-Mateo, J., Martin, V.: Information reconciliation for quantum key distribution. Quantum Inf. Comput. 11(3), 226–238 (2011)

    MATH  MathSciNet  Google Scholar 

  12. Elkouss, D., Martinez-Mateo, J., Martin, V.: Untainted puncturing for irregular low-density parity-check codes. IEEE Wirel. Commun. Lett. 1(6), 585–588 (2012)

    Article  Google Scholar 

  13. Han, T.S.: Information-Spectrum Methods in Information Theory. Springer, Berlin (2003)

  14. Hayashi, M.: Practical evaluation of security for quantum key distribution. Phys. Rev. A 74(2), 022307 (2006)

    Article  ADS  Google Scholar 

  15. Hayashi, M.: Second-order asymptotics in fixed-length source coding and intrinsic randomness. IEEE Trans. Inf. Theory 54(10), 4619–4637 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  16. Hayashi, M.: Information spectrum approach to second-order coding rate in channel coding. IEEE Trans. Inf. Theory 55(11), 4947–4966 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  17. Hayashi, M., Tsurumaru, T.: Concise and tight security analysis of the Bennett–Brassard 1984 protocol with finite key lengths. New J. Phys. 14(9), 093014 (2012)

    Article  ADS  Google Scholar 

  18. Hu, X.-Y., Eleftheriou, E., Arnold, D.-M.: Regular and irregular progressive edge-growth tanner graphs. IEEE Trans. Inf. Theory 51(1), 386–398 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  19. Jouguet, P., Kunz-Jacques, S., Leverrier, A.: Long-distance continuous-variable quantum key distribution with a Gaussian modulation. Phys. Rev. A 84(6), 062317 (2011)

    Article  ADS  Google Scholar 

  20. Leverrier, A.: Theoretical study of continuous-variable quantum key distribution. Ph.D. thesis, Telecom ParisTech, Paris, France, (2009)

  21. Leverrier, A., Alléaume, R., Boutros, J., Zémor, G., Grangier, P.: Multidimensional reconciliation for continuous-variable quantum key distribution. Phys. Rev. A 77, 042325 (2008)

    Article  ADS  Google Scholar 

  22. Leverrier, A., Grangier, P.: Unconditional security proof of long-distance continuous-variable quantum key distribution with discrete modulation. Phys. Rev. Lett. 102(18), 180504 (2009)

    Article  ADS  Google Scholar 

  23. Leverrier, A., Grangier, P.: Continuous-variable quantum-key-distribution protocols with a non-Gaussian modulation. Phys. Rev. A 83(4), 042312 (2011)

    Article  ADS  Google Scholar 

  24. Lim, C.C.W., Portmann, C., Tomamichel, M., Renner, R., Gisin, N.: Device-independent quantum key distribution with local Bell test. Phys. Rev. X 3(3), 031006 (2013)

    Google Scholar 

  25. Lo, H.-K., Chau, H., Ardehali, M.: Efficient quantum key distribution scheme and a proof of its unconditional security. J. Cryptol. 18(2), 133–165 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  26. Martinez-Mateo, J., Elkouss, D., Martin, V.: Key reconciliation for high performance quantum key distribution. Sci. Rep. 3(1576), 1–6 (2013)

    MATH  Google Scholar 

  27. Mayers, D.: Unconditional security in quantum cryptography. J. ACM 48(3), 351–406 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  28. Morelos-Zaragoza, R.H.: The Art of Error Correcting Coding. Wiley, Hoboken (2006)

    Book  Google Scholar 

  29. Moroder, T., Curty, M., Lütkenhaus, N.: One-way quantum key distribution: simple upper bound on the secret key rate. Phys. Rev. A 74(5), 052301 (2006)

    Article  ADS  Google Scholar 

  30. Pacher, C., Lechner, G., Portmann, C., Maurhart, O., Peev, M.: Efficient QKD Postprocessing Algorithms. In: QCrypt 2012, Singapore, (2012)

  31. Pfister, C., Coles, P. J., Wehner, S., Lütkenhaus, N.: Sifting attacks in finite-size quantum key distribution. arXiv preprint arXiv:1506.07502 (2015)

  32. Polyanskiy, Y., Poor, H.V., Verdú, S.: Channel coding rate in the finite blocklength regime. IEEE Trans. Inf. Theory 56(5), 2307–2359 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  33. Renner, R.: Security of Quantum Key Distribution. Ph.D. thesis, ETH Zurich, (2005)

  34. Scarani, V., Bechmann-Pasquinucci, H., Cerf, N., Dušek, M., Lütkenhaus, N., Peev, M.: The security of practical quantum key distribution. Rev. Mod. Phys. 81(3), 1301–1350 (2009)

    Article  ADS  Google Scholar 

  35. Scarani, V., Renner, R.: Quantum cryptography with finite resources: unconditional security bound for discrete-variable protocols with one-way postprocessing. Phys. Rev. Lett. 100(20), 200501 (2008)

    Article  ADS  Google Scholar 

  36. Shor, P.W., Preskill, J.: Simple proof of security of the BB84 quantum key distribution protocol. Phys. Rev. Lett. 85(2), 441–444 (2000)

    Article  ADS  Google Scholar 

  37. Slepian, D., Wolf, J.: Noiseless coding of correlated information sources. IEEE Trans. Inf. Theory 19(4), 471–480 (1973)

    Article  MATH  MathSciNet  Google Scholar 

  38. Strassen, V.: Asymptotische Abschätzungen in Shannons Informationstheorie. In: Transactions of the Third Prague Conference on Information Theory, pp. 689–723. Prague (1962)

  39. Tan, V., Tomamichel, M.: The third-order term in the normal approximation for the AWGN channel. IEEE Trans. Inf. Theory 61(5), 2430–2438 (2015)

    Article  MATH  MathSciNet  Google Scholar 

  40. Tan, V.Y., Kosut, O.: On the dispersions of three network information theory problems. IEEE Trans. Inf. Theory 60(2), 881–903 (2014)

    Article  MATH  MathSciNet  Google Scholar 

  41. Tomamichel, M., Hayashi, M.: A hierarchy of information quantities for finite block length analysis of quantum tasks. IEEE Trans. Inf. Theory 59(11), 7693–7710 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  42. Tomamichel, M., Leverrier, A.: A rigorous and complete proof of finite key security of quantum key distribution. arXiv preprint arXiv:1506.08458 (2015)

  43. Tomamichel, M., Lim, C.C.W., Gisin, N., Renner, R.: Tight finite-key analysis for quantum cryptography. Nat. Commun. 3, 634 (2012)

    Article  ADS  Google Scholar 

  44. Tomamichel, M., Martinez-Mateo, J., Pacher, C., Elkouss, D.: Fundamental finite key limits for information reconciliation in quantum key distribution. In: IEEE International Symposium on Information Theory (ISIT), 2014, pp. 1469–1473. IEEE (2014)

  45. Tomamichel, M., Tan, V.Y.F.: A tight upper bound for the third-order asymptotics for most discrete memoryless channels. IEEE Trans. Inf. Theory 59(11), 7041–7051 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  46. Tyurin, I.: A refinement of the remainder in the Lyapunov theorem. Theory Probab. Appl. 56(4), 693–696 (2010)

    Article  MATH  Google Scholar 

  47. Varodayan, D., Aaron, A., Girod, B.: Rate-adaptive codes for distributed source coding. Signal Process. 86(11), 3123–3130 (2006)

    Article  MATH  Google Scholar 

  48. Walenta, N., Burg, A., Caselunghe, D., Constantin, J., Gisin, N., Guinnard, O., Houlmann, R., Junod, P., Korzh, B., Kulesza, N., Legré, M., Lim, C.W., Lunghi, T., Monat, L., Portmann, C., Soucarros, M., Thew, R.T., Trinkler, P., Trolliet, G., Vannel, F., Zbinden, H.: A fast and versatile quantum key distribution system with hardware key distillation and wavelength multiplexing. New J. Phys. 16(1), 013047 (2014)

    Article  ADS  Google Scholar 

  49. Wang, L., Colbeck, R., Renner, R.: Simple channel coding bounds. In: Proceedings of IEEE ISIT, pp.1804–1808. IEEE, (2009)

  50. Yassaee, M.H., Aref, M.R., Gohari, A.: A technique for deriving one-shot achievability results in network information theory. In: Proceedings of IEEE ISIT, (2013)

Download references

Acknowledgements

MT thanks N. Beaudry, S. Bratzik, F. Furrer, M. Hayashi, C.C.W. Lim, and V.Y.F. Tan for helpful comments and pointers to related work. MT is supported by an Australian Research Council Discovery Early Career Researcher Award (DECRA) fellowship. JM has been funded by the Spanish Ministry of Economy and Competitiveness through project Continuous Variables for Quantum Communications (CVQuCo), TEC2015-70406-R. CP has been funded by the Vienna Science and Technology Fund (WWTF) through project ICT10-067 (HiPANQ). DE was supported via STW and the NWO Vidi grant “Large quantum networks from small quantum devices”.

Author information

Authors and Affiliations

  1. Centre for Quantum Software and Information, University of Technology Sydney, Sydney, NSW, 2007, Australia

    Marco Tomamichel

  2. Center for Computational Simulation, Universidad Politecnica de Madrid, 28660, Boadilla del Monte, Spain

    Jesus Martinez-Mateo

  3. Digital Safety & Security Department, AIT Austrian Institute of Technology, Donau-City-Straße 1, 1220, Vienna, Austria

    Christoph Pacher

  4. QuTech, Delft University of Technology, P.O. Box 5046, 2600 GA, Delft, The Netherlands

    David Elkouss

Authors
  1. Marco Tomamichel
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jesus Martinez-Mateo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Christoph Pacher
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. David Elkouss
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Marco Tomamichel.

Additional information

Part of these results without the technical derivations were published in the proceedings of the International Symposium on Information Theory, Honolulu (2014) [44].

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tomamichel, M., Martinez-Mateo, J., Pacher, C. et al. Fundamental finite key limits for one-way information reconciliation in quantum key distribution. Quantum Inf Process 16, 280 (2017). https://doi.org/10.1007/s11128-017-1709-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-017-1709-5

Keywords

Search

Navigation