Abstract
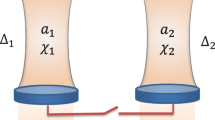
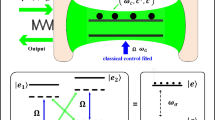
We consider and theoretically analyze a Bose-Einstein condensate (BEC) trapped inside an optomechanical system consisting of single-mode optical cavity with a moving end mirror. The BEC is formally analogous to a mirror driven by radiation pressure with strong nonlinear coupling. Such a nonlinear enhancement can make the oscillator display chaotic behavior. By establishing proper oscillator couplings, we find that this chaotic motion can be synchronized with other oscillators, even an oscillator network. We also discuss the scheme feasibility by analyzing recent experiment parameters. Our results provide a promising platform for the quantum signal transmission and quantum logic control, and they are of potential applications in quantum information processing and quantum networks.






Similar content being viewed by others
References
Vinokur, V.M., et al.: Superinsulator and quantum synchronization. Nature 452, 613–615 (2008)
Heinrich, G., Ludwig, M., Qian, J., Kubala, B., Marquardt, F.: Collective dynamics in optomechanical arrays. Phys. Rev. Lett. 107, 043603 (2011)
Zhirov, O.V., Shepelyansky, D.L.: Synchronization and bistability of a qubit coupled to a driven dissipative oscillator. Phys. Rev. Lett. 100, 014101 (2008)
Orth, P.P., Roosen, D., Hofstetter, W., Hur, K.L.: Dynamics, synchronization, and quantum phase transitions of two dissipative spins. Phys. Rev. B 82, 144423 (2010)
Zhirov, O.V., Shepelyansky, D.L.: Quantum synchronization and entanglement of two qubits coupled to a driven dissipative resonator. Phys. Rev. B 80, 014519 (2009)
Giorgi, G.L., Plastina, F., Francica, G., Zambrini, R.: Spontaneous synchronization and quantum correlation dynamics of open spin systems. Phys. Rev. A 88, 042115 (2013)
Lee, T.E., Sadeghpour, H.R.: Quantum synchronization of quantum van der Pol oscillators with trapped ions. Phys. Rev. Lett. 111, 234101 (2013)
Xu, M.H., Tieri, D.A., Fine, E.C., Thompson, J.K., Holland, M.J.: Synchronization of two ensembles of atoms. Phys. Rev. Lett. 113, 154101 (2014)
Walter, S., Nunnenkamp, A., Bruder, C.: Quantum synchronization of a driven self-sustained oscillator. Phys. Rev. Lett. 112, 094102 (2014)
Ying, L., Lai, Y.C., Grebogi, C.: Quantum manifestation of a synchronization transition in optomechanical systems. Phys. Rev. A 90, 053810 (2014)
Shlomi, K., et al.: Synchronization in an optomechanical cavity. Phys. Rev. E 91, 032910 (2015)
Samoylova, M., Piovella, N.M., Robb, G.R., Bachelard, R., Courteille, Ph.W.: Synchronization of Bloch oscillations by a ring cavity. arXiv:1503.05616
Mari, A., Farace, A., Didier, N., Giovannetti, V., Fazio, R.: Measures of quantum synchronization in continuous variable systems. Phys. Rev. Lett. 111, 103605 (2013)
Ameri, V., et al.: Mutual information as an order parameter for quantum synchronization. Phys. Rev. A 91, 012301 (2015)
Choi, S.H., Ha, S.Y.: Quantum synchronization of the Schrödinger-Lohe model. J. Phys. A Math. Theor. 47, 355104 (2014)
Manzano, G., Galve, F., Giorgi, G.L., Hernández-García, E., Zambrini, R.: Synchronization, quantum correlations and entanglement in oscillator networks. Sci. Rep. 3, 1439 (2013)
Li, W.L., Li, C., Song, H.S.: Quantum synchronization in an optomechanical system based on Lyapunov control. Phys. Rev. E 93, 062221 (2016)
Walter, S., Nunnenkamp, A., Bruder, C.: Quantum synchronization of two Van der Pol oscillators. Ann. Phys. (Leipzig) 527, 131 (2015)
Chacón, R., Palmero, F., Cuevas-Maraver, J.: Impulse-induced localized control of chaos in starlike networks. Phys. Rev. E 93, 062210 (2016)
Zhang, J., et al.: Quantum internet using code division multiple access. Sci. Rep. 3, 2211 (2013)
Li, W.L., Li, C., Song, H.S.: Quantum parameter identification for a chaotic atom ensemble system. Phys. Lett. A 380, 672–677 (2016)
Modi, K., Brodutch, A., Cable, H., Paterek, T., Vedral, V.: The classical-quantum boundary for correlations: discord and related measures. Rev. Mod. Phys. 84, 1655–1707 (2012)
Streltsov, A., Lee, S., Adesso, G.: Concentrating tripartite quantum information. Phys. Rev. Lett. 115, 030505 (2015)
Campbell, S., et al.: Global quantum correlations in finite-size spin chains. New J. Phys. 15, 043033 (2013)
Zhang, J., Zhang, Y., Yu, C.S.: entropic uncertainty relation and information exclusion relation for multiple measurements in the presence of quantum memory. Sci. Rep. 5, 11701 (2015)
Li, W.L., Zhang, F.Y., Li, C., Song, H.S.: Quantum synchronization in a star-type cavity QED network. Commun. Nonlinear Sci. Numer. Simul. 42, 121–131 (2017)
Li, W.L., Li, C., Song, H.S.: Quantum synchronization and quantum state sharing in irregular complex network. arXiv:1606.08113
Marquardt, F., Girvin, S.M.: Optomechanics. Physics 2, 40 (2009)
Aspelmeyer, M., Kippenberg, T.J., Marquardt, F.: Cavity optomechanics. Rev. Mod. Phys. 86, 1391–1452 (2014)
Wang, G., Huang, L., Lai, Y.C., Grebogi, C.: Nonlinear dynamics and quantum entanglement in optomechanical systems. Phys. Rev. Lett. 112, 110406 (2014)
Chan, J., et al.: Laser cooling of a nanomechanical oscillator into its quantum ground state. Nature 478, 89–92 (2011)
Verhagen, E., Deléglise, S., Weis, S., Schliesser, A., Kippenberg, T.J.: Quantum-coherent coupling of a mechanical oscillator to an optical cavity mode. Nature 482, 63–67 (2012)
Zhang, W.Z., Cheng, J., Liu, J.Y., Zhou, L.: Controlling photon transport in the single-photon weak-coupling regime of cavity optomechanics. Phys. Rev. A 91, 063836 (2015)
Zhang, Y., Zhang, J., Yu, C.S.: Photon statistics on the extreme entanglement. Sci. Rep. 6, 24098 (2016)
Cheng, J., Zhang, W.Z., Zhou, L., Zhang, W.: Preservation macroscopic entanglement of optomechanical systems in non-Markovian environment. Sci. Rep. 6, 23678 (2016)
Mari, A., Eisert, J.: Gently modulating optomechanical systems. Phys. Rev. Lett. 103, 213603 (2009)
Farace, A., Giovannetti, V.: Enhancing quantum effects via periodic modulations in optomechanical systems. Phys. Rev. A 86, 013820 (2012)
Lü, L., et al.: Determination of configuration matrix element and outer synchronization among networks with different topologies. Physica A 461, 833–839 (2016)
Lü, L., Chen, L.S., Bai, S.Y., Li, G.: A new synchronization tracking technique for uncertain discrete network with spatiotemporal chaos behaviors. Physica A 460, 314–325 (2016)
Sun, H.J., Cao, H.J.: Synchronization of two identical and non-identical Rulkov models. Commun. Nonlinear Sci. Numer. Simul. 40, 15–27 (2016)
Shepelev, I.A., Slepnev, A.V., Vadivasova, T.E.: Different synchronization characteristics of distinct types of traveling waves in a model of active medium with periodic boundary conditions. Commun. Nonlinear Sci. Numer. Simul. 38, 206–217 (2016)
Zhang, K., Chen, W., Bhattacharya, M., Meystre, P.: Hamiltonian chaos in a coupled BEC-optomechanical-cavity system. Phys. Rev. A 81, 013802 (2010)
Yasir, K.A., Liu, W.M.: Tunable bistability in hybrid Bose-Einstein condensate optomechanics. Sci. Rep. 5, 10612 (2015)
Horak, P., Barnett, S.M., Ritsch, H.: Coherent dynamics of Bose-Einstein condensates in high-finesse optical cavities. Phys. Rev. A 61, 033609 (2000)
Vitali, D., et al.: Optomechanical entanglement between a movable mirror and a cavity field. Phys. Rev. Lett. 98, 030405 (2007)
Gardiner, C.W., Zoller, P.: Quantum Noise. Chapter 3. Springer, Berlin (2000). Please check and confirm the book title for reference [46] is correct
Giovannetti, V., Vitali, D.: Phase-noise measurement in a cavity with a movable mirror undergoing quantum Brownian motion. Phys. Rev. A 63, 023812 (2001)
Buchmann, L.F., Wright, E.M., Meystre, P.: Phase conjugation in quantum optomechanics. Phys. Rev. A 88, 041801(R) (2013)
Weiss, T., Kronwald, A., Marquardt, F.: Noise-induced transitions in optomechanical synchronization. New. J. Phys. 18, 013043 (2016)
Bakemeier, L., Alvermann, A., Fehske, H.: Route to Chaos in optomechanics. Phys. Rev. Lett. 114, 013601 (2015)
Lü, L., Li, C., Li, G., Sun, A., Yan, Z., Rong, T., Gao, Y.: Synchronization transmission of laser pattern signal within uncertain switched network. Commun. Nonlinear. Sci. Numer. Simulat. 47, 267 (2017)
Lü, X.Y., Jing, H., Ma, J.Y., Wu, Y.: PT-symmetry-breaking chaos in optomechanics. Phys. Rev. Lett. 114, 253601 (2015)
Lü, L., Li, C.R., Chen, L.S.: Projective synchronization of the small world delayed network with uncertainty. Nonlinear Dyn. 76, 1633 (2014)
Galve, F., Giorgi, G.L., Zambrini, R.: Quantum correlations and synchronization measures. arXiv:1610.05060
Lü, L., Li, C.R., Chen, L.S., Zhao, G.N.: New technology of synchronization for the uncertain dynamical network with the switching topology. Nonlinear Dyn. 86(1), 655–666 (2016)
Schultz, P., et al.: Tweaking synchronization by connectivity modifications. Phys. Rev. E 93, 062211 (2016)
Mahata, S., Das, S., Gupte, N.: Synchronization in area-preserving maps: effects of mixed phase space and coherent structures. Phys. Rev. E 93, 062212 (2016)
Ludwig, M., Kubala, B., Marquardt, F.: The optomechanical instability in the quantum regime. New J. Phys. 10, 095013 (2008)
Acknowledgements
All authors thank Jiong Cheng, Wenzhao Zhang and Yang Zhang for the useful discussion. This research was supported by the National Natural Science Foundation of China (Grant Nos. 11574041 and 11175033) and the Fundamental Research Funds for the Central Universities (DUT13LK05).
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix
Simplification of the Bose-Einstein condensate Hamiltonian
The total Hamiltonian corresponding to such a system can be expressed as \(\hat{H}=\hat{H}_\mathrm{{c}}+\hat{H}_\mathrm{{a}}+\hat{H}_\mathrm{{d}}\), where
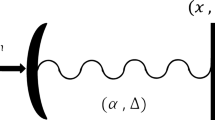
is the standard Hamiltonian of optomechanical system with the radiation pressure term \(\hbar {g}_0\hat{a}^\dagger \hat{a}\hat{q}\) [28, 29]. \(\hat{H}_\mathrm{{a}}\) describes the BEC and its interaction with the light field and correspondingly, \(H_\mathrm{{d}}\) denotes the Hamiltonian of the thermal reservoir which hence to dissipation. In Eq. (1), \(\hat{a}\) (\(\hat{a^{\dagger }}\)) is the bosonic annihilation (creation) operator for the optical cavity field, \(\hat{q}\) (\(\hat{p}\)) is the position (momentum) operator for the oscillator. \(\omega _\mathrm{{c}}\), \(\omega _\mathrm{{p}}\) and \(\omega _\mathrm{{m}}\) are the intrinsic frequencies of the cavity field, pump field and the oscillator, respectively. \(g_0\) is the single-photon coupling coefficient satisfying \(g_0=(\omega _\mathrm{{c}}/L)\sqrt{\hbar /m\omega _\mathrm{{m}}}\), where L and m are the cavity length and the oscillator mass, respectively [28]. E is the driving intensity of the pump field. In the rotating frame, \(\hat{H}_\mathrm{{c}}\) is given explicitly by
via introducing cavity-pump detuning \(\Delta _\mathrm{{c}}=\omega _\mathrm{{p}}-\omega _\mathrm{{c}}\).
The BEC trapped in a cavity optomechanical system can be simplified as a one-dimensional motion model. Previous works have proved that the internal excited state dynamic of the atom can be adiabatically eliminated if the light-atom detuning \(\Delta _\mathrm{{a}}\) is large enough [44]. Here, we assume that the atom spontaneous emission can be ignored, in addition, two-body interaction can also be neglected if atomic density is low enough. Therefore, \(\hat{H}_\mathrm{{a}}\) becomes [42]
where \(\hat{\Psi }(x)\) (\(\hat{\Psi }^{\dagger }(x)\)) is a bosonic field annihilation (creation) operator and \(m_\mathrm{{a}}\) is the atomic mass. In this expression, \(U_0\) is the far off-resonant vacuum Rabi frequency and \(k=\omega _\mathrm{{p}}/c\) is the wave number of the light field. Here, we consider the optical field is so weak that the momentum side modes of the BEC are generated at \(\pm 2\hbar k\). Then, the field operator \(\hat{\Psi }(x)\) can be expanded as
Here, the bosonic annihilation operators \(\hat{c_0}\) and \(\hat{c_2}\) are, respectively, the zero-momentum state and side-mode component. By substituting Eq. (13) into Eq. (12) and finishing the integral, the Hamiltonian \(\hat{H}_\mathrm{{a}}\) can be simplified as
where \(\omega _\mathrm{{r}}=\hbar k^2/2 m_\mathrm{{a}}\) is the effective frequency and \(\hat{c}^\dagger _0\hat{c}_0\) (\(\hat{c}^\dagger _2\hat{c}_2\)) is the corresponding particle number operator of the zero-momentum mode (the side mode). Here, we assume that \(\hat{c}^\dagger _0\hat{c}_0=N-\hat{c}^\dagger _2\hat{c}_2\), i.e., the particle loss is ignored and the population of zero-momentum mode is far greater than that of the side mode. If N is large enough, the zero-momentum mode can be regarded as a classical field, i.e., \(\hat{c}_0\) and \(\hat{c}^\dagger _0\) are thought as the c-numbers \(\sqrt{N}\). With this condition, Eq. (14) becomes
and the total Hamiltonian of the system can be gained
Rights and permissions
About this article
Cite this article
Li, W., Li, C. & Song, H. Quantum synchronization of chaotic oscillator behaviors among coupled BEC–optomechanical systems. Quantum Inf Process 16, 80 (2017). https://doi.org/10.1007/s11128-017-1517-y
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11128-017-1517-y