Skip to main content
SpringerLink
Log in

Convergence analysis of high-order compact alternating direction implicit schemes for the two-dimensional time fractional diffusion equation

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

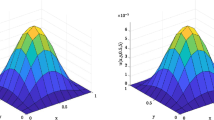
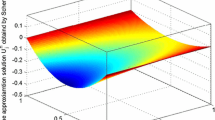
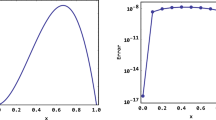
High-order compact finite difference method with operator-splitting technique for solving the two dimensional time fractional diffusion equation is considered in this paper. The Caputo derivative is evaluated by the L1 approximation, and the second order derivatives with respect to the space variables are approximated by the compact finite differences to obtain fully discrete implicit schemes. Alternating Direction Implicit (ADI) method is used to split the original problem into two separate one dimensional problems. One scheme is given by replacing the unknowns by the values on the previous level directly and a correction term is added for another scheme. Theoretical analysis for the first scheme is discussed. The local truncation error is analyzed and the stability is proved by the Fourier method. Using the energy method, the convergence of the compact finite difference scheme is proved. Numerical results are provided to verify the accuracy and efficiency of the two proposed algorithms. For the order of the temporal derivative lies in different intervals \(\left(0,\frac{1}{2}\right)\) or \(\left[\frac{1}{2},1\right)\), corresponding appropriate scheme is suggested.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Brunner, H., Ling, L., Yamamoto, M.: Numerical simulations of 2D fractional subdiffusion problems. J. Comput. Phys. 229, 6613–6622 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  2. Chen, C.M., Liu, F., Turner, I., Anh, V.: A Fourier method for the fractional diffusion equation describing sub-diffusion. J. Comput. Phys. 227, 886–897 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chen, S., Liu, F., Zhuang, P., Anh, V.: Finite difference approximations for the fractional Fokker–Plank equation. Appl. Math. Model. 33, 256–273 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  4. Chen, C.M., Liu, F., Turner, I., Anh, V.: Numerical schemes and multivariate extrapolation of a two-dimensional anomalous sub-diffusion equation. Numer. Algorithms 54, 1–21 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  5. Cui, M.R.: Compact finite difference method for the fractional diffusion equation. J. Comput. Phys. 228, 7792–7804 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  6. Cui, M.R.: Compact alternating direction implicit method for two-dimensional time fractional diffusion equation. J. Comput. Phys. 231, 2621–2633 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  7. Dendy, J.E. Jr.: Alternating direction methods for nonlinear time-dependent problems. SIAM J. Numer. Anal. 14, 313–326 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  8. Douglas, J. Jr.: On the numerical integration of u xx  + u yy  = u t by implicit methods. J. Soc. Ind. Appl. Math. 3, 42–65 (1955)

    Article  MATH  Google Scholar 

  9. Douglas, J. Jr., Gunn, J.E.: A general formulation of alternating direction method, part I. Parabolic and hyperbolic problems. Numer. Math. 6, 428–453 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  10. Ervin, V.J., Heuer, N., Roop, J.P.: Numerical approximation of a time dependent, nonlinear, space-fractional diffusion equation. SIAM J. Numer. Anal. 45, 572–591 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  11. Gao, G.H., Sun, Z.Z.: A compact finite difference scheme for the fractional sub-diffusion equations. J. Comput. Phys. 230, 586–595 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  12. Hirsch, R.S.: Higher order accurate difference solutions of fluid mechanics problems by a compact difference technique. J. Comput. Phys. 24, 90–109 (1975)

    Article  Google Scholar 

  13. Langlands, T.A.M., Henry, B.I.: The accuracy and stability of an implicit solution method for the fractional diffusion equation. J. Comput. Phys. 205, 719–736 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  14. Lele, S.K.: Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103, 16–42 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  15. Li, C.P., Chen, A., Ye, J.J.: Numerical approaches to fractional calculus and fractional ordinary differential equation. J. Comput. Phys. 230, 3352–3368 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  16. Li, D.Y., Chen, G.N.: Introduction to the Finite Difference Methods for Parabolic Equations. Science Press, Beijing (1995) (in Chinese)

    Google Scholar 

  17. Li, X., Xu, Ch.: A space-time spectral method for the time fractional diffusion equation. SIAM J. Numer. Anal. 47, 2108–2131 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  18. Liao, H.L., Sun, Z.Z.: Maximum norm error bounds of ADI and compact ADI methods for solving parabolic equations. Numer. Methods Partial Differ. Equ. 26, 37–60 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  19. Liao, W., Zhu, J., Khaliq, A.Q.M.: An efficient high-order algorithm for solving systems of reaction-diffusion equations. Numer. Methods Partial Differ. Equ. 18, 340–354 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  20. Meerschaert, M.M., Scheffler, H.P., Tadjeran, C.: Finite difference methods for two-dimensional fractional dispersion equation. J. Comput. Phys. 211, 249–261 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  21. Metzler, R., Klafter, J.: The random walk’s guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339, 1–77 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  22. Miller, K.S., Ross, B.: An Introduction to the Fractional Calculus and Fractional Differential Equations. Wiley, New York (1993)

    MATH  Google Scholar 

  23. Oldham, K., Spanier, J.: The Fractional Calculus: Theory and Applications of Differentiation and Integration of Arbitray Order. Academic Press, New York (1974)

    Google Scholar 

  24. Peaceman, D.W., Rachford, H.H. Jr.: The numerical solution of parabolic and elliptic differential equations. J. Soc. Ind. Appl. Math. 3, 28–41 (1955)

    Article  MathSciNet  MATH  Google Scholar 

  25. Podlubny, I.: Fractional Differential Equations, An Introduction to Fractional Derivatives, Fractional Differential Equations, Some Methods of Their Solution and Some of Their Applications. Academic Press, San Diego (1999)

    Google Scholar 

  26. Podlubny, I.: Matrix approach to discrete fractional calculus. Fract. Calc. Appl. Anal. 3, 359–386 (2000)

    MathSciNet  MATH  Google Scholar 

  27. Podlubny, I., Chechkin, A., Skovranek, T., Chen, Y., Jara, B.M.V.: Matrix approach to discrete fractional calculus II: partial fractional differential equations. J. Comput. Phys. 228, 3137–3153 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  28. Ramos, J.I.: Implicit, compact, linearized θ-methods with factorization for multidimensional reaction-diffusion equations. Appl. Math. Comput. 94, 17–43 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  29. Samarskii, A.A., Andreev, V.B.: Difference Methods for Elliptic Equations, Nauka, Moscow, 1976 (Translated into Chinese). Science Press, Beijing (1984)

    Google Scholar 

  30. Shen, S., Liu, F., Anh, V.: Numerical approximations and solution techniques for the space-time RieszCCaputo fractional advection-diffusion equation. Numer. Algorithms 56, 383–403 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  31. Sun, Z.Z., Li, X.L.: A compact alternating direction implicit difference method for reaction diffusion equations. Math. Numer. Sin. 27, 209–224 (2005) (in Chinese)

    MathSciNet  MATH  Google Scholar 

  32. Tadjeran, C., Meerschaert, M.M.: A second-order accurate numerical method for the two-dimensional fractional diffusion equation. J. Comput. Phys. 220, 813–823 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  33. Thomas, J.W.: Numerical partial differential equations: finite difference methods. In: Texts in Applied Mathematics, vol. 22. Springer, Berlin (1995)

    Google Scholar 

  34. Wang, H., Wang, K., Sircar, T.: A direct O(N log2 N) finite difference method for fractional diffusion equations. J. Comput. Phys. 229, 8095–8104 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  35. Wang, H., Wang, K.: An O(N log2 N) alternating-direction finite difference method for two-dimensional fractional diffusion equations. J. Comput. Phys. 230, 7830–7839 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  36. Yanenko, N.N.: The Method of Fractional Steps, The Solution of Problems of Mathematical Physics in Several Variables. Springer, Berlin (1971)

    Book  MATH  Google Scholar 

  37. Yuste, S.B., Acedo, L.: An explicit finite difference method and a new von Neumann-type stability analysis for fractional diffusion equations. SIAM J. Numer. Anal. 42, 1862–1874 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  38. Zhang, Y.N., Sun, Z.Z.: Alternating direction implicit schemes for the two-dimensional fractional sub-diffusion equation. J. Comput. Phys. 230, 8713–8728 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  39. Zhuang, P., Liu, F., Anh, V., Turner, I.: Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 47, 1760–1781 (2009)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics, Shandong University, Jinan, 250100, Shandong, China

    Mingrong Cui

Authors
  1. Mingrong Cui
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mingrong Cui.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Cui, M. Convergence analysis of high-order compact alternating direction implicit schemes for the two-dimensional time fractional diffusion equation. Numer Algor 62, 383–409 (2013). https://doi.org/10.1007/s11075-012-9589-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-012-9589-3

Keywords

Mathematics Subject Classifications (2010)

Search

Navigation