Skip to main content
SpringerLink
Log in

Multi-lump formations from lump chains and plane solitons in the KP1 equation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

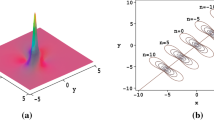
We show that complex higher-order lump patterns can be constructed in two different ways within the Kadomtsev–Petviashvili (KP1) equation which describes nonlinear wave processes in media with positive dispersion. In the first approach, we start with solutions describing stationary moving higher-order lump chains. By degenerating these solutions, we obtain first coupled lump chains which reduce then to multi-lump bound states in the limit when the period of lump chains goes to infinity. In another approach, a skilful technique is exploited to derive multi-lump bound states directly from the N-soliton solution of the KP1 equation presented in the Hirota form. It is shown then that through the proper selection of soliton parameters, these higher-order solutions can be reduced to the various lump patterns, such as triangular, polygonal patterns, and so on. The suggested approaches can be extended to another one- and multi-dimensional integrable systems to derive complex bound states and rogue waves.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Data availability

All data generated or analyzed during this study are included in this published article.

References

  1. Kadomtsev, B.B., Petviashvili, V.I.: On the stability of solitary waves in weakly dispersing media. Sov. Phys. Dokl. 15, 539–541 (1970)

    MATH  Google Scholar 

  2. Ablowitz, M.J., Segur, H.: Solitons and the Inverse Scattering Transform. SIAM, Philadelphia, PA (1981)

    MATH  Google Scholar 

  3. Dryuma, V.S.: Analytic solution of the two-dimensional Korteweg–de Vries (KdV) equation. JETP Lett. 19, 753–754 (1974)

    Google Scholar 

  4. Zakharov, V.E., Shabat, A.B.: A scheme for integrating the nonlinear equations of mathematical physics by the method of the inverse scattering problem. I. Funct. Anal. Appl. 8, 226–235 (1974)

    MATH  Google Scholar 

  5. Zakharov, V.E.: Turbulence in integrable systems. Stud. Appl. Math. 122, 219–234 (2009)

    MathSciNet  MATH  Google Scholar 

  6. Krichever, I.M.: Rational solutions of the Kadomtsev–Petviashvili equation and integrable systems of \(N\) particles on a line. Funct. Anal. Appl. 12, 59–61 (1978)

    MATH  Google Scholar 

  7. Manakov, S.V., Zakharov, V.E., Bordag, L.A., Its, A.R., Matveev, V.B.: Two-dimensional solitons of the Kadomtsev–Petviashvili equation and their interaction. Phys. Lett. A 63, 205–206 (1977)

    Google Scholar 

  8. Pelinovskii, D.E., Stepanyants, Y.A.: New multisoliton solutions of the Kadomtsev–Petviashvili equation. JETP Lett. 57, 24–28 (1993)

    Google Scholar 

  9. Kajiwara, K., Ohta, Y.: Determinant structure of the rational solutions for the Painlevé II equation. J. Math. Phys. 37, 4693–4704 (1996)

    MathSciNet  MATH  Google Scholar 

  10. Kodama, Y.: KP Solitons and the Grassmannians: Combinatorics and Geometry of Two-Dimensional Wave Patterns. SpringerBriefs in Mathematical Physics. Springer, Berlin (2017)

    MATH  Google Scholar 

  11. Petviashvili, V.I.: Equation of an extraordinary soliton. Fiz. Plazmy 2, 469–472 (1976)

    Google Scholar 

  12. Pelinovsky, D.E., Stepanyants, Y.A.: Convergence of Petviashvili’s iteration method for numerical approximation of stationary solutions of nonlinear wave equations. SIAM J. Numer. Anal. 42(3), 1110–1124 (2004)

    MathSciNet  MATH  Google Scholar 

  13. Satsuma, J., Ablowitz, M.J.: Two-dimensional lumps in nonlinear dispersive systems. J. Math. Phys. 20, 1496–1503 (1979)

    MathSciNet  MATH  Google Scholar 

  14. Zhang, Z., Li, B., Chen, J.C., Guo, Q.: The nonlinear superposition between anomalous scattering of lumps and other waves for KPI equation. Nonlinear Dyn. 108, 4157–4169 (2022)

    Google Scholar 

  15. Gorshkov, K.A., Pelinovsky, D.E., Stepanyants, Y.A.: Normal and anomalous scattering, formation and decay of bound states of two-dimensional solitons described by the Kadomtsev–Petviashvili equation. JETP 104, 2704–2720 (1993)

    Google Scholar 

  16. Hu, W.C., Huang, W.H., Lu, Z.M., Stepanyants, Y.A.: Interaction of multi-lumps within the Kadomtsev–Petviashvili equation. Wave Motion 77, 243–256 (2018)

    MathSciNet  MATH  Google Scholar 

  17. Lu, Z.M., Tian, E.M., Grimshaw, R.: Interaction of two lump solitons described by the Kadomtsev–Petviashvili I equation. Wave Motion 40, 123–135 (2004)

    MathSciNet  MATH  Google Scholar 

  18. Abramyan, L.A., Stepanyants, Y.A.: Two-dimensional multisolitons: stationary solutions of Kadomtsev–Petviashvili equation. Radiophys. Quantum Electron. 28(1), 20–26 (1985)

    MathSciNet  Google Scholar 

  19. Chakravarty, S., Zowada, M.: Dynamics of KPI lumps. J. Phys. A-Math. Theor. 55, 195701 (2022)

    MathSciNet  MATH  Google Scholar 

  20. Chakravarty, S., Zowada, M.: Classification of KPI lumps. J. Phys. A-Math. Theor. 55, 215701 (2022)

    MathSciNet  MATH  Google Scholar 

  21. Stepanyants, Y.A., Zakharov, D.V., Zakharov, V.E.: Lump interactions with plane solitons. Radiophys. Quantum Electron. 64(10), 665–680 (2022)

    Google Scholar 

  22. Wen, X.Y., Yan, Z.Y.: Higher-order rational solitons and rogue-like wave solutions of the (2+ 1)-dimensional nonlinear fluid mechanics equations. Commun. Nonlinear Sci. Numer. Simul. 43, 311–329 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Dong, J.Y., Ling, L.M., Zhang, X.E.: Kadomtsev–Petviashvili equation: one-constraint method and lump pattern. Physica D 432, 133152 (2022)

    MathSciNet  MATH  Google Scholar 

  24. Yang, B., Yang, J.K.: Pattern transformation in higher-order lumps of the Kadomtsev–Petviashvili I equation. J. Nonlinear Sci. 32, 52 (2022)

    MathSciNet  MATH  Google Scholar 

  25. Zhang, Z., Li, B., Chen, J.C., Guo, Q., Stepanyants, Y.A.: Degenerate lump interactions within the Kadomtsev–Petviashvili equation. Commun. Nonlinear Sci. Numer. Simul. 112, 106555 (2022)

    MathSciNet  MATH  Google Scholar 

  26. Clarkson, P.A., Dowie, E.: Rational solutions of the Boussinesq equation and applications to rogue waves. Trans. Math. Appl. 1, tnx003 (2017)

    MathSciNet  MATH  Google Scholar 

  27. Galkin, V.M., Pelinovsky, D.E., Stepanyants, Y.A.: The structure of the rational solutions to the Boussinesq equation. Physica D 80, 246–255 (1995)

    MathSciNet  MATH  Google Scholar 

  28. Tajiri, M., Murakami, Y.: Rational growing mode: exact solutions to the Boussinesq equation. J. Phys. Soc. Jpn. 60, 2791–2792 (1991)

    MathSciNet  Google Scholar 

  29. Rao, J.G., Liu, Y.B., Qian, C., He, J.S.: Rogue waves and hybrid solutions of the Boussinesq equation. Z. Naturforsch. A 72, 307–314 (2017)

    Google Scholar 

  30. Yang, B., Yang, J.K.: General rogue waves in the Boussinesq equation. J. Phys. Soc. Jpn. 89, 024003 (2020)

    Google Scholar 

  31. Yang, B., Yang, J.K.: Rogue wave patterns in the nonlinear Schrödinger equation. Physica D 419, 132850 (2021)

    MATH  Google Scholar 

  32. Yang, B., Yang, J.K.: Universal rogue wave patterns associated with the Yablonskii–Vorob’ev polynomial hierarchy. Physica D 425, 132958 (2021)

    MathSciNet  MATH  Google Scholar 

  33. Yang, B., Yang, J.K.: Rogue waves in (2+1)-dimensional three-wave resonant interactions. Physica D 432, 133160 (2022)

    MathSciNet  MATH  Google Scholar 

  34. Yuan, F., Cheng, Y., He, J.S.: Degeneration of breathers in the Kadomttsev–Petviashvili I equation. Commun. Nonlinear Sci. Numer. Simul. 83, 105027 (2020)

    MathSciNet  MATH  Google Scholar 

  35. Bogdanov, L.V., Zakharov, V.E.: The Boussinesq equation revisited. Physica D 165, 137–162 (2002)

    MathSciNet  MATH  Google Scholar 

  36. Hirota, R.: The Direct Method in Soliton Theory. Cambridge University Press, Cambridge (2004)

    MATH  Google Scholar 

  37. Ablowitz, M.J., Satsuma, J.: Solitons and rational solutions of nonlinear evolution equations. J. Math. Phys. 19, 2180–2186 (1978)

    MathSciNet  MATH  Google Scholar 

  38. Gdanov, S.K., Trubnikov, B.A.: Soliton chains in a plasma with magnetic viscosity. JETP Lett. 39, 129–132 (1984)

    Google Scholar 

  39. Zaitsev, A.A.: Formation of stationary nonlinear waves by superposition of solitons. Sov. Phys. Dokl. 28, 720–722 (1983)

    Google Scholar 

  40. Wang, L.H., He, J.S., Xu, H., Wang, J., Porsezian, K.: Generation of higher-order rogue waves from multibreathers by double degeneracy in an optical fiber. Phys. Rev. E 95, 042217 (2017)

    MathSciNet  Google Scholar 

  41. Zhang, Z., Li, B., Chen, J.C., Guo, Q.: Construction of higher-order smooth positons and breather positons via Hirota’s bilinear method. Nonlinear Dyn. 105, 2611–2618 (2021)

    Google Scholar 

  42. Zhang, Z., Chen, J.C., Guo, Q.: Multiple-pole solutions and degeneration of breather solutions to the focusing nonlinear Schrödinger equation. Commun. Theor. Phys. 74, 045002 (2022)

    Google Scholar 

  43. Guo, B.L., Ling, L.M., Liu, Q.P.: Nonlinear Schrödinger equation: generalized Darboux transformation and rogue wave solutions. Phys. Rev. E 85, 026607 (2012)

    Google Scholar 

  44. He, J.S., Zhang, H.R., Wang, L.H., Porsezian, K., Fokas, A.S.: Generating mechanism for higher-order rogue waves. Phys. Rev. E 87, 052914 (2013)

    Google Scholar 

  45. Lester, C., Gelash, A., Zakharov, D., Zakharov, V.: Lump chains in the KP-I equation. Stud. Appl. Math. 147, 1425–1442 (2021)

    MathSciNet  MATH  Google Scholar 

  46. Rao, J.G., He, J.S., Malomed, B.A.: Resonant collisions between lumps and periodic solitons in the Kadomtsev–Petviashvili I equation. J. Math. Phys. 63, 013510 (2022)

    MathSciNet  MATH  Google Scholar 

  47. Rao, J.G., Chow, K.W., Mihalache, D., He, J.S.: Completely resonant collision of lumps and line solitons in the Kadomtsev–Petviashvili I equation. Stud. Appl. Math. 147, 1007–1035 (2021)

    MathSciNet  MATH  Google Scholar 

  48. Zhang, Z., Li, B., Chen, J., Guo, Q., Stepanyants, Y.: Peculiarities of resonant interactions of lump chains within the KP1 equation. Phys. Scr. submitted 30 May (2022)

Download references

Acknowledgements

This research is supported by the Natural Science Foundation of Guangdong Province of China (No. 2021A1515012214), the Science and Technology Program of Guangzhou (No. 2019050001), National Natural Science Foundation of China (No. 12175111) and K.C.Wong Magna Fund in Ningbo University. The authors sincerely thank Dr. Junchao Chen (Lishui University) for the discussions. Yu.A. Stepanyants acknowledges the funding provided by the Council of the grants of the President of the Russian Federation for the state support of Leading Scientific Schools of the Russian Federation (Project No. NSH–70.2022.1.5).

Funding

This study was funded by the Natural Science Foundation of Guangdong Province of China (No. 2021A1515012214) and the Science and Technology Program of Guangzhou (No. 2019050001).

Author information

Authors and Affiliations

  1. Guangdong Provincial Key Laboratory of Nanophotonic Functional Materials and Devices, South China Normal University, Guangzhou, 510631, People’s Republic of China

    Zhao Zhang & Qi Guo

  2. School of Mathematical Sciences, Dalian University of Technology, Dalian, 116024, People’s Republic of China

    Xiangyu Yang

  3. School of Mathematics and Statistics, Ningbo University, Ningbo, 315211, People’s Republic of China

    Biao Li

  4. School of Mathematics, Physics and Computer Sciences, University of Southern Queensland, Toowoomba, QLD, 4350, Australia

    Yury Stepanyants

  5. Department of Applied Mathematics, Nizhny Novgorod State Technical University, n.a. R.E. Alekseev, 24 Minin St., Nizhny Novgorod, Russia, 603950

    Yury Stepanyants

Authors
  1. Zhao Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiangyu Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Biao Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Qi Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Yury Stepanyants
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Qi Guo.

Ethics declarations

Conflict of interest

We declare that we have no conflict of interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Z., Yang, X., Li, B. et al. Multi-lump formations from lump chains and plane solitons in the KP1 equation. Nonlinear Dyn 111, 1625–1642 (2023). https://doi.org/10.1007/s11071-022-07903-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07903-8

Keywords

Search

Navigation