Skip to main content
SpringerLink
Log in

Mean-square stability of the zero equilibrium of the nonlinear delay differential equation: Nicholson’s blowflies application

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

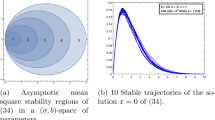
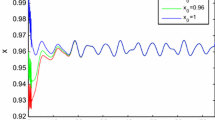
We are concerned about the stochastic nonlinear delay differential equation. The stochasticity arises from the white Gaussian noise, which is the time derivative of the standard Brownian motion. The main objective of this paper is to introduce a new technique using the Lyapunov functional for the study of stability of the zero solution of the stochastic delay differential system. Constructing a new appropriate deterministic system in the neighborhood of the origin is an effective way to investigate the necessary and sufficient conditions of stability in the sense of the mean square. Nicholson’s blowflies equation is one of the major problems in ecology; necessary conditions for the possible extinction of the Nicholson’s blowflies population are investigated. We support our theoretical results by providing areas of stability and some numerical simulations of the solution of the system using the Euler–Maruyama scheme, which is mean square stable Maruyama (Rendiconti del Circolo Matematico di Palermo 4(1):48, 1955), Cao et al. (Appl Math Comput 159(1):127–135, 2004).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Availability of data and materials

The data sets generated and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Maruyama, G.: Continuous markov processes and stochastic equations. Rendiconti del Circolo Matematico di Palermo 4(1), 48 (1955)

    Article  MathSciNet  MATH  Google Scholar 

  2. Cao, W., Liu, M., Fan, Z.: Ms-stability of the euler-maruyama method for stochastic differential delay equations. Appl. Math. Comput. 159(1), 127–135 (2004)

    MathSciNet  MATH  Google Scholar 

  3. Gopalsamy, K.: Stability and Oscillations in Delay Differential Equations of Population Dynamics, 74th edn. Springer, Berlin (2013)

    MATH  Google Scholar 

  4. Győri, I.: Delay differential and integro-differential equations in biological compartment models. Syst. Sci. 8(2–3), 167–187 (1982)

    MathSciNet  MATH  Google Scholar 

  5. Kuang, Y.: Delay Differential Equations: With Applications in Population Dynamics. Academic Press, Cambridge (1993)

    MATH  Google Scholar 

  6. Brännström, Å.: Modelling Animal Populations: Tools and Techniques. Doctoral thesis. Umeå University, Faculty of Science and Technology, Mathematics and Mathematical Statistics (2004)

  7. Rodney David, D.: Ordinary and Delay Differential Equations, 20th edn. Springer, Berlin (2012)

    Google Scholar 

  8. Erneux, T.: Applied Delay Differential Equations, 3rd edn. Springer, Berlin (2009)

    MATH  Google Scholar 

  9. Gopalsamy, K., Zhang, B.G.: On delay differential equations with impulses. J. Math. Anal. Appl. 139(1), 110–122 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  10. Hale, J.K., Verduyn Lunel, S.M., Verduyn, L.S., Lunel, S.M.: Introduction to Functional Differential Equations, vol. 99. Springer, Berlin (1993)

    Book  MATH  Google Scholar 

  11. Makay, G.: On the asymptotic stability of the solutions of functional differential equations with infinite delay. J. Differ. Equ. 108(1), 139–151 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  12. Taylor, S.R.: Probabilistic Properties of Delay Differential Equations. arXiv preprint arXiv:1909.02544 (2019)

  13. El-Metwally, H., Sohaly, M.A., Elbaz, I.M.: Stochastic global exponential stability of disease-free equilibrium of hiv/aids model. Eur. Phys. J. Plus 135(10), 1–14 (2020)

    Article  Google Scholar 

  14. Mao, X.: Numerical solutions of stochastic functional differential equations. LMS J. Comput. Math. 6, 141–161 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  15. Ma, L., Ning, X., Huo, X., Zhao, X.: Adaptive finite-time output-feedback control design for switched pure-feedback nonlinear systems with average dwell time. Nonlinear Anal. Hybrid Syst. 37, 100908 (2020)

    Article  MathSciNet  Google Scholar 

  16. Cai, J., Rui, Y., Wang, B., Mei, C., Shen, L.: Decentralized event-triggered control for interconnected systems with unknown disturbances. J. Franklin Inst. 357(3), 1494–1515 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  17. Shaikhet, L.: Lyapunov Functionals and Stability of Stochastic Functional Differential Equations. Springer, Berlin (2013)

    Book  MATH  Google Scholar 

  18. Wang, W., Shi, C., Chen, W.: Stochastic nicholson-type delay differential system. Int. J. Control 94, 1–8 (2019a)

    MathSciNet  Google Scholar 

  19. Wang, W., Wang, L., Chen, W.: Stochastic nicholson’s blowflies delayed differential equations. Appl. Math. Lett. 87, 20–26 (2019b)

    Article  MathSciNet  MATH  Google Scholar 

  20. Blythe, S., Mao, X., Liao, X.: Stability of stochastic delay neural networks. J. Franklin Inst. 338(4), 481–495 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  21. Park, J.H., Kwon, O.M.: Analysis on global stability of stochastic neural networks of neutral type. Mod. Phys. Lett. B 22(32), 3159–3170 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  22. Zhou, L., Guangda, H.: Almost sure exponential stability of neutral stochastic delayed cellular neural networks. J. Control Theory Appl. 6(2), 195–200 (2008)

    Article  MathSciNet  Google Scholar 

  23. Huang, C., Yang, X., Cao, J.: Stability analysis of nicholson’s blowflies equation with two different delays. Math. Comput. Simul. 171, 201–206 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  24. Bradul, N., Shaikhet, L.: Stability of the positive point of equilibrium of nicholson’s blowflies equation with stochastic perturbations: numerical analysis. Discrete Dyn. Nature Soc. 2007, 1–26 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  25. Shaikhet, L.: Stability of equilibriums of stochastically perturbed delay differential neoclassical growth model. Discrete Contin. Dyn. Syst., Ser. B 22(4), 1565–1573 (2017)

    MathSciNet  MATH  Google Scholar 

  26. Berezansky, L., Idels, L., Troib, L.: Global dynamics of nicholson-type delay systems with applications. Nonlinear Anal. Real World Appl. 12(1), 436–445 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  27. Gikhman, I.I., Skorokhod, A.V.: The Theory of Stochastic Processes II. Springer, Berlin (2004)

    Book  MATH  Google Scholar 

  28. Kolmanovskii, V., Myshkis, A.: Introduction to the Theory and Applications of Functional Differential Equations, 463rd edn. Springer, Berlin (2013)

    MATH  Google Scholar 

  29. Gurney, W.S.C., Blythe, S.P., Nisbet, R.M.: Nicholson’s blowflies revisited. Nature 287(5777), 17–21 (1980)

    Article  Google Scholar 

  30. Shu, H., Wang, L., Wu, J.: Global dynamics of nicholson’s blowflies equation revisited, onset and termination of nonlinear oscillations. J. Differ. Equ. 255(9), 2565–2586 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  31. Berezansky, L., Braverman, E., Idels, L.: Nicholson’s blowflies differential equations revisited: main results and open problems. Appl. Math. Model. 34(6), 1405–1417 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  32. Wang, W., Wang, L., Chen, W.: Existence and exponential stability of positive almost periodic solution for nicholson-type delay systems. Nonlinear Anal. Real World Appl. 12(4), 1938–1949 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  33. Van Hien, L.: Global asymptotic behaviour of positive solutions to a non-autonomous nicholson’s blowflies model with delays. J. Biol. Dyn. 8(1), 135–144 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  34. Mao, X.: Stochastic Differential Equations and Applications. Elsevier, Amsterdam (2007)

    MATH  Google Scholar 

  35. Sohaly, M.A., Yassen, M.T., Elbaz, I.M.: Stochastic consistency and stochastic stability in mean square sense for cauchy advection problem. J. Differ. Equ. Appl. 24(1), 59–67 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  36. Villafuerte, L., Braumann, C.A., Cortés, J.C., Jódar, L.: Random differential operational calculus: theory and applications. Comput. Math. Appl. 59(1), 115–125 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  37. Qiuying, L.: Stability of sirs system with random perturbations. Physica A 388(18), 3677–3686 (2009)

    Article  MathSciNet  Google Scholar 

  38. Evans, L.C.: An Introduction to Stochastic Differential Equations, vol. 82. American Mathematical Society, Providence (2012)

    Google Scholar 

  39. Mao, X.: Exponential Stability of Stochastic Differential Equations. Marcel Dekker, New York (1994)

    MATH  Google Scholar 

  40. Mohammed, S.-E.A.: Stochastic Functional Differential Equations, Vol. 99. Pitman Advanced Publishing Program, Boston, London, Melbourne (1984)

  41. Biagini, F., Hu, Y., Øksendal, B., Zhang, T.: Stochastic Calculus for Fractional Brownian Motion and Applications. Springer, Berlin (2008)

    Book  MATH  Google Scholar 

  42. Mishura, I.S., Misura, J.S., Mishura, Y., Mishura, I.S., Misura, Û.S.: Stochastic Calculus for Fractional Brownian Motion and Related Processes, vol. 1929. Springer, Berlin (2008)

Download references

Acknowledgements

Not applicable.

Funding

This work was supported by the Mathematics Department—Mansoura University of Egypt.

Author information

Authors and Affiliations

  1. Mathematics Department, Faculty of Science, Mansoura University, Mansoura, Egypt

    H. El-Metwally, M. A. Sohaly & I. M. Elbaz

  2. Basic Sciences Department, Faculty of Engineering, The British University in Egypt, El Sherouk City, Cairo, Egypt

    I. M. Elbaz

Authors
  1. H. El-Metwally
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. A. Sohaly
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. I. M. Elbaz
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Not applicable.

Corresponding author

Correspondence to I. M. Elbaz.

Ethics declarations

Conflict of interest

The author declares that there is no conflict of interests regarding the publication of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

El-Metwally, H., Sohaly, M.A. & Elbaz, I.M. Mean-square stability of the zero equilibrium of the nonlinear delay differential equation: Nicholson’s blowflies application. Nonlinear Dyn 105, 1713–1722 (2021). https://doi.org/10.1007/s11071-021-06696-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-021-06696-6

Keywords

Search

Navigation