Global bifurcations and homoclinic chaos in nonlinear panel optomechanical resonators under combined thermal and radiation stresses

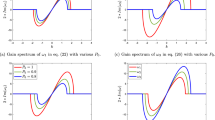
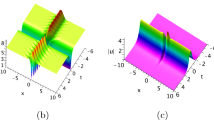
Abstract

In this paper, we investigate the nonlinear interaction and time-evolution of confined optical, thermal and mechanical modes in a three-dimensional optomechanical resonator. We model a high-finesse cavity with a thin thermo-elastic panel placed at the center of two highly reflective mirrors that is subjected to an incoming continuous-wave electromagnetic field. The latter is constructed by constraining the light-structure interaction to a first-order scattering phenomenon in the classical interpretation modeled as a spatio-temporal perturbation around a time-harmonic field. We employ a Galerkin-based separation of variables decomposition on the resultant fields and replace them with their nonlinear modal counterparts. The resulting dynamical system is thus governed by the combined effects of thermal and radiation stresses which yield a complex spatially dependent self-excited bifurcation structure where Hopf bifurcations give rise to periodic limit-cycle solutions. In regions where coexisting solutions are found, homoclinic connections ensue codimension-two Bogdanov–Takens and Double-Hopf bifurcations and that for a range of control parameters a global homoclinic Shilnikov bifurcation culminates with a distinct period-doubling route to chaos. We note that the current formulation demonstrates the essential contribution of coupled thermal and radiation stresses to the bifurcation structure of nonlinear light-structure interaction systems and may shed light to modal energy transfer mechanisms and scattering phenomena.

Appendices

Appendix A: non-dimensional constants

The non-dimensional constants of (12) are:

$$ \begin{aligned} & \tilde{\alpha }_{1} = \frac{{2a^{2} }}{{b^{2} }} ,\tilde{\alpha }_{2} = \frac{{a^{4} }}{{b^{4} }} , \tilde{\rho }_{1} = \frac{{\left| {\tilde{\omega }_{\text{in}} \epsilon_{0} \left( {\epsilon_{r} - 1} \right)} \right|\eta_{0} \hat{I}_{0} }}{{2\rho h\omega_{s}^{2} c_{0} }} \\ & \tilde{\beta }_{1} = \frac{{6\lambda_{\text{in}}^{2} }}{{h^{2} }} , \tilde{\beta }_{2} = \frac{{6\nu \lambda_{\text{in}}^{2} a^{2} }}{{b^{2} h^{2} }} ,\tilde{\beta }_{3} = \frac{{12\left( {1 - \nu } \right)\lambda_{\text{in}}^{2} a^{2} }}{{b^{2} h^{2} }} ,\tilde{\beta }_{4} = \frac{{6\lambda_{\text{in}}^{2} a^{4} }}{{b^{4} h^{2} }} , \\ & \tilde{\gamma }_{1} = \frac{{12\alpha_{T} a^{2} \left( {1 + \nu } \right)}}{{h^{2} }} ,\tilde{\gamma }_{2} = \frac{{a^{2} \tilde{\gamma }_{1} }}{{b^{2} }} , \tilde{\gamma }_{3} = \frac{{h\tilde{\gamma }_{1} }}{{\lambda_{\text{in}} }} ,\tilde{\gamma }_{4} = \frac{{a^{2} h\tilde{\gamma }_{1} }}{{b^{2} \lambda_{\text{in}} }} , \\ \end{aligned} $$

(66)

where $\hat{I}_{0} = \frac{{\left| {\hat{e}_{0}^{2} } \right|}}{{\eta_{0} }}$ is the laser intensity in W/m² where $\eta_{0} = \frac{1}{{\epsilon_{0} c_{0} }} = 376.73\left[ \varOmega \right]$ is free space impedance.

The non-dimensional constants related to the thermal field in (13) are:

$$ \begin{aligned} & \tilde{\eta }_{1} = \frac{k}{{\bar{C}_{p} a^{2} \omega_{s} }} ,\tilde{\eta }_{2} = \frac{k}{{\bar{C}_{p} b^{2} \omega_{s} }} , \tilde{\eta }_{3} = \frac{k}{{\bar{C}_{p} h^{2} \omega_{s} }} , \tilde{\rho }_{2} = \frac{{\text{Re} \left( {\tilde{\sigma }} \right)\eta_{0} \hat{I}_{0} }}{{2\bar{C}_{p} T_{0} \omega_{s} }} \\ & \tilde{\sigma }_{1} = \frac{{E\alpha_{T} \lambda_{\text{in}} h}}{{\bar{C}_{p} \left( {1 - \nu } \right)a^{2} }} ,\tilde{\sigma }_{2} = \frac{{a^{2} \tilde{\sigma }_{1} }}{{b^{2} }} ,\tilde{\sigma }_{3} = \frac{{\lambda_{\text{in}} \tilde{\sigma }_{1} }}{h} , \tilde{\sigma }_{4} = \frac{{\lambda_{\text{in}} a^{2} \tilde{\sigma }_{1} }}{{hb^{2} }} \\ \end{aligned} $$

(67)

where $\bar{C}_{p} = \rho c_{p} + \frac{{E\alpha_{T}^{2} T_{0} \left( {1 + \nu } \right)}}{{\left( {1 - \nu } \right)\left( {1 - 2\nu } \right)}}$ is an equivalent heat capacity per unit volume at constant stress.

The non-dimensional constants in (22) are:

$$ \chi_{1} = \frac{{c_{0}^{2} }}{{a^{2} \omega_{s}^{2} }} , \chi_{2} = \frac{{c_{0}^{2} }}{{b^{2} \omega_{s}^{2} }} , \chi_{3} = \frac{{c_{0}^{2} }}{{\lambda_{in}^{2} \omega_{s}^{2} }} $$

(68)

Appendix B: mode functions derivation

B.1 Panel mechanical modes

A Ritz method solution to (46)_a as expressed in (48) is based on building the solution as a linear combination of one-dimensional modes in (x) and (y) directions with Boundary conditions of S–S and F–F beams, respectively:

$$ \varphi_{l}^{\left( x \right)} = \sin \left( {k_{l}^{\left( x \right)} x} \right) $$

(69)

$$ \begin{aligned} & \varphi_{1}^{\left( y \right)} = 1 , \varphi_{2}^{\left( y \right)} = \sqrt 3 \left( {1 - \frac{2y}{{\alpha_{r} }}} \right) \\ & \varphi_{m \ge 3}^{\left( y \right)} = \sin \left( {k_{m}^{\left( y \right)} y} \right) + \sinh \left( {k_{m}^{\left( y \right)} y} \right) + B_{m} \left( {\cos \left( {k_{m}^{\left( y \right)} y} \right) + \cosh \left( {k_{m}^{\left( y \right)} y} \right)} \right) \\ \end{aligned} $$

(70)

where $k_{l}^{\left( x \right)} = l\pi$, $l = 1,2, \ldots ,M$ are the solutions to $\sin \left( k \right)\sinh \left( k \right) = 0$; $k_{1}^{\left( y \right)} = k_{2}^{\left( y \right)} = 0$ for $m = 1$ and $m = 2$, $k_{m}^{\left( y \right)} = 4.73,7.85,10.99, \ldots$ for $m = 3,4, \ldots ,M$ are the solutions to $\cos \left( k \right)\cosh \left( k \right) = 1$ and $B_{m} = \left( {\cos \left( {k_{m}^{\left( y \right)} y} \right) - \cosh \left( {k_{m}^{\left( y \right)} y} \right)} \right)/\left( {\sin \left( {k_{m}^{\left( y \right)} y} \right) + \sinh \left( {k_{m}^{\left( y \right)} y} \right)} \right)$.

The kinetic ($T$) and potential ($V$) energies of the panel [66] for a specific Eigen-mode are defined as:

$$ \begin{aligned} & T = \varOmega_{n}^{2} \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \varphi_{n}^{2} {\text{d}}x{\text{d}}y, \\ & V = \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \left( {\varphi_{n,xx}^{2} + \alpha_{r}^{4} \varphi_{n,yy}^{2} + 2\nu \alpha_{r}^{2} \varphi_{n,xx} \varphi_{n,yy} + 2\left( {1 - \nu } \right)\alpha_{r}^{2} \varphi_{n,xy}^{2} } \right){\text{d}}x{\text{d}}y . \\ \end{aligned} $$

(71)

From conservation of energy, V and T are equated to yield:

$$ \varOmega_{n}^{2} = \frac{V}{{\mathop \int \nolimits_{ - 1/2}^{1/2} \mathop \int \nolimits_{ - 1/2}^{1/2} \varphi_{n}^{2} {\text{d}}x{\text{d}}y}} $$

(72)

Substituting (48) into (72) and minimizing with respect to $a_{lm}^{n}$ yield a generalized eigenvalue problem with $\varOmega_{n}$ and $a_{lm}^{n}$ as eigenvalues and eigenvectors, respectively:

$$ \mathop \sum \limits_{m = 1}^{M} \mathop \sum \limits_{l = 1}^{M} \left( {C_{lmij} - \varOmega_{n}^{2} B_{lmij}^{{\left( {0,0,0,0} \right)}} } \right)a_{lm}^{n} = 0 $$

(73)

where

$$ B_{lmij}^{{\left( {p,q,s,r} \right)}} = \mathop \int \limits_{ - 1/2}^{1/2} \frac{{{\text{d}}^{p} }}{{{\text{d}}x^{p} }}\left( {\varphi_{l}^{\left( x \right)} } \right)\frac{{{\text{d}}^{q} }}{{{\text{d}}x^{q} }}\left( {\varphi_{i}^{\left( x \right)} } \right){\text{d}}x\mathop \int \limits_{ - 1/2}^{1/2} \frac{{{\text{d}}^{s} }}{{{\text{d}}y^{s} }}\left( {\varphi_{m}^{\left( y \right)} } \right)\frac{{{\text{d}}^{r} }}{{{\text{d}}y^{r} }}\left( {\varphi_{j}^{\left( y \right)} } \right){\text{d}}y, $$

(74)

$$ C_{lmij} = B_{lmij}^{{\left( {2,2,0,0} \right)}} + \alpha_{r}^{2} B_{lmij}^{{\left( {0,0,2,2} \right)}} + \nu \alpha_{r}^{2} \left( {B_{lmij}^{{\left( {2,0,0,2} \right)}} + B_{lmij}^{{\left( {0,2,2,0} \right)}} } \right) + 2\left( {1 - \nu } \right)\alpha_{r}^{2} B_{lmij}^{{\left( {1,1,1,1} \right)}} , $$

(75)

and $l,m,i,j = 1,2, \ldots M$. Some of the mode shapes of an S–F–S–F panel are showed in Fig. 23.

Fig. 23

1st three mechanical modes of an S–F–S–F panel (n = 1 (1, 1), n = 2 (1, 2) and n = 3 (1, 3))

B.2 Panel thermal modes

The constants for the thermal field modes (49) are:

$$ \begin{aligned} & \hat{\lambda }_{lmn} = \sqrt {\left( {\pi^{2} \left( {l^{2} \tilde{\eta }_{1} + m^{2} \tilde{\eta }_{2} } \right) + i\varOmega_{n} } \right)/\tilde{\eta }_{3} } \\ & A_{lmn} = \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \left( {\tilde{\sigma }_{1} \varphi_{nxx} + \tilde{\sigma }_{2} \varphi_{nyy} } \right)\sin \left( {l\pi \left( {x + 0.5} \right)} \right)\sin \left( {m\pi \left( {y + 0.5} \right)} \right){\text{d}}x{\text{d}}y \\ \end{aligned} $$

(76)

where some of the thermal modes shapes of an S–F–S–F panel are shown in Fig. 24.

Fig. 24

Top: 1st three thermal modes (arbitrary $\zeta$) of an S–F–S–F panel. Bottom: $\psi \left( {x,y = 0,\zeta } \right)$ for $a = b = 40\;\upmu{\text{m}}$ and $h = 30\;\upmu{\text{m}}$

B.3 EM field modes

Omitting the Gaussian profile $\varGamma \left( {x,y} \right)$ in (36), the 1D electric ($\mathcal{E}_{lm}$) and magnetic (${\mathcal{H}}_{lm}$) fields modes of the multilayered cavity in Fig. 25 are [67]:

$$ \begin{aligned} \left[ {\begin{array}{*{20}c} {\mathcal{E}_{lm} \left( {z,w} \right)} \\ {{\mathcal{H}}_{lm} \left( {z,w} \right)} \\ \end{array} } \right] & = \left[ {\begin{array}{*{20}c} 1 \\ {\frac{{n_{l} }}{{\eta_{0} }}} \\ \end{array} } \right]E_{lm}^{ + } \left( {w\left( {x,y,t} \right)} \right)e^{{ - ik_{m} n_{l} \left( {z - z_{l} } \right)}} \\ & \quad + \left[ {\begin{array}{*{20}c} 1 \\ { - \frac{{n_{l} }}{{\eta_{0} }}} \\ \end{array} } \right]E_{lm}^{ - } \left( {w\left( {x,y,t} \right)} \right)e^{{ + ik_{m} n_{l} \left( {z - z_{l} } \right)}} , \\ \end{aligned} $$

(77)

where $m = 0, \pm 1, \pm 2, \ldots$ is mode number, $l = 0,..,4$ is layer number and $z_{l} = a_{l} + b_{l} w$ is the z location at the interface, as depicted in Fig. 25 ($z_{0} = 0$, $z_{1} = \tilde{V}_{1} - \tilde{h}/2 + w$, $z_{2} = \tilde{V}_{1} + \tilde{h}/2 + w$ and $z_{3} = \tilde{V}_{2}$). Note that the EM field modes have only outgoing fields, thus $E_{0}^{ + } = E_{4}^{ - } = 0$. The remaining $E_{lm}^{ \pm }$ terms for $l = 0,..,3$ ($E_{4m}^{ + } = 1$) are:

Fig. 25

2D sketch of a lossy cavity with panel in the center

$$ \begin{aligned} & \left[ {\begin{array}{*{20}c} 0 \\ {E_{0m}^{ - } } \\ \end{array} } \right] = M_{1} M_{2} M_{3} M_{4} \left[ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right] \\ & \left[ {\begin{array}{*{20}c} {E_{1m}^{ + } } \\ {E_{1m}^{ - } } \\ \end{array} } \right] = M_{2} M_{3} M_{4} \left[ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right] \\ & \left[ {\begin{array}{*{20}c} {E_{2m}^{ + } } \\ {E_{2m}^{ - } } \\ \end{array} } \right] = M_{3} M_{4} \left[ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right] \\ & \left[ {\begin{array}{*{20}c} {E_{3m}^{ + } } \\ {E_{3m}^{ - } } \\ \end{array} } \right] = M_{4} \left[ {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \right] \\ \end{aligned} $$

(78)

where

$$ \begin{aligned} & M_{1} = \left[ {\begin{array}{*{20}c} {\left( {1 - \frac{{ik_{m} \nu_{1} }}{2}} \right)e^{{ik_{m} \left( {\tilde{V}_{1} - \frac{{\tilde{h}}}{2} - w} \right)}} } & {\frac{{ik_{m} \nu_{1} }}{2}e^{{ - ik_{m} \left( {\tilde{V}_{1} - \frac{{\tilde{h}}}{2} - w} \right)}} } \\ { - \frac{{ik_{m} \nu_{1} }}{2}e^{{ik_{m} \left( {\tilde{V}_{1} - \frac{{\tilde{h}}}{2} - w} \right)}} } & {\left( {1 + \frac{{ik_{m} \nu_{1} }}{2}} \right)e^{{ - ik_{m} \left( {\tilde{V}_{1} - \frac{{\tilde{h}}}{2} - w} \right)}} } \\ \end{array} } \right], \\ & M_{2} = \left[ {\begin{array}{*{20}c} {e^{{ik_{m} n_{2} \tilde{h}}} } & {\rho_{2} e^{{ - ik_{m} n_{2} \tilde{h}}} } \\ {\rho_{2} e^{{ik_{m} n_{2} \tilde{h}}} } & {e^{{ - ik_{m} n_{2} \tilde{h}}} } \\ \end{array} } \right], \\ \end{aligned} $$

$$ \begin{aligned} & M_{3} = \left[ {\begin{array}{*{20}c} {e^{{ik_{m} \left( {\tilde{V}_{2} - \tilde{V}_{1} - \frac{{\tilde{h}}}{2} - w} \right)}} } & {\rho_{3} e^{{ - ik_{m} \left( {\tilde{V}_{2} - \tilde{V}_{1} - \frac{h}{2} - w} \right)}} } \\ {\rho_{3} e^{{ik_{m} \left( {\tilde{V}_{2} - \tilde{V}_{1} - \frac{h}{2} - w} \right)}} } & {e^{{ - ik_{m} \left( {\tilde{V}_{2} - \tilde{V}_{1} - \frac{h}{2} - w} \right)}} } \\ \end{array} } \right], \\ & M_{4} = \left[ {\begin{array}{*{20}c} {1 - \frac{{ik_{m} \nu_{4} }}{2}} & {\frac{{ik_{m} \nu_{4} }}{2}} \\ {\frac{{ik_{m} \nu_{4} }}{2}} & {1 + \frac{{ik_{m} \nu_{4} }}{2}} \\ \end{array} } \right]. \\ \end{aligned} $$

The cavity modes frequencies are found by equating the first line in (78)_a to zero ($\left\{ {M_{1} M_{2} M_{3} M_{4} } \right\}_{11} = 0$), yielding the transcendental equation of (38).

Substituting ${\mathbb{E}}_{m}$ into (32) and assuming $\omega_{s} \ll \tilde{\omega }_{\text{in}}$ yields the expression for the spatio-temporal current density:

$$ {\mathbb{J}}_{m} = {\mathcal{J}}_{m} e_{m} \left( t \right). $$

(79)

where ${\mathcal{J}}_{m} = \frac{{\left( {\epsilon_{r} - 1} \right)}}{{i\left| {\left( {\epsilon_{r} - 1} \right)} \right|}}\mathcal{E}_{m}$.

To find the spatio-temporal magnetic field ${\mathbb{H}}_{m} ,{\mathbb{E}}_{m}$ is substituted into (33), the result is multiplied by ${\hat{\mathcal{E}}}_{m}^{*}$, integrated over the entire volume and rearranged to yield (assuming $\omega_{s} \ll \tilde{\omega }_{\text{in}}$) the reduced parameter $h_{m} \left( t \right)$:

$$ h_{m} \left( t \right) = \frac{2\pi }{i}\frac{{\iiint {\epsilon_{r} \mathcal{E}_{m} {\hat{\mathcal{E}}}_{m}^{*} {\text{d}}x{\text{d}}y{\text{d}}z}}}{{\iiint {\left( {\partial_{z} {\mathcal{H}}_{m} } \right){\hat{\mathcal{E}}}_{m}^{*} {\text{d}}x{\text{d}}y{\text{d}}z}}}e_{m} \left( t \right). $$

(80)

Thus:

$$ {\mathbb{H}}_{m} \left( t \right) = {\mathcal{H}}_{m} h_{m} \left( t \right) = {\hat{\mathcal{H}}}_{m} e_{m} \left( t \right) , $$

(81)

where ${\hat{\mathcal{H}}}_{m} = \frac{2\pi }{i}{\mathcal{H}}_{m} \frac{{\iiint {\epsilon_{r} \mathcal{E}_{m} {\hat{\mathcal{E}}}_{m}^{*} {\text{d}}x{\text{d}}y{\text{d}}z}}}{{\iiint {\left( {\partial_{z} {\mathcal{H}}_{m} } \right){\hat{\mathcal{E}}}_{m}^{*} {\text{d}}x{\text{d}}y{\text{d}}z}}}$.

Appendix C: reduced model constants and functions

C.1 Parameters related to the EM field

The optical functions in (44) are:

$$ {\mathcal{K}}_{m}^{\left( p \right)} \left( {q_{1} , \ldots ,q_{N} } \right) = \frac{{{\hat{\mathcal{K}}}_{m}^{\left( p \right)} \left( {q_{1} , \ldots ,q_{N} } \right)}}{{{\mathcal{K}}_{m}^{\left( 5 \right)} \left( {q_{1} , \ldots ,q_{N} } \right)}} $$

(82)

where $p = 1, \ldots ,5$ and ${\hat{\mathcal{K}}}_{m}^{\left( i \right)} \left( {q_{1} , \ldots ,q_{N} } \right)$ are expressed through the following integrals:

$$ \begin{aligned} & {\hat{\mathcal{K}}}_{mi}^{\left( 1 \right)} \left( {q_{1, \ldots ,N} } \right) = \iiint {\epsilon_{r} \left( z \right)\left( {\chi_{1} \left( {\varphi_{ix} \mathcal{E}_{m,wx} + \varphi_{ixx} \mathcal{E}_{m,w} } \right) + \chi_{2} \left( {\varphi_{iy} \mathcal{E}_{m,wy} + \varphi_{iyy} \mathcal{E}_{m,w} } \right)} \right){\hat{\mathcal{E}}}_{m}^{*} } \\ & {\hat{\mathcal{K}}}_{mij}^{\left( 2 \right)} \left( {q_{1, \ldots ,N} } \right) = \iiint {\epsilon_{r} \left( z \right)\left( {\chi_{1} \varphi_{ix} \varphi_{jx} + \chi_{2} \varphi_{iy} \varphi_{jy} } \right)\mathcal{E}_{m,ww} {\hat{\mathcal{E}}}_{m}^{*} {\text{d}}^{3} r} \\ & {\hat{\mathcal{K}}}_{mi}^{\left( 3 \right)} \left( {q_{1, \ldots ,N} } \right) = \iiint {\epsilon_{r} \left( z \right)\varphi_{i} \mathcal{E}_{m,w} {\hat{\mathcal{E}}}_{m}^{*} {\text{d}}^{3} r} \\ & {\hat{\mathcal{K}}}_{m}^{\left( 4 \right)} \left( {q_{1, \ldots ,N} } \right) = \iiint {\epsilon_{r} \left( z \right)\left( {\frac{1}{{n_{z}^{2} \omega_{in}^{2} }}\left( {\chi_{1} \mathcal{E}_{in,xx} + \chi_{2} \mathcal{E}_{in,yy} + \chi_{3} \mathcal{E}_{in,zz} } \right) + \mathcal{E}_{in} } \right){\hat{\mathcal{E}}}_{m}^{*} {\text{d}}^{3} r} \\ & {\hat{\mathcal{K}}}_{m}^{\left( 5 \right)} \left( {q_{1, \ldots ,N} } \right) = \iiint {\epsilon_{r} \left( z \right)\delta \omega_{m} \left( {1 + \frac{{\delta \omega_{m} }}{{2\omega_{in} }}} \right)\mathcal{E}_{m} {\hat{\mathcal{E}}}_{m}^{*} {\text{d}}^{3} r} \\ \end{aligned} $$

(83)

Since $\mathcal{E}_{m}$ are functions of the transverse coordinates $\left( {x,y} \right)$ and of the spatio-temporal displacement $w\left( {x,y,t} \right) = \mathop \sum \limits_{i} \varphi_{i} \left( {x,y} \right)q_{i} \left( t \right)$, an analytic integral on ${\hat{\mathcal{K}}}_{m}^{\left( p \right)}$ is not applicable. However, we can approximate it by using a two-dimensional trapezoidal rule in the $\left( {x, y} \right)$ axes, then solve the $z$ axis separately:

$$ \begin{aligned} {\hat{\mathcal{K}}}_{m}^{\left( i \right)} \left( {q_{1, \ldots ,N} } \right) & = \mathop \int \limits_{{0^{ - } }}^{{V_{2}^{ + } }} \left( {\mathop \int \limits_{ - 0.5}^{0.5} \mathop \int \limits_{ - 0.5}^{0.5} \epsilon_{r} \left( z \right){\text{d}}K_{m}^{\left( p \right)} \left( {x,y,z,\mathop \sum \limits_{k = 1}^{N} \varphi_{n} \left( {x,y} \right)q_{n} \left( t \right)} \right){\text{d}}x{\text{d}}y} \right){\text{d}}z \\ & = \frac{1}{4LK}\mathop \sum \limits_{l = - L}^{L} \mathop \sum \limits_{k = - K}^{K} \alpha_{lk} \mathop \int \limits_{{0^{ - } }}^{{V_{2}^{ + } }} \int_{r} \left( z \right){\text{d}}K_{m}^{\left( p \right)} \left( {x_{l} ,y_{l} ,z,\mathop \sum \limits_{k = 1}^{N} \varphi_{n} \left( {x_{l} ,y_{k} } \right)q_{n} \left( t \right)} \right){\text{d}}z \\ \end{aligned} $$

(84)

where $\epsilon_{r} {\text{d}}K_{m}^{\left( p \right)}$ is any one of the integrands in (83), $\left( {x_{l} ,y_{k} } \right)$ are the transverse integration points, $\alpha_{lk}$ are weighting parameters ($\alpha_{ \pm L, \pm K} = 1$ or $\alpha_{l, \pm K} = \alpha_{ \pm L,k} = 2$ at the edges and $\alpha_{lk} = 4$ at the inner points) and (2L + 1,2 K + 1) are the number of integration points in each axis. Note that the integration in z must be analytical or at least solved by a fast-converging numerical scheme in order for it to be practical to use in each iteration of a FDTD solver.

A simplification of the above integrals is done by assuming the integrand ${\text{d}}K_{m}^{\left( p \right)}$ can be separated to a multiplication of functions with different variables, i.e. ${\text{d}}K_{m}^{\left( p \right)} = {\text{d}}K_{xy} \left( {x,y} \right){\text{d}}K_{z} \left( {z,w\left( {x,y,t} \right)} \right)$. The integrand ${\text{d}}K_{xy}$ is assumed to be integrable and is solved outside the FDTD scheme and ${\text{d}}K_{z}$ is assumed to have weak dependence on $w\left( {x,y,t} \right)$ and is replaced with ${\text{d}}K_{z} \left( {z,w\left( {x_{0} ,y_{0} ,t} \right)} \right)$. Thus, ${\hat{\mathcal{K}}}_{m}^{\left( p \right)}$ are simplified to:

$$ {\hat{\mathcal{K}}}_{m}^{\left( p \right)} \left( {q_{1, \ldots ,N} } \right) = \mathop \int \limits_{ - 0.5}^{0.5} \mathop \int \limits_{ - 0.5}^{0.5} {\text{d}}K_{xy} \left( {x,y} \right){\text{d}}x{\text{d}}y\mathop \int \limits_{{0^{ - } }}^{{V_{2}^{ + } }} \left( {\epsilon_{r} \left( z \right){\text{d}}K_{z} \left( {z,\mathop \sum \limits_{k = 1}^{N} \varphi_{n} \left( {x_{0} ,y_{0} } \right)q_{n} \left( t \right)} \right)} \right){\text{d}}z. $$

(85)

C.2 Parameters related to the elastic field

The elastic field (50) constants are:

$$ \varOmega_{n}^{2} = {\iint }\left( {\varphi_{n,xxxx} + \tilde{\alpha }_{1} \varphi_{n,xxyy} + \tilde{\alpha }_{2} \varphi_{n,yyyy} } \right)\varphi_{n} {\text{d}}x{\text{d}}y , $$

(86)

$$ \begin{aligned} \beta_{nijk} & = - {\iint }\left( {\left( {\tilde{\beta }_{1} \varphi_{i,x} \varphi_{j,x} + \tilde{\beta }_{2} \varphi_{i,y} \varphi_{j,y} } \right) + \tilde{\beta }_{3} \varphi_{i,x} \varphi_{j,y} \varphi_{k,xy} } \right. \\ & \quad \left. { + \;\left( {\tilde{\beta }_{2} \varphi_{i,x} \varphi_{j,x} + \tilde{\beta }_{4} \varphi_{i,y} \varphi_{j,y} } \right)} \right)\varphi_{n} {\text{d}}x{\text{d}}y, \\ \end{aligned} $$

(87)

$$ \begin{aligned} & \gamma_{n}^{\left( 1 \right)} = \iiint {\zeta \left( {\tilde{\gamma }_{3} \psi_{n,xx} + \tilde{\gamma }_{4} \psi_{n,yy} } \right)\varphi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta ,} \\ & \gamma_{nij}^{\left( 2 \right)} = \iiint {\left( {\tilde{\gamma }_{1} \varphi_{i,xx} + \tilde{\gamma }_{2} \varphi_{i,yy} } \right)\psi_{j} \varphi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta .} \\ \end{aligned} $$

(88)

The spatially averaged variable radiation pressure on the panel is:

$$ {\mathcal{F}}_{nkl} \left( {q_{1, \ldots ,N} } \right) = \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \varphi_{n} \mathop \int \limits_{{\tilde{V}_{1} - \frac{{\tilde{h}}}{2} + w}}^{{\tilde{V}_{1} + \frac{{\tilde{h}}}{2} + w}} {\mathcal{J}}_{k} {\hat{\mathcal{H}}}_{l}^{*} {\text{d}}x{\text{d}}y{\text{d}}z , $$

(89)

where ${\mathcal{J}}_{m} \left( {q_{1, \ldots ,N} } \right)$ and ${\hat{\mathcal{H}}}_{m} \left( {q_{1, \ldots ,N} } \right)$ are derived in “Appendix B”.

C.3 Parameters related to the thermal field

The thermal field (51) constants are:

$$ \eta_{n} = \iiint {\left( {\tilde{\eta }_{1} \psi_{n,xx} + \tilde{\eta }_{2} \psi_{n,yy} + \tilde{\eta }_{3} \psi_{n,\zeta \zeta } } \right)\psi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta } $$

(90)

$$ \begin{aligned} & \sigma_{n}^{\left( 1 \right)} = \iiint {\zeta \left( {\tilde{\sigma }_{1} \varphi_{n,xx} + \tilde{\sigma }_{2} \varphi_{n,yy} } \right)\psi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta } \\ & \sigma_{nij}^{\left( 2 \right)} = \iiint {\left( {\tilde{\sigma }_{3} \varphi_{i,x} \varphi_{j,x} + \tilde{\sigma }_{4} \varphi_{i,y} \varphi_{j,y} } \right)\psi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta } \\ & \sigma_{nij}^{\left( 3 \right)} = \iiint {\zeta \psi_{i} \left( {\tilde{\sigma }_{1} \varphi_{j,xx} + \tilde{\sigma }_{2} \varphi_{j,yy} } \right)\psi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta } \\ & \sigma_{nijk}^{\left( 2 \right)} = - \iiint {\psi_{i} \left( {\tilde{\sigma }_{3} \varphi_{j,x} \varphi_{k,x} + \tilde{\sigma }_{4} \varphi_{j,y} \varphi_{k,y} } \right)\psi_{n} {\text{d}}x{\text{d}}y{\text{d}}\zeta } \\ \end{aligned} $$

(91)

The spatially averaged variable heat function is:

$$ {\mathcal{Q}}_{nkl} \left( {q_{1, \ldots ,N} } \right) = \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \mathop \int \limits_{ - 1/2}^{1/2} \psi_{n}^{*} \mathcal{E}_{k} \mathcal{E}_{l}^{*} {\text{d}}x{\text{d}}y{\text{d}}\zeta . $$

(92)

C.4 Parameters related to the limiting adiabatic system

The linear and nonlinear damping coefficients in (58) are:

$$ \begin{aligned} & \delta_{01}^{\left( T \right)} = - \frac{{\gamma_{1} \sigma_{1} }}{\eta } ,\delta_{11}^{\left( T \right)} = - \frac{{\gamma_{2} \sigma_{1} + \gamma_{1} \sigma_{2} }}{\eta } , \delta_{21}^{\left( T \right)} = - \frac{{\gamma_{2} \sigma_{2} }}{\eta } \\ & \delta_{02}^{\left( T \right)} = - \frac{{\gamma_{1} \sigma_{1} \sigma_{3} }}{{\eta^{2} }} , \delta_{12}^{\left( T \right)} = - \frac{{\gamma_{1} \sigma_{1} \sigma_{4} }}{{\eta^{2} }} , \delta_{03}^{\left( T \right)} = - \frac{{\gamma_{1} \sigma_{1} \sigma_{3}^{2} }}{{\eta^{3} }} \\ \end{aligned} $$

(93)

$$ \delta_{ij}^{\left( W \right)} = - \left. {\frac{{\partial^{i + j} \Delta {\mathcal{W}}}}{{\partial x_{1}^{i} \partial x_{2}^{j} }}} \right|_{{\left( {0,0} \right)}} $$

(94)

where $x_{1} = q$ and $x_{2} = \dot{q}$.

Appendix D: stability analysis definitions

The coefficients of the characteristic polynomial related to (61) are:

$$ \begin{aligned} c_{0} & = \left( {\eta \rho_{1} {\mathcal{F}}_{e} + \tilde{\gamma }_{e} \rho_{2} {\mathcal{Q}}_{e} } \right)\left( {\left( {G_{q} r_{e} + V_{q} s_{e} } \right)\kappa_{e} + 2\left( {G_{q} s_{e} - V_{q} r_{e} } \right)\left( {\Delta \omega_{0} + \Delta_{e} } \right)} \right) \\ & \quad + \;\left( {\rho_{2} \tilde{\gamma }_{e} \left( {r_{e}^{2} + s_{e}^{2} } \right){\mathcal{Q}}_{e} - \omega_{q}^{2} \eta } \right)\omega_{r}^{2} \\ c_{1} & = \left( {\omega_{q}^{2} + \tilde{\sigma }_{e} \tilde{\gamma }_{e} - \delta \eta } \right)\omega_{r}^{2} + 2\left( {G_{q} r_{e} + V_{q} s_{e} } \right)\left( {\left( {\eta - \frac{{\kappa_{e} }}{2}} \right)\rho_{1} {\mathcal{F}}_{e} + \tilde{\gamma }_{e} \rho_{2} {\mathcal{Q}}_{e} } \right) \\ & \quad + \;\left( {\rho_{2} \tilde{\gamma }_{e} \left( {r_{e}^{2} + s_{e}^{2} } \right){\mathcal{Q}}_{e} - \omega_{q}^{2} \eta } \right)\kappa_{e} - 2\left( {\Delta \omega_{0} + \Delta_{e} } \right)\rho_{1} {\mathcal{F}}_{e} \left( {G_{q} s_{e} - V_{q} r_{e} } \right) \\ c_{2} & = \left( {\delta - \eta } \right)\omega_{r}^{2} + \left( {\omega_{q}^{2} + \tilde{\sigma }_{e} \tilde{\gamma }_{e} - \delta \eta } \right)\kappa_{e} + \rho_{2} \tilde{\gamma }_{e} \left( {r_{e}^{2} + s_{e}^{2} } \right){\mathcal{Q}}_{e} \\ & \quad - \;\eta \omega_{q}^{2} - 2\rho_{1} {\mathcal{F}}_{e} \left( {G_{q} r_{e} + V_{q} s_{e} } \right) \\ c_{3} & = \omega_{r}^{2} + \left( {\delta - \eta } \right)\kappa_{e} + \omega_{q}^{2} + \tilde{\sigma }_{e} \tilde{\gamma }_{e} - \delta \eta \\ c_{4} & = \kappa_{e} + \delta - \eta \\ \end{aligned} $$

(95)

For an fifth-order dynamical system (${\dot{\mathbf{x}}} = {\mathbf{F}}\left( {\mathbf{x}} \right) ;{\mathbf{x}} \in {\mathbb{R}}^{5}$), the resultant (${\mathcal{R}}$) of the characteristic polynomial $\mathop \sum \nolimits_{n = 0}^{5} c_{n} \lambda^{n}$ is defined by (Sylvester form) [51]:

$$ {\mathcal{R}} = \left| {\begin{array}{*{20}c} {c_{0} } & {c_{2} } & {c_{4} } & 0 \\ 0 & {c_{0} } & {c_{2} } & {c_{4} } \\ {c_{1} } & {c_{3} } & 1 & 0 \\ 0 & {c_{1} } & {c_{3} } & 1 \\ \end{array} } \right| $$

(96)

In addition, the sub-resultants ${\mathcal{S}}_{i}$ (i = 1, 2) for a fifth-order system are:

$$ {\mathcal{S}}_{1} = \left| {\begin{array}{*{20}c} {c_{2} } & {c_{4} } \\ {c_{3} } & 1 \\ \end{array} } \right| , {\mathcal{S}}_{2} = \left| {\begin{array}{*{20}c} {c_{0} } & {c_{4} } \\ {c_{1} } & 1 \\ \end{array} } \right| $$

(97)

Thus, the sub-resultant criterion is defined by:

$$ \fancyscript{h} = {\mathcal{S}}_{1} \cdot {\mathcal{S}}_{2} $$

(98)

According to theory, codimension-one bifurcation points are found for ${\mathcal{R}} = 0$. Higher-order bifurcations are determined by the sign of $\fancyscript{h}$, as explained in the main text.