Geometrically exact equation of motion for large-amplitude oscillation of cantilevered pipe conveying fluid

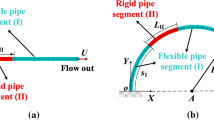
Abstract

Theoretical modeling and dynamic analysis of cantilevered pipes conveying fluid are presented with particular attention to geometric nonlinearities in the case of large-amplitude oscillations. To derive a new version of nonlinear equation of motion, the rotation angle of the centerline of the pipe is utilized as the generalized coordinate to describe the motion of the pipe. By using variational operations on energies of the pipe system with respect to either lateral displacement or rotation angle of the centerline, two kinds of new equations of motion of the cantilever are derived first based on Hamilton’s principle. It is interesting that these two governing equations are geometrically exact, different-looking but essentially equivalent. With the aid of Taylor expansion, one of the newly developed equations of motion can be degenerated into previous Taylor-expansion-based governing equation expressed in the form of lateral displacement. Then, the proposed new equation of motion is linearized to determine the stability of the cantilevered pipe system. Finally, nonlinear analyses are conducted based on the current geometrically exact model. It is shown that the cantilevered pipe would undergo limit-cycle oscillation after flutter instability is induced by the internal fluid flow. As expected, quantitative agreement between geometrically exact model and Taylor-expansion-based model can be achieved when the oscillation amplitude of the pipe is relatively small. However, remarkable difference between the results of oscillation amplitudes predicted using these two models would occur for large-amplitude oscillations. The main reason is that in the Taylor-expansion-based model, high-order geometric nonlinearities have been neglected when applying the Taylor expansion, thus yielding some deviation when large-amplitude oscillations are generated. Consequently, the proposed new geometrically exact equation of motion is more reliable for large-amplitude oscillations of cantilevered pipes conveying fluid.

Appendix

As can be seen from Eqs. (7)–(9) and (11), the variations of various terms can be expressed by three possible virtual displacements, including $\delta u$, $\delta w$ and $\delta \theta$. In order to derive the governing equation and boundary conditions of this dynamical system, expressing these variations in terms of one single virtual displacement is necessary. In this part, the various variations will be, respectively, expressed by $\delta w$ and $\delta \theta$ for the purpose of deriving the governing equations in Sects. 2.1 and 2.2.

The relationships of $\delta u$, $\delta w$ and $\delta \theta$ can be obtained via Eqs. (2) and (3). The variational operations on Eqs. (2) and (3) lead to

$$\begin{aligned} \delta \theta= & {} \frac{\delta {w}'}{\cos \theta },\,\,\delta {u}'=-\tan \theta \delta {w}',\nonumber \\ \delta u= & {} -\tan \theta \delta w+\int _0^{x_0 } {\left( {\tan \theta } \right) ^{\prime }\delta w\mathrm{d}x_0 } \end{aligned}$$

(48a)

$$\begin{aligned} \delta {u}'= & {} -\sin \theta \delta \theta ,\,\,\delta {w}'=\cos \theta \delta \theta ,\nonumber \\ \delta u= & {} -\int _0^{x_0 } {\sin \theta \delta \theta \mathrm{d}x_0 } ,\,\,\delta w=\int _0^{x_0 } {\cos \theta \delta \theta \mathrm{d}x_0 }\nonumber \\ \end{aligned}$$

(48b)

To maintain consistency with Sect. 2.1, we will firstly utilize $\delta w$ as the virtual displacement for derivation purpose. The substitution of Eq. (48a) into Eq. (7) generates another form of the first term in Eq. (7) as

$$\begin{aligned}&-\int _{t_1 }^{t_2 } \int _0^L \left[ \left( {m+M} \right) \ddot{u}+M\dot{U}\left( {{u}'+1} \right) \right. \nonumber \\&\qquad +\left. 2MU{\dot{u}}' \right] \delta u\mathrm{d}x_0 \mathrm{d}t \nonumber \\&\quad =\int _{t_1 }^{t_2 } \int _0^L \left[ \left( {m+M} \right) \ddot{u}+M\dot{U}\left( {{u}'+1} \right) \right. \nonumber \\&\qquad +\left. 2MU{\dot{u}}' \right] \tan \theta \delta w\mathrm{d}x_0 \mathrm{d}t \nonumber \\&\qquad -\int _{t_1 }^{t_2 } \int _0^L \left( \int _{x_0 }^L \left[ \left( {m+M} \right) \ddot{u}+M\dot{U}\left( {{u}'+1} \right) \right. \right. \nonumber \\&\qquad +\left. \left. 2MU{\dot{u}}' \right] \mathrm{d}x_0 \right) \left( {\tan \theta } \right) ^{\prime }\delta w\mathrm{d}x_0 \mathrm{d}t \end{aligned}$$

(49)

with the aid of the following important equation given by Semler et al. [29]

$$\begin{aligned}&\int _0^L {g\left( {x_0 } \right) \left( {\int _0^{x_0 } {f\left( {x_0 } \right) \delta w\mathrm{d}x_0 } } \right) \mathrm{d}x_0 } \nonumber \\&\quad =\int _0^L {\left( {\int _{x_0 }^L {g\left( {x_0 } \right) \mathrm{d}x_0 } } \right) f\left( {x_0 } \right) \delta w\mathrm{d}x_0 } \end{aligned}$$

(50)

By using Eq. (48a) and Eq. (8), the variation of the strain energy can be rewritten as

$$\begin{aligned}&\delta \int _{t_1 }^{t_2 } {\mathcal {V}\mathrm{d}t} =-{\textit{EI}}\int _{t_1 }^{t_2 } {\left. {\frac{{\theta }''}{\cos \theta }\delta w} \right| _0^L \mathrm{d}t} \nonumber \\&\quad +{\textit{EI}}\int _{t_1 }^{t_2 } {\int _0^L {\left( {\frac{{\theta }''}{\cos \theta }} \right) ^{\prime }\delta w} \mathrm{d}x_0 \mathrm{d}t} \end{aligned}$$

(51)

The similar operation on Eq. (9) leads to

$$\begin{aligned}&\delta \int _{t_1 }^{t_2 } {\mathcal {G}\mathrm{d}t} =\left( {m+M} \right) g\int _{t_1 }^{t_2 } {\int _0^L {\tan \theta \delta w\mathrm{d}x_0 } \mathrm{d}t}\nonumber \\&\quad -\int _{t_1 }^{t_2 } {\int _0^L {\left( {L-x_0 } \right) \left( {m+M} \right) g\left( {\tan \theta } \right) ^{\prime }\delta w\mathrm{d}x_0 } \mathrm{d}t}\nonumber \\ \end{aligned}$$

(52)

The second term of the right-hand side of Eq. (11) can be further written as

$$\begin{aligned} \begin{aligned} B&=MU^{2}\int _{t_1 }^{t_2 } {\left( {{x}'_L \delta x_L +{z}'_L \delta z_L } \right) \mathrm{d}t} \\&=MU^{2}\int _{t_1 }^{t_2 } \left[ \cos \theta _L \delta \left( {\int _0^L {\cos \theta \mathrm{d}x_0 } } \right) \right. \\&\quad +\left. \sin \theta _L \delta w_L \right] \mathrm{d}t \\&=MU^{2}\int _{t_1 }^{t_2 } {\int _0^L {\cos \theta _L \left( {\tan \theta } \right) ^{\prime }\delta w\mathrm{d}x_0 } \mathrm{d}t} \end{aligned} \end{aligned}$$

(53)

The variations expressed by the virtual displacement $\delta \theta$ are called for the derivation in Sect. 2.2. With the aid of Eq. (50), the substitution of Eq. (48b) into Eq. (7) yields another form of the first term in Eq. (7), i.e.,

$$\begin{aligned}&-\int _{t_1 }^{t_2 } \int _0^L \left[ \left( {m+M} \right) \ddot{u}+M\dot{U}\left( {{u}'+1} \right) \right. \nonumber \\&\qquad +\left. 2MU{\dot{u}}' \right] \delta u\mathrm{d}x_0 \mathrm{d}t \nonumber \\&\quad =\int _{t_1 }^{t_2 } \int _0^L \left( \int _{x_0 }^L \left[ \left( {m+M} \right) \ddot{u}+M\dot{U}\left( {{u}'+1} \right) \right. \right. \nonumber \\&\qquad +\left. \left. 2MU{\dot{u}}' \right] \mathrm{d}x_0 \right) \sin \theta \delta \theta \mathrm{d}x_0 \mathrm{d}t \end{aligned}$$

(54)

The second term in the variation of the kinetic energy (see Eq. (7)) can be rewritten as

$$\begin{aligned} \begin{aligned}&-\int _{t_1 }^{t_2 } \int _0^L \left[ \left( {m+M} \right) \ddot{w}+M\dot{U}{w}'\right. \\&\qquad +\left. 2MU{\dot{w}}' \right] \delta w\mathrm{d}x_0 \mathrm{d}t \\&\quad =-\int _{t_1 }^{t_2 } \int _0^L \left( \int _{x_0 }^L \left[ \left( {m+M} \right) \ddot{w}+M\dot{U}{w}'\right. \right. \\&\qquad +\left. \left. 2MU{\dot{w}}' \right] \mathrm{d}x_0 \right) \cos \theta \delta \theta \mathrm{d}x_0 \mathrm{d}t \\ \end{aligned} \end{aligned}$$

(55)

An analogous variational procedure on the gravitational energy leads to

$$\begin{aligned}&\delta \int _{t_1 }^{t_2 } {\mathcal {G}\mathrm{d}t} \nonumber \\&\quad =\int _{t_1 }^{t_2 } \int _0^L {\left[ {\left( {m+M} \right) g\left( {L-x_0 } \right) \sin \theta \delta \theta } \right] \mathrm{d}x_0 } \mathrm{d}t\nonumber \\ \end{aligned}$$

(56)

Furthermore, the last term in Eq. (11) may be given by

$$\begin{aligned} B= & {} MU^{2}\int _{t_1 }^{t_2 } \left[ \cos \theta _L \left( {\delta \int _0^L {\cos \theta \mathrm{d}x_0 } } \right) \right. \nonumber \\&+\left. \sin \theta _L \left( {\delta \int _0^L {\sin \theta \mathrm{d}x_0 } } \right) \right] \mathrm{d}t \nonumber \\= & {} MU^{2}\int _{t_1 }^{t_2 } {\int _0^L {\sin \left( {\theta _L -\theta } \right) \delta \theta \mathrm{d}x_0 } \mathrm{d}t} \end{aligned}$$

(57)