Skip to main content
SpringerLink
Log in

Nonlinear dynamics of piezoelectric-based active nonlinear vibration absorber using time delay acceleration feedback

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

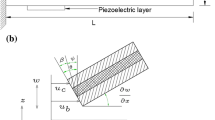
In this paper, dynamic analysis of an active nonlinear vibration absorber using lead zirconate titanate (PZT) stack actuator has been carried out considering time delay in the acceleration feedback. Here the primary system is modelled as a harmonically base excited system with a nonlinear spring, damper and mass which is subjected to an external harmonic force. The smart absorber consists of a nonlinear spring, mass and damper system along with a linear spring connected in series with the PZT stack actuator. In the proposed model, the active control force is produced by the combination of spring and the PZT stack actuator which requires less voltage compared to that of the conventional system where the actuator is directly connected to the primary system and one can tune the frequency ratio of the absorber actively. The nonlinear governing equation of motion of the system is derived and solved by using a modified harmonic balance method. The steady-state response is obtained by using Newton’s method, and the stability of the system is studied using the reduced equations. It has been shown that the proposed novel vibration absorber which has inbuilt fail-safe design can absorb the vibration of the system more effectively with negligible damping than the available passive and active vibration absorbers. Also, the Den Hartog’s equal peaks are achieved when the primary system is subjected to both harmonic force and base excitation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

References

  1. Dolatabadi, N., Theodossiades, S., Rothberg, S.J.: Passive control of piston secondary motion using nonlinear energy absorbers. J. Vib. Acoust. 139(5), 051009 (2017)

    Google Scholar 

  2. Reina, G., Rose, G.D.: Active vibration absorber for automotive suspensions: a theoretical study. Int. J. Heavy Veh. Syst. 23(1), 21–39 (2016)

    Google Scholar 

  3. Gong, D., Zhou, J.S., Sun, W.J.: On the resonant vibration of a flexible railway car body and its suppression with a dynamic vibration absorber. J. Vib. Control 19(5), 649–657 (2013)

    Google Scholar 

  4. El-Sayed, A.T., Kamel, M., Eissa, M.: Vibration reduction of a pitch-roll ship model with longitudinal and transverse absorbers under multi excitations. Math. Comput. Model. 52(9–10), 1877–1898 (2010)

    MathSciNet  MATH  Google Scholar 

  5. Yong, C., Zimcik, D.G., Wickramasinghe, V.K., Nitzsche, F.: Research of an active tunable vibration absorber for helicopter vibration control. Chin. J. Aeronaut. 16(4), 203–211 (2003)

    Google Scholar 

  6. Ebrahimzade, N., Dardel, M., Shafaghat, R.: Performance comparison of linear and nonlinear vibration absorbers in aeroelastic characteristics of a wing model. Nonlinear Dyn. 86(2), 1075–1094 (2016)

    Google Scholar 

  7. Rodriguez, J., Cranga, P., Chesne, S., Gaudiller, L.: Hybrid active suspension system of a helicopter main gearbox. J. Vib. Control 24(5), 956–974 (2018)

    Google Scholar 

  8. Shakeri, S., Samani, F.S.: Application of linear and nonlinear vibration absorbers in micro-milling process in order to suppress regenerative chatter. Nonlinear Dyn. 89(2), 851–862 (2017)

    Google Scholar 

  9. Miguélez, M.H., Rubio, L., Loya, J.A., Fernández-Sáez, J.: Improvement of chatter stability in boring operations with passive vibration absorbers. Int. J. Mech. Sci. 52(10), 1376–1384 (2010)

    Google Scholar 

  10. Viguié, R., Kerschen, G., Golinval, J.C., McFarland, D.M., Bergman, L.A., Vakakis, A.F., Van de Wouw, N.: Using passive nonlinear targeted energy transfer to stabilize drill-string systems. Mech. Syst. Signal Process. 23(1), 148–169 (2009)

    Google Scholar 

  11. Sun, J.Q., Jolly, M.R., Norris, M.A.: Passive, adaptive and active tuned vibration absorbers—a survey. J. Mech. Des. 117(B), 234–242 (1995)

    Google Scholar 

  12. Soto, M.G., Hojjat, A.: Tuned mass dampers. Arch. Comput. Methods Eng. 20(4), 419–431 (2013)

    Google Scholar 

  13. Munteanu, L., Chiroiu, V., Sireteanu, T.: On the response of small buildings to vibrations. Nonlinear Dyn. 73(3), 1527–1543 (2013)

    MathSciNet  Google Scholar 

  14. Frahm, H.: Device for damping vibrations of bodies, US Patent Nos. 989, 958, pp. 3576–3580 (1911)

  15. Ormondroyd, J., Den Hartog, J.P.: The theory of the dynamic vibration absorber. J. Appl. Mech. 50(7), 9–22 (1928)

    Google Scholar 

  16. Den Hartog, J.P.: Mechanical Vibrations. Dover Publications Inc., New York (1985)

    MATH  Google Scholar 

  17. Asami, T.: Optimal design of double-mass dynamic vibration absorbers arranged in series or in parallel. J. Vib. Acoust. 139(1), 011015 (2017)

    Google Scholar 

  18. Cheung, Y.L., Wong, W.O.: H\(_\infty \) and H\(_2\) optimizations of a dynamic vibration absorber for suppressing vibrations in plates. J. Sound Vib. 320(1–2), 29–42 (2009)

    Google Scholar 

  19. Buki, E., Katz, R., Zacksenhouse, M., Schlesinger, I.: Vib-bracelet: a passive absorber for attenuating forearm tremor. Med. Biol. Eng. Comput. 56, 923–930 (2018)

    Google Scholar 

  20. Wong, W.O., Fan, R.P., Cheng, F.: Design optimization of a viscoelastic dynamic vibration absorber using a modified fixed-points theory. J. Acoust. Soc. Am. 143(2), 1064–1075 (2018)

    Google Scholar 

  21. Fisco, N.R., Adeli, H.: Smart structures: part II—hybrid control systems and control strategies. Sci. Iran. 18(3), 285–295 (2011)

    Google Scholar 

  22. Viana, F.A.C., Kotinda, G.I., Rade, D.A., Steffen, J.V.: Tuning dynamic vibration absorbers by using ant colony optimization. Comput. Struct. 86(13–14), 1539–1549 (2008)

    Google Scholar 

  23. Krenk, S., Høgsberg, J.: Tuned mass absorber on a flexible structure. J. Sound Vib. 333(6), 1577–1595 (2014)

    Google Scholar 

  24. Cheung, Y.L., Wong, W.O., Cheng, L.: A subsystem approach for analysis of dynamic vibration absorbers suppressing broadband vibration. J. Sound Vib. 342, 75–89 (2015)

    Google Scholar 

  25. Pham, T.T., Pernot, S., Lamarque, C.H.: Competitive energy transfer between a two degree-of-freedom dynamic system and an absorber with essential nonlinearity. Nonlinear Dyn. 62(3), 573–592 (2010)

    MathSciNet  MATH  Google Scholar 

  26. Mohanty, S., Dwivedy, S.K.: Linear and nonlinear analysis of piezoelectric based vibration absorber with acceleration feedback. Proc. Eng. 144, 584–591 (2016)

    Google Scholar 

  27. Berardengo, M., Cigada, A., Guanziroli, F., Manzoni, S.: Modelling and control of an adaptive tuned mass damper based on shape memory alloys and eddy currents. J. Sound Vib. 349, 18–38 (2015)

    Google Scholar 

  28. Wang, X., Yang, B., Guo, S., Zhao, W.: Nonlinear convergence active vibration absorber for single and multiple frequency vibration control. J. Sound Vib. 411, 289–303 (2017)

    Google Scholar 

  29. Kim, S.M., Wang, S., Brennan, M.J.: Dynamic analysis and optimal design of a passive and an active piezo-electrical dynamic vibration absorber. J. Sound Vib. 330(4), 603–614 (2011)

    Google Scholar 

  30. Weber, F., Maślanka, M.: Frequency and damping adaptation of a TMD with controlled MR damper. Smart Mater. Struct. 21(5), 055011 (2012)

    Google Scholar 

  31. Nguyen, S.D., Choi, S., Nguyen, Q.H.: A new fuzzy-disturbance observer-enhanced sliding controller for vibration control of a train–car suspension with magneto-rheological dampers. Mech. Syst. Signal Process. 105, 447–466 (2018)

    Google Scholar 

  32. Benacchio, S., Malher, A., Boisson, J., Touzé, C.: Design of a magnetic vibration absorber with tunable stiffnesses. Nonlinear Dyn. 85(2), 893–911 (2016)

    MathSciNet  Google Scholar 

  33. Chatterjee, S.: Optimal active absorber with internal state feedback for controlling resonant and transient vibration. J. Sound Vib. 329, 5397–5414 (2010)

    Google Scholar 

  34. Fisco, N.R., Adeli, H.: Smart structures: part i—active and semi-active control. Sci. Iran. 18(3), 275–284 (2011)

    Google Scholar 

  35. Mallik, A.K., Chatterjee, S.: Principles of Passive and Active Vibration Control. East West Press, New Delhi (2014)

    Google Scholar 

  36. Bauomy, H.S.: Active vibration control of a dynamical system via negative linear velocity feedback. Nonlinear Dyn. 77(1–2), 413–423 (2014)

    MathSciNet  MATH  Google Scholar 

  37. Cheung, Y.L., Wong, W.O., Cheng, L.: Design optimization of a damped hybrid vibration absorber. J. Sound Vib. 331, 750–766 (2012)

    Google Scholar 

  38. Habib, G., Detroux, T., Viguié, R., Kerschen, G.: Nonlinear generalization of Den Hartog’s equal-peak method. Mech. Syst. Signal Process. 52, 17–28 (2015)

    Google Scholar 

  39. Gatti, G.: Fundamental insight on the performance of a nonlinear tuned mass damper. Meccanica 53, 111–123 (2017)

    MathSciNet  Google Scholar 

  40. Rizos, D., Feltrin, G., Motavalli, M.: Structural identification of a prototype pre-stressable leaf-spring based adaptive tuned mass damper: nonlinear characterization and classification. Mech. Syst. Signal Process. 25(1), 205–221 (2011)

    Google Scholar 

  41. Renault, A., Thomas, O., Mahé, H.: Numerical antiresonance continuation of structural systems. Mech. Syst. Signal Process. 116, 963–984 (2019)

    Google Scholar 

  42. Cirillo, G.I., Habib, G., Kerschen, G., Sepulchre, R.: Analysis and design of nonlinear resonances via singularity theory. J. Sound Vib. 392, 295–306 (2017)

    Google Scholar 

  43. Kučera, V., Pilbauer, D., Vyhlídal, T., Olgac, N.: Extended delayed resonators: design and experimental verification. Mechatronics 41, 29–44 (2017)

    Google Scholar 

  44. Bronkhorst, K.B., Febbo, M., Lopes, E.M., Bavastri, C.A.: Experimental implementation of an optimum viscoelastic vibration absorber for cubic nonlinear systems. Eng. Struct. 163, 323–331 (2018)

    Google Scholar 

  45. Carbajal, F.B., Navarro, G.S.: Active vibration in duffing mechanical systems using dynamic vibration absorbers. J. Sound Vib. 333, 3019–3030 (2014)

    Google Scholar 

  46. Ji, J.C.: Design of a nonlinear vibration absorber using three-to-one internal resonances. Mech. Syst. Signal Process. 42, 236–246 (2014)

    Google Scholar 

  47. Fallah, N., Ebrahimnejad, M.: Active control of building structures using piezoelectric actuators. Appl. Soft Comput. 13(1), 449–461 (2013)

    Google Scholar 

  48. Jalili, N., Knowles IV, D.W.: Structural vibration control using an active resonator absorber: modeling and control implementation. Smart Mater. Struct. 13(5), 998 (2004)

    Google Scholar 

  49. Chatterjee, S.: On the principle of impulse damper: a concept derived from impact damper. J. Sound Vib. 312(4–5), 584–605 (2008)

    Google Scholar 

  50. Zhao, Y.Y., Xu, J.: Using the delayed feedback control and saturation control to suppress the vibration of the dynamical system. Nonlinear Dyn. 67(1), 735–753 (2012)

    MathSciNet  MATH  Google Scholar 

  51. Collette, C., Chesne, S.: Robust hybrid mass damper. J. Sound Vib. 375, 19–27 (2016)

    Google Scholar 

  52. Amer, Y.A., El-Sayed, A.T., Kotb, A.A.: Nonlinear vibration and of the Duffing oscillator to parametric excitation with time delay feedback. Nonlinear Dyn. 85(4), 2497–2505 (2016)

    MathSciNet  Google Scholar 

  53. Olgac, N., Elmali, H., Hosek, M., Renzulli, M.: Active vibration control of distributed systems using delayed resonator with acceleration feedback. J. Dyn. Syst. Meas. Control 119, 380–389 (1997)

    MATH  Google Scholar 

  54. Rivaz, H., Rohling, R.: An active dynamic vibration absorber for a hand-held vibro-elastography probe. ASME J. Vib. Acoust. 129, 101–112 (2007)

    Google Scholar 

  55. Jalili, N., Olgac, N.: A sensitivity study on optimum delayed feedback vibration absorber. ASME J. Dyn. Syst. Meas. Control 122, 314–321 (2000)

    Google Scholar 

  56. Chatterjee, S.: Vibration control by recursive time delayed acceleration feedback. J. Sound Vib. 317(1–2), 67–90 (2008)

    Google Scholar 

  57. Habib, G., Kerschen, G., Stepan, G.: Chatter mitigation using the nonlinear tuned vibration absorber. Int. J. Non-linear Mech. 91, 103–112 (2017)

    Google Scholar 

  58. Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. Wiley, Hoboken (2008)

    MATH  Google Scholar 

  59. Anh, N.D., Nguyen, N.X.: Design of TMD for damped linear structures using the dual criterion of equivalent linearization method. Int. J. Mech. Sci. 77, 164–170 (2013)

    Google Scholar 

  60. Xiang, P., Nishitani, A.: Optimum design and application of non-traditional tuned mass damper toward seismic response control with experimental test verification. Earthq. Eng. Struct. Dyn. 44(13), 2199–2220 (2015)

    Google Scholar 

  61. Yang, C., Li, D., Li, C.: Dynamic vibration absorbers for vibration control within a frequency band. J. Sound Vib. 330(8), 1582–1598 (2011)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Indian Institute of Technology Guwahati, Guwahati, 781039, India

    S. Mohanty & S. K. Dwivedy

Authors
  1. S. Mohanty
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. K. Dwivedy
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Mohanty.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix-I

$$\begin{aligned} a_1= & {} -\,2\Omega \cos \varphi _1 +2\xi _1 \sin \varphi _1 \\&+\, 2F_{c1} \Omega \sin \varphi _1 \sin \Omega \tau \\&-\, 2F_{c1} \Omega \cos \varphi _1 \cos \Omega \tau \\ a_2= & {} 2\xi _1 A\cos \varphi _1 +2\Omega A\sin \varphi _1 \\&+\, 2F_{c1} A\Omega \sin \varphi _1 \cos \Omega _d \\&+\, 2F_{c1} A\Omega \cos \varphi _1 \sin \Omega \tau \\ a_3= & {} -\, 2\xi _2 \sin \varphi _2 \hbox {, }a_4 =-2\xi _2 B\cos \varphi _2 \\ a_5= & {} 2\Omega \sin (\varphi _1 )+2\xi _1 \cos (\varphi _1 ) \\&+\, 2F_{c1} \Omega \sin \varphi _1 \cos \Omega \tau \\&+\, 2F_{c1} \Omega \cos \varphi _1 \sin \Omega \tau \\ a_6= & {} -\, 2\xi _1 A\sin \varphi _1 +2\Omega A\cos \varphi _1 \\&-\, 2F_{c1} A\Omega \sin \varphi _1 \sin \Omega \tau \\&+\, 2F_{c1} A\Omega \cos \varphi _1 \cos \Omega \tau \\ a_7= & {} -\, 2\xi _2 \cos \varphi _2 \hbox {, }a_8 =2\xi _2 B\sin \varphi _2 \\ a_9= & {} -\, 2\mu \Omega \cos \varphi _1 -2F_{c1} \Omega \sin \varphi _1 \sin \Omega \tau \\&+\, 2F_{c1} \Omega \cos \varphi _1 \cos \Omega \tau \\ a_{10}= & {} 2\mu \Omega A\sin \varphi _1 -2F_{c1} A\Omega \sin \varphi _1 \cos \Omega \tau \\&-\, 2F_{c1} A\Omega \cos \varphi _1 \sin \Omega \tau \\ a_{11}= & {} -\,2\mu \Omega \cos \varphi _2 +2\xi _2 \sin \varphi _2 \\ a_{12}= & {} 2\xi _2 B\cos \varphi _2 +2\mu \Omega B\sin \varphi _2 \\ a_{13}= & {} 2\mu \Omega \sin \varphi _1 -2F_{c1} \Omega \sin \varphi _1 \cos \Omega \tau \\&-\, 2F_{c1} \Omega \cos \varphi _1 \sin \Omega \tau \\ a_{14}= & {} 2\Omega \mu A\cos \varphi _1 +2F_{c1} A\Omega \sin \varphi _1 \sin \Omega \tau \\&-\, 2F_{c1} A\Omega \cos \varphi _1 \cos \Omega \tau \\ a_{15}= & {} 2\mu \Omega \sin \varphi _2 +2\xi _2 \cos \varphi _2 \\ a_{16}= & {} -\, 2\xi _2 B\sin \varphi _2 +2\mu \Omega B\cos \varphi _2 \\ b_1= & {} N_1 \cos \varphi _1 +N_2 \sin \varphi _1 \\&+\, N_3 \cos \varphi _2 +N_4 \sin \varphi _2 +N_{b1} \\ b_2= & {} -\, N_1 \sin \varphi _1 +N_2 \cos \varphi _1 \\&-\, N_3 \sin \varphi _2 +N_4 \cos \varphi _2 -F_1 +N_{b2} \\ b_3= & {} N_5 \cos \varphi _2 +N_6 \sin \varphi _2 \\&+\, N_7 \sin \varphi _1 +N_8 \\ b_4= & {} -\, N_5 \sin \varphi _2 +N_6 \cos \varphi _2 \\&+\, N_7 \cos \varphi _1 +N_9 \\ N_1= & {} -\,2\xi _1 A\Omega ,N_2 =\left( {-\Omega ^{2}+1} \right) A, \\ N_3= & {} 2\xi _2 B\Omega ,N_4 =-\left( {\alpha B+0.75\beta B^{3}} \right) , \\ N_{b1}= & {} -\,\alpha _\mathrm{r} B\sin \varphi _2 +0.75\alpha _{13} A^{3}\sin \varphi _1 \\&-\, F_{c1} A\Omega ^{2}\sin \left( {\varphi _1 +\Omega \tau } \right) \\&-\, 0.75A^{2}Y\sin \left( {2\varphi _1 } \right) \cos \left( \gamma \right) \\&+\, 1.5\alpha _{13} AY^{2}\sin \varphi _1 \\&-\, 0.75\alpha _{13} AY^{2}\sin \varphi _1 \cos \left( {2\gamma } \right) \\&-\, Y\sin \left( \gamma \right) -0.75\alpha _{13} Y^{3}\sin \left( \gamma \right) \\&-\, 1.5\alpha _{13} A^{2}Y\sin \left( \gamma \right) \\&+\, 0.75\alpha _{13} A^{2}Y\cos \left( {2\varphi _1 } \right) \sin \left( \gamma \right) \\&-\, 0.75\alpha _{13} AY^{2}\sin \left( {2\gamma } \right) \cos \left( {\varphi _1 } \right) , \\ N_5= & {} -\,2\xi _2 B\Omega ,N_6 =-\mu \Omega ^{2}B+\alpha B+0.75\beta B^{3}, \\ N_7= & {} -\,\mu \Omega ^{2}A, \\ N_{b2}= & {} \alpha _\mathrm{r} B\cos \varphi _2 +0.75\alpha _{13} A^{3}\cos \varphi _1 \\&-\, F_{c1} A\Omega ^{2}\cos \left( {\varphi _1 +\Omega \tau } \right) -Y\cos \left( \gamma \right) \\&-\, 0.75\alpha _{13} Y^{3}\cos \left( \gamma \right) -1.5\alpha _{13} A^{2}Y\cos \left( \gamma \right) \\&-\, 0.75\alpha _{13} A^{2}Y\cos \left( \gamma \right) \cos \left( {2\varphi _1 } \right) \\&+\, 1.5\alpha _{13} AY^{2}\cos \left( {\varphi _1 } \right) \\&+\, 0.75\alpha _{13} AY^{2}\cos \left( {\varphi _1 } \right) \cos \left( {2\gamma } \right) \\&+\, 0.75\alpha _{13} A^{2}Y\sin \left( {2\varphi _1 } \right) \sin \left( \gamma \right) \\&+\, 0.75\alpha _{13} AY^{2}\sin \left( {2\gamma } \right) \sin \left( {\varphi _1 } \right) , \\ N_8= & {} -\,\alpha _\mathrm{r} B\sin \varphi _2 +F_{c1} A\Omega ^{2}\sin \left( {\varphi _1 +\Omega \tau } \right) , \\ N_9= & {} -\,\alpha _\mathrm{r} B\cos \varphi _2 +F_{c1} A\Omega ^{2}\cos \left( {\varphi _1 +\Omega \tau } \right) \end{aligned}$$

Jacobian matrix obtained as

$$\begin{aligned} J=\left[ {{\begin{array}{llll} {\frac{\partial f_1 }{\partial A}}&{} {\frac{\partial f_1 }{\partial \varphi _1 }}&{} {\frac{\partial f_1 }{\partial B}}&{} {\frac{\partial f_1 }{\partial \varphi _2 }} \\ {\frac{\partial f_2 }{\partial A}}&{} {\frac{\partial f_2 }{\partial \varphi _1 }}&{} {\frac{\partial f_2 }{\partial B}}&{} {\frac{\partial f_2 }{\partial \varphi _2 }} \\ {\frac{\partial f_3 }{\partial A}}&{} {\frac{\partial f_3 }{\partial \varphi _1 }}&{} {\frac{\partial f_3 }{\partial B}}&{} {\frac{\partial f_3 }{\partial \varphi _2 }} \\ {\frac{\partial f_4 }{\partial A}}&{} {\frac{\partial f_4 }{\partial \varphi _1 }}&{} {\frac{\partial f_4 }{\partial B}}&{} {\frac{\partial f_4 }{\partial \varphi _2 }} \\ \end{array} }} \right] , \end{aligned}$$

Appendix-II

Fig. 21
figure 21

a MATLAB SIMULINK model of the system b subsystem model for passive nonlinear vibration absorber and linear primary system with external harmonic excitation. c Subsystem model for active nonlinear vibration absorber and nonlinear primary system under harmonic external force and base excitation by time delay acceleration feedback

Full size image

The SIMULINK model for the passive and active system are shown in Fig. 21

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mohanty, S., Dwivedy, S.K. Nonlinear dynamics of piezoelectric-based active nonlinear vibration absorber using time delay acceleration feedback. Nonlinear Dyn 98, 1465–1490 (2019). https://doi.org/10.1007/s11071-019-05271-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-019-05271-4

Keywords

Search

Navigation