Semi-inverse method in nonlinear mechanics: application to couple shell- and beam-type oscillations of single-walled carbon nanotubes

Abstract

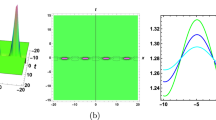
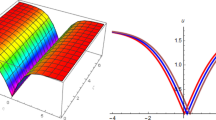
The resonant interaction of the nonlinear normal modes which belong to different vibration branches of the carbon nanotubes (CNTs) is studied by the efficient semi-inverse asymptotic method. Under the condition of the 1:1 resonance of the beam-like and circumferential flexure modes we obtain the dynamical equations, the solutions of which describe the coupled stationary states. They are characterized by the non-uniform distribution of the energy along the circumferential coordinate. The non-stationary solutions for the obtained equations correspond to the slow change of the energy distribution. It is shown that adequate description of considered resonance processes can be achieved in terms of new variables, which correspond to the coordinates of some domains of the CNT. These variables are the linear combinations of the shell- and beam-like normal modes. Using such variables we have analysed not only nonlinear normal modes, but also the limiting phase trajectories describing the strongly non-stationary dynamics. The evolution of the considered resonance processes with the oscillation amplitude growth is analysed by the phase portrait method and verified by the numerical integration of the respective dynamical equations.

Appendix

The kinetic energy of the CNT can be written as follows:

$$\begin{aligned} E_\mathrm{kin} = \frac{1}{2} \int _{0}^{1} \int _{0}^{2\pi } \left( \left( \frac{\partial u}{\partial t} \right) ^2 + \left( \frac{\partial v}{\partial t} \right) ^2 + \left( \frac{\partial w}{\partial t} \right) ^2 \right) d \xi d \theta . \end{aligned}$$

Taking into account the energy of elastic deformation (1), one can write the equations of motion, the linearized approximation of which can be represented as follows:

$$\begin{aligned} \frac{\partial ^2 u}{\partial t^2}= & {} -\,\alpha ^2 \frac{\partial ^2 u}{\partial \xi ^2}-\frac{1}{2} \frac{\partial ^2 u}{\partial \theta ^2}+\,\alpha (1-\nu ) \left( \frac{\partial ^2 v}{\partial \xi \partial \theta } \right) \\&+\,\frac{1}{96} \beta ^2 (\nu -1) \left( \frac{\partial ^2 u}{\partial \theta ^2}-3 \alpha \frac{\partial ^2 v}{\partial \xi \partial \theta }+4 \alpha \frac{\partial ^3 w}{\partial \xi \partial \theta ^2} \right) \\&-\,\alpha \nu \left( \frac{\partial ^2 v}{\partial \xi \partial \theta }+\frac{\partial w}{\partial \xi } \right) \\ \frac{\partial ^2 v}{\partial t ^2}= & {} -\,\alpha \nu \frac{\partial ^2 u}{\partial \xi \partial \theta }-\frac{1}{2} \alpha (1-\nu ) \left( \frac{\partial ^2 u}{\partial \xi \partial \theta } + \alpha \frac{\partial ^2 v}{\partial \xi ^2} \right) \\&+\,\frac{1}{32} \alpha \beta ^2 (\nu -1) \left( -\frac{\partial ^2 u}{\partial \xi \partial \theta } + 3 \alpha \frac{\partial ^2 v}{\partial \xi ^2} - 4 \alpha \frac{\partial ^3 w}{\partial \xi ^2 \partial \theta } \right) \\&-\,\frac{1}{12} \beta ^2 \left( \frac{\partial ^2 v}{\partial \theta ^2}- \alpha ^2 \nu \frac{\partial ^3 w}{\partial \xi ^2 \partial \theta } -\,\frac{\partial ^3 w}{\partial \theta ^3} \right) \\&- \left( \frac{\partial ^2 v }{\partial \theta ^2}+ \frac{\partial w}{\partial \theta } \right) \\ \frac{\partial ^2 w}{\partial t^2}= & {} \frac{1}{24} \alpha \beta ^2 (\nu -1) \left( -\frac{\partial ^3 u}{\partial \xi \partial \theta ^2} +\,3 \alpha \frac{\partial ^3 v}{\partial \xi ^2 \theta }-4 \alpha \frac{\partial ^4 w}{\partial \xi ^4} \right) \\&+\, \left( \alpha \nu \frac{\partial u}{\partial \xi } + \frac{\partial v}{\partial \theta } + w \right) \\&-\, \frac{1}{12} \beta ^2 \left( \frac{\partial ^3 v}{\partial \theta ^3} - \alpha ^2 \nu \frac{\partial ^4 w}{\partial \xi ^2 \partial \theta ^2} - \frac{\partial ^4 w}{\partial \theta ^4} \right) \\&+\,\frac{1}{12} \alpha ^2 \beta ^2 \left( -\nu \frac{\partial ^3 v}{\partial \xi ^2 \partial \theta }-\alpha ^2 \frac{\partial ^4 w}{\partial \xi ^4}+ \nu \frac{\partial ^4 w}{\partial \xi ^2 \partial \theta ^2} \right) . \end{aligned}$$