Vibration suppression of a time-varying stiffness AMB bearing to multi-parametric excitations via time delay controller

Abstract

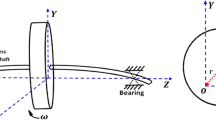
The applications of active magnetic bearings are growing in industry due to its amazing advantages in reducing friction losses. In this research, the vibration of a two-degree-of-freedom rotor, active magnetic bearings system is suppressed via a nonlinear time delay controller at the confirmed worst resonance case. The selected resonance case is the simultaneous primary and sub-harmonic resonance case. The main aim of this paper was to study the effects of the nonlinear, time delay controller on the behavior of the vibrating system. The multiple time scale perturbation technique is applied to obtain an approximate solution to the second-order approximation. The steady-state solution is obtained around the worst resonance case. The stability of the system is studied applying both frequency response equations and phase-plane method. The worst resonance case is confirmed applying numerical technique. The effects of the different parameters on the steady-state response of the vibrating system are investigated. The obtained approximate solution is validated numerically. Some recommendations are given regarding the design of such system. At the end of the work, a comparison is made with the available published work.

Appendix

$$\begin{aligned} \xi _1&= \frac{3}{4\omega _1 }(\alpha _2 +\omega _1^2 \alpha _6 )a^{2}+\frac{1}{2\omega _1 }(\alpha _3 +\omega _2^2 \alpha _6 )b^{2}\\&-g_1 \cos (\omega _1 t_{\dot{q}}) \\ \eta _1&= -\frac{1}{\omega _1^2 }f_{11}^2 \!+\! \left( \frac{\mu }{2}-\frac{3}{8}\alpha _5 a^{2}\!-\!\frac{1}{4}\alpha _5 b^{2}\!+\!\frac{g_1 }{2}\cos (\omega _1 t_{\dot{q}} )\!\right) ^{2}\\&+\left( \frac{3}{8\omega _1 }(\alpha _2 +\omega _1^2 \alpha _6 )a^{2} +\frac{1}{4\omega _1 }(\alpha _3 +\omega _2^2 \alpha _6 )b^{2}\right. \\&\quad \quad \left. -\frac{g_1 }{2}\sin (\omega _1 t_{\dot{q}})\right) ^{2} \\ \xi _2&= \frac{3}{4\omega _2 }(\beta _2 +\omega _2^2 \alpha _6 )b^{2}+\frac{1}{2\omega _2 }(\beta _3 +\omega _1^2 \alpha _6 )a^{2}\\&\quad -g_2 \sin (\omega _2 t_{\dot{q}} )\\ \eta _2&= -\frac{1}{16\omega _2^2 }F_2^2 +\left( \frac{\mu }{2}-\frac{3}{8}\alpha _5 b^{2}-\frac{1}{4}\alpha _5 a^{2}+\frac{g_2 }{2}\cos (\omega _2 t_{\dot{q}} )\right) ^{2} \\&+\left( \frac{3}{8\omega _2 }(\beta _2 +\omega _2^2 \alpha _6 )b^{2}+\frac{1}{4\omega _2 }(\beta _3 +\omega _1^2 \alpha _6 )a^{2}-\frac{g_2 }{2}\sin (\omega _2 t_{\dot{q}} )\right) ^{2}\\ \xi _3&= \frac{3}{4\omega _1 }(\alpha _2 +\omega _1^2 \alpha _6 )a^{2}-g_1 \cos (\omega _1 t_{\dot{q}} )\\ \eta _3&= -\frac{1}{\omega _1^2 }f_{11}^2 +\left( \frac{\mu }{2}-\frac{3}{8}\alpha _5 a^{2}+\frac{g_1 }{2}\cos (\omega _1 t_{\dot{q}} )\right) ^{2} \\&+\left( \frac{3}{8\omega _1 }(\alpha _2 +\alpha _6 \omega _1^2 )a^{2}-\frac{g_1 }{2}\sin (\omega _1 t_{\dot{q}} )\right) ^{2} \\ \xi _4&= \frac{3}{4\omega _2 }(\beta _2 +\omega _2^2 \alpha _6 )b^{2}-g_2 \sin (\omega _2 t_{\dot{q}} )\\ \eta _4&= -\frac{1}{16\omega _2^2 }F_2^2 +\left( \frac{\mu }{2}-\frac{3}{8}\alpha _5 b^{2}+\frac{g_2 }{2}\cos (\omega _2 t_{\dot{q}})\right) ^{2} \\&+\left( \frac{3}{8\omega _2 }(\beta _2 +\alpha _6 \omega _2^2 )b^{2}-\frac{g_2 }{2}\sin (\omega _2 t_{\dot{q}})\right) ^{2} \end{aligned}$$