Skip to main content
SpringerLink
Log in

A new fast and efficient active steganalysis based on combined geometrical blind source separation

  • Published:
Multimedia Tools and Applications Aims and scope Submit manuscript

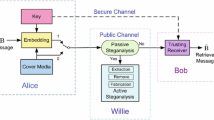
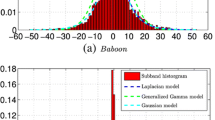
Abstract

We have presented a new active steganalysis method in order to break the block-discrete cosine transform (DCT) coefficients steganography. Our method is based on a combination of the Blind Source Separation (BSS) technique and Maximum A posteriori (MAP) estimator. We have additionally introduced a new geometrical BSS method based on the minimum range of mixed sources which reduces the computational cost of the proposed steganalysis. The high efficiency of this new combined method has been confirmed by enough experiments. These experiments show that, compared to the previous active steganalysis methods our active steganalysis method not only reduces the error rate but also causes a low computational cost.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Abate JE (1967) Linear and adaptive delta modulation. Proc IEEE 55:298–308

    Article  Google Scholar 

  2. Ambalavanan A, Chandramouli R (2007) Blind source separation for steganalytic secret message estimation. Proc SPIE. p 650507

  3. Avcibas I, Memon N, Sankur B (2003) Steganalysis using image quality metrics. Image Process IEEE Trans On 12:221–229

    Article  MathSciNet  Google Scholar 

  4. Azzabou N, Paragios N, Guichard F (2007) Uniform and textured regions separation in natural images towards MPM adaptive denoising. Scale Space Var Methods Comput Vis 418–429

  5. Babaie-Zadeh M, Jutten C, Nayebi K (2002) A geometric approach for separating post non-linear mixtures. Proc XI Eur. Signal Process. Conf. EUSIPCO 2002. pp 11–14.

  6. Chandramouli R (2003) A mathematical framework for active steganalysis. Multimed Syst 9:303–311

    Article  Google Scholar 

  7. Crouse MS, Nowak RD, Baraniuk RG (1998) Wavelet-based statistical signal processing using hidden Markov models. Signal Process IEEE Trans On 46:886–902

    Article  MathSciNet  Google Scholar 

  8. Fan F, Jiazhen W, Xiaoqin L, Huijuan F (2007) An Active Steganalysis Method of Block-DCT Image Information Hiding. Electron Meas Instrum. 2007 ICEMI07 8th Int Conf On. pp 2–849

  9. Fridrich J, Kodovskỳ J (2013) Steganalysis of LSB replacement using parity-aware features. Inf Hiding pp 31–45

  10. Harmsen JJ, Pearlman WA (2003) Steganalysis of additive-noise modelable information hiding. Proc SPIE pp 131–142

  11. Hyvarinen A (1999) Fast and robust fixed-point algorithms for independent component analysis. Neural Netw IEEE Trans On 10:626–634

    Article  Google Scholar 

  12. Hyvrinen A, Karhunen J, Oja E (2001) Independent component analysis. John Willey Sons

  13. Johnson NF, Jajodia S (1998) Steganalysis of images created using current steganography software. Inf. Hiding. pp 273–289

  14. Johnson NF, Jajodia S (1998) Steganalysis: the investigation of hidden information. Inf Technol Conf IEEE pp 113–116

  15. Jutten C, Jutten Zadeh M (2006) Source separation: principles, current advances and applications. IAR Annu Meet Nancy Fr 1–10

  16. Lyu S, Farid H (2006) Steganalysis using higher-order image statistics. Inf Forensic Secur IEEE Trans On 1:111–119

    Article  Google Scholar 

  17. MATLAB - The language of technical computing - math works. http://www.mathworks.com/products/matlab/. Accessed 10 Dec 2013

  18. Nissar A, Mir A (2010) Classification of steganalysis techniques: a study. Digit Signal Process 20:1758–1770

    Article  Google Scholar 

  19. Papoulis A, Probability RV (1991) Stochastic processes. McGraw-hill New York

  20. Pevny T, Bas P, Fridrich J (2010) Steganalysis by subtractive pixel adjacency matrix. IEEE Trans Inf Forensic Secur 5:215–224. doi:10.1109/TIFS.2010.2045842

    Article  Google Scholar 

  21. Portilla J, Strela V, Wainwright MJ, Simoncelli EP (2003) Image denoising using scale mixtures of Gaussians in the wavelet domain. Image Process IEEE Trans On 12:1338–1351

    Article  MATH  MathSciNet  Google Scholar 

  22. Puntonet C, Mansour A, Jutten C (1995) geometrical algorithm for blind separation of sources. Actes XVeme Colloq. GRETSI. pp 273–276.

  23. UC Berkeley Computer Vision Group - Contour Detection and Image Segmentation - Resources. http://www.eecs.berkeley.edu/Research/Projects/CS/vision/grouping/resources.html#bsds500. Accessed 31 Mar 2013

  24. Vielva L, Erdogmus D, Principe JC (2001) Underdetermined blind source separation using a probabilistic source sparsity model. Proc Int Conf Indep Compon Anal Blind Source Sep ICA. pp 675–679

  25. Wenzhe L, Bo X, Zhe Z, Wenxia R (2009) Active steganalysis with only one stego image. Fuzzy Syst Knowl Discov. 2009 FSKD09 Sixth Int Conf On. pp 345–348

  26. Xu B, Zhang Z, Wang J, Liu X (2007) Improved BSS based schemes for Active steganalysis. Softw Eng Artif Intell Netw ParallelDistributed Comput 2007 SNPD 2007 Eighth ACIS Int Conf On. pp 815–818

  27. Yan Y, Li LT, Xue JB et al (2013) Study on universal steganalysis for BMP images based on multi-domain features. Appl Mech Mater 278:1906–1909

    Article  Google Scholar 

  28. Yu X, Tan T, Wang Y (2004) Reliable detection of BPCS-steganography in natural images. Image Graph Proc Third Int Conf On pp 333–336

Download references

Author information

Authors and Affiliations

  1. Department of Electrical Engineering, Ferdowsi University of Mashhad, Mashhad, Iran

    Hamed Modaghegh & Seyed Alireza Seyedin

Authors
  1. Hamed Modaghegh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Seyed Alireza Seyedin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hamed Modaghegh.

Appendix

Appendix

Proof of Propositions which was stated in paper.

Proof of Proposition 1:

We have by (7):

$$ \begin{array}{cc}\hfill \theta =0,\pi \Rightarrow \left\{\begin{array}{c}\hfill {z}_1=\pm {s}_1\hfill \\ {}\hfill {z}_2=\pm {s}_2\hfill \end{array}\right.,\hfill & \hfill \theta =\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.,\raisebox{1ex}{$3\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.\Rightarrow \left\{\begin{array}{c}\hfill {z}_1=\pm {s}_2\hfill \\ {}\hfill {z}_2=\pm {s}_1\hfill \end{array}\right.\hfill \end{array} $$
(25)

So it is clear that:

$$ \min \left( \max {z}_1, \max {z}_2\right)= \min \left( \max {s}_1, \max {s}_2\right) $$
(26)

Now let us proof (8) for other θ. Without loss of generality assume:

$$ \max {s}_1=K \max {s}_2 $$
(27)

Then according to (1–1), we have:

$$ \begin{array}{l} \max {z}_1= \max {s}_2\times \left(\left| \cos \theta \right|K+\left| \sin \theta \right|\right)\\ {} \max {z}_2= \max {s}_2\times \left(\left| \sin \theta \right|K+\left| \cos \theta \right|\right)\end{array} $$
(28)
$$ \begin{array}{l} \max {z}_1= \max {s}_1\times \left(\left| \sin \theta \right|/K+\left| \cos \theta \right|\right)\\ {} \max {z}_2= \max {s}_1\times \left(\left| \cos \theta \right|/K+\left| \sin \theta \right|\right)\end{array} $$
(29)

Using (28) gives us:

$$ \begin{array}{l}K\ge 1\Rightarrow \min \left( \max {s}_1, \max {s}_2\right)= \max {s}_2\\ {}\kern2em , \max {z}_1\ge \max {s}_2\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\\ {}\kern2em , \max {z}_2\ge \max {s}_2\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\\ {}\kern2em \Rightarrow \max {z}_1, \max {z}_2\ge \min \left( \max {s}_1, \max {s}_2\right)\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\ \left(1-6\right)\end{array} $$
(30)

And for other value of K we use (29):

$$ \begin{array}{l}K<1\Rightarrow \min \left( \max {s}_1, \max {s}_2\right)= \max {s}_1\\ {}\kern2em , \max {z}_1\ge \max {s}_1\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\\ {}\kern2em , \max {z}_2\ge \max {s}_1\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\\ {}\kern2em \Rightarrow \max {z}_1, \max {z}_2\ge \min \left( \max {s}_1, \max {s}_2\right)\times \left(\left| \sin \theta \right|+\left| \cos \theta \right|\right)\end{array} $$
(31)

As it can be seen (30) and (31) are equal so it can be written:

$$ \max {z}_1, \max {z}_2\ge \min \left( \max {s}_1, \max {s}_2\right)\times \left(\left| \cos \theta \right|+\left| \sin \theta \right|\right) $$
(32)

Since \( \theta \ne 0,\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.,\pi, \raisebox{1ex}{$3\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right. \) and triangle inequality theorem:

$$ \begin{array}{l}\left| \cos \theta \right|+\left| \sin \theta \right|>1\Rightarrow \max {z}_1, \max {z}_2> \min \left( \max {s}_1, \max {s}_2\right)\\ {}\Rightarrow \min \left( \max {z}_1, \max {z}_2\right)> \min \left( \max {s}_1, \max {s}_2\right)\end{array} $$
(33)

Proof of Proposition 2:

According to (7), we have:

$$ \begin{array}{c}\hfill \max {z}_1=\left| \cos \theta \right| \max {s}_1+\left| \sin \theta \right| \max {s}_2\hfill \\ {}\hfill \max {z}_2=\left| \sin \theta \right| \max {s}_1+\left| \cos \theta \right| \max {s}_2\hfill \\ {}\hfill \min \left({z}_1\right)=\left| \cos \theta \right| \min \left({s}_1\right)+\left| \sin \theta \right| \min \left({s}_2\right)\hfill \\ {}\hfill \min \left({Z}_2\right)=\left| \sin \theta \right| \min \left({S}_1\right)+\left| \cos \theta \right| \min \left({S}_2\right)\hfill \end{array} $$
(34)

Without loss of generality assume:

$$ Range\left({s}_1\right)=K\times Range\left({s}_2\right) $$
(35)

Using (34) gives us:

$$ \begin{array}{c}\hfill Range\left({z}_1\right)= \max {z}_1- \min {z}_1=\left(\left| \cos \theta \right| \max {s}_1+\left| \sin \theta \right| \max {s}_2\right)-\left(\left| \cos \theta \right| \min {s}_1+\left| \sin \theta \right| \min {s}_2\right)\hfill \\ {}\hfill =\left| \cos \theta \right|\left( \max {s}_1- \min {s}_1\right)+\left| \sin \theta \right|\left( \max {s}_2- \min {s}_2\right)=\left| \cos \theta \right| Range\left({s}_1\right)+\left| \sin \theta \right| Range\left({s}_2\right)\hfill \\ {}\hfill =\left| \cos \theta \right|K\times Range\left({s}_2\right)+\left| \sin \theta \right| Range\left({s}_2\right)= Rang\left({s}_2\right)\times \left(\left| \cos \theta \right|K+\left| \sin \theta \right|\right)\hfill \end{array} $$
(36)
$$ Range\left({z}_1\right)=\left| \cos \theta \right| Range\left({s}_1\right)+\left| \sin \theta \right|/ KRange\left({s}_1\right)= Range\left({s}_1\right)\times \left(\left| \cos \theta \right|+\left| \sin \theta \right|/K\right) $$
(37)

In a similar way, we write above equations for Range(z 2 ):

$$ Range\left({z}_2\right)=\left| \sin \theta \right|K\times Range\left({s}_2\right)+\left| \cos \theta \right| Range\left({s}_2\right)= Range\left({s}_2\right)\times \left(\left| \sin \theta \right|K+\left| \cos \theta \right|\right) $$
(38)
$$ Range\left({z}_2\right)=\left| \sin \theta \right| Range\left({s}_1\right)+\left| \cos \theta \right|/ KRange\left({s}_1\right)= Range\left({s}_1\right)\left(\left| \sin \theta \right|+\left| \cos \theta \right|/K\right) $$
(39)

By using (35),(36) and (38) we obtain:

$$ \begin{array}{l}K\ge 1\kern0.48em \Rightarrow Range\left({s}_1\right)> Range\left({s}_2\right)\Rightarrow \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)= Range\left({s}_2\right)\\ {}\kern0.72em Range\left({z}_1\right)> Range\left({s}_2\right)\\ {}\kern0.6em Range\left({z}_2\right)> Range\left({s}_2\right)\\ {}\kern0.6em Range\left({z}_2\right), Range\left({z}_1\right)> Range\left({s}_2\right)= \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)\end{array} $$
(40)

Also (35), (37) and (39) give us:

$$ \begin{array}{l}K<1\kern0.48em \Rightarrow Range\left({s}_1\right)< Range\left({s}_2\right)\Rightarrow \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)= Range\left({s}_1\right)\\ {}\kern0.72em Range\left({z}_1\right)> Range\left({s}_1\right)\\ {}\kern0.6em Range\left({z}_2\right)> Range\left({s}_1\right)\\ {}\kern0.6em Range\left({z}_1\right), Range\left({z}_2\right)> Range\left({s}_1\right)= \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)\end{array} $$
(41)

So we can write for every K:

$$ \begin{array}{l} Range\left({z}_1\right), Range\left({z}_2\right)> \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)\\ {} \min \left( Range\left({z}_1\right), Range\left({z}_2\right)\right)> \min \left( Range\left({s}_1\right), Range\left({s}_2\right)\right)\end{array} $$
(42)

Proof of Proposition 3:

According to (12) and (13) for θ i  = − θ we have:

$$ \left[\begin{array}{c}\hfill {z}_1^{-\theta}\hfill \\ {}\hfill {z}_2^{-\theta}\hfill \end{array}\right]= rotate\left(-\theta \right) rotate\left(\theta \right)\left[\begin{array}{c}\hfill {s}_1\hfill \\ {}\hfill {s}_2\hfill \end{array}\right]=\left[\begin{array}{c}\hfill {s}_1\hfill \\ {}\hfill {s}_2\hfill \end{array}\right] $$
(43)

The Eq. (14) is true for every θ i so we can substitute it for − θ:

$$ \min \left({z}_{{}_1}^{\theta_0},{z}_2^{\theta_0}\right)\le \min \left({z}_1^{-\theta },{z}_2^{-\theta}\right)= \min \left({s}_1,{s}_2\right) $$
(44)

Also preposition 1 gives us:

$$ \min \left({z}_{{}_1}^{\theta_0},{z}_2^{\theta_0}\right)\ge \min \left({s}_1,{s}_2\right) $$
(45)

From (44) and (45) and sandwich theorem we obtain:

$$ \min \left({z}_{{}_1}^{\theta_0},{z}_2^{\theta_0}\right)= \min \left({s}_1,{s}_2\right) $$
(46)

Finally according to preposition 1 and (46) we can conclude that \( {\theta}_0=0,\raisebox{1ex}{$\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right.,\pi, \raisebox{1ex}{$3\pi $}\!\left/ \!\raisebox{-1ex}{$2$}\right. \) or:

$$ \left\{\begin{array}{c}\hfill {z}_{{}_1}^{\theta_0}=\pm {s}_1\hfill \\ {}\hfill {z}_{{}_2}^{\theta_0}=\pm {s}_2\hfill \end{array}\kern0.96em or\kern0.6em \right.\left\{\begin{array}{c}\hfill {z}_{{}_1}^{\theta_0}=\pm {s}_2\hfill \\ {}\hfill {z}_{{}_2}^{\theta_0}=\pm {s}_1\hfill \end{array}\right. $$
(47)

Rights and permissions

Reprints and permissions

About this article

Cite this article

Modaghegh, H., Seyedin, S.A. A new fast and efficient active steganalysis based on combined geometrical blind source separation. Multimed Tools Appl 74, 5825–5843 (2015). https://doi.org/10.1007/s11042-014-1890-9

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11042-014-1890-9

Keywords

Search

Navigation