Effect of the third invariant on the formation of necking instabilities in ductile plates subjected to plane strain tension

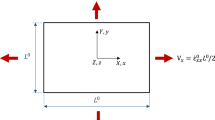
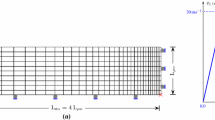
Abstract

In this paper, we have investigated the effect of the third invariant of the stress deviator on the formation of necking instabilities in isotropic metallic plates subjected to plane strain tension. For that purpose, we have performed finite element calculations and linear stability analysis for initial equivalent strain rates ranging from $10^{-4}\,\text {s}^{-1}$ to $8 \cdot 10^{4}\,\text {s}^{-1}$. The plastic behavior of the material has been described with the isotropic Drucker (J Appl Mech 16:349–357, 1949) yield criterion, which depends on both the second and third invariant of the stress deviator, and a parameter c which determines the ratio between the yield stresses in uniaxial tension and in pure shear $\sigma _T / \tau _Y$. For $c=0$, Drucker (J Appl Mech 16:349–357, 1949) yield criterion reduces to the von Mises (ZAMM J Appl Math Mech/Zeitschrift für Angewandte Mathematik und Mechanik 8(3):161–185, 1928) yield criterion while for $c=81/66$, the Hershey–Hosford (J Appl Mech 76:241–249, 1954; Proceedings of the seventh North American metalworking research conference, 1979) $\left( m=6\right)$ yield criterion is recovered. The results obtained with both finite element calculations and linear stability analysis show the same overall trends and there is also quantitative agreement for most of the loading rates considered. In the quasi-static regime, while the specimen elongation when necking occurs is virtually insensitive to the value of the parameter c, both finite element results and analytical calculations using Considère (Ann Ponts et Chaussées 9:574–775, 1885) criterion show that the necking strain increases as the parameter c decreases, bringing out the effect of the third invariant of the stress deviator on the formation of quasi-static necks. In contrast, at high initial equivalent strain rates, when the influence of inertia on the necking process becomes important, both finite element simulations and linear stability analysis show that the effect of the third invariant is reversed, notably for long necking wavelengths, with the specimen elongation when necking occurs increasing as the parameter c increases, and the necking strain decreasing as the parameter c decreases.

Appendices

Appendix A: Stability analysis results and finite element calculations with prescribed stretch rate

In this section, we show finite element results and stability analysis predictions obtained imposing the initial axial strain rate ${\dot{\varepsilon }}^0_{xx}$ as the loading condition, instead of the initial equivalent strain rate $\dot{{\bar{\varepsilon }}}^0$, as in all other calculations performed in this work (see Sect. 3.1). The results correspond to Material 2 ($c=-1.5$), Material 3 ($c=81/66$) and Material 4 ($c=2.25$). The stability analysis predictions are obtained with the critical cumulative instability index $I_c=3.75$. We also include finite element results for Material 2 imposing the initial equivalent strain rate.

Figure 17a, b show the evolution of the necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ and the necking stretch ${F}^{\textit{neck}}_{xx}$ with $L^0/h^0$ for ${\dot{\varepsilon }}^0_{xx}=4000\,\text {s}^{-1}$. The results obtained for the three materials are very similar to those reported Fig. 10a, b for an imposed initial equivalent strain rate of $\dot{{\bar{\varepsilon }}}^0=4000\,\text {s}^{-1}$. Moreover, Fig. 18a, b display the ${\bar{\varepsilon }}^{\textit{neck}} - L^0/h^0$ and ${F}^{\textit{neck}}_{xx}-L^0/h^0$ curves for ${\dot{\varepsilon }}^0_{xx}=80{,}000\,\text {s}^{-1}$. The results are also quantitatively similar to those reported in Fig. 13a, b for $\dot{{\bar{\varepsilon }}}^0=80{,}000\,\text {s}^{-1}$, suggesting that imposing either the initial equivalent strain rate or the initial stretch rate in the calculations yields similar results. However, there are qualitative differences on the effect of the parameter c on the necking strain and the necking stretch. Specifically, both finite element calculations and stability analysis predictions presented in Fig. 18a show that for ${\dot{\varepsilon }}^0_{xx}=80{,}000\,\text {s}^{-1}$ the necking strain is greater as the parameter c decreases (i.e. as the rate of accumulation of plastic deformation increases), while this is not the case when the initial equivalent strain rate is imposed, since the smaller necking strain for long wavelengths corresponds to $c=-1.5$ (see Fig. 13a). In addition, Fig. 18b shows that, while the relative order of the ${F}^{\textit{neck}}_{xx}-L^0/h^0$ curves for the three materials is the same obtained for $\dot{{\bar{\varepsilon }}}^0=80{,}000\,\text {s}^{-1}$, the difference in the necking stretch for the whole range of wavelengths is less (see Fig. 13b).

Fig. 17

Comparison between finite element results (FEM) and linear stability analysis predictions (LSA) obtained with Drucker yield criterion [11] for Material 2 ($c=-1.5$ and $\sigma _T / \tau _Y=1.674$), Material 3 (Hershey–Hosford for a BCC material, $c=81/66$ and $\sigma _T / \tau _Y=1.790$) and Material 4 ($c=2.25$ and $\sigma _T / \tau _Y=1.852$), respectively. The initial stretch rate along the axial direction is ${\dot{\varepsilon }}^0_{xx}=4000\,\text {s}^{-1}$. a Necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ versus $L^0/h^0$. b Necking stretch ${F}^{\textit{neck}}_{xx}$ versus $L^0/h^0$. In the finite element simulations the amplitude of the imperfection is $\Delta =0.2{\%}$. In the linear stability analysis the critical cumulative instability index is $I_c=3.75$. We also include finite element results for Material 2 ($c=-1.5$) imposing the initial equivalent strain rate $\dot{{\bar{\varepsilon }}}^0=4000\,\text {s}^{-1}$

Fig. 18

Comparison between finite element results (FEM) and linear stability analysis predictions (LSA) obtained with Drucker yield criterion [11] for Material 2 ($c=-1.5$ and $\sigma _T / \tau _Y=1.674$), Material 3 (Hershey–Hosford for a BCC material, $c=81/66$ and $\sigma _T / \tau _Y=1.790$) and Material 4 ($c=2.25$ and $\sigma _T / \tau _Y=1.852$), respectively. The initial stretch rate along the axial direction is ${\dot{\varepsilon }}^0_{xx}=80{,}000\,\text {s}^{-1}$. a Necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ versus $L^0/h^0$. b Necking stretch ${F}^{\textit{neck}}_{xx}$ versus $L^0/h^0$. In the finite element simulations the amplitude of the imperfection is $\Delta =0.2{\%}$. In the linear stability analysis the critical cumulative instability index is $I_c=3.75$. We also include finite element results for Material 2 ($c=-1.5$) imposing the initial equivalent strain rate $\dot{{\bar{\varepsilon }}}^0=80{,}000\,\text {s}^{-1}$

Appendix B: Additional comparison between critical instantaneous instability index and critical cumulative instability index

In this section, we present additional comparisons between the predictions obtained with the critical instantaneous instability index ${\hat{\eta }}^+_c=19$ and the critical cumulative instability index $I_c=3.75$ for Material 1 (von Mises material, $c=0$). Namely, Fig. 19 shows the evolution of the necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ with $L^0/h^0$ for five different initial equivalent strain rates $\dot{{\bar{\varepsilon }}}^0=400\,\text {s}^{-1}$, $4000\,\text {s}^{-1}$, $10{,}000\,\text {s}^{-1}$, $20{,}000\,\text {s}^{-1}$ and $80{,}000\,\text {s}^{-1}$. The stability analysis results are compared with finite element calculations.

For the lower initial equivalent strain rates, $400\,\text {s}^{-1}$, $4000\,\text {s}^{-1}$ and $10{,}000\,\text {s}^{-1}$, the predictions of the stability analysis with both criteria are similar (especially for $400\,\text {s}^{-1}$), and find qualitative and quantitative agreement with the finite element calculations, being the predictions obtained with ${\hat{\eta }}^+_c=19$ closer to the numerical simulations. On the other hand, for greater initial equivalent strain rates, there are important differences between the results obtained with ${\hat{\eta }}^+_c=19$ and $I_c=3.75$ so that, contrary to what occurs at lower strain rates, for long wavelengths the instantaneous instability index predicts values of the necking strain greater than the cumulative instability index (see also Fig. 6). The different results obtained with both criteria are particularly noticeable for $80{,}000\,\text {s}^{-1}$, for this strain rate the predictions with $I_c=3.75$ being much closer to the finite element calculations than the results obtained with ${\hat{\eta }}^+_c=19$, showing that the analytical predictions based on the instantaneous instability index overestimate the role of inertia on the necking strain.

Fig. 19

Necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ versus $L^0/h^0$. Comparison between finite element results (FEM) and linear stability analysis predictions (LSA) obtained with Material 1 (von Mises material, $c=0$ and $\sigma _T / \tau _Y=\sqrt{3}$). The initial equivalent strain rate is: a $\dot{{\bar{\varepsilon }}}^0=400\,\text {s}^{-1}$, b $\dot{{\bar{\varepsilon }}}^0=4000\,\text {s}^{-1}$, c $\dot{{\bar{\varepsilon }}}^0=10{,}000\,\text {s}^{-1}$, d $\dot{{\bar{\varepsilon }}}^0=20{,}000\,\text {s}^{-1}$ and e $\dot{{\bar{\varepsilon }}}^0=80{,}000\,\text {s}^{-1}$. In the finite element simulations the amplitude of the imperfection is $\Delta =0.2{\%}$. Linear stability analysis results are shown for the critical cumulative instability index $I_c=3.75$, and the critical instantaneous instability index ${\hat{\eta }}^+_c=19$

Appendix C: Stability analysis results with strain rate dependent critical cumulative instability index

In this section, we compare finite element results with stability analysis predictions obtained using strain rate dependent critical cumulative index. The calculations are performed with Material 1 (von Mises material, $c=0$ and $\sigma _T / \tau _Y=\sqrt{3}$), and the results correspond to four different initial equivalent strain rates: $400\,\text {s}^{-1}$, $10{,}000\,\text {s}^{-1}$, $20{,}000\,\text {s}^{-1}$ and $80{,}000\,\text {s}^{-1}$. The critical cumulative instability index is taken to be a second order polynomial $I_c=F+ G \, \dot{{\bar{\varepsilon }}}^0 + H \left( \dot{{\bar{\varepsilon }}}^0\right) ^2$, whose coefficients $F=0.96454$, $G=8.883 \cdot 10^{-5}$ and $H=-4.7983 \cdot 10^{-10}$ have been determined obtaining the value of $I_c$ for three initial equivalent strain rates, $400\,\text {s}^{-1}$, $40{,}000\,\text {s}^{-1}$ and $80{,}000\,\text {s}^{-1}$, following the methodology discussed in Sect. 6.1. Similar procedure has been recently used by Jacques and Rodríguez-Martínez [25] to determine the evolution of the critical cumulative index with the strain rate, to predict multiple necking formation in viscoplastic metallic bars subjected to dynamic stretching.

Figure 20 shows that the stability analysis predictions obtained with strain rate dependent critical cumulative index are in quantitative agreement with the unit-cell calculations, within the whole range of wavelengths investigated, and for all the strain rates considered (including strain rates other than those used to calibrate $I_c$). It becomes apparent that accounting for the strain rate dependence of $I_c$ improves the predictions of the stability analysis, yet at the expense of needing additional calibration data. A throughout discussion of the pros and cons of considering the functional dependence of $I_c$ on the strain rate is left for a future work.

Fig. 20

Necking strain ${\bar{\varepsilon }}^{\textit{neck}}$ versus $L^0/h^0$. Comparison between finite element results (FEM) and linear stability analysis predictions (LSA) obtained for Material 1 (von Mises material, $c=0$ and $\sigma _T / \tau _Y=\sqrt{3}$). The results correspond to four different initial equivalent strain rates $\dot{{\bar{\varepsilon }}}^0=400\,\text {s}^{-1}$, $10{,}000\,\text {s}^{-1}$, $20{,}000\,\text {s}^{-1}$ and $80{,}000\,\text {s}^{-1}$. In the finite element simulations the amplitude of the imperfection is $\Delta =0.2{\%}$. In the linear stability analysis the critical cumulative instability index is taken strain rate dependent $I_c=0.96454+8.883 \cdot 10^{-5} \, \dot{{\bar{\varepsilon }}}^0-4.7983 \cdot 10^{-10} \cdot \left( \dot{{\bar{\varepsilon }}}^0\right) ^2$