Linear stability of piezoelectric-controlled discrete mechanical systems under nonconservative positional forces

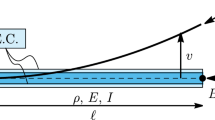
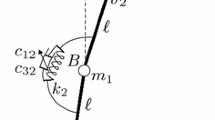
Abstract

Discrete models of piezoelectric controlled mechanical systems, subjected to follower forces, are derived in this paper. A targeted strategy of control is first discussed, leading to detect three novel linear controllers resembling, in the order, the Tuned Mass Damper with large and small mass, respectively, and Energy Sink, used in the literature for controlling linear and nonlinear oscillations. Here, however, and differently from those systems, coupling between the controller and the main structure is of gyroscopic type, whose magnitude is governed by an electro-mechanical parameter. Based on an eigenvalue sensitivity analysis, carried out via suitably selected perturbation methods, the effectiveness of the three controllers is investigated. As an application, the two-degree-of-freedom Ziegler column, undergoing Hopf bifurcation triggered by a follower force, equipped with piezoelectric controllers, is studied, and the different strategies proposed numerically compared.

Appendix: Discrete piezoelectro-mechanical model

We consider a discrete piezoelectro-mechanical system. In it, we distinguish three subsystems, each made of elementary components, namely: a structure, consisting of masses (M), springs (S) and dashpots (D); an electrical circuit, made of capacitors (C), inductors (L), resistors (R) and, occasionally, transformers; a set of piezoelectric devices (P).

The system is conveniently regarded as constituted by point joints, connected by one-dimensional devices. We consider the structure made by mechanical joints, at which masses are lumped, interconnected by S and D devices. Similarly, we consider the circuit as constituted by electrical joints, linked by C,L,R devices. Finally, each of the P devices links a couple of mechanical joints and a couple of electrical joints. The state of the system is defined by n _m displacements ${\mathbf{X}}:=\left\{ X_{I}\right\}$ of the mechanical joints and n _e flux linkages ${\mathbf{Y}}:=\left\{ \psi _{J}\right\}$ at the electrical joints, together with their time-derivatives ${\dot{\mathbf{X}}},{\dot{\mathbf{Y}}}$ (we remember that the flux linkage at a point is defined as the time-integral of the potential V _J at that point, namely ${{\dot{\psi}}}_{J}:=V_{J}$).

1.1 Constitutive laws

The linear constitutive laws of the structure components are:

$$f_{i}^{S}=k_{i}\varDelta l_{i}^{S},\qquad f_{i}^{D}=b_{i}\varDelta \dot{l}_{i}^{D}$$

(39)

where $f_{i}^{H}\,\left( H=S,D\right)$ are forces, k _i spring stiffnesses, b _i damping coefficients and $\varDelta l_{i}^{H}$ elongations.

The constitutive laws of the circuit components are:

$$Q_{j}^{C}=C_{j}\varDelta {\dot{\psi}}_{j}^{C},\qquad \dot{Q}_{j}^{L}=\frac{1}{L_{j}}\varDelta \psi _{j}^{L},\qquad \dot{Q}_{j}^{R}=\frac{1}{R_{j}}\varDelta {\dot{\psi}}_{j}^{R}$$

(40)

where $Q_{j}^{\alpha }\,\left( \alpha =C,L,R\right)$ are electric charges, $C_{j},\, L_{j},\, R_{j}$ capacitances, inductances and resistances, respectively, and $\varDelta \psi _{j}^{\alpha }$ is the flux-linkage difference between the two terminals of the device. According to a (one of the two possible [37]) electro-mechanical analogy, the capacitance corresponds to the mass, the inverse of the inductance to the stiffness, the inverse of the resistance to the viscous damping, while the time-derivative of the charge corresponds to the force, and the flux linkage to the displacement.

The constitutive law of the piezoelectric components is taken in the following gyroscopic-type form [58]:

$$\left\{ \begin{array}{l} f_{k}^{P}\\ Q_{k}^{P} \end{array}\right\} =\left[ \begin{array}{ll} k_{k}^{P} &{} -g_{k}^{P}\\ g_{k}^{P} &{} C_{k}^{P} \end{array}\right] \left\{ \begin{array}{l} \varDelta l_{k}^{P}\\ \varDelta {\dot{\psi}}_{k}^{P} \end{array}\right\}$$

(41)

where $k_{k}^{P}$, $C_{k}^{P}$, $g_{k}^{P}$ are stiffness, capacitance and electro-mechanical coupling, respectively.

By collecting forces ${\mathbf{f}}_{\alpha }:=\left\{ f_{i}^{\alpha }\right\}$ $\left( \alpha =S,D,P\right)$ as well charges ${\mathbf{Q}}_{\alpha }:=\left\{ Q_{j}^{\alpha }\right\}$ $\left( \alpha =C,L,R,P\right)$, the constitutive laws (39), (40), (41) also read:

$$\begin{aligned} \begin{aligned} {\mathbf{f}}_{S}&={\mathbf{k}}{\mathbf{\varvec{\Updelta}}}\varvec{l}_{S},\,\,\,\,\,\,\,\,{\mathbf{f}}_{D}={\mathbf{b}}{\mathbf{\varvec{\Updelta}}}{\dot{\varvec l}}_{D}\\ {\mathbf{Q}}_{C}&={\mathbf{C}}\varvec{\Updelta }{\dot{{\varvec{\psi}}}}_{C},\,\,{\dot{\mathbf{Q}}}_{L}={\mathbf{L}}^{-1}\varvec{\Updelta }\varvec{\psi }_{L},\;{\dot{\mathbf{Q}}}_{R}={\mathbf{R}}^{-1}\varvec{\Updelta }{\dot{{\varvec{\psi}}}}_{R}\\ \left\{ \begin{array}{l} {\mathbf{f}}_{P}\\ {\mathbf{Q}}_{P} \end{array}\right\}&=\left[ \begin{array}{ll} {\mathbf{k}}_{P} &{} -{\mathbf{g}}_{P}\\ {\mathbf{g}}_{P} &{} {\mathbf{C}}_{P} \end{array}\right] \left\{ \begin{array}{l} {\mathbf{\varvec{\Updelta}}}\varvec{l}_{P}\\ \varvec{\Updelta }{\dot{{\varvec{\psi}}}}_{P} \end{array}\right\} \end{aligned} \end{aligned}$$

(42)

where:

$$\begin{aligned} \begin{aligned}{\mathbf{k}}&:={\rm diag}\left[ k_{i}\right] ,&{\mathbf{b}}&:={\rm diag}\left[ b_{i}\right] \\ {\mathbf{C}}&:={\rm diag}\left[ C_{j}\right] ,&{\mathbf{L}}^{-1}&:={\rm diag}\left[ 1/L_{j}\right] ,&\,{\mathbf{R}}^{-1}&:={\rm diag}\left[ 1/R_{j}\right] \\ {\mathbf{k}}_{P}&:={\rm diag}\left[ k_{k}^{P}\right] ,&{\mathbf{C}}_{P}&:={\rm diag}\left[ C_{k}^{P}\right] ,&\,{\mathbf{g}}_{P}&:={\rm diag}\left[ g_{k}^{P}\right] \end{aligned} \end{aligned}$$

(43)

1.2 Topology

Accounting for topology (and geometry) of the structure, the set of the spring elongations ${\mathbf{\varvec{\Updelta}}}\varvec{l}_{S}:=\left\{ \Updelta l_{i}^{S}\right\}$ and of the dashpot elongation-rates ${\mathbf{\varvec{\Updelta}}}{\dot{\varvec l}}_{D}:=\left\{ \Updelta \dot{l}_{i}^{D}\right\}$ is expressed in terms of nodal displacements and velocities:

$$\begin{aligned} {\mathbf{\varvec{\Updelta}}}\varvec{l}_{S}={\mathbf{D}}_{S}{\mathbf{X}},\qquad {\mathbf{\varvec{\Updelta}}}{\dot{\varvec l}}_{D}={\mathbf{D}}_{D}{\dot{\mathbf{X}}} \end{aligned}$$

(44)

where ${\mathbf{D}}_{H}\,\left( H=S,D\right)$ are kinematic operators. Topology of the circuit provides the differences of the flux linkages and their derivatives, $\varvec{\Delta }{\dot{{\varvec{\psi}}}}_{C}:=\left\{ \Delta {\dot{\psi}}_{j}^{C}\right\}$, $\varvec{\Delta }\varvec{\psi }_{L}:=\left\{ \Delta \psi _{j}^{L}\right\}$, $\varvec{\Delta }{\dot{{\varvec{\psi}}}}_{R}:=\left\{ \Delta {\dot{\psi}}_{j}^{R}\right\}$, expressed in terms of the homologous quantities at the joints:

$$\begin{aligned} \varvec{\Delta }{\dot{{\varvec{\psi}}}}_{C}={\mathbf{D}}_{C}{\dot{\mathbf{Y}}},\qquad \varvec{\Delta }\varvec{\psi }_{L}={\mathbf{D}}_{L}{\mathbf{Y}},\qquad \varvec{\Delta }{\dot{{\varvec{\psi}}}}_{R}={\mathbf{D}}_{R}{\dot{\mathbf{Y}}} \end{aligned}$$

(45)

For the piezoelectric arrangement, for which ${\mathbf{\varvec{\Delta}}}\varvec{l}_{P}:=\left\{ \Delta l_{k}^{P}\right\}$, $\varvec{\Delta }{\dot{{\varvec{\psi}}}}_{P}:=\left\{ \Delta {\dot{\psi}}_{k}^{P}\right\}$, we similarly have:

$$\begin{aligned} {\mathbf{\varvec{\Delta}}}\varvec{l}_{P}={\mathbf{D}}_{PM}{\mathbf{X}},\qquad \varvec{\Delta }{\dot{{\varvec{\psi}}}}_{P}={\mathbf{D}}_{PE}{{{\dot{\mathbf{Y}}}}} \end{aligned}$$

(46)

All the ${\mathbf{D}}_{H}$ will be referred as topological operators.

1.3 Hamilton principle

The evolution equations are derived by the extended Hamilton principle:

$$\begin{aligned} \delta H:=\int _{t_{1}}^{t_{2}}\left( \delta T-\delta U+\delta W\right) {\text {d}}t=0,\quad \forall \,\delta {\mathbf{X}},{\mathbf{\delta Y}} \end{aligned}$$

(47)

where T is kinetic energy, U the potential energy, and $\delta W$ is the work of the nonconservative forces done in the virtual variation of the state, $\delta {\mathbf{X}},{\mathbf{\delta Y}}.$ Moreover $\left[ t_{1},t_{2}\right]$ is an arbitrary interval of time, at whose ends motion is prescribed.

Concerning the kinetic energy, this is the sum of two contributions, relevant to the masses of the structure and of the piezoelectric devices, respectively; this latter, however, is usually negligible, so that:

$$\begin{aligned} T=\frac{1}{2}{\dot{\mathbf{X}}}^{T}{\mathbf{M}}_{M}{\dot{\mathbf{X}}} \end{aligned}$$

(48)

where ${\mathbf{M}}_{M}$ is the structure inertia matrix.

The potential energy of the system is the sum of four contributions, namely $U:=U_{S}+U_{C}+U_{L}+U_{P}$, relevant to springs, capacitors, inductors and piezoelectric devices, respectively. Accounting for the constitutive laws (42) and for the topology (44)-(46), they read:

$$\begin{aligned} \begin{aligned}&U_{S}=\frac{1}{2}{\mathbf{f}}_{S}^{T}{\mathbf{\varvec{\Delta}}}\varvec{l}_{S}=\frac{1}{2}{\mathbf{X}}^{T}{\mathbf{D}}_{S}^{T}{\mathbf{k}}{\mathbf{D}}_{S}{\mathbf{X}}=\frac{1}{2}{\mathbf{X}}^{T}{\mathbf{K}}_{S}{\mathbf{X}}\\&U_{C}=-\frac{1}{2}{\mathbf{Q}}_{C}^{T}\varvec{\Delta }{\dot{{\varvec{\psi}}}}_{C}=-\frac{1}{2}{\dot{\mathbf{Y}}}^{T}{\mathbf{D}}_{C}^{T}{\mathbf{C}}{\mathbf{D}}_{C}{\dot{\mathbf{Y}}}=-\frac{1}{2}{\dot{\mathbf{Y}}}^{T}{\mathbf{M}}_{C}{\dot{\mathbf{Y}}}\\&U_{L}=\frac{1}{2}{\dot{\mathbf{Q}}}_{L}^{T}\varvec{\Delta }\varvec{\psi }_{L}=\frac{1}{2}{\mathbf{Y}}^{T}{\mathbf{D}}_{L}^{T}{\mathbf{L}}^{-1}{\mathbf{D}}_{L}{\mathbf{Y}}=\frac{1}{2}{\mathbf{Y}}^{T}{\mathbf{K}}_{L}{\mathbf{Y}} \end{aligned} \end{aligned}$$

(49)

while:

$$\begin{aligned} \begin{aligned}&U_{P} = \;\;\frac{1}{2}\left( {\mathbf{f}}_{P}^{T}{\mathbf{\varvec{\Delta}}}\varvec{l}_{P}-{\mathbf{Q}}_{P}^{T}\varvec{\Delta }{\dot{{\varvec{\psi}}}}_{P}\right) \\&= \;\;\frac{1}{2}\left( {\mathbf{X}}^{T}{\mathbf{D}}_{PM}^{T}{\mathbf{k}}_{P}{\mathbf{D}}_{PM}{\mathbf{X}}-{\dot{\mathbf{Y}}}^{T}{\mathbf{D}}_{PE}^{T}{\mathbf{C}}_{P}{\mathbf{D}}_{PE}{\dot{\mathbf{Y}}}\right) \\&\;\; -\frac{1}{2}\left( {\dot{\mathbf{Y}}}^{T}{\mathbf{D}}_{PE}^{T}{\mathbf{g}}_{P}{\mathbf{D}}_{PM}{\mathbf{X}}+{\mathbf{X}}^{T}{\mathbf{D}}_{PM}^{T}{\mathbf{g}}_{P}{\mathbf{D}}_{PE}{\dot{\mathbf{Y}}}\right) \\&= \;\;\frac{1}{2}\left( {\mathbf{X}}^{T}{\mathbf{K}}_{P}{\mathbf{X}}-{\dot{\mathbf{Y}}}^{T}{\mathbf{M}}_{P}{\dot{\mathbf{Y}}}\right) -{\dot{\mathbf{Y}}}^{T}{\mathbf{G}}{\mathbf{X}} \end{aligned} \end{aligned}$$

(50)

Here, the following positions hold for matrices:

$$\begin{aligned} \begin{aligned}{\mathbf{K}}_{S}&:={\mathbf{D}}_{S}^{T}{\mathbf{k}}{\mathbf{D}}_{S},&\,{\mathbf{K}}_{L}&:={\mathbf{D}}_{L}^{T}{\mathbf{L}}^{-1}{\mathbf{D}}_{L},&{\mathbf{K}}_{P}&:={\mathbf{D}}_{PM}^{T}{\mathbf{k}}_{P}{\mathbf{D}}_{PM}\\ {\mathbf{M}}_{C}&:={\mathbf{D}}_{C}^{T}{\mathbf{C}}{\mathbf{D}}_{C},&\,{\mathbf{M}}_{P}&:={\mathbf{D}}_{PE}^{T}{\mathbf{C}}_{P}{\mathbf{D}}_{PE},&{\mathbf{G}}&:={\mathbf{D}}_{PE}^{T}{\mathbf{g}}_{P}{\mathbf{D}}_{PM} \end{aligned} \end{aligned}$$

(51)

to be referred, in the order, as: the spring, inductance and piezoelectric stiffnesses; the capacitor and piezoelectric masses; the electro-mechanical gyroscopic coupling. All stiffness and mass matrices are squared and symmetric; the gyroscopic matrix is generally non-squared and, even when it is squared, is generally non-symmetric. Since, usually, $\left\| {\mathbf{K}}_{P}\right\| \ll \left\| {\mathbf{K}}_{S}\right\|$, the piezoelectric contribution to the system stiffness is neglected. Note that the capacitor potential formally appears as (changed of sign) kinetic energy, since it depends on ${\dot{\mathbf{Y}}}$.

By collecting the previous contributions, and differently grouping them, we have:

$$\begin{aligned} \begin{aligned}&T=\frac{1}{2}{\dot{\mathbf{X}}}^{T}{\mathbf{M}}_{M}{\dot{\mathbf{X}}}+\frac{1}{2}{\dot{\mathbf{Y}}}^{T}\left( {\mathbf{M}}_{C}+{\mathbf{M}}_{P}\right) {\dot{\mathbf{Y}}}\\&U=\frac{1}{2}{\mathbf{X}}^{T}\left( {\mathbf{K}}_{S}+{\mathbf{K}}_{P}\right) {\mathbf{X}}+\frac{1}{2}{\mathbf{Y}}^{T}{\mathbf{K}}_{L}{\mathbf{Y}}-{\dot{\mathbf{Y}}}^{T}{\mathbf{G}}{\mathbf{X}} \end{aligned} \end{aligned}$$

(52)

or:

$$\begin{aligned} \begin{aligned}&T-U&= \;\;\frac{1}{2}{\dot{\mathbf{X}}}^{T}{\mathbf{M}}_{m}{\dot{\mathbf{X}}}+\frac{1}{2}{\dot{\mathbf{Y}}}^{T}{\mathbf{M}}_{e}{\dot{\mathbf{Y}}}-\frac{1}{2}{\mathbf{X}}^{T}{\mathbf{K}}_{m}{\mathbf{X}}\\&&\;\; -\frac{1}{2}{\mathbf{Y}}^{T}{\mathbf{K}}_{e}{\mathbf{Y}}+{\dot{\mathbf{Y}}}^{T}{\mathbf{G}}{\mathbf{X}} \end{aligned} \end{aligned}$$

(53)

where we defined:

$$\begin{aligned} \begin{aligned} {\mathbf{M}}_{m}&:={\mathbf{M}}_{M},&\,{\mathbf{M}}_{e}&:={\mathbf{M}}_{C}+{\mathbf{M}}_{P}\\ {\mathbf{K}}_{m}&:={\mathbf{K}}_{S}+{\mathbf{K}}_{P},&\,{\mathbf{K}}_{e}&:={\mathbf{K}}_{L} \end{aligned} \end{aligned}$$

(54)

as the mechanical (m) and the electrical (e) mass and stiffness matrices, respectively. Usually, since piezoelectric devices already behave as capacitors, no further capacitors are needed in the circuit, so that ${\mathbf{M}}_{C}={\mathbf{0}}$.

Concerning nonconservative actions, they consist of: (a) the virtual work of the damping forces ${\mathbf{f}}_{D}$ over the elongation-rate $\delta \left( {\mathbf{\varvec{\Delta}}}\varvec{l}_{D}\right)$ of the dashpots; (b) the complementary virtual work expended by the charge-rates at resistors ${\dot{\mathbf{Q}}}_{R}$ over the virtual variation of the flux linkage differences $\delta \left( {\mathbf{\varvec{\Delta}}}\varvec{\psi }{}_{R}\right)$ of the resistors; (c) the virtual work expended by the external forces acting on the structure, ${\mathbf{f}}_{nc}:=-{\mathbf{H}}{\mathbf{X}}$, here assumed to be linear and homogeneous in the displacements (circulatory forces, with ${\mathbf{H}}$ the circulatory matrix, [2]), over the virtual displacements $\delta {\mathbf{X}}$. They, respectively, read:

$$\begin{aligned} \begin{aligned}\delta W_{D}&=-{\mathbf{f}}_{D}^{T}\delta \left( {\mathbf{\varvec{\Delta}}}\varvec{l}_{D}\right) =-\delta {\mathbf{X}}^{T}{\mathbf{D}}_{D}^{T}{\mathbf{b}}{\mathbf{D}}_{D}\dot{\mathbf{X}}=-\delta {\mathbf{X}}^{T}{\mathbf{B}}_{m}{\dot{\mathbf{X}}}\\ \delta W_{R}&=-{\dot{\mathbf{Q}}}_{R}\delta \left( {\mathbf{\varvec{\Delta}}}\varvec{\psi }{}_{R}\right) =-\delta {\mathbf{Y}}^{T}{\mathbf{D}}_{R}^{T}{\mathbf{\mathbf{R}}}^{-1}{\mathbf{D}}_{R}\dot{\mathbf{Y}}=-\delta {\mathbf{Y}}^{T}{\mathbf{B}}_{e}{\dot{\mathbf{Y}}}\\ \delta W_{nc}&=-{\mathbf{f}}_{nc}^{T}\delta {\mathbf{X}}=-\delta {\mathbf{X}}^{T}{\mathbf{H}}{\mathbf{X}} \end{aligned} \end{aligned}$$

(55)

where:

$$\begin{aligned} {\mathbf{B}}_{m}:={\mathbf{D}}_{D}^{T}{\mathbf{b}}{\mathbf{D}}_{D},\qquad {\mathbf{B}}_{e}:={\mathbf{D}}_{R}^{T}{\mathbf{R}}^{-1}{\mathbf{D}}_{R} \end{aligned}$$

(56)

are the mechanical and electrical damping matrices, respectively.

By substituting Eq. (53) and (55) in the Principle (47), this latter reads $\delta H_m+\delta H_e+\delta H_{em}=0$, $\forall \,\delta {\mathbf{X}},\,\delta {\mathbf{Y}}$, where:

$$\begin{aligned} \begin{aligned}&\delta H_{m}\ \; := \int _{t_{1}}^{t_{2}}\left( \delta \dot{\mathbf{X}}^{T}{\mathbf{M}}_{m}\dot{\mathbf{X}}-{\mathbf{\delta X}}^{T}{\mathbf{K}}_{m}{\mathbf{X}}-\delta {\mathbf{X}}^{T}{\mathbf{B}}_{m}\dot{\mathbf{X}}\right) {\rm d}t\\& \; \quad -\int _{t_{1}}^{t_{2}}{\mathbf{\delta X}}^{T}{\mathbf{H}}{\mathbf{X}}{\rm d}t\\&\delta H_{e} := \; \int _{t_{1}}^{t_{2}}\left( \delta \dot{\mathbf{Y}}^{T}{\mathbf{M}}_{e}\dot{\mathbf{Y}}-{\mathbf{\delta Y}}^{T}{\mathbf{K}}_{e}{\mathbf{Y}}-{\mathbf{\delta Y}}^{T}{\mathbf{B}}_{e}\dot{\mathbf{Y}}\right) {\rm d}t\\&\delta H_{em} := \;\int _{t_{1}}^{t_{2}}\left( \delta {\mathbf{X}}^{T}{\mathbf{G}}^{T}{\dot{\mathbf{Y}}}+{\delta \dot{\mathbf{Y}}}{}^{T}{\mathbf{G}}{\mathbf{X}}\right) {\rm d}t \end{aligned} \end{aligned}$$

(57)

After integration by parts, the Eq. (1) is finally obtained.