A new class of interdependent shape polynomials for the FE dynamic analysis of Mindlin plate Timoshenko beam

Abstract

This paper proposes a new class of shape polynomials, able of solving the well known shear-locking phenomena and the high-order time derivative effect problems which can suffer the Mindlin plate model. These polynomials eliminate the inconsistency of the higher-order spectra because are built on the consistent version of the governing equations previously proposed by Elishakoff. Using these equations, the new class of interdependent shape polynomials is obtained by introducing a new kinematic variable: the fictitious deflection. The interdependence of the polynomials ensures that the corresponding finite element model is free of locking. Lastly, the interdependent shape polynomials for the Timoshenko beam model are derived as a special case.

Appendix

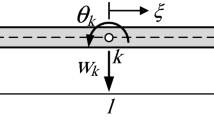
A n-nodes FE is taken into account and it is supposed that the vector ${\bar{\mathbf{u}}}$ collects the nodal values of $\bar{U}$, of $- \partial \bar{U}/\partial x$ and of $- \partial \bar{U}/\partial y$. Likewise, the vector u collects the nodal values of U, $\varPhi_{x}$ and $\varPhi_{y}$. If the jth, the j + 1th and the j + 2th element of ${\bar{\mathbf{u}}}$, ($\bar{u}_{j}$, $\bar{u}_{j + 1}$ and $\bar{u}_{j + 2}$), are the nodal values of $\bar{U}$, $- \partial \bar{U}/\partial x$ and $- \partial \bar{U}/\partial y$ at the node of coordinates $\left( {x_{j} ,y_{j} } \right)$, using the relationships given in Eqs. (20) and the approximation for $\bar{U}$ given in Eq. (21a), the following expressions are obtained:

$$ \begin{aligned} u_{j} & = \bar{u}_{j} + \left[ { - \left( {\frac{{r_{I} }}{{r_{A} }} + \frac{D}{S}} \right)\left. {\nabla^{2} {\varvec{\uppsi}}^{T} } \right|_{\begin{subarray}{l} x = x_{j} \\ y = y_{j} \end{subarray} } } \right]{\bar{\mathbf{u}}}; \quad u_{j + 1} = \bar{u}_{j + 1} + \left[ {\frac{{r_{I} }}{{r_{A} }}\left. {\frac{{\partial \nabla^{2} {\varvec{\uppsi}}^{T} }}{\partial x}} \right|_{\begin{subarray}{l} x = x_{j} \\ y = y_{j} \end{subarray} } } \right]{\bar{\mathbf{u}}}; \hfill \\ u_{j + 2} &= \bar{u}_{j + 2} + \left[ {\frac{{r_{I} }}{{r_{A} }}\left. {\frac{{\partial \nabla^{2} {\varvec{\uppsi}}^{T} }}{\partial y}} \right|_{\begin{subarray}{l} x = x_{j} \\ y = y_{j} \end{subarray} } } \right]{\bar{\mathbf{u}}} \hfill \\ \end{aligned} $$

(40a-c)

where u _j , u _j+1 and u _j+2 are the nodal values of U, $\varPhi_{x}$ and $\varPhi_{y}$ at the node of coordinates $\left( {x_{j} ,y_{j} } \right)$. Applying the above equations for all the n nodes of the element, that is for $j = 1,2, \ldots n$, it is possible to write the following compact equation:

$$ {\mathbf{u}} = \left( {{\mathbf{I}} + {\mathbf{C}}} \right){\bar{\mathbf{u}}} $$

(41)

where C is a constant square matrix of order 3n whose kth row is equal to:

• (a) the function vector $- \left( {r_{I} /r_{A} + D/S} \right)\nabla^{2} {\varvec{\uppsi}}^{T}$ evaluated at the corresponding nodal coordinates, if the u _k element is a deflection;

• (b) the function vector $- r_{I} /r_{A} \partial \nabla^{2} {\varvec{\uppsi}}^{T} /\partial x$ evaluated at the corresponding nodal coordinates, if the u _k element is a rotation $\varPhi_{x}$;

• (c) the function vector $- r_{I} /r_{A} \partial \nabla^{2} {\varvec{\uppsi}}^{T} /\partial y$ evaluated at the corresponding nodal coordinates, if the u _k element is a rotation $\varPhi_{y} .$

Equations (23) in the text follow immediately from Eq. (41).