Skip to main content
SpringerLink
Log in

Vibration control of helicopter blade flapping via time-delay absorber

  • Published:
Meccanica Aims and scope Submit manuscript

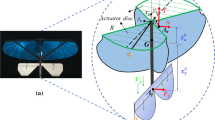
Abstract

In this study, the controller is used to suppress the vibration due to rotor the helicopter blade flapping motion. The objective of this paper is to investigate the effect of time-delay absorber on the vibrating system when subjected to multi-parametric excitation forces. The equations of motion are described by coupled nonlinear differential equations. The averaging method is applied to obtain the frequency response equations near simultaneous sub-harmonic and internal resonance. The stability of the obtained nonlinear solution is studied and solved numerically. Numerical simulations show the steady state response amplitude versus the detuning parameter and the effects of the parameters system and controller. Effectiveness of the absorber E a is about 2.7×105 of the main system (X).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  1. Sun Z, Xu W, Yang X, Fang T (2007) Effects of time delays on bifurcation and chaos in a non-autonomous system with multiple time delays. Chaos Solitons Fractals 31:39–53

    Article  ADS  MATH  MathSciNet  Google Scholar 

  2. Yaman M (2009) Direct and parametric excitation of a nonlinear cantilever beam of varying orientation with time delay state feedback. J Sound Vib 324:892–920

    Article  ADS  Google Scholar 

  3. Maccari A (2001) The response of a parametrically excited van der Pol oscillator to a time delay state feedback. Nonlinear Dyn 26:105–119

    Article  MATH  MathSciNet  Google Scholar 

  4. Maccari A (2006) Vibration control for parametrically excited Lienard systems. Int J Non-Linear Mech 41:146–155

    Article  MATH  MathSciNet  Google Scholar 

  5. Maccari A (2008) Vibration amplitude control for a van der Pol-Duffing oscillator with time delay. J Sound Vib 317:20–29

    Article  ADS  Google Scholar 

  6. Nbendjo NBR, Tchoukuegno R, Woafo P (2003) Active control with delay of vibration and chaos in a double well Duffing oscillator. Chaos Solitons Fractals 18:345–353

    Article  ADS  MATH  Google Scholar 

  7. Nbendjo NBR, Woafo P (2009) Modeling and optimal active control with delay of the dynamics of a strongly nonlinear beam. J Adv Res Dynam Control Syst 57–74

  8. Naik RD, Singru PM (2011) Resonance stability and chaotic vibration of a quarter-car vehicle model with time-delay feedback. Commun Nonlinear Sci Numer Simul 16:3397–3410

    Article  ADS  MATH  Google Scholar 

  9. Wang H, Liu J (2005) Stability and bifurcation analysis in a magnetic bearing system with time delays. Chaos Solitons Fractals 26:813–825

    Article  ADS  MATH  MathSciNet  Google Scholar 

  10. Sayed M, Kamel M (2011) Stability study and control of helicopter blade flapping vibrations. Appl Math Model 35:2820–2837

    Article  MATH  MathSciNet  Google Scholar 

  11. El-Gohary HA, El-Ganaini WAA (2011) Vibration suppression via time-delay absorber described by non-linear differential equations. Adv Theor Appl Mech 4(2):49–67

    Google Scholar 

  12. El-Gohary HA, El-Ganaini WAA (2012) Vibration suppression of a dynamical system to multi-parametric excitations via time-delay absorber. Appl Math Model 36:35–45

    Article  MATH  MathSciNet  Google Scholar 

  13. Ji JC, Leung AYT (2002) Resonance of a nonlinear SDOF system with two time-delays on linear feedback control. J Sound Vib 253:985–1000

    Article  ADS  MATH  MathSciNet  Google Scholar 

  14. Ji JC, Leung AYT (2002) Bifurcation control of parametrically excited Duffing system. Nonlinear Dyn 27:411–417

    Article  MATH  MathSciNet  Google Scholar 

  15. Fofana MS, Ryba PB (2004) Parametric stability of non-linear time delay equations. Int J Non-Linear Mech 39:79–91

    Article  MATH  MathSciNet  Google Scholar 

  16. Oueini SS, Chin CM, Nayfeh AH (2000) Response of two quadratic coupled oscillators to a principal parametric excitation. J Vib Control 6:1115–1133

    Article  Google Scholar 

  17. Oueini SS, Nayfeh AH (2000) Analysis and application of a nonlinear vibration absorber. J Vib Control 6:999–1016

    Article  Google Scholar 

  18. El-Bassiouny AF (2006) Fundamental and subharmonic resonances of harmonically oscillation with time delay state feedback. Shock Vib 13:65–83

    Article  Google Scholar 

  19. El-Bassiouny AF (2006) Vibration control of a cantilever beam with time delay state feedback. Z Naturforsch 61a:1–12

    Google Scholar 

  20. El-Bassiouny AF (2006) Stability and oscillation of two coupled Duffing equations with time delay state feedback. Phys Scr 75:726–735

    Article  MathSciNet  Google Scholar 

  21. El-Bassiouny AF (2005) Internal resonance of a nonlinear vibration absorber. Phys Scr 72:203–211

    Article  ADS  Google Scholar 

  22. El-Bassiouny AF (2010) Resonances of a nonlinear SDOF system with time-delay in linear feedback control. Phys Scr 81:015007

    Article  ADS  Google Scholar 

  23. Nayfeh AH (1985) Problems in perturbation. Wiley, New York

    MATH  Google Scholar 

  24. http://www.strutpatent.com/patent/d0646728/foldable-rotor-blade-for-toy-helicopter

  25. http://www.aviastar.org/helicopters_eng/bell_222.php

Download references

Acknowledgements

The authors would like to thank the reviewers for their valuable comments and suggestions for improving the quality of this paper.

Author information

Authors and Affiliations

  1. Department of Basic Sciences, Modern Academy for Engineering and Technology, Maadi, Egypt

    A. T. El-Sayed

  2. Department of Mathematics, Faculty of Science, Zagazig University, Zagazig, Egypt

    H. S. Bauomy

  3. Department of Mathematics, College of Arts and Science in Wadi Addawasir, Salman Bin Abdulaziz University, P.O. Box 54, Wadi Addawasir, 11991, Saudi Arabia

    H. S. Bauomy

Authors
  1. A. T. El-Sayed
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. H. S. Bauomy
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. T. El-Sayed.

Appendix

Appendix

$$\begin{aligned} & L_{1} = \biggl[ - \varepsilon \zeta_{1} \omega_{1} + \varepsilon \frac{F_{1}}{4\omega_{1}}\sin \gamma_{10} \biggr], \\ &L_{2} = \biggl[\varepsilon \frac{F_{1}a_{10}}{4\omega_{1}}\cos \gamma_{10}\biggr], \\ &L_{3} = \biggl[\varepsilon \frac{\beta}{2\omega_{1}}\sin \gamma_{20}\biggr], \\ &L_{4} = \biggl[\varepsilon \frac{\beta a_{20}}{2\omega_{1}}\cos \gamma_{20}\biggr], \\ & L_{5} = \biggl[ - \frac{\varepsilon \sigma_{1}}{a_{10}} - \varepsilon \frac{9\alpha_{1}a_{10}}{4\omega_{1}} + \varepsilon \frac{F_{1}}{2\omega_{1}a_{10}}\cos \gamma_{10} \biggr], \\ &L_{6} = - \biggl[\varepsilon \frac{F_{1}}{2\omega_{1}}\sin \gamma_{10}\biggr], \\ &L_{7} = \biggl[\varepsilon \frac{\beta}{\omega_{1}a_{10}}\cos \gamma_{20}\biggr], \\ &L_{8} = - \biggl[\varepsilon \frac{\beta a_{20}}{\omega_{1}a_{10}}\sin \gamma_{20}\biggr], \\ &L_{9} = - \biggl[\varepsilon \frac{\alpha_{2}}{2\omega_{2}}\{ \sin \gamma_{20}\cos \omega_{1}\tau + \cos \gamma_{20} \sin \omega_{1}\tau \} \biggr], \\ &L_{10} = - \varepsilon \zeta_{2}\omega_{2}, \\ & L_{11} = - \biggl[\varepsilon \frac{\alpha_{2}a_{10}}{2\omega_{2}}\{ \cos \gamma_{20}\cos \omega_{1}\tau - \sin \gamma_{20} \sin \omega_{1}\tau \} \biggr], \\ &L_{12} = - \biggl[\varepsilon \frac{\alpha_{2}}{2\omega_{2}a_{20}}\{ \cos \gamma_{20}\cos \omega_{1}\tau - \sin \gamma_{20} \sin \omega_{1}\tau \} \biggr] \\ & L_{13} = \biggl[\frac{\varepsilon \sigma_{2}}{a_{20}} - \frac{1}{2a_{20}}\varepsilon \sigma_{1}\biggr], \\ &L_{14} = \biggl[\varepsilon \frac{\alpha_{2}a_{10}}{2\omega_{2}a_{20}}\{ \sin \gamma_{20}\cos \omega_{1}\tau + \cos \gamma_{20} \sin \omega_{1}\tau \} \biggr] \end{aligned}$$
$$\begin{aligned} r_{1} &= ( - L_{14} - L_{1} - L_{6} - L_{10}) \\ r_{2} &= (L_{1}L_{6} + L_{1}L_{10} + L_{1}L_{14} + L_{6}L_{10} + L_{6}L_{14} \\ &\quad {} + L_{10}L_{14} - L_{11}L_{13} - L_{5}L_{2} - L_{9}L_{3} - L_{12}L_{4}) \\ r_{3} &= \bigl( - L_{6}L_{10}L_{14} + L_{1}L_{11}L_{13} - L_{1}L_{6}L_{10} \\ &\quad {} - L_{1}L_{6}L_{14} - L_{1}L_{10}L_{14} + L_{5}L_{2}L_{10} \\ &\quad {} - L_{9}L_{13}L_{4} + L_{9}L_{3}L_{14} + L_{6}L_{11}L_{13} - L_{9}L_{2}L_{7} \\ &\quad {}- L_{12}L_{2}L_{8} + L_{5}L_{2}L_{14} + L_{12}L_{6}L_{4} \\ &\quad {} + L_{9}L_{6}L_{3} + L_{12}L_{4}L_{10} - L_{12}L_{3}L_{11}\bigr) \\ r_{4} &= (L_{1}L_{6}L_{10}L_{14} - L_{9}L_{2}L_{13}L_{8} + L_{9}L_{6}L_{13}L_{4} \\ &\quad {} - L_{1}L_{6}L_{11}L_{13} - L_{5}L_{2}L_{10}L_{14} + L_{12}L_{2}L_{8}L_{10}\\ &\quad {}- L_{12}L_{6}L_{4}L_{10} - L_{12}L_{2}L_{7}L_{11} + L_{9}L_{2}L_{7}L_{14}\\ &\quad {} + L_{12}L_{6}L_{3}L_{11} + L_{5}L_{2}L_{11}L_{13} - L_{9}L_{6}L_{3}L_{14}) \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Cite this article

El-Sayed, A.T., Bauomy, H.S. Vibration control of helicopter blade flapping via time-delay absorber. Meccanica 49, 587–600 (2014). https://doi.org/10.1007/s11012-013-9813-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-013-9813-9

Keywords

Search

Navigation