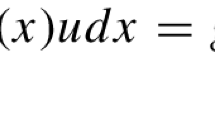We study the asymptotic behavior of the spectrum of the Dirichlet problem for a formally selfadjoint elliptic system of differential equations with rapidly oscillating coefficients and changing sign density ρ. Since the factor ρ at the spectral parameter changes sign, the problem possesses two – positive and negative – infinitely large sequences of eigenvalues. Their asymptotic structure essentially depends on whether the mean \( \overline \rho \) over the periodicity cell vanishes. In particular, in the case \( \overline \rho = 0 \), the homogenized problem becomes a quadratic pencil. Bibliography: 20 titles.
Similar content being viewed by others
References
J. Nečas, Les méthodes in théorie des équations elliptiques, Masson-Academia, Paris-Prague (1967).
S. A. Nazarov, “Selfadjoint elliptic boundary value problems. The polynomial property and formally positive operators” [in Russian], Probl. Mat. Anal. 16, 167–192 (1997); English transl.: J. Math. Sci., New York 92, No. 6, 4338–4353 (1998).
S. A. Nazarov, “The polynomial property of selfadjoint elliptic boundary value problems and the algebraic description of their attributes” [in Russian], Uspekhi Mat. Nauk 54, No. 5, 77–142 (1999); English transl.: Russ. Math. Surv. 54, No. 5, 947–1014 (1999).
O. A. Ladyzhenskaya, Boundary-Value Problems in Mathematical Physics [in Russian], Nauka, Moscow (1973).
S. G. Lekhnitskij, Theory of Elasticity of an Anisotropic Body [in Russian], Nauka, Moscow (1977); English transl.: Mir Publishers, Moscow (1981).
S. A. Nazarov, Asymptotic Theory of Thin Plates and Rods. Vol. 1. Dimension Reduction and Integral Estimates, Nauchanaya Kniga (IDMI), Novosibirck (2002).
V. T. Grinchenko, A. F. Ulitko, and N. A. Shul’ga, Mechanics of Connected Fields in Elements of Constructions [in Russian], Naukova Dumka, Kiev (1989).
V. Z. Parton and B. A. Kudryavtsev, Electric/elasticity of piezoelectric and electrically conducting bodies [in Russian], Nauka, Moscow (1988).
S. A. Nazarov, “Uniform estimates of remainders in asymptotic expansions of solutions to the problem on eigenoscillaitons of a piezoelectric plate” [in Russian], Probl. Mat. Anal. 25, 99–188 (2003); English transl.: J. Math. Sci., New York 144, No. 5, 1657–1725 (2003).
M. Sh. Birman and M. Z. Solomyak, Spectral Theory of Selfadjoint Operators in Hilbert Space [in Russian], Leningrad State University, Leningrad (1980).
S. Kesavan, “Homogenization of Elliptic Eigenvalue Problems: Part 1,” Appl. Math. Optim. 5, 153–167 (1979); Part 2: ibid. 5, 197–216 (1979).
V. V. Zhikov, S. M. Kozlov, and O. A. Oleinik, Homogenization of Differential Operators and Integral Functions [in Russian], Fizmatgiz, Moscow (1993); English transl.: Springer, Berlin etc. (1994).
M. Vanninatan, “Homogenization of eigenvalue problems in perforated domains,” Pro. Indian Acad. Sci. (Math. Sci.) 90, No. 3, 239–271 (1981).
O. A. Oleinik, A. S. Shamaev, and G. A. Yosifyan, Mathematical Problems in the Theory of Strongly Inhomogeneous Elastic Media [in Russian], Moscow State Univ., Moscow (1990).
G. Cardone, A. Corbo Esposito, and S. A. Nazarov, “Korn’s inequality for periodic solids and convergence rate of homogenization,” Appl. Anal. 88, No. 6, 847–876 (2009).
I. M. Gelfand and G. E. Shilov, Generalized Functions and Operations over Them [in Russian], Fizmatgiz, Moscow (1958).
M. I. Vishik and L. A. Lusternik, “Regular degeneration and boundary layer for linear differential equations with small parameter” [in Russian] Uspekhi Mat. Nauk 12, No. 5, 3–122 (1957).
I. C. Gohberg and M. G. Krein, Introduction to the Theory of Linear Nonself-Adjoint Operators in Hilbert Space [in Russian], Nauka, Moscow (1965); English transl.: Am. Math. Soc., Providence, RI (1969).
S. A. Nazarov, “On the asymptotics of the spectrum of a thin plate problem of elasticity” [in Russian], Sib. Mat. Zh. 41, No. 4, 895–912 (2000); English transl.: Sib. Math. J. 41, No. 4, 744–750 (2000).
V. L. Berdicevskii, “High-frequency longwave oscillations of plates” [in Russian], Dokl. Akad. Nauk SSSR 236, No. 6, 1319–1322 (1977).
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated from Problems in Mathematical Analysis 48, July 2010, pp. 75–107
Rights and permissions
About this article
Cite this article
Nazarov, S.A., Pyatnitskii, A.L. Homogenization of the spectral Dirichlet problem for a system of differential equations with rapidly oscillating coefficients and changing sign density. J Math Sci 169, 212–248 (2010). https://doi.org/10.1007/s10958-010-0047-2
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10958-010-0047-2