Should patients skip late doses of medication? A pharmacokinetic perspective

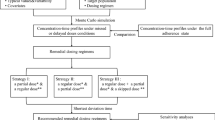
Abstract

Missed doses, late doses, and other dosing irregularities are major barriers to effective pharmacotherapy, especially for the treatment of chronic conditions. What should a patient do if they did not take their last dose at the prescribed time? Should they take it late or skip it? In this paper, we investigate the pharmacokinetic effects of taking a late dose. We consider a single compartment model with linear absorption and elimination for a patient instructed to take doses at regular time intervals. We suppose that the patient forgets to take a dose and then realizes some time later and must decide what remedial steps to take. Using mathematical analysis, we derive several metrics which quantify the effects of taking the dose late. The metrics involve the difference between the drug concentration time courses for the case that the dose is taken late and the case that the dose is taken on time. In particular, the metrics are the integral of the absolute difference over all time, the maximum of the difference, and the maximum of the integral of the difference over any single dosing interval. We apply these general mathematical formulas to levothyroxine, atorvastatin, and immediate release and extended release formulations of lamotrigine. We further show how population variability can be immediately incorporated into these results. Finally, we use this analysis to propose general principles and strategies for dealing with dosing irregularities.

Appendix

In this Appendix, we collect details of the mathematical analysis. We also present a fourth metric in Sect. 5.3 for studying the effects of a late dose.

Derivation of mathematical formulas

To calculate $\rho ^{\text {skip}}$ in (15), we note that

$$\begin{aligned} |c^{\text {skip}}(t)-c(t)| =c_{0}(t), \end{aligned}$$

(45)

where $c_{0}(t)$ is defined in (5). Integrating (45) yields (16).

To calculate $\rho _{-}$ and $\rho _{+}$ defined in (13)-(14), we first use that (10) and (8) imply that $\rho _{-}$ and $\rho _{+}$ can be written in terms of $\delta (t,d)$ in (8) as

$$\begin{aligned} \rho _{-} =\frac{-1}{\tau {\langle }c^{\text {perf}}{\rangle }}\int _{0}^{{{s_{0}}}}\delta (t,d)\,\text {d}t,\quad \rho _{+} =\frac{1}{\tau {\langle }c^{\text {perf}}{\rangle }}\int _{{{s_{0}}}}^{\infty }\delta (t,d)\,\text {d}t, \end{aligned}$$

(46)

where ${{s_{0}}}>d>0$ is such that $\delta (t,d)<0$ for all $t\in (0,{{s_{0}}})$ and $\delta (t,d)>0$ for all $t>{{s_{0}}}$. Solving for ${{s_{0}}}$ yields

$$\begin{aligned} {{s_{0}}} =\frac{1}{k_{\text {a}}-k_{\text {e}}}\ln \Big (\frac{e^{k_{\text {a}}d}-1}{e^{k_{\text {e}}d}-1}\Big )>d >0. \end{aligned}$$

(47)

Integrating (46) using (8) and (47) yields (17), which then yields (19) by (12).

To calculate $\nu$ defined in (24), we first use (10) to obtain that $\nu$ can be written in terms of $\delta$ as

$$\begin{aligned} \nu =\frac{1}{{\langle }c^{\text {perf}}{\rangle }}\max _{t\in {\mathbb {R}}}\delta (t,d). \end{aligned}$$

(48)

A simple calculus exercise yields that $\delta (t,d)$ is maximized at $t={{s_{1}}}$, where

$$\begin{aligned} {{s_{1}}} =\frac{1}{k_{\text {a}}-k_{\text {e}}}\log \Big [\frac{(e^{k_{\text {a}}d}-1)k_{\text {a}}}{(e^{k_{\text {e}}d}-1)k_{\text {e}}}\Big ]. \end{aligned}$$

(49)

Plugging (49) into (48) yields the formula for $\nu$ in (26).

To calculate $\gamma$ in (25), we first use (10) to obtain that $\gamma$ can be written in terms of $\delta$ as

$$\begin{aligned} \gamma =\frac{1}{{\langle }c^{\text {perf}}{\rangle }}\max _{t\in {\mathbb {R}}}\frac{1}{\tau }\int _{t}^{t+\tau }\delta (s,d)\,\text {d}s. \end{aligned}$$

(50)

Integrating (50) and performing a simple calculus exercise yields that the maximum in (50) occurs at $t={{s_{2}}}$, where

$$\begin{aligned} {{s_{2}}} =\frac{1}{k_{\text {a}}-k_{\text {e}}}\log \Big (\frac{(e^{k_{\text {a}}\tau }-1) (e^{k_{\text {a}}d}-1)}{(e^{k_{\text {e}}\tau }-1) (e^{k_{\text {e}}d}-1)}\Big )-\tau . \end{aligned}$$

(51)

Plugging (51) into (50) yields the formula for $\gamma$ in (27).

Monotonicity

We now prove (32) and (33). Applying the chain rule to (19) yields

$$\begin{aligned} \begin{aligned} \frac{\partial }{\partial k_{\text {a}}}{{{\rho }}}&=2f_{x}(k_{\text {a}}d,k_{\text {e}}d)d,\quad \frac{\partial }{\partial k_{\text {e}}}{{{\rho }}} =2f_{y}(k_{\text {a}}d,k_{\text {e}}d)d,\\ \frac{\partial }{\partial d}{{{\rho }}}&=2f_{x}(k_{\text {a}}d,k_{\text {e}}d)k_{\text {a}}+2f_{x}(k_{\text {a}}d,k_{\text {e}}d)k_{\text {e}}, \end{aligned} \end{aligned}$$

(52)

where $f_{x}$ and $f_{y}$ denote the partial derivatives of f in (18) with respect to x and y, respectively. Specifically,

$$\begin{aligned} f_{x}(x,y) =\frac{y (\frac{e^y-1}{e^x-1})^{\frac{x}{x-y}} \left( e^x (y-x)-\left( e^x-1\right) \log \left( e^y-1\right) +\left( e^x-1\right) \log \left( e^x-1\right) \right) }{(x-y)^2}, \end{aligned}$$

(53)

and the formula for $f_{y}(x,y)$ is obtained from (53) upon swapping x and y. Though (53) is a complicated expression, it is easy to plot as a function of $x>0$ and $y>0$ to obtain that

$$\begin{aligned} f_{x}(x,y)>0,\quad f_{y}(x,y)>0. \end{aligned}$$

(54)

Hence, (54) and the expressions in (52) yield (32).

The sign of the partial derivatives of $\gamma$ in (33) follow immediately from (27), the chain rule, and (54).

To obtain the sign of the partial derivatives of $\nu$ in (33), we first note that the partial derivative of g in (28) with respect to x is

$$\begin{aligned} g_{x}(x,y) =\Big (\frac{y (x \log (x)+y-x \log (y)-x)}{(x-y)^2}\Big )\left( \frac{y}{x}\right) ^{\frac{x}{x-y}}, \end{aligned}$$

(55)

and the formula for $g_{y}(x,y)$ is obtained from (55) upon swapping x and y. Though (55) is a complicated expression, it is easy to plot as a function of $x>0$ and $y>0$ to obtain that

$$\begin{aligned} g_{x}(x,y)>0,\quad g_{y}(x,y)>0. \end{aligned}$$

(56)

Hence, the sign of the partial derivatives of $\nu$ in (33) follow immediately from using (26), the chain rule, and (54) and (56).

Maximum concentration

In the main text of the paper, the metric $\nu$ measures the maximum amount that $c^{d}(t)$ can rise above c(t). To obtain the value for the maximum of $c^{d}(t)$ rather than how the $c^{d}(t)$ rises above c(t), we must make assumptions about the patient’s adherence before and after the late dose. For simplicity, we assume that the patient has perfect adherence for a long time before and after the late dose. Letting $\{c^{\text {perf},d}(t)\}_{t\in {\mathbb {R}}}$ denote this concentration time course, we prove below that

$$\begin{aligned} \max _{t\in {\mathbb {R}}}c^{\text {perf},d}(t) =\max _{j\in \{j^{*},j^{*}+1\}}\big (\delta (j\tau +t_{j},d)+c^{\text {perf}}(t_{j})\big ), \end{aligned}$$

(57)

where $\delta$ is defined in (8) and

$$\begin{aligned} j^{*}&={\Big \lfloor {\frac{1}{k_{\text {a}}\tau -k_{\text {e}}\tau }\log \Big (\frac{(e^{k_{\text {a}}\tau }-1) (e^{k_{\text {a}}d}-1)}{(e^{k_{\text {e}}\tau }-1) (e^{k_{\text {e}}d}-1)}\Big )-1}\Big \rfloor }, \end{aligned}$$

(58)

$$\begin{aligned} t_{j}&=\frac{1}{k_{\text {a}}-k_{\text {e}}}\log \Big (\frac{k_{\text {a}}(e^{k_{\text {e}}\tau }-1) [(e^{k_{\text {a}}\tau }-1) (e^{k_{\text {a}}d}-1)+e^{(j +1)k_{\text {a}}\tau }] e^{j (k_{\text {e}}-k_{\text {a}})\tau }}{k_{\text {e}}(e^{k_{\text {a}}\tau }-1) [(e^{k_{\text {e}}\tau }-1) (e^{k_{\text {e}}d}-1)+e^{(j+1) k_{\text {e}}\tau }]}\Big ). \end{aligned}$$

(59)

In (58), we use the floor function notation, in which ${\lfloor {x}\rfloor }$ denotes the largest integer less than or equal to x.

While the complicated formulas in (57)-(59) do not offer much intuition, they can be easily plotted to investigate how the maximum concentration depends on the various parameters. In Fig. 8, we plot

$$\begin{aligned} \theta :=\frac{\max _{t\in {\mathbb {R}}}c^{\text {perf},d}(t)-{\langle }c^{\text {perf}}{\rangle }}{{\langle }c^{\text {perf}}{\rangle }}, \end{aligned}$$

(60)

which is a dimensionless measure of how far $c^{\text {perf},d}(t)$ rises above ${\langle }c^{\text {perf}}{\rangle }$, relative to ${\langle }c^{\text {perf}}{\rangle }$. The top left panel in Fig. 8 is for no delay ($d=0$) to show how the concentration time courses rises above the average ${\langle }c^{\text {perf}}{\rangle }$ for perfect adherence. In this case of no delay, $\theta$ has a simpler formula which we denote by $\theta ^{\text {perf}}$,

$$\begin{aligned} \theta ^{\text {perf}} =&\lim _{d\rightarrow 0}\theta =\frac{k_{\text {a}}k_{\text {e}}\tau }{k_{\text {a}}-k_{\text {e}}} \Bigg (\frac{\left( \frac{k_{\text {e}}(e^{k_{\text {a}}\tau }-1)}{k_{\text {a}}(e^{k_{\text {e}}\tau }-1)}\right) ^{\frac{k_{\text {e}}}{k_{\text {a}}-k_{\text {e}}}}}{e^{k_{\text {e}}\tau }-1}\nonumber \\&-\frac{\left( \frac{k_{\text {e}}(e^{k_{\text {a}}\tau }-1)}{k_{\text {a}}(e^{k_{\text {e}}\tau }-1)}\right) ^{\frac{k_{\text {a}}}{k_{\text {a}}-k_{\text {e}}}}}{e^{k_{\text {a}}\tau }-1}\Bigg )-1. \end{aligned}$$

(61)

The bottom right panel shows that even in the extreme case of a double dose ($d=\tau$), the drug concentration rises only slightly above the average if the absorption and/or elimination rate is sufficiently slow compared to $1/\tau$. Conversely, this plot shows that the drug concentration can rise far above the average if both the absorption and elimination rate is sufficiently fast compared to $1/\tau$. Analogous to Figs. 2 and 4, the letter markers in Fig. 8 are for the drugs in Table 1.

Fig. 8

Maximum relative increase in concentration $\theta$ in (60) for different values of the delay $d\in [0,\tau ]$. Note that the top left panel is for $d=0$ (see (61)). The letters L, A, I and X correspond to different drugs. See the text for details

To obtain the formula for $\max _{t\in {\mathbb {R}}}c^{\text {perf},d}(t)$ in (57), we first note that

$$\begin{aligned} c^{\text {perf},d}(t) =c^{\text {perf}}(t)+c^{\text {perf},d}(t)-c^{\text {perf}}(t) =c^{\text {perf}}(t)+\delta (t,d), \end{aligned}$$

(62)

where $c^{\text {perf}}(t)$ is defined in (6). Now, $\delta (t,d)$ is strictly increasing for $t\in ({{s_{0}}},{{s_{1}}})$ and strictly decreasing for $t\in ({{s_{1}}},\infty )$. It therefore follows that ${{s_{2}}}\in ({{s_{0}}},{{s_{1}}})$ is such that $\delta ({{t_{1}}},d)>\delta (t_{0},d)$ if ${{t_{1}}}\in ({{s_{2}}},{{s_{2}}}+\tau )$ and $t_{0}\notin ({{s_{2}}},{{s_{2}}}+\tau )$. Since $c^{\text {perf}}(t)$ is periodic with period $\tau$, it follows from (62) that the maximum of $c^{\text {perf},d}(t)$ must occur at some $t\in [{{s_{2}}},{{s_{2}}}+\tau ]$. Now, since $j^{*}$ in (58) satisfies $j^{*}={\lfloor {{{s_{2}}}/\tau }\rfloor }$, it follows that the maximum of $c^{\text {perf},d}(t)$ must occur at some $t\in [j^{*}\tau ,(j^{*}+2)\tau ]$ since $[{{s_{2}}},{{s_{2}}}+\tau ]\subset [j^{*}\tau ,(j^{*}+2)\tau ]$. If $t=j\tau +s$ for some fixed integer $j\in {\mathbb {Z}}$ and some time $s\in [0,\tau ]$, then it follows immediately from (6) that

$$\begin{aligned} c^{\text {perf}}(j\tau +s)&=\frac{DF}{V}\frac{k_{\text {a}}}{k_{\text {a}}-k_{\text {e}}}\nonumber \\&\qquad \big [e^{-k_{\text {e}}s}/(1-e^{-k_{\text {e}}\tau })-e^{-k_{\text {a}}s}/(1-e^{-k_{\text {a}}\tau })\big ]. \end{aligned}$$

(63)

Hence, (62) implies $c^{\text {perf},d}(j\tau +s)=C_{j}^{d}(s)$ where $C_{j}^{d}(s)$ is defined to be

$$\begin{aligned} C_{j}^{d}(s)&:=\frac{DF}{V}\frac{k_{\text {a}}}{k_{\text {a}}-k_{\text {e}}}\nonumber \\&\quad \big [e^{-k_{\text {e}}s}/(1-e^{-k_{\text {e}}\tau })-e^{-k_{\text {a}}s}/(1-e^{-k_{\text {a}}\tau })\big ]\nonumber \\&\qquad +\delta (j\tau +s,d). \end{aligned}$$

(64)

Differentiating (64) with respect to s shows that $\frac{\text {d}}{\text {d}s}C_{j}^{d}(s)=0$ if $s=t_{j}$ where $t_{j}$ is defined in (59). Hence, we have obtained (57).

To obtain $\max _{t\in {\mathbb {R}}}c^{\text {perf}}(t)$, we merely differentiate (63) with respect to s to find the maximum. Plugging the resulting expression into the definition of $\theta$ in (60) yields the formula for $\theta ^{\text {perf}}$ in (61).