Skip to main content
SpringerLink
Log in

Adapted numerical modelling of the Belousov–Zhabotinsky reaction

  • Original Paper
  • Published:
Journal of Mathematical Chemistry Aims and scope Submit manuscript

Abstract

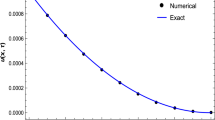
Adapted numerical schemes for the integration of differential equations generating periodic wavefronts have reported benefits in terms of accuracy and stability. This work is focused on differential equations modelling chemical phenomena which are characterized by an oscillatory dynamics. The adaptation is carried out through the exponential fitting technique, which is specially suitable to follow the apriori known qualitative behavior of the solution. In particular, we have merged this strategy with the information coming from existing theoretical studies and especially the observation of time series. Numerical tests will be provided to show the effectiveness of this problem-oriented approach.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. P. Albrecht, A new theoretical approach to RK methods. SIAM J. Numer. Anal. 24(2), 391–406 (1987)

    Article  Google Scholar 

  2. B.P. Belousov, An oscillating reaction and its mechanism, Sborn. referat. radiat. med. (Collection of abstracts on radiation medicine), Medgiz (1959)

  3. M.A. Budroni, F. Rossi, A novel mechanism for in situ nucleation of spirals controlled by the interplay between phase fronts and reaction–diffusion waves in an oscillatory medium. J. Phys. Chem. C 119(17), 9411–9417 (2015)

    Article  CAS  Google Scholar 

  4. A. Cardone, LGr Ixaru, B. Paternoster, Exponential fitting direct quadrature methods for Volterra integral equations. Numer. Algorithms 55(4), 467–480 (2010)

    Article  Google Scholar 

  5. R. D’Ambrosio, E. Esposito, B. Paternoster, Exponentially fitted two-step hybrid methods for \(y^{\prime \prime }=f(x, y)\). J. Comput. Appl. Math. 235(16), 4888–4897 (2011)

    Article  Google Scholar 

  6. R. D’Ambrosio, E. Esposito, B. Paternoster, Exponentially fitted two-step Runge–Kutta methods: construction and parameter selection. Appl. Math. Comput. 218(14), 7468–7480 (2012)

    Google Scholar 

  7. R. D’Ambrosio, E. Esposito, B. Paternoster, Parameter estimation in exponentially fitted hybrid methods for second order differential problems. J. Math. Chem. 50(1), 155–168 (2012)

    Article  CAS  Google Scholar 

  8. R. D’Ambrosio, M. Moccaldi, B. Paternoster, Adapted numerical methods for advection-reaction-diffusion problems generating periodic wavefronts. Comput. Math. Appl. 74(5), 1029–1042 (2017)

    Article  Google Scholar 

  9. R. D’Ambrosio, M. Moccaldi, B. Paternoster, F. Rossi, On the employ of time series in the numerical treatment of differential equations modeling oscillatory phenomena. Commun. Comput. Inf. Sci. 708, 179–187 (2017)

    Google Scholar 

  10. R. D’Ambrosio, M. Moccaldi, B. Paternoster, F. Rossi, Stochastic Numerical Models of Oscillatory Phenomena, in Artificial Life and Evolutionary Computation, Wivace 2017 Workshop, Venice, 19-21 September 2017, Springer (2018)

  11. R. D’Ambrosio, B. Paternoster, Numerical solution of a diffusion problem by exponentially fitted finite difference methods, SpringerPlus 3(1), https://doi.org/10.1186/2193-1801-3-425 (2014)

    Article  PubMed  PubMed Central  Google Scholar 

  12. R. D’Ambrosio, B. Paternoster, Numerical solution of reaction-diffusion systems of \(\lambda \)–\(\omega \) type by trigonometrically fitted methods. J. Comput. Appl. Math. 294(C), 436–445 (2016)

    Article  Google Scholar 

  13. R. D’Ambrosio, B. Paternoster, G. Santomauro, Revised exponentially fitted Runge–Kutta–Nyström methods. Appl. Math. Lett. 30, 56–60 (2014)

    Article  Google Scholar 

  14. I.R. Epstein, J.A. Pojman, An Introduction to Nonlinear Chemical Dynamics: Oscillations, Waves, Patterns, and Chaos (Oxford University Press, New York, 1998)

    Google Scholar 

  15. I.R. Epstein, B. Xu, Reaction-diffusion processes at the nano- and microscales. Nat. Nanotechnol. 11(4), 312–319 (2016)

    Article  CAS  PubMed  Google Scholar 

  16. R.J. Field, M. Burger, Oscillations and Traveling Waves in Chemical Systems (Wiley, New York, 1985)

    Google Scholar 

  17. R,J. Field, E. Körös, R.M. Noyes, Oscillations in chemical systems. II. Thorough analysis of temporal oscillation in bromate-cerium-malonic acid system. J. Am. Chem. Soc. 94, 8649–8664 (1972)

    Article  CAS  Google Scholar 

  18. R.J. Field, R.M. Noyes, Oscillations in chemical systems. IV. Limit cycle behaviour in a model of a real chemical reaction. J. Chem. Phys. 60, 1877–1884 (1974)

    Article  CAS  Google Scholar 

  19. W. Gautschi, Numerical integration of ordinary differential equations based on trigonometric polynomials. Numer. Math. 3, 381–397 (1961)

    Article  Google Scholar 

  20. LGr Ixaru, B. Paternoster, A conditionally P-stable fourth-order exponential-fitting method for \(y^{\prime \prime }=f(x, y)\). J. Comput. Appl. Math. 106(1), 87–98 (1999)

    Article  Google Scholar 

  21. LGr Ixaru, G. Vanden Berghe, Exponential Fitting (Kluwer, Dordrecht, 2004)

    Book  Google Scholar 

  22. N. Marchettini, M.A. Budroni, F. Rossi, M. Masia, M.L.T. Liveri, M. Rustici, Role of the reagents consumption in the chaotic dynamics of the Belousov–Zhabotinsky oscillator in closed unstirred reactors. Phys. Chem. Chem. Phys. 12(36), 11062–11069 (2010)

    Article  CAS  PubMed  Google Scholar 

  23. J.D. Murray, Mathematical Biology (Springer, New York, 2004)

    Book  Google Scholar 

  24. B. Paternoster, Runge–Kutta(-Nyström) methods for ODEs with periodic solutions based on trigonometric polynomials. Appl. Numer. Math. 28(2), 401–412 (1998)

    Article  Google Scholar 

  25. B. Paternoster, Present state-of-the-art in exponential fitting. A contribution dedicated to Liviu Ixaru on his 70th birthday. Comput. Phys. Commun. 183, 2499–2512 (2012)

    Article  CAS  Google Scholar 

  26. F. Rossi, M.A. Budroni, N. Marchettini, J. Carballido-Landeira, Segmented waves in a reaction–diffusion–convection system, Chaos: an interdisciplinary. J. Nonlinear Sci. 22(3), 037109 (2012)

    Google Scholar 

  27. F. Rossi, M.A. Budroni, N. Marchettini, L. Cutietta, M. Rustici, M.L.T. Liveri, Chaotic dynamics in an unstirred ferroin catalyzed Belousov–Zhabotinsky reaction. Chem. Phys. Lett. 480, 322–326 (2009)

    Article  CAS  Google Scholar 

  28. F. Rossi, S. Ristori, M. Rustici, N. Marchettini, E. Tiezzi, Dynamics of pattern formation in biomimetic systems. J. Theor. Biol. 255(4), 404–412 (2008)

    Article  CAS  PubMed  Google Scholar 

  29. S.K. Scott, Oscillations, Waves and Chaos in Chemical Kinetics (Oxford University Press, Oxford, 1994)

    Google Scholar 

  30. T.P. Souza, J. Perez-Mercader, Entrapment in giant polymersomes of an inorganic oscillatory chemical reaction and resulting chemo-mechanical coupling. Chem. Commun. 50(64), 8970–8973 (2014)

    Article  Google Scholar 

  31. R. Tamate, T. Ueki, M. Shibayama, R. Yoshida, Self-oscillating vesicles: spontaneous cyclic structural change of synthetic diblock copolymers. Angew. Chem. Int. Ed. 53(42), 11248–11252 (2014)

    Article  CAS  Google Scholar 

  32. A.F. Taylor, Mechanism and phenomenology of an oscillating chemical reaction. Prog. React. Kinet. Mech. 27(4), 247–325 (2002)

    Article  CAS  Google Scholar 

  33. K. Torbensen, F. Rossi, O.L. Pantani, S. Ristori, A. Abou-Hassan, Interaction of the Belousov–Zhabotinsky reaction with phospholipid engineered membranes. J. Phys. Chem. B 119(32), 10224–10230 (2015)

    Article  CAS  PubMed  Google Scholar 

  34. K. Torbensen, F. Rossi, S. Ristori, A. Abou-Hassan, Chemical communication and dynamics of droplet emulsions in networks of Belousov–Zhabotinsky micro-oscillators produced by microfluidics. Lab Chip 17(7), 1179–1189 (2017)

    Article  CAS  PubMed  Google Scholar 

  35. K. Torbensen, S. Ristori, F. Rossi, A. Abou-Hassan, Tuning the chemical communication of oscillating microdroplets by means of membrane composition. J. Phys. Chem. C 121(24), 13256–13264 (2017)

    Article  CAS  Google Scholar 

  36. J.J. Tyson, A Quantitative Account of Oscillations, Bistability, and Traveling Waves in the Belousov–Zhabotinskii Reaction, Oscillations and Traveling Waves in Chemical Systems (Wiley, New York, 1985), pp. 93–144

    Google Scholar 

  37. J.J. Tyson, What everyone should know about the Belousov–Zhabotinsky reaction. Lect. Notes Biomath. 100, 569–587 (1994)

    Article  Google Scholar 

  38. A.N. Zaikin, A.M. Zhabotinsky, Concentration wave propagation in two-dimensional liquid-phase self-oscillating system. Nature 225(5232), 535–537 (1970)

    Article  CAS  PubMed  Google Scholar 

  39. A.M. Zhabotinsky, Periodic processes of the oxidation of malonic acid in solution (study of the kinetics of Belousov’s reaction). Biofizika 9, 306–311 (1964)

    Google Scholar 

  40. A.M. Zhabotinsky, F. Rossi, A brief tale on how chemical oscillations became popular: an interview with anatol Zhabotinsky. Int. J. Des. Nat. Ecodyn. 1(4), 323–326 (2006)

    Article  Google Scholar 

Download references

Acknowledgements

Raffaele D’Ambrosio, Martina Moccaldi and Beatrice Paternoster are members of the INdAM Research group GNCS. The work is supported by GNCS-Indam project.

Author information

Authors and Affiliations

  1. Department of Engineering and Computer Science and Mathematics, University of L’Aquila, L’Aquila, Italy

    Raffaele D’Ambrosio

  2. Department of Mathematics, University of Salerno, Fisciano, SA, Italy

    Martina Moccaldi & Beatrice Paternoster

  3. Department of Chemistry and Biology, University of Salerno, Fisciano, SA, Italy

    Federico Rossi

Authors
  1. Raffaele D’Ambrosio
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Martina Moccaldi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Beatrice Paternoster
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Federico Rossi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Raffaele D’Ambrosio.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

D’Ambrosio, R., Moccaldi, M., Paternoster, B. et al. Adapted numerical modelling of the Belousov–Zhabotinsky reaction. J Math Chem 56, 2876–2897 (2018). https://doi.org/10.1007/s10910-018-0922-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10910-018-0922-5

Keywords

Search

Navigation