Bogdanov–Takens and Triple Zero Bifurcations for a Neutral Functional Differential Equations with Multiple Delays

Abstract

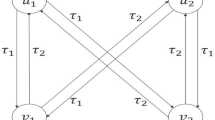
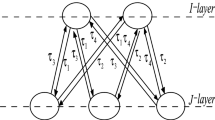
In this paper, a neutral functional differential equation with multiple delays is considered. In a first step, we assumed some sufficient hypotheses to guarantee the existence of the Bogdanov–Takens and the triple-zero bifurcations. In a second step, the normal form of the two bifurcations is obtained by using the reduction on the center manifold and the theory of the normal form. Finally, we applied our study to a class of three-neuron bidirectional associative memory networks, its dynamic behaviors are studied and proved by an example and its numerical simulations.

A Appendix

In this part, we will define the notations:

$$\begin{aligned} H_{1}= & {} \left( A_{1}+\displaystyle \sum _{i=1}^{q}B_{i1}\right) \varphi _{1}^{0}, \\ H_{2}= & {} A_{1}\varphi _{2}^{0}+\displaystyle \sum _{i=1}^{q}B_{i1}(\varphi _{2}^{0}-\tau _{i}\varphi _{1}^{0}), \\ H_{3}= & {} A_{1}\varphi _{3}^{0}+ \displaystyle \sum _{i=1}^{q}B_{i1}\left( \varphi _{3}^{0}-\tau _{i}\varphi _{2}^{0} +\frac{1}{2}\tau _{i}^{2}\varphi _{1}^{0}\right) , \\ H_{4}= & {} \left( A_{2}+\displaystyle \sum _{i=1}^{q}B_{i2}\right) \varphi _{1}^{0}, \\ H_{5}= & {} A_{2}\varphi _{2}^{0}+\displaystyle \sum _{i=1}^{q}B_{i2}(\varphi _{2}^{0}-\tau _{i}\varphi _{1}^{0}), \\ H_{6}= & {} A_{2}\varphi _{3}^{0}+ \displaystyle \sum _{i=1}^{q}B_{i2}\left( \varphi _{3}^{0}-\tau _{i}\varphi _{2}^{0} +\frac{1}{2}\tau _{i}^{2}\varphi _{1}^{0}\right) , \\ H_{7}= & {} \left( A_{3}+\displaystyle \sum _{i=1}^{q}B_{i3}\right) \varphi _{1}^{0}, \\ H_{8}= & {} A_{3}\varphi _{2}^{0}+\displaystyle \sum _{i=1}^{q}B_{i3}\left( \varphi _{2}^{0}-\tau _{i}\varphi _{1}^{0}\right) , \\ H_{9}= & {} A_{3}\varphi _{3}^{0}+ \displaystyle \sum _{i=1}^{q}B_{i3}\left( \varphi _{3}^{0}-\tau _{i}\varphi _{2}^{0} +\frac{1}{2}\tau _{i}^{2}\varphi _{1}^{0}\right) , \\ H_{10}= & {} \displaystyle \sum _{j=1}^{n}\displaystyle \sum _{0\le k\le l\le q}D_{jkl}\varphi _{1}^{0}\varphi _{1j}^{0}, \\ H_{11}= & {} \displaystyle \sum _{j=1}^{n}\displaystyle \sum _{0\le k\le l\le q}D_{jkl}(\varphi _{2}^{0}-\tau _{l}\varphi _{1}^{0})(\varphi _{2j}^{0}-\tau _{k}\varphi _{1j}^{0}), \\ H_{12}= & {} \displaystyle \sum _{j=1}^{n}\displaystyle \sum _{0\le k\le l\le q}D_{jkl}\left( \varphi _{3}^{0}-\tau _{l}\varphi _{2}^{0}+\frac{1}{2}\tau _{l}^{2}\varphi _{1}^{0}\right) \left( \varphi _{3j}^{0}-\tau _{l}\varphi _{2j}^{0}+\frac{1}{2}\tau _{l}^{2}\varphi _{1j}^{0}\right) , \\ H_{13}= & {} \displaystyle \sum _{j=1}^{n}\displaystyle \sum _{0\le k\le l\le q}D_{jkl}((\varphi _{2}^{0}-\tau _{l}\varphi _{1}^{0})\varphi _{1j}^{0} + (\varphi _{2j}^{0}-\tau _{k}\varphi _{1j}^{0})\varphi _{1}^{0}), \\ H_{14}= & {} \displaystyle \sum _{j=1}^{n}\displaystyle \sum _{0\le k\le l\le q}D_{jkl}\left( \varphi _{1j}^{0}\left( \varphi _{3}^{0} - \tau _{l}\varphi _{2}^{0} + \frac{1}{2}\tau _{l}^{2}\varphi _{1}^{0}\right) + \varphi _{1}^{0}\left( \varphi _{3j}^{0} - \tau _{k}\varphi _{2j}^{0} + \frac{1}{2}\tau _{k}^{2}\varphi _{1j}^{0}\right) \right) . \end{aligned}$$