Introduction

For a long time, there has been a growing interest in teaching student-centered mathematics lessons. From a modern point of view, at least since the 1980s, such lessons are viewed from perspectives of mathematics and mathematics learning as inherently social activities (Cobb & Yackel, 1996; Schoenfeld, 1992), and involve students solving problems in their own personal way and communicating their various ideas regarding a specific problem (Cobb et al. 1993; Stein et al. 2008). Student-centered mathematics teaching comprises developing the students’ collective construction of mathematics by encouraging students to think, to articulate their thinking, and to discuss each other’s thinking in whole-class discussions.

Most mathematics teachers incorporate some student-centered elements into their lessons, but mathematics teaching is still predominantly “traditional”. In the Netherlands, most mathematics teachers generally rely on text-books (Blockhuis et al. 2016). These textbooks tend to present mathematics as a finished system. They neither support teachers in building on students’ personal ideas, nor in developing students’ mathematical thinking (Gravemeijer et al. 2016). Teacher reliance on such textbooks results in one-paragraph-per-lesson teaching: Teachers explain theory, demonstrate one solution method for a particular type of problem, and then students practice that method in several exercises.

Whole-class discussions are considered to be an important element of student-centered teaching, as can be inferred from a steadily increasing number of research papers as well as from a number of review studies (e.g., Herbel-Eisenmann et al. 2017; Ryve, 2011). For this study, we define whole-class discourse as whole-class conversations about students’ various solution methods and ideas that center around a particular mathematical problem or question. In line with Cohen’s (2011) description of discussions as a specific form of instructional discourse, we define whole-class discussions as whole-class discourse in which students actively engage with each other’s ideas. Other important aspects of mathematical whole-class discussions are: they are built on student thinking, everyone participates, the establishment of truth is based upon logical argumentation, and the class is guided towards certain mathematical practices and ideas (for an overview, see Walshaw & Anthony, 2008).

Orchestrating whole-class discussions is demanding for teachers. For example, particular social norms should be negotiated and established (Yackel & Cobb, 1996), teachers have to notice students’ mathematical thinking (for an introduction into mathematics teacher noticing, see Sherin et al. 2011), and teachers need to help students engage with each other’s thinking. A particularly challenging aspect of discourse-basedFootnote 1 teaching is how to achieve the right balance between building on students’ thinking on the one hand, and guiding them towards mathematical goals on the other (Sherin, 2002; Stein et al. 2008). Sherin (2002) describes one teacher’s development in discourse-based teaching as a “balancing act” as his attention shifts between the process of discussion and the mathematical content. Even highly skilled teachers struggle with listening to and building on student thinking while keeping an “eye on the mathematical horizon” (Ball, 1993). Stein et al. (2008) present the five practices model to support teachers in “orchestrating productive mathematical discussions” [emphasis added] that “build on student thinking and also advance important mathematical ideas” (p. 314). This model helps teachers prepare their work in orchestrating whole-class discussions, by “expanding the time to make instructional decisions from seconds to minutes (or even hours)” (p. 321). Nonetheless, especially in-the-moment decision-making that is required to connect teacher actions during interaction on the one hand with the development of student thinking on the other remains inherently complex (Scherrer & Stein, 2013).

Teacher efforts to build on their students’ thinking during whole-class discussions involve complex in-the-moment decision-making: Teachers need to listen to students’ articulated mathematics thinking, connect student thinking with their own mathematical thinking and with other students’ thinking, and decide to how to respond. Such interpretation and decision-making is closely connected to teacher conceptions such as knowledge and orientations. Research has yielded many insights into teacher conceptions, interpretation, and decision-making with regard to building on student thinking (see theoretical background below), but less is known about how these come together at specific moments in student-teacher interaction. Furthermore, experienced teachers’ novice attempts at orchestrating whole-class discourse have been understudied. We aim to make a contribution by investigating in-the-moment decision-making of five experienced mathematics teachers during their novice attempts at orchestrating whole-class discourse. Before providing the theoretical background for the study and introducing a conceptual model of teacher decision-making, we first present our research question:

How can we characterize teacher in-the-moment decision-making during novice attempts at orchestrating whole-class discourse with regard to building on student mathematical thinking?

Theoretical background

We are fundamentally interested in teacher in-the-moment decision-making during interaction with students in whole-class discourse, because although many teachers share the intention of fostering and building on student thinking, in practice, teachers find it difficult to sustain building whole-class discourse on student thinking (Nathan & Knuth, 2003; Sherin, 2002). Understanding teacher decision-making during interaction involves asking the questions: 1) what decisions do teachers make in interaction, and how do teacher actions facilitate building on student thinking?, 2) how do teachers make decisions?, and 3) why do teachers make these decisions? In this section, we will sketch what research in mathematics education has achieved with regard to these questions. First, we will elaborate on the outcomes of decision-making—teachers’ actions in interaction with their students—and how teacher actions support building on student thinking. Second, we will consider the process of decision-making and introduce our conceptual model. Third, we will go into the reasons why teachers make certain decisions—the role that underlying conceptions play.

Teacher actions that build on student thinking

Building on student thinking involves teacher actions that foster students’ engagement with instances of student mathematical thinking that are made public. Van Zoest et al. (2017) investigate instances of student mathematical thinking that have high potential and, “if made the object of discussion, [could] foster learners’ understanding of important mathematical ideas” (p. 33). They conceptualize building as taking advantage of these high-potential instances of student thinking. In the same line of research, Stockero et al. (2020) define the practice of building as “making an instance of student mathematical thinking the object of consideration by the class in order to engage the class in making sense of that thinking to better understand an important mathematical idea” (p. 239). In their conceptualization, student thinking must meet specific characteristics—be high-potential—in order to be able to build on it.

In our study, we deviate slightly from the conceptualization above. Especially in teachers’ novice attempts at discourse-based teaching, we regard any instance of student mathematical thinking that concerns the lesson’s problem as important and worthwhile to discuss publicly to some extent, as this supports students in the important goals of thinking, articulating their thinking, and engaging with each other’s thinking. For us, at the level of teacher actions during whole-class discourse, teachers build on student mathematical thinking if they take mathematics that a student has shared and keep it in the public space for further exploration and make sure that the class is engaging with it. To clarify our deviation from the earlier conceptualization, we adapt the definition by Stockero et al. (2020) above and define building on student mathematical thinking as making any “instance of student mathematical thinking [that concerns the lesson’s problem] the object of consideration by the class in order to engage the class in making sense of that thinking” (p. 239).

In this paragraph, we further elaborate our conceptualization of building on student thinking. During whole-class discussions, instances of student mathematical thinking can comprise utterances of a single word, questions, reactions, and even presentations of entire solution methods. These instances create an opportunity to build on student thinking, and teachers can take advantage of these opportunities in various ways. For example, teachers can probe for additional explanation or clarification, or ask other students to repeat, respond, or explain in their own words. In contrast, if the teacher moves on to the next idea or solution method without probing for reactions, the instance of student thinking is not built upon—even though it has been shared in whole-class discourse.

Two well-known types of whole-class discourse are important to discern here, because they involve teachers who aim to build on student thinking, but whose teacher actions do not fully contribute to such building. First, Stein et al. (2008) describe “show and tell” discourse, in which several students present their solution methods, but the teacher does not build on these students’ thinking by fostering other students’ engagement. Such whole-class discourse does little with regard to guiding the class toward important mathematical ideas (Stein et al. 2008). Second, the pattern of “initiation-response-evaluation” (IRE) as described by Mehan (1979), Cazden (1988), and many others, comprises: a teacher question, a student response, and a teacher evaluation of the response. In this classic form, IRE discourse involves limited building on student thinking. However, instead of evaluation, the third move can also serve different functions that do build on student thinking (Wells 1998). Teachers regularly use hybrid forms of IRE patterns, shifting between teacher moves that support more or less building on student thinking (Brodie 2011; Scott et al. 2006). Often, teachers initiate an elaboration to foster student explanation, while in subsequent actions they funnel the discourse toward specific answers (Imm and Stylianou 2012; Truxaw and DeFranco 2008). Hence, in many cases were teachers create opportunities to build on student thinking, they do not take full advantage of these opportunities. To find out why, we have to look further into the process of teacher in-the-moment decision-making.

Teacher decision-making: a model

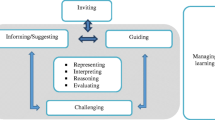
Roughly, teachers’ in-the-moment decisions are based upon two things: what goes on in their minds and what happens around them. To unpack the complexity of teachers’ in-the-moment decision-making with regard to building on student thinking, we constructed a conceptual model (see Fig. 1). In this model, we view teachers’ in-the-moment decision-making as a process that combines the teacher’s interpretation of student thinking with the teacher’s own conceptions, which results in a decision that determines how to react to student actions during interaction. The right side of the model depicts the student-teacher interaction that takes place during whole-class discourse, while the left side represents the situation-specific process of teacher decision-making that we aimed to approximate during a stimulated recall interview. In this section, we consider the process of teacher in-the-moment decision-making, and provide theoretical framing for three core concepts of the current study: teacher decision-making, teacher interpretation of student thinking, and teacher conceptions.

Fig. 1
figure 1

Model of a teacher's decision-making during interaction with students

Full size image

Our model of teacher decision-making is largely based upon three existing models, which are all focused—as is ours—on finding connections between what teachers know, believe, and want, and what teachers actually do. First, the model of teacher noticing by Jacobs et al. (2010): (1) attending to student mathematical thinking, (2) making sense of that thinking, and (3) deciding how to respond. This model has a clear focus on student mathematical thinking and helps to discern interpretation and decision-making, but does not include underlying conceptions. Second, Stahnke et al. (2016), adopt a model by Blömeke et al. (2015) of similar “skills”: perception,Footnote 2 interpretation, and decision-making, and place these “situation-specific skills” in between teacher dispositions and teacher “performance”. This model includes teacher conceptions, but does not separately discern their influence on interpretation and decisions. Some studies in their review do reveal strong connections between particular situation-specific skills and conceptions—primarily knowledge (Kersting et al. 2016; Norton et al. 2011). Third, Schoenfeld (2011) argues that people’s decision-making processes—including interpretation—can be modelled as a function of their orientations, goals, and resources. He underscores that teacher conceptions shape interpretation and decision-making, but this model does not have a specific place for student thinking, and on itself is too broad for our specific aim.

With our specific research purpose in mind—analyzing teacher in-the-moment decision-making with regard to building on student thinking in whole-class discourse, we combine aspects of the three models above and adopt the perspective that decisions are based upon interpretation of student thinking and on teacher conceptions and that teacher conceptions also shape interpretation.

By “interpreting student thinking”, we mean making sense of students’ articulated thinking. Teachers cannot “see” their students’ thoughts directly; they instead make mental models of students’ mathematics (Lesh & Lehrer, 2003; Steffe & Thompson, 2000) based upon what students say and do. Teacher interpretation of student thinking comprises at least: 1) taking the student’s perspective to figure out what the student could have been thinking, and 2) positioning the student’s thinking in one’s own conceptions of the relevant mathematics to figure out how to support development of the student’s thinking.

We define “teacher conceptions” as what goes on in a teacher’s mind that influences theirFootnote 3 response to classroom situations and is not an in-the-moment interpretation of classroom occurrences, such as student remarks. The word “conceptions” is used as a “general category containing constructs such as beliefs, knowledge, understanding, preferences, meanings, and views” (Leatham, 2006, p. 92). We deliberately choose this umbrella term because our aim is not to outline what different understandings, beliefs and goals teachers have, but how specific conceptions emerge and dominate during in-the-moment decision-making. Research in the field of mathematics education has shifted from static and cognitive conceptualizations toward more situation-dependent conceptualizations of conceptions (Depaepe et al. 2013; Stahnke et al. 2016; Zhang & Morselli, 2016). Our theoretical stance is one that places teacher conceptions neither solely in the teacher’s mind, nor solely in the social classroom context. Rather, we assume a complex relationship between teacher conceptions and the social classroom context: teacher conceptions have influence on interpretation and decision-making and thus, their actions, and vice versa, contextual features influence how certain conceptions dominate in the decision-making process. We view teachers’ conceptions as “sensible systems” (Leatham, 2006) and teachers as “complex, sensible people who have reasons for the many decisions they make” (p.100). Our aim is to investigate those reasons.

Why teachers make the decisions they make

Crucial to teachers’ instructional decision-making are their conceptions of mathematics. Two types of teacher conceptions—and their relation with teachers’ actions—have been widely studied: Beliefs and knowledge. Teacher beliefs with regard to the nature of mathematics and learning mathematics play a role in their in-the-moment decisions. For example, if a teacher views mathematics as a field in which content is “cut and dried” and does not provide opportunities for creative thought, this teacher is prone to teach students a single step-by-step solution method for each problem and unlikely to pay attention to different student solution methods (Thompson, 1984). Furthermore, teacher mathematical knowledge and teacher noticing—including interpretation and decision-making—are tied empirically and theoretically (e.g., Cengiz et al. 2011; Thomas et al. 2017). Research into teachers’ beliefs and knowledge with regard to mathematics and learning mathematics has brought much insight into teaching practice. However, for several reasons, this does not suffice to understand teachers’ in-the-moment decision-making.

First, in addition to conceptions of mathematics and learning mathematics, teacher decision-making also involves other types of conceptions that may dominate and result in specific decisions. For example, Skott (2001) shows how, at certain moments, beliefs with regard to teaching mathematics become less relevant for a teacher than his goal to make all students feel successful. Likewise, Thompson (1984) describes how a teacher’s concern for disruptive behavior sometimes overshadows conceptions of learning mathematics, and Thomas and Yoon (2014) show how contextual constraints and a teacher’s concerns for students’ affect result in teacher decisions that hinder building on student thinking. Hence, teachers’ conceptions of their students and of their own role as a teacher also shape their in-the-moment decision-making.

Secondly, teachers have conceptions with regard to orchestrating mathematical whole-class discourse. Sherin (2002) points to the influence of teacher beliefs with regard to the balance between process and content of mathematical whole-class discussions. Stockero et al. (2020) investigate teacher orientations toward building on student thinking in whole-class discussions and the extent to which these orientations support the teacher in such building. Orientations with high potential for building regard students as legitimate individual thinkers and underscore the importance of carefully exploring all students’ thinking and supporting discussion of student ideas (Stockero et al. 2020). The authors also identified orientations with some, but low, potential. These are aimed at supporting building, but in isolation do not provide enough support, such as orientations that probe the teacher to consider lesson goals during decision-making.

Thirdly, orchestrating whole-class discourse requires mathematical thinking, both during and before the lesson. On the one hand, teacher conceptions are not stable, and a teacher does not have access to all their relevant knowledge at all times. Teachers may demonstrate certain mathematical knowledge in one setting, but fail to demonstrate the same knowledge elsewhere (Hodgen, 2011). Knowing particular mathematics—at the time it is needed—requires activity from the teacher. In particular, being able to build on student thinking in whole-class discourse requires teachers to think mathematically—at the spot—with regard to the problem and its solution methods as well as with regard to their students’ mathematics. Teachers can prepare themselves mathematically for in-the-moment decision-making by anticipating what students will think and do and by trying to solve the lesson’s problem from their own and their students’ perspectives (Stein et al. 2008).

On the other hand, the teacher’s own conceptions of the problem and its solution methods do have a clear influence on the way they interpret student thinking. For example, teachers may “overhear” their own reasoning in students’ partial explanations, or “underhear” aspects of students’ solution methods that do not fit their own solution methods (Wallach & Even, 2005). Cengiz et al. (2011) describe how teacher Kasey endeavors building on her students’ thinking but struggles with attaining a collective mathematical argument for the last step of a solution method. In a post-lesson interview, Kasey’s own discussion of the last step of the solution method remained “somewhat vague” (p. 368) and the authors argue that, had she had access to more profound arguments, these would have supported her in helping her students reason through a mathematical argument.

To summarize, teachers’ own mathematical thinking with regard to the problem and its solution methods is crucial in their in-the-moment decision-making. In addition, more general conceptions with regard to teaching, mathematics, and students also shape their decision-making, and it is our aim to investigate how specific conceptions and interpretations emerge and interact at certain moments of decision-making.

This study

Previous case studies on the teacher’s work in discourse-based teaching and building on students’ thinking usually either involve teachers who are highly skilled or single out the teachers who were most successful in changing their practice (e.g., Leinhardt & Steele, 2005; Sherin, 2002). For example, Dyer and Sherin (2016) conceptualize teachers’ in-the-moment thinking similar to us, and identify two highly skilled teachers’ instructional reasoning about their interpretation of student thinking that could lead to responsive teacher actions. Jacobs et al. (2010) compare different groups of teachers with regard to the three components of noticing, and their findings assert novice-attemptersFootnote 4 as an interesting group to study on its own. All the more so, because teachers might give up on discourse-based teaching if they experience their attempts as unsuccessful (Smith 1996). Specific insights into why and how novice-attempters make the decisions they make can help professional development facilitators and teacher educators in supporting the intensive, ongoing, development that is required in learning to orchestrate whole-class discussions.

Teachers who aim to build on student thinking often create many opportunities to do so, but do not completely seize such opportunities, as in the “show and tell” and “IRE” patterns discussed above. In this study, we investigated the in-the-moment decision-making of five Dutch experienced mathematics teachers during their novice attempts at orchestrating whole-class discourse. By analyzing their decision-making involved in opportunities to build on student thinking that were not completely seized, we aim to contribute to research and practice concerning the orchestration of whole-class discussions.

As elaborated in the theoretical background, much is learnt about how teachers make decisions, what conceptions teachers have, and how whole-class discourse can build on student thinking. However, less is known about how all these aspects come together during actual in-the-moment decision-making. To illustrate this point: the review by Stahnke et al. (2016) includes only one study that investigates interpretation as well as decision-making (Dyer & Sherin, 2016). In this study, we aim to connect the different concepts in our model and contribute to a holistic view of teacher in-the-moment decision-making. Furthermore, previous studies often investigate teachers in situations away from their own teaching. By combining lesson observations with video-stimulated recall interviews, we aim to approximate the teachers’ actual in-the-moment decision-making where it matters: during interaction with their students.

Methods

This small-scale qualitative study comprises analysis of five teachers’ in-the-moment decision-making during whole-class discourse. Classroom video data and interview data were combined to analyze the teachers’ decision-making from two complementary perspectives, namely (see Fig. 1): (1) the actual teacher-student interaction during whole-class discourse, and (2) the teachers’ process of decision-making about their actions during interaction based upon their conceptions and interpretation of student thinking.

Data collection

To find participants, the principal researcherFootnote 5 organized a workshop entitled “Whole-class discourse in mathematics lessons”, and advertised among Dutch networks for mathematics teachers. For the teachers, enrollment was voluntary and comprised three components: participation in the four-hour workshop, orchestration of one discourse-based lesson—observed and video-recorded by the researcher—and reflection on the lesson in a subsequent interview.

During the workshop, the researcher orchestrated a group discussion with the participants modelled according to the five practices (Stein et al. 2008). The remainder of the workshop involved discussions focused on examples of discourse-based lessons: text excerpts taken from literature (Blanton et al. 2001; Hufferd-ackles et al. 2004), video recordings from a previous study (Authors 2019), and examples of problems taken from the researcher’s own teaching. The discussions revolved around “whys” and “hows” of orchestrating whole-class discussions. The “whys” involved the ideas that students construct their own ways of understanding, that student talk provides an entrance to their thinking, and that students collectively learn from each other’s perspectives. The “hows” involved preparation by means of finding a suitable problem and using the five practices, and how teachers’ actions can contribute to building on student thinking.

Participants

Five higher secondary school mathematics teachers from across the Netherlands participated in this study. In total, seven teachers participated in the workshop and the subsequent data-collection. Two of them were not included in this study because the observed lesson did not include whole-class discourse about students’ solution methods. The participants’ teaching experience varied from between less than five years (two teachers), to between 10 and 20 years (two teachers), and to more than 25 years (one teacher). Three of the teachers were in possession of first-degreeFootnote 6 teaching qualifications. The other two were in possession of second-degree teaching qualifications, and were studying to receive first-degree qualifications. Although the participating teachers all had experience teaching mathematics, they had little experience orchestrating whole-class discourse about students’ various ideas.

Preparation of lessons

After participating in the workshop, each individual teacher prepared a discourse-based mathematics lesson and invited the researcher to observe. They chose a lesson that fit into their program, so usually a lesson that comprised a single paragraph from the textbook. Instead of preparing an explanation of theory and some exercises for practice, they chose a single problem or task for the students to work on in groups, and they prepared whole-class discourse about the students’ solution methods. Most of the teachers utilized the five practices (Stein et al. 2008) in some way while fashioning their lessons. The teachers shared their preparation with the researcher, who provided feedback in the form of questions for the teachers to consider.

Observations and stimulated recall interviews

To study the teachers’ in-the-moment decision-making process, we combined two instruments for data-collection: (1) the lesson was observed and video-recordings were adopted to capture student-teacher interaction during whole-class discourse, and (2) stimulated recall interviews were adopted to investigate teacher decision-making during the interaction. As researchers, we do not have direct access to the in-the-moment decision-making process of teachers. Stimulated recall interviews are described as an instrument to approximate teachers’ “interactive cognitions” (Meijer et al. 2002, p. 150)—or in other words, what goes on in the teacher’s mind during teaching. We approximated the teachers’ in-the-moment decision-making by: interviewing each teacher at school—directly after the lesson, using stimulated recall to get close to moments of decision-making, and asking the teachers about what they were thinking at those moments.

The teacher and researcher watched the video together, with either of them pressing pause if they wanted to reflect on something. The researcher selected episodes that involved opportunities to build on student thinking. He asked the teacher about their decision-making in these instances by starting with the teacher and student actions—“What happened here?”, asking for interpretation—“What is the mathematics in this student’s statement”, asking for decision-making—“What were your considerations?”, and proceeding to underlying conceptions with questions such as “Why?” or “Why is that important?”. Other questions emerged during the interview. During the interviews, which lasted between 60 and 120 min, audio recordings were made for analysis. Together with the video recordings of whole-class discussions, these formed the data for this study.

Data preparation and analysis

The qualitative data analysis in this study involved several steps as listed below. These steps were concurrent processes, with analysis moving back and forth throughout the steps (Miles et al. 2014). The first and third authors were the main coders, while all authors discussed the analysis to reach a consensus in order to safeguard the overall quality.

Step 1: Transcripts and case documents

All audio recordings of interviews were transcribed verbatim. The audio recordings were linked to the interviews using Atlas.ti software, and linked to the relevant parts of the videos. For each teacher, we combined all essential data regarding teacher decision-making into a single case document. Each case document contains general information about the lesson, the problem that the students worked on, and the teacher’s goals for the lesson. The main element of the case document is a table that connects each lesson-episode that was reflected upon during the interview with its relevant interview fragments. In both columns, quotes are used to ground the descriptions in the data and to illustrate the descriptions. In Appendix A in Supplementary Information we have included a sample of a case document.

Step 2: Coding separate aspects of teacher decision-making

Each case document contained between 13 and 17 episodes, and these episodes comprised the units of analysis. The episodes were coded with regard to separate parts of our conceptual model. Student and teacher actions were identified separately in the lesson episodes. Interpretation, teacher conceptions, and decisions were identified in the interview fragments: they were either articulated by the teachers or inferred from their comments during the interview, as the teachers related to their actions during the lesson-episode. During the coding process, we repeatedly went back to the original transcripts or recordings for clarification and verification of the input of the case documents and the corresponding coding.

The coding of student-teacher interaction was performed mostly deductively, based on a previous study (Kooloos et al. 2020). The coding of interpretation and decisions was inductive, open coding: for each episode, we coded whether and how the teacher interpreted student thinking, and what decision they made. For teacher conceptions, we first labeled all specific conceptions that we identified in each episode. Then we categorized the conceptions in four categories, namely conceptions of; teaching, mathematics, students, and the relevant mathematics. Conceptions about the relevant mathematics are teachers’ personal thoughts about solving a problem, as well as their thoughts about the mathematics covered in the lesson and about their mathematical goals. Appendix B in Supplementary Information contains an overview of the conception coding, including examples derived from the data. The categories supported us in structuring the analysis and finding patterns among the episodes. For an overview, we developed a data table that, for each episode, contains separate cells for student-teacher interaction, conceptions, interpretation, decision, and additional contextual information.

Step 3: Identifying main patterns of decision-making

For each episode, we analyzed the teacher’s decision-making by identifying how the different constructs—coded in the preceding step and collected in the data table—related to each other. In a process of back-and-forth analysis of the data table and the case documents—and sometimes the original transcripts and recordings, we identified several patterns of decision-making. Four patterns were the most frequent, occurring either in all, or in all but one of the lessons. These four main patterns of decision-making concern critical moments in which the teachers had—in interaction with students—created opportunities to build on student thinking, but did not completely seize those opportunities. During their lessons, the teachers continually made decisions. Only some of those decisions were discussed in the interview, and some involved more (or less) building on student thinking. However, the main patterns concerned opportunities that were not completely seized, and these are elaborated in the results section.

To specify the conceptions involved in the four main patterns of teachers’ decision-making, we further analyzed all episodes associated with those pattern. Teacher conceptions of the relevant mathematics played a role in each pattern, although to a varying extent. With regard to the teachers’ conceptions of teaching, mathematics, and students, we identified two predominant themes in their conceptions that ran across these categories: steering to the mathematical lesson goals, and attaining student understanding. These findings will be elaborated in the results section.

Role of the researcher

The principle researcher in this study had several roles, apart from being the researcher. First, he was a coach in that he organized and facilitated the workshop that all teachers attended at the beginning of the study. In this way, he influenced teacher conceptions with regard to teaching discourse-based mathematics. However, through the workshop, he ensured that during data collection there would be actual whole-class discourse to capture, and they established a shared language about whole-class discourse. Second, he was a colleague-teacher with five years of experience. Such experience can lead to researcher-bias because of familiarity with the context (Lyle 2003). However, the experience also helped in establishing trust and understanding between the researcher and the teachers.

Results

In this section, we aim to answer our research question. First, we present our overall findings with regard to the teachers’ decision-making. In the main part of this section, we present data and identify the four main patterns of teacher decision-making. We present data gathered from two teachers, Jesse and Denise, because they provide a broad set of examples, and because these examples are exemplary for the particular patterns of decision-making as identified among the five teachers.

Overall findings

With regard to the right-hand side of our conceptual model, we found the whole-class discourse mainly comprised presentations of solution methods and conceptual elaborations. Identifying these two types of interaction helped us in organizing the analysis and the findings, and they are presented here to illustrate the overarching decision-making of the teachers during whole-class discourse before zooming in on their in-the-moment decision-making at particular instances.

Presentations of solution methods refers to students’ presentations of their solution methods to a specific mathematical problem. In general, each presenting student was able to present their complete solution method uninterrupted by questions or other interference. After a presentation was finished, the teacher generally did not foster other students’ engagement other than asking if they understood.

Conceptual elaboration refers to the teacher encouraging the class to discuss specific mathematical ideas. The interaction consisted of the teacher asking questions, with the students then responding to the question, and the teacher following up, either through expanding students’ replies, by pointing to a student, or by repeating the relevant question. In many instances, student responses were vague utterances like “perpendicular” or “Pythagoras”.

Both types of interaction do, to a certain extent, involve building on students’ mathematical thinking. The presenting students were given the opportunity to share their thinking with regard to their solution method, and at some instances, the student’s solution method was taken as the basis for a conceptual elaboration. Furthermore, during conceptual elaboration, the teachers encouraged students to give explanations and share their thought, and they refrained from explaining themselves. However, as could be expected regarding the novice nature of the teachers’ practices of whole-class discourse, the teachers often moved the discourse forward instead of fostering students’ engagement with each other’s thinking.

With regard to the left-hand side of our conceptual model, we found evidence that teachers’ decision-making is shaped by their own conceptions and on their interpretation of student thinking and that their conceptions also shape their interpretation. The decision-making of the teachers in this study was, to a certain extent, based upon conceptions that are in line with general ideas of student-centered teaching, including the ideas that “students must think mathematically” and “students should introduce important ideas and make connections”. Based on these conceptions, the teachers created many opportunities to build on student thinking. However, we found four main patterns of decision-making that resulted in opportunities to build on student thinking that were not completely seized. The teachers’ decision-making in these patterns was shaped by their conceptions of the relevant mathematics and by conceptions that centered around student understanding and lesson goals. These conceptions can roughly be summarized as “The lesson goals must be mentioned”, “Students need to understand the mathematics that is being discussed”, and “Students will indicate if they do not understand”.

Four patterns of decision-making

Here we present the four patterns of decision-making that comprise our main findings. For each pattern, we present: (i) a concise description of the decision-making pattern, (ii) an episode that illustrates an enactment of the decision-making pattern during teacher-student interaction, as captured on video, (iii) the decision-making as has been revealed during the interview, and (iv) an analysis of how the teacher’s decision-making has been based upon their personal conceptions and interpretation of student thinking. Table 1 provides contextual information regarding teachers Jesse’s and Denise’s lessons to understand the examples that are given to explain our main findings.

Table 1 Contextual information regarding two lessons
Full size table

Checking for student understanding

When a student presents their solution method, the teacher has an opportunity to build on the student’s thinking by getting others to engage with the solution method. In our data, the teachers often reacted to students’ presentations by asking the class whether everything was clear to them. In most cases, no one responded to these questions, and teachers decided to move the discourse forward. The following episode from Jesse’s lesson was an exception, because one student did respond by saying that it was not clear to her.

Jesse, episode 1

Thijs is the first student to present his solution method. He stands in front of the class and shows the steps he has taken, pointing to his drawing on the whiteboard (See Figure 2 for an illustration of the solution methods). After Thijs finishes his presentation, Jesse asks whether the method is clear to everyone. One student says it is not clear and that the presentation went by too quickly for her; Jesse then asks Thijs to repeat his explanation, and tells him to pay attention to his pace while doing so. Thijs shows his steps a second time. Jesse asks whether it is clear to everyone, and no one replies.

Fig. 2
figure 2

Illustration of solution methods

Full size image

During the interview, Jesse explained that he had wanted to make sure that everybody “understood” the method that was presented. “I decided to ask this question to check whether it was clear, and if students say it is clear enough, I am inclined to believe them”. He explained that he had not considered further engaging students with Thijs’ solution method. He just wanted to get to the conceptual elaboration of “perpendicular”, and he thought “that would fit perfectly after this first solution method. Then we have that out of the way”. When one student had said she did not understand the solution method, Jesse had considered asking a different student to explain the solution method the second time, but he did not want to make it too scholastic because, “What is important is that they understand it”, and he wanted to move on to the subsequent conceptual elaboration related to his mathematical goal for the lesson (see episode 2 below).

In this first episode, Jesse’s decision-making was based upon several conceptions concerning student understanding and mathematical goals: “The lesson goals must be mentioned”, “Students need to understand the mathematics that is being discussed”, “Students understand a solution method after one student’s presentation”, and “Students will indicate if they do not understand”. Jesse interpreted Thijs’ solution method as a correct method consisting of multiple correct steps. Jesse gave one of the steps—regarding “perpendicular”—specific attention because it was closely connected to one of his mathematical goals for the lesson. These conceptions and interpretation resulted in a decision to check for understanding and moving on. Jesse’s interpretation of the statement that the presentation went too quickly seems to be a literal acceptance of the statement. Together with the previously mentioned conceptions, this made him decide to ask Thijs to repeat his presentation but slower.

Quickly responding to a student comment with regard to a solution method

After some presentations of solution methods, a student responded by making a comment, such as “I do not agree”, or “Are those the same solution methods?”. Such comments provide the teacher with an opportunity to explore and build on the student’s thinking. The teachers decided not to explore such comments, and if they did respond, it was only in passing, as illustrated by the following example.

Jesse, episodeFootnote 7 3

Sarah is the second student to present her solution method. The method is: drawing a line through \(P\) perpendicular to \(l\), counting the squares, and using Pythagoras’ theorem. After her presentation, Jesse asks the class, “Is this method clear? Counting squares. Yes, clear?” Thom openly wonders: “Is that mathematical enough?” Jesse replies by saying, “This is definitely mathematical, but later I am going to ask you to do this without counting squares. Then you will not be able to use the grids”. Jesse starts a conceptual elaboration to generalize Sarah’s method of counting squares, consuming the remaining fifteen minutes of the lesson.

During the interview, Jesse confirmed his statement about the method being “mathematical enough” saying, “I do not see why it would not be”. Jesse said it was mathematically sound because “we use the ideal situation with those slopes which are nice numbers, and the point is also nice. That is why we can count squares”. He had not considered asking other students to respond to either Sarah’s solution method or to Thom’s wondering whether it was “mathematical enough”, because he wanted to “steer to the slope, perpendicular”, i.e., his mathematical goal.

In this episode, the mathematical argumentation underlying Sarah’s solution method is not made explicit, and the student comment, “Is this mathematical enough?” seems like a genuine question. In fact, Sarah said she “drew” the perpendicular line, so her method may have relied on careful drawing instead of mathematical reasoning. Thom’s wondering could have been taken as a starting point for a discussion of Sarah’s thinking and the mathematics involved in her method. Moreover, such a discussion could have been a good preparation to move on to generalizing the method and discussing how slopes of perpendicular lines relate to each other. Jesse did start a conversation to generalize the method, but he did not first discuss the mathematics involved in Sarah’s method, nor did he explore Thom’s wondering. Jesse’s own description of the mathematics involved in Sarah’s solution method, “We use the ideal situation with those slopes which are nice numbers, and the point is also nice. That is why we can count squares” did not involve an explicit mathematical argumentation. He did not refer to mathematical concepts such as rotations or congruence of triangles. So it seems that Jesse’s own conception of the relevant mathematics made him interpret Sarah’s explanation as correct and “mathematical enough”. In addition, his conceptions of teaching urged him to move the discourse to a general method related to his lesson goal. This resulted in a decision not to further explore Thom’s wondering.

Confirming a student utterance as a concluding argument

During conceptual elaboration, the teachers were trying to achieve their mathematical goals during whole-class discourse about certain aspects of presented solution methods. In these elaborations, they struggled with balancing different conceptions of teaching that can be summarized as: a) “Students should introduce important ideas and make connections” and b) “The lesson goals must be mentioned”. In several conceptual elaborations, students made incomplete or incorrect statements related to the teacher’s goal, which provides an opportunity to explore and build on their thinking. In several cases, however, the teacher decided to confirm a statement that was incomplete or incorrect. The following is an example from Jesse’s lesson. This conceptual elaboration took place after Thijs’ presentation of his solution method, and before Sarah’s presentation.

Jesse, episode 2

After Thijs’ second presentation of his solution method, Jesse initiates a conceptual elaboration to discuss why the shortest distance from a point to a line is “perpendicular”, one of his two main goals for the lesson. First, he points out what he regards as an important aspect of Thijs’ method, namely “something with shortest distance”, and a student calls out “perpendicular”. What follows is a sequence of teacher questions and student responses concerning why not going perpendicular will not yield a shorter distance, in which Jesse keeps probing for explanations:

Jesse: “Why is that?”

Eric:“It’s longer”

Jesse:“Why?”

Britta:“Diagonal line”

Jesse: “Continue”

Terry:“More centimeters”

Jesse asks, “Can we explain mathematically why it’s longer?” A student replies, “Pythagoras”, and Jesse concludes, “Pythagoras’ theorem. That is why you have to go perpendicular”.

During the interview, Jesse explained that he had considered elaborating further on the argumentation of “Pythagoras” in reference to why the perpendicular distance is the correct distance, “but it seemed so obvious that I did not think it was necessary”. He reckoned that all his students had understood the argumentation: “‘Because the diagonal line is longer,’ said Britta. That seemed logical to me”, and “the diagonal line is always longer because it is the addition of the two other lines”.

In this episode, Jesse decided to confirm “Pythagoras” as a concluding argument. This can be regarded as a key moment of the discourse, because it concerned one of Jesse’s mathematical goals for the lesson. Nevertheless, the mathematical argumentation did not get articulated clearly. Jesse’s conception of the relevant mathematics comprised that Pythagoras’ theorem was a sufficient argument for the claim that the perpendicular way is the shortest way, because “the diagonal line is always longer because it is the addition of the other two other lines” (which it is not, rather its square is the addition of the squares of the other two sides). Jesse did not make the mathematical argumentation explicit for himself; therefore his conception of the relevant mathematics was somewhat intuitive. His reckoning that all students understood the argumentation may partly have been an interpretation of their body language or facial expression. However, it seems mainly based on his own conceptions of the relevant mathematics that he regards as “logical” and a conception of students that they think in the same way as he does. Based on his own conception of the relevant mathematics, Jesse interpreted Britta’s partial explanation, “diagonal line” as “Because the diagonal line is always longer”, and he interpreted the partial explanation “Pythagoras” as a correct concluding argument. Based on these conceptions and interpretations, he made a deliberate decision to accept “Pythagoras” as a sufficient argument for the claim.

Probing for explanation in the teacher’s own line of thinking

During conceptual elaboration, the teachers sometimes did not build on specific student utterances that could have been regarded as opportunities because they did not realize that these comments were correct or at least worth discussing. This was mainly due to the teachers’ own conceptions of the relevant mathematics which in turn shaped their interpretation of student thinking.

Denise, episode 1

Two similar solutions methods are being discussed. Both methods make use of repeated drawings without replacements. One solution method is complete and correct, and the other solution method uses the incorrect combinations. The first method correctly uses \(\left(\begin{array}{c}6\\ 1\end{array}\right), \left(\begin{array}{c}6\\ 2\end{array}\right)\), \(\left(\begin{array}{c}6\\ 3\end{array}\right), \left(\begin{array}{c}6\\ 4\end{array}\right)\), and \(\left(\begin{array}{c}6\\ 5\end{array}\right)\), and the second solution method repeatedly uses \(\left(\begin{array}{c}20\\ 6\end{array}\right)\). Denise has declared the first solution method as correct, and she probes students to explain why it is correct.

Wessel: “Out of six doors you can get one price, and the other five, you can put them anywhere”.

Denise: “How do the probabilities show that?”.

Wessel: “Oh, there are six numbers!”

Denise: “Yes, but that is the same in the other method”.

Denise: “Maeve, what is your idea?”

Maeve: “There are six doors, but it does not necessarily have to be the first. They could all be it”.

Denise: “Uh yes… And if I look at those probabilities, six choose one is the number of sequences. Number of sequences why? In what?”

During the interview, Denise realized that Wessel and Maeve both contributed fruitfully to the conceptual elaboration at hand. “It only comes through now, that they both said the right things”. “I did hear them, but that is not the line of thinking in my mind. In my mind it was that the probabilities can be in different sequences”. In hindsight, Denise thought she should have built on the students’ thinking: “I went on too fast. I did not let their words come through in my mind”.

In this episode, Wessel and Maeve both gave partial explanations involving the idea of choosing doors in different sequences. Denise decided to react to both students by probing for an explanation in another representation by referring to the formulas in the solution method. Denise’s own conception of the relevant mathematics focused on the sequences of probabilities and was based upon algebra, whereas her students’ statements focused on the contextual features of the problem. This interfered with her interpretation of her students’ thinking. It seems that Denise’s conceptions of mathematics presupposed that mathematics is the correct use of formal language, and that Denise’s conceptions of her students’ presupposed that their understanding was similar to her own. Together with Denise’s conceptions of the relevant mathematics, this resulted in a limited interpretation of the students’ thinking and a disconnect between student utterances and teacher reactions.

Discussion

The aim of this study was to investigate in-the-moment decision-making of five experienced mathematics teachers during their novice attempts at orchestrating whole-class discourse. By getting students to think and talk, the teachers created many moments of decision-making for themselves. Not surprisingly, at particular moments, teacher decision-making did not take full advantage of opportunities to build on student thinking. The whole-class discourse in these lessons was a hybrid form, comprising mostly presentations of solution methods and conceptual elaborations initiated by the teacher. In this sense, the whole-class discourse combined elements of “show and tell” (Stein et al. 2008) and of “IRE” patterns in which the teacher eventually funneled toward their lesson goals (Imm and Stylianou 2012; Truxaw and DeFranco 2008).

Our analysis of four main patterns of decision-making contributes to unpacking the complexity of teacher in-the-moment decision-making. Our model (Fig. 1) appears to be suitable for understanding teacher in-the moment decision-making with regard to building on student thinking. Through conceptually extricating interpretation and decisions and then connecting them to teacher conceptions and teacher-student interactions, we have gained new insights into how teachers’ conceptions, interpretation, and decision-making interrelate and influence student-teacher interaction.

We found that the teachers’ interpretation and decision-making was largely based on two types of teacher conceptions: 1) conceptions that centered around the ideas of “getting to” their mathematical lesson goals and achieving student “understanding”, and 2) conceptions of the relevant mathematics. Our results show that at several critical moments, the teachers felt urged to move the discourse forward to their goals. “Understanding” was regarded as pivotal and as a prerequisite to move on. They checked by asking the students whether they understood, based on conceptions that students know “whether” they “understand” and that students will speak up if they do not understand.

Furthermore, the teachers’ interpretation of student thinking was always in line with their own conceptions of the relevant mathematics: recognizing student thinking that matched their own conceptions of the solution methods or that connected to their own conceptions of the mathematical goals. This is in line with findings by Wallach and Even (2005), who show that a teacher’s own conceptions of solution methods shapes their interpretation of student thinking. The teachers’ own conceptions of the relevant mathematics seemed, at times, inadequate to make decisions to guide the discourse toward the lesson goals while also maintaining building on student thinking. Cengiz et al. (2011) also describe how that a teacher’s own explanation of a solution method lacked clarity, and how this impeded her in supporting her students’ thinking during whole-class discourse.

Explanations

Taking a perspective on teacher conceptions—and decision-making—as “sensible systems” (Leatham, 2006) helps to further explain our findings. Our findings show that teachers’ conceptions that guided their decision-making were centered around mathematical goals and student understanding. This is not surprising, because helping students understand mathematics can be seen as the mathematics teacher’s main task, and working toward specific goals is part of every teacher’s practice. Not only are the teachers’ various conceptions with regard to teaching, mathematics, and students reasonable, these conceptions also form a coherent system. For example, if students should understand the mathematical goal, and students will speak up if they do not understand, than “mentioning” the mathematical goal seems sufficient. Furthermore, the teacher conceptions of the relevant mathematics made explicit in the interviews were at the level of utterances that were expected from students, so these sufficed to recognize whether students “understood” and whether the lesson goals were mentioned. Thus, the identified teacher conceptions formed a coherent system, and their decision-making was completely reasonable.

Our first explanation for why teachers’ decision-making—although completely reasonable—did not always result in building on student thinking, involves their novelty in orchestrating whole-class discourse. Based on their experience in teaching, they were used to rely on a well-established system of teacher conceptions. Based on their novelty in whole-class discourse, their conceptions that have high potential with regard to building on student thinking were probably not firmly connected to their existing system of conceptions and may have formed a somewhat isolated system (Leatham, 2006). Thomas and Yoon (2014) describe how, even a teacher’s strong conceptions regarding student-centered teaching can be sidelined by a well-connected system of conceptions to relieve experienced tensions in the system. To illustrate this explanation: it seems that Jesse’s new conception with regard to whole-class discourse, that “students should introduce important ideas and make connections”, led him to probe students to share their ideas about the “shortest distance”, but his established system of conceptions led him to confirm an incomplete utterance to relieve the tension of eagerly probing students to say the right thing.

A second explanation involves the teachers’ conceptions with regard to student understanding and mathematical goals. Stockero et al. (2020) argue that teacher orientations suggesting student understanding must be checked and lesson goals must be considered are low-potential: in isolation they provide limited support to build on student thinking. The teachers in our study put great emphasis on understanding and goals. However, their conceptions of these ideas did simply not require building on student thinking in the sense that we conceptualized it. The teachers seemed to conceive student understanding as similar to their own understanding, and as something students have or not. If, in addition, they conceive students as being able and inclined to say whether they do understand, then there is little incentive for encouraging the class to engage with a presented solution method. If, in addition, teachers conceptions regarding goals comprise that these goals must be mentioned, then there is no reason to encourage students to articulate their thinking with regard to the mathematical goal once it has been mentioned. Being more explicit about what understanding means or what it means to achieve a mathematical goal seemed, for these teachers, either unnecessary or too big a challenge. Furthermore, being specific about student understanding and mathematical goals requires being explicit about the relevant mathematics, which brings us to our third explanation.

A third explanation concerns teachers’ conceptions of the relevant mathematics. These conceptions remained, at critical moments, at the level of what they expected of their students. They did not have more explicit mathematical argumentation or connections at their disposal as a model for further developing student thinking. This resulted in limited interpretation of student thinking. Furthermore, their conceptions of solution methods and goals as revealed during the interviews sometimes lacked mathematical language or argumentation, and made them settle for student presentations and utterances with limited mathematical language or argumentation. This explanation is closely connected to the preceding one: to have a clear mathematical goal requires ideas of how to develop students’ various ideas toward that goal. Furthermore, building on student thinking requires insight into “how”—instead of “whether”—students understand the mathematics. Such insight requires explicit conceptions of the relevant mathematics, or “unpacked” mathematical knowledge (Ball et al. 2008).

Our fourth explanation lies in the nature of the teachers’ conceptions of the relevant mathematics. For us, teacher conceptions of the relevant mathematics comprises teacher mathematical thinking, and thus, can be seen as an activity. It seems that the teachers regarded their conceptions of the relevant mathematics as static “knowledge”. They did not seem inclined to think mathematically at the spot if a student shared an idea. Rather, the teachers recognized whether the student idea fit their own conceptions, and these conceptions were mostly formed before the lesson. In other words, the teachers did not take an inquiry stance towards the mathematics and students’ thinking. Such an inquiry stance comprises an inclination to think mathematically—before and also during whole-class discourse—and seems to be a prerequisite for building on student thinking.

Limitations of the study

In addition to well-known limitations inherent in all small-scale qualitative research, some limitations with regard to the method and the model adopted should be taken into account when evaluating the results of this study. First, we are aware that the social environment and the students play a crucial role in student-teacher interaction in whole-class discourse. However, our conceptual model focusses on the teacher’s individual conceptions, interpretation, and decision-making, and student mathematical thinking is the only influence from outside the teacher included in the model.

Second, our method does not reveal the teachers’ actual decision-making process as taking place in student-teacher interaction, but only their reflections in hindsight and as probed by the researcher. Evidently, during the watching of an episode, the teachers’ thoughts are different than in the moment of the actual decision-making during the episode, and they have time to develop new thoughts (Yinger 1986). Moreover, our analysis proposes a reflective decision-making process, although much of the teachers’ behavior could be routine-based (Mason 2016). If teachers’ decision-making is partly tacit or routinized, they will probably not have access to all details of their decision-making. What our analysis does provide is an approximation of teachers’ decision-making that does account for the teachers’ own reflective view on their decision-making and does presents explanations for what happens at particular critical moments.

Finally, during the interview we did aim to draw out teachers’ decision-making, conceptions and interpretations. However, our model that connects the different constructs was finalized later, during analysis. Adopting the finalized model may have supported the interviewer in probing for more articulation of how the different constructs relate.

Implications for research and practice

Our findings and the presented explanations of our findings give rise to several implications for research and practice.

First, teachers’ conceptions regarding understanding and goals were of great importance for their decision-making, but provided limited support for building on student thinking. This implies that teacher educatorsFootnote 8 should support teachers in reflecting on what they mean by understanding and by mathematical goals, how goals can be formulated, and how understanding and the achievement of goals can be recognized in students’ articulated thinking. Such deepening and expanding of the teachers’ existing system of conceptions may lead to changes in their decision-making. Further research is needed to investigate how teachers’ conceptions with regard to understanding and goals can develop with experience in discourse-based teaching, and in connection to their decision-making.

Second, teachers’ conceptions of the relevant mathematics seemed limited in their support of interpreting and building on student thinking during in-the-moment decision-making. This calls for further extension of the field of how teachers’ conceptions of the relevant mathematics play a role in their in-the-moment interpretation and decision-making. Teacher educators can take our findings into account and support teachers in expanding their mathematical conceptions with regard to the mathematics that they aim to teach. For example, by collectively articulating the mathematical argumentation that underlies (steps) of solution methods and the connections between solution methods.

Third, it seems that teachers regard their own mathematical conceptions as static, instead of as an activity. In research too, teachers’ mathematical conceptions are often framed as more or less static “knowledge”. We think reframing the teachers’ mathematical conceptions that play a role in their decision-making as “teacher mathematical thinking” can support teachers in taking an inquiry stance toward the mathematics, which could support their interpretation of student thinking. Teacher educators may contribute to this by focusing on teachers’ as well as students’ mathematical thinking involved in teaching a lesson. Both the fields of research and practice would benefit from a theoretical conceptualization of teacher mathematical thinking and empirical studies to ground this in practice.

In addition, the method that we used, including reflection on decision-making during specific moments in a lesson the teacher had just taught, seems promising in developing teachers’ awareness of their decision-making. The teachers experienced their reflection during the interviews as very rich learning activities. Such activities could also be taken up with colleagues to learn from each other’s teaching and from each other’s students.

Conclusions

Whole-class discussions that build on student ideas are an important aspect of teaching students mathematical thinking, but underrepresented in teaching practice. Our investigation into teachers’ in-the-moment decision-making during their novice-attempts at orchestrating whole-class discourse sheds light on the complexity of the teacher’s work in building on student thinking. Our findings reveal patterns of decision-making that—however reasonable—result in missed opportunities with regard to building on student thinking. Teachers’ interpretation of student thinking was always in line with the teachers’ own conceptions of the relevant mathematics, and their decision-making was based on these interpretations and on teacher conceptions that center around student understanding and mathematical goals. During critical moments, these teacher conceptions were not explicit enough to build on student thinking. More explicit conceptions of what student understanding comprises and of what it means to achieve mathematical goals with students may enable teachers to maintain building on student thinking. This goes hand in hand with more explicit teacher conceptions of the mathematics involved in a lesson, and thus with teacher mathematical thinking. A next step in research and practice concerning the orchestration of whole-class discourse that builds on student ideas lies in elaborating on the role of teachers’ mathematical thinking.